Hirsch M., Smale S., Devaney R., Differential Equations, Dynamical Systems, and an Introduction to Chaos
Подождите немного. Документ загружается.

This Page Intentionally Left Blank

13
Applications in Mechanics
We turn our attention in this chapter to the earliest important examples of
differential equations that, in fact, are connected with the origins of calculus.
These equations were used by Newton to derive and unify the three laws of
Kepler. In this chapter we give a brief derivation of two of Kepler’s laws and
then discuss more general problems in mechanics.
The equations of Newton, our starting point, have retained importance
throughout the history of modern physics and lie at the root of that part of
physics called classical mechanics. The examples here provide us with concrete
examples of historical and scientific importance. Furthermore, the case we
consider most thoroughly here, that of a particle moving in a central force
gravitational field, is simple enough so that the differential equations can be
solved explicitly using exact, classical methods (just calculus!). However, with
an eye toward the more complicated mechanical systems that cannot be solved
in this way, we also describe a more geometric approach to this problem.
13.1 Newton’s Second Law
We will be working with a particle moving in a force field F . Mathematically, F
is just a vector field on the (configuration) space of the particle, which in our
case will be
R
n
. From the physical point of view, F (X) is the force exerted on
a particle located at position X ∈
R
n
.
The example of a force field we will be most concerned with is the gravita-
tional field of the sun: F (X) is the force on a particle located at X that attracts
the particle to the sun. We go into details of this system in Section 13.3.
277

278 Chapter 13 Applications in Mechanics
The connection between the physical concept of a force field and the math-
ematical concept of a differential equation is Newton’s second law: F = ma.
This law asserts that a particle in a force field moves in such a way that the
force vector at the location X of the particle, at any instant, equals the acceler-
ation vector of the particle times the mass m. That is, Newton’s law gives the
second-order differential equation
mX
= F(X).
As a system, this equation becomes
X
= V
V
=
1
m
F(X )
where V = V (t) is the velocity of the particle. This is a system of equations on
R
n
×R
n
. This type of system is often called a mechanical system with n degrees
of freedom.
A solution X(t ) ⊂
R
n
of the second-order equation is said to lie in config-
uration space. The solution of the system (X (t ), V (t )) ⊂
R
n
× R
n
lies in the
phase space or state space of the system.
Example. Recall the simple undamped harmonic oscillator from Chapter 2.
In this case the mass moves in one dimension and its position at time t is given
by a function x(t ), where x :
R → R. As we saw, the differential equation
governing this motion is
mx
=−kx
for some constant k>0. That is, the force field at the point x ∈
R is given
by −kx.
Example. The two-dimensional version of the harmonic oscillator allows
the mass to move in the plane, so the position is now given by the vector
X(t ) = (x
1
(t), x
2
(t)) ∈ R
2
. As in the one-dimensional case, the force field is
F(X ) =−kX so the equations of motion are the same
mX
=−kX
with solutions in configuration space given by
x
1
(t) = c
1
cos(
√
k/mt) + c
2
sin(
√
k/mt)
x
2
(t) = c
3
cos(
√
k/mt) + c
4
sin(
√
k/mt)
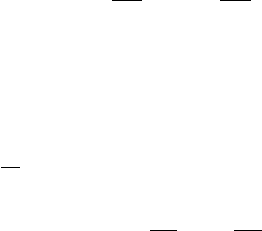
13.1 Newton’s Second Law 279
for some choices of the c
j
as is easily checked using the methods of
Chapter 6.
Before dealing with more complicated cases of Newton’s law, we need to
recall a few concepts from multivariable calculus. Recall that the dot product
(or inner product ) of two vectors X, Y ∈
R
n
is denoted by X ·Y and defined by
X · Y =
n
i=1
x
i
y
i
where X = (x
1
, ..., x
n
) and Y = (y
1
, ..., y
n
). Thus X ·X =|X |
2
.IfX, Y : I →
R
n
are smooth functions, then a version of the product rule yields
(X · Y )
= X
· Y + X · Y
,
as can be easily checked using the coordinate functions x
i
and y
i
.
Recall also that if g :
R
n
→ R, the gradient of g, denoted grad g ,is
defined by
grad g (X) =
∂g
∂x
1
(X), ...,
∂g
∂x
n
(X)
.
As we saw in Chapter 9, grad g is a vector field on
R
n
.
Next, consider the composition of two smooth functions g ◦ F where
F :
R → R
n
and g : R
n
→ R. The chain rule applied to g ◦ F yields
d
dt
g (F(t )) = grad g (F(t )) · F
(t)
=
n
i=1
∂g
∂x
i
(F(t ))
dF
i
dt
(t).
We will also use the cross product (or vector product) U × V of vectors
U , V ∈
R
3
. By definition,
U × V =
(
u
2
v
3
− u
3
v
2
, u
3
v
1
− u
1
v
3
, u
1
v
2
− u
2
v
1
)
∈
R
3
.
Recall from multivariable calculus that we have
U × V =−V × U =|U ||V |N sin θ
where N is a unit vector perpendicular to U and V with the orientations of
the vectors U , V , and N given by the “right-hand rule.” Here θ is the angle
between U and V .

280 Chapter 13 Applications in Mechanics
Note that U ×V = 0 if and only if one vector is a scalar multiple of the other.
Also, if U × V = 0, then U × V is perpendicular to the plane containing U
and V .IfU and V are functions of t in
R, then another version of the product
rule asserts that
d
dt
(U × V ) = U
× V + U × V
as one can again check by using coordinates.
13.2 Conservative Systems
Many force fields appearing in physics arise in the following way. There is a
smooth function U :
R
n
→ R such that
F(X ) =−
∂U
∂x
1
(X),
∂U
∂x
2
(X), ...,
∂U
∂x
n
(X)
=−grad U (X).
(The negative sign is traditional.) Such a force field is called conservative. The
associated system of differential equations
X
= V
V
=−
1
m
grad U (X )
is called a conservative system. The function U is called the potential energy
of the system. [More properly, U should be called a potential energy since
adding a constant to it does not change the force field −grad U (X).]
Example. The planar harmonic oscillator above corresponds to the force
field F (X) =−kX . This field is conservative, with potential energy
U (X ) =
1
2
k |X|
2
.
For any moving particle X (t) of mass m, the kinetic energy is defined to be
K =
1
2
m|V (t )|
2
.
Note that the kinetic energy depends on velocity, while the potential energy
is a function of position. The total energy (or sometimes simply energy)is
defined on phase space by E = K +U . The total energy function is important
13.3 Central Force Fields 281
in mechanics because it is constant along any solution curve of the system.
That is, in the language of Section 9.4, E is a constant of the motion or a first
integral for the flow.
Theorem. (Conservation of Energy) Let (X (t ), V (t )) be a solution curve
of a conservative system. Then the total energy E is constant along this solution
curve.
Proof: To show that E(X(t )) is constant in t , we compute
˙
E =
d
dt
1
2
m|V (t )|
2
+ U (X(t ))
= mV · V
+ (grad U ) · X
= V · (−grad U ) + (grad U ) · V
= 0.
We remark that we may also write this type of system in Hamiltonian form.
Recall from Chapter 9 that a Hamiltonian system on
R
n
× R
n
is a system of
the form
x
i
=
∂H
∂y
i
y
i
=−
∂H
∂x
i
where H : R
n
× R
n
→ R is the Hamiltonian function. As we have seen, the
function H is constant along solutions of such a system. To write the conser-
vative system in Hamiltonian form, we make a simple change of variables. We
introduce the momentum vector Y = mV and then set
H = K + U =
1
2m
y
2
i
+ U (x
1
, ..., x
n
).
This puts the conservative system in Hamiltonian form, as is easily checked.
13.3 Central Force Fields
A force field F is called central if F(X) points directly toward or away from the
origin for every X. In other words, the vector F(X ) is always a scalar multiple
of X :
F(X ) = λ(X )X
282 Chapter 13 Applications in Mechanics
where the coefficient λ(X) depends on X. We often tacitly exclude from con-
sideration a particle at the origin; many central force fields are not defined
(or are “infinite”) at the origin. We deal with these types of singularities in
Section 13.7. Theoretically, the function λ(X) could vary for different values
of X on a sphere given by |X|=constant. However, if the force field is
conservative, this is not the case.
Proposition. Let F be a conservative force field. Then the following statements
are equivalent:
1. F is central;
2. F(X) = f (|X|)X;
3. F(X) =−grad U (X ) and U (X ) = g (|X |).
Proof: Suppose (3) is true. To prove (2) we find, from the chain rule:
∂U
∂x
j
= g
(|X|)
∂
∂x
j
x
1
2
+ x
2
2
+ x
3
2
1/2
=
g
(|X|)
|X|
x
j
.
This proves (2) with f (|X|) =−g
(|X|)/|X |. It is clear that (2) implies (1). To
show that (1) implies (3) we must prove that U is constant on each sphere
S
α
={X ∈ R
n
||X|=α > 0}.
Because any two points in
S
α
can be connected by a curve in S
α
, it suffices
to show that U is constant on any curve in
S
α
. Hence if J ⊂ R is an interval
and γ : J →
S
α
is a smooth curve, we must show that the derivative of the
composition U ◦ γ is identically 0. This derivative is
d
dt
U (γ (t )) = grad U (γ (t )) · γ
(t)
as in Section 13.1. Now grad U (X) =−F(X) =−λ(X )X since F is central.
Thus we have
d
dt
U (γ (t )) =−λ(γ (t ))γ (t ) · γ
(t)
=−
λ(γ (t ))
2
d
dt
|
γ (t )
|
2
= 0
because |γ (t)|≡α.

13.3 Central Force Fields 283
Consider now a central force field, not necessarily conservative, defined on
R
3
. Suppose that, at some time t
0
, P ⊂ R
3
denotes the plane containing the
position vector X (t
0
), the velocity vector V (t
0
), and the origin (assuming, for
the moment, that the position and velocity vectors are not collinear). Note
that the force vector F(X (t
0
)) also lies in P. This makes it plausible that the
particle stays in the plane
P for all time. In fact, this is true:
Proposition. A particle moving in a central force field in
R
3
always moves in
a fixed plane containing the origin.
Proof: Suppose X (t ) is the path of a particle moving under the influence of
a central force field. We have
d
dt
(X × V ) = V × V + X × V
= X × X
= 0
because X
is a scalar multiple of X . Therefore Y = X(t) ×V (t ) is a constant
vector. If Y = 0, this means that X and V always lie in the plane orthogonal
to Y , as asserted. If Y = 0, then X
(t) = g (t )X(t) for some real function g (t).
This means that the velocity vector of the moving particle is always directed
along the line through the origin and the particle, as is the force on the particle.
This implies that the particle always moves along the same line through the
origin. To prove this, let (x
1
(t), x
2
(t), x
3
(t)) be the coordinates of X(t). Then
we have three separable differential equations:
dx
k
dt
= g (t )x
k
(t), for k = 1, 2, 3.
Integrating, we find
x
k
(t) = e
h(t)
x
k
(0), where h(t ) =
t
0
g (s) ds.
Therefore X(t) is always a scalar multiple of X(0) and so X (t ) moves in a fixed
line and hence in a fixed plane.
The vector m(X ×V ) is called the angular momentum of the system, where
m is the mass of the particle. By the proof of the preceding proposition, this
vector is also conserved by the system.
Corollary. (Conservation of Angular Momentum) Angular momentum
is constant along any solution curve in a central force field.

284 Chapter 13 Applications in Mechanics
We now restrict attention to a conservative central force field. Because of
the previous proposition, the particle remains for all time in a plane, which we
may take to be x
3
= 0. In this case angular momentum is given by the vector
(0, 0, m(x
1
v
2
− x
2
v
1
)). Let
= m(x
1
v
2
− x
2
v
1
).
So the function is also constant along solutions. In the planar case we also
call the angular momentum. Introducing polar coordinates x
1
= r cos θ and
x
2
= r sin θ,wefind
v
1
= x
1
= r
cos θ − r sin θθ
v
2
= x
2
= r
sin θ + r cos θθ
.
Then
x
1
v
2
− x
2
v
1
= r cos θ(r
sin θ + r cos θθ
) − r sin θ (r
cos θ − r sin θθ
)
= r
2
(cos
2
θ + sin
2
θ)θ
= r
2
θ
.
Hence in polar coordinates, = mr
2
θ
.
We can now prove one of Kepler’s laws. Let A(t) denote the area swept
out by the vector X (t ) in the time from t
0
to t. In polar coordinates we have
dA =
1
2
r
2
dθ . We define the areal velocity to be
A
(t) =
1
2
r
2
(t)θ
(t),
the rate at which the position vector sweeps out area. Kepler observed that the
line segment joining a planet to the sun sweeps out equal areas in equal times,
which we interpret to mean A
= constant. We have therefore proved more
generally that this is true for any particle moving in a conservative central force
field.
We now have found two constants of the motion or first integrals for a
conservative system generated by a central force field: total energy and angular
momentum. In the 19th century, the idea of solving a differential equation
was tied to the construction of a sufficient number of such constants of the
motion. In the 20th century, it became apparent that first integrals do not exist
for differential equations very generally; the culprit here is chaos, which we
will discuss in the next two chapters. Basically, chaotic behavior of solutions of
a differential equation in an open set precludes the existence of first integrals
in that set.

13.4 The Newtonian Central Force System 285
13.4 The Newtonian Central Force
System
We now specialize the discussion to the Newtonian central force system. This
system deals with the motion of a single planet orbiting around the sun. We
assume that the sun is fixed at the origin in
R
3
and that the relatively small
planet exerts no force on the sun. The sun exerts a force on a planet given by
Newton’s law of gravitation, which is also called the inverse square law. This
law states that the sun exerts a force on a planet located at X ∈
R
3
whose
magnitude is gm
s
m
p
/r
2
, where m
s
is the mass of the sun, m
p
is the mass of the
planet, and g is the gravitational constant. The direction of the force is toward
the sun. Therefore Newton’s law yields the differential equation
m
p
X
=−gm
s
m
p
X
|X|
3
.
For clarity, we change units so that the constants are normalized to one and
so the equation becomes more simply
X
= F(X) =−
X
|X|
3
.
where F is now the force field. As a system of differential equations, we have
X
= V
V
=−
X
|X|
3
.
This system is called the Newtonian central force system. Our goal in this section
is to describe the geometry of this system; in the next section we derive a
complete analytic solution of this system.
Clearly, this is a central force field. Moreover, it is conservative, since
X
|X|
3
= grad U (X)
where the potential energy U is given by
U (X ) =−
1
|X|
.
Observe that F(X) is not defined at 0; indeed, the force field becomes infinite
as the moving mass approaches collision with the stationary mass at the origin.
As in the previous section we may restrict attention to particles moving in the
plane
R
2
. Thus we look at solutions in the configuration space C = R
2
−{0}.
We denote the phase space by
P = (R
2
−{0}) × R
2
.
