Heitjans P., Karger J. (Eds.). Diffusion in Condensed Matter: Methods, Materials, Models

Подождите немного. Документ загружается.

262 Franz Faupel and Klaus R¨atzke

simulations of the dense random packing of soft spheres of single component

glasses [64] indicate the existence of a high concentration of relatively large

octahedral and a portion of even larger interstices similar in size to a relaxed

vacancy in crystals. In multicomponent glasses the large voids may be ﬁlled by

the smaller atoms. Brandt [65] has demonstrated the stability of vacancies at

0 K in computer simulations of a one-component system. However, this result

does not allow one to draw conclusions on the stability of vacancies at high

temperatures. Bennet et al. [66] have shown vacancies to be unstable in a

Lennard-Jones computer glass at elevated temperatures, whereas a Keating

potential was found to stabilize a point defect. More recent simulations based

in realistic metallic interatomic potentials have shown vacancy-like defects to

be stable on the limited time scale below 1 ns [67].

So far we have focussed on irreversible structural relaxations. These can

be associated with long-range atomic transport. The aforementioned fast

reversible relaxation phenomena are most pronounced in multicomponent

glasses and have been attributed to local rearrangements of atoms within of

the general framework of β relaxation (Sect. 6.4, [68]). Anelastic coopera-

tive changes in the local structure, which may lead to a diﬀerent chemical

short-range order, seem to be involved [69,70].

In addition to fast reversible relaxation, slow reversible changes are ex-

pected to occur for many properties near the glass transition temperature

[62]. As mentioned above the rapid crystallization of almost all conven-

tional glasses near T

, however, made it diﬃcult to study this behavior.

Gerling et al. [71] utilizing an up-quenching technique and neutron irradia-

tion [72] have demonstrated the occurrence of reversible changes in ductility

and density in several amorphous alloys. In the new bulk glasses, of course,

reversibility of properties can always be achieved by annealing above T

and

subsequent cooling [59].

6.5.2 Possible Diﬀusion Mechanisms

We have seen that metallic glasses are prone to structural relaxation, phase

separation, and crystallization. Diﬀusion plays a mayor role in these processes

and is also important for solid-state amorphization alluded to in Sect. 6.5.1.

Nevertheless, despite of a considerable amount of research eﬀort during the

last decades [4, 5, 45, 56, 73–76], the knowledge of diﬀusion in amorphous al-

loys has long been rather limited. Even after the discovery of the new bulk

metallic glasses with their stimulating eﬀect on research several issues re-

main controversial. As for conventional metallic glasses, this is partly due to

experimental diﬃculties, such as the onset of crystallization, which restricts

the maximum annealing temperature and time to values corresponding to a

typical diﬀusion length of some ten nm. In order to circumvent the problems

concomitant with recording diﬀusion proﬁles on this length scale, conclusions

were often drawn from results of indirect methods like measurements of the

crystallization kinetics, viscosity, resistivity, magnetic anisotropy, and from

6 Diﬀusion in Metallic Glasses and Supercooled Melts 263

x-ray and M¨oßbauer techniques. Often, however, an unequivocal relationship

between the measured quantity and the diﬀusion coeﬃcient in the amorphous

phase could not be established. Moreover, despite employing direct techniques

based on high-resolution ion-beam depth proﬁling in combination with a ra-

diotracer method, mass spectrometry, or Auger analysis, many investigators,

particularly in the early measurements, did not properly take into account

structural relaxation. We have seen in Sect. 6.5.1 that the diﬀusivity is very

sensitive to relaxation, and that reproducible results can only be obtained in

well relaxed samples.

While, in view of the disordered nature of amorphous alloys, one would ex-

pect a temperature-dependent eﬀective activation energy (Sect. 6.4), it is now

well established that Arrhenius plots for diﬀusion in the relaxed metastable

amorphous state are linear, i.e. they exhibit a constant activation energy H.

This has often been interpreted as being indicative of a diﬀusion mechanism

similar to that in crystals, where vacancy-like defects in thermal equilibrium

are the carriers of diﬀusion, even though resulting D

values were sometimes

many orders of magnitude diﬀerent from those typical of a vacancy mech-

anism. Moreover, numerous observations, e.g., the occurrence of anomalous

hydrogen diﬀusion [29] (cf. Sect. 6.4) and the diﬀerence in the activation en-

ergies for self-diﬀusion and magnetic relaxation, Kronm¨uller and Frank [27]

point to a broad spectrum of activation energies. Apparently, only a narrow

range of this spectrum is probed during long-range diﬀusion experiments in

the small accessible temperature interval. Furthermore, it has been shown

that compensation of side and saddle point disorder (see Fig. 6.2) may also

lead to a almost linear Arrhenius plot [26, 77]. An additional explanation of

the linear Arrhenius plots can be given in terms of a highly cooperative dif-

fusion mechanism, discussed below, which averages over local diﬀerences in

the barrier heights.

There are several experimental results that lend support to the idea of

defect-mediated diﬀusion in metallic glasses. For example, diﬀusion can be

enhanced by irradiation, which in crystalline materials produces additional

point defects. The annealing behavior of the radiation-induced excess volume

also resembles that of crystals [78,79]. Moreover, the crystallization kinetics

of amorphous (FeNi)

(PB)

were studied under hydrostatic pressure [76]. The

resulting activation volume of the order of one atomic volume was taken as

evidence of diﬀusion via point-defectlike entities in thermal equilibrium. It

should be noted, however, that the evaluation of diﬀusion coeﬃcients from

measurements of the crystallization kinetics is a rather indirect approach

which has often been criticized [73,80]. Direct measurements of the pressure

dependence of diﬀusion will be discussed in detail in Sect. 6.5.4.

Tu and Chou [81] studied interdiﬀusion in electron-beam evaporated

amorphous NiZr trilayer ﬁlms. Void formation was observed when both Ni

and Zr diﬀused. Since the voids formed on the side with the higher con-

centration of the slower species this was termed ‘opposite Kirkendall eﬀect’.

264 Franz Faupel and Klaus R¨atzke

No voids were seen when Ni was the dominant diﬀusing species. The au-

thors concluded that self diﬀusion in the glass can be mediated by localized

vacancy-like defects, which may agglomerate like vacancies in crystals if their

concentration is out of equilibrium, as well as by non-localized ‘free-volume

defects’. A free-volume model of diﬀusion in metallic glasses has been pro-

posed by Spaepen [55]. The original theory for liquids (Sect. 6.3) was mod-

iﬁed by introduction of an activation barrier for a diﬀusive jump into an

evolving hole. The free volume concept was corroborated by Chason and Mi-

zoguchi [82] who evaluated diﬀusion coeﬃcients from the shift in position of

the modulated diﬀraction peak in compositionally modulated amorphous Fe-

Ti ﬁlms as function of densiﬁcation. The expected exponential dependence

of D on the free volume was indeed observed. Hahn and coworkers [83] mea-

sured the dependence of tracer diﬀusion on atomic size and alloy composition

in amorphous Ni-Zr. From similarities to α-zirconium they drew the conclu-

sion that small atoms, including Ni, diﬀuse by an interstitial-like mechanism,

using the interstices of the amorphous structure as jump positions. Such an

interstitial model of diﬀusion has been proposed by Ahmadzadeh and Can-

tor [84]. For larger atoms like Zr diﬀusion was explained in terms of the

coordinated motion of many atoms creating a localized free volume defect.

Cooperative diﬀusion mechanisms in metallic glasses have also been pro-

posed by others, [55, 73, 85–87], mostly in view of the extreme variety of

values alluded to above, which reﬂects a corresponding variability of the

entropy of diﬀusion (Chap. 1). Furthermore, the ln D

-vs-H relationship de-

viates strongly from that for single-jump diﬀusion in crystals. The envisioned

atomistic picture is often reminiscent of or directly related to the free-volume

approach. Highly collective thermally activated processes, such as chain-like

displacements of many atoms have been observed in computer simulations,

which will be discussed in more detail below [41,88, 89].

The new bulk metallic glasses have oﬀered the opportunity to measure

diﬀusion in the supercooled liquid state and to investigate changes in the

transport mechanism concomitant with the glass transition and even in the

equilibrium melt. Several groups have carried out diﬀusion measurements

in bulk glasses during the last years by means of the radiotracer technique

[90,91] or secondary ion mass spectrometry [92] (see Chap. 1 for experimental

techniques). One generally observes a kink in the Arrhenius plot near the

caloric glass transition temperature as measured, e.g., by diﬀerential scanning

calorimetry (DSC). In accord with the expected time scale dependence of the

caloric glass transition temperature the kink is shifted to lower temperatures

for slower diﬀusing elements, and for Al no kink is observed in the investigated

temperature range, for instance. The kink in the Arrhenius plot has often been

interpreted as being due to a change in the diﬀusion mechanism [92, 93]. In

particular, a transition from single-atom hopping to liquid-like viscous ﬂow

has been proposed at the caloric glass transition temperature T

[94,95].

6 Diﬀusion in Metallic Glasses and Supercooled Melts 265

The notion of a change in the mechanism of atomic transport at T

is in

conﬂict with predictions of the mode coupling theory (MCT) [96] discussed

in Sect. 6.4. According to MCT, a gradual transition in the atomic transport

mechanism from solid-like thermally activated local hopping – envisioned as

a highly collective process involving many atoms [25] – to liquid-like motion

occurs well above T

at a microscopic glass transition temperature T

. T

does not depend on the time scale of the experiment and is the temperature

where, upon cooling, due to the concomitant increase of density, the cage

formed by the neighboring atoms of a given atom is frozen in and can only

be overcome by hopping processes.

In the present light we are left with the following questions:

1. Is diﬀusion in fully relaxed metallic glasses generally mediated by thermal

equilibrium defects similar to vacancies in crystals as often suggested (see

review articles [18, 97])?

2. If thermal defects do not mediate diﬀusion, is diﬀusion in relaxed amor-

phous alloys a highly cooperative process as one would infer from com-

puter simulations and the concept of ‘medium assisted hopping’ discussed

in Sect. 6.4?

3. Which role do non-equilibrium defects play?

4. Do we have to consider diﬀerent mechanisms for diﬀusion in relaxed and

as-quenched samples, in other words: how does quenched-in excess volume

aﬀect diﬀusion?

5. Is there a change in the diﬀusion mechanism at the caloric glass transition

temperature or does the change occur above the critical temperature Tc

where, according to the mode coupling theory liquid-like atomic motion

sets in?

In the next sections we shall see that these questions can be answered

satisfactorily by critical experiments. Investigations of the isotope-mass de-

pendence of diﬀusion in relaxed samples (Sect. 6.5.3) are pertinent to the

second question. In Sect. 6.5.4 measurements of the pressure dependence of

diﬀusion will be discussed, which are related to the role of equilibrium defects.

The role of excess volume will be addressed in Sect. 6.5.5 mainly based on

measurements of the isotope eﬀect during structural relaxation. Section 6.6

will be devoted to the 5th question. Here we will also discuss very recent

diﬀusion and isotope measurements in the equilibrium liquid.

6.5.3 Isotope Eﬀect

A salient feature of diﬀusion in metallic glasses is the extremely small isotope

eﬀect. The isotope eﬀect E for diﬀusion of two isotopes with diﬀusivities D

and masses m

is deﬁned as ( [98], see also Chap. 1, Sect. 1.7.2)

E =(D

− 1)

)



− 1



. (6.9)

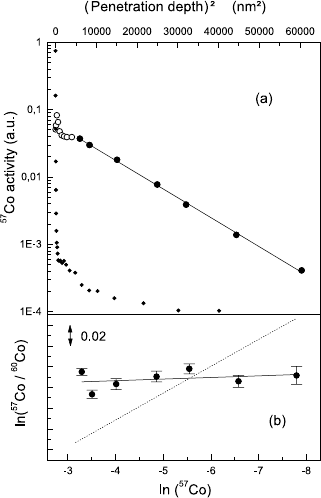
266 Franz Faupel and Klaus R¨atzke

Fig. 6.3. (a) Typical penetra-

tion proﬁle of

Co diﬀusion in

at 603 K and 1 h

annealing time. The activity is

plotted vs. the square of penetra-

tion depth. (The very ﬁrst data

points (open symbols)areaﬀected

by surface eﬀects and were not

taken in to account.) The resolu-

tion function of the depth proﬁl-

ing technique, obtained from a thin

tracer layer without annealing, is

shown in black. (b) Corresponding

isotope eﬀect proﬁle. The activity

ratio of

Co and

Co is plotted

vs. the

Co activity on a logarith-

mic scale. The dotted line indicates

an isotope eﬀect of E =1andis

shown for comparison (from [60]).

For single jump diﬀusion in densely packed lattices E is generally of the order

of unity because of the m

−1/2

dependence of the attempt frequency [98],

although correlation eﬀects may give rise to a signiﬁcant reduction of E,

particularly in case of diﬀusion of diluted impurities due to impurity-vacancy

binding (Chap. 1) [99]. E can also be strongly reduced by relaxation of the

surrounding [100] and a broad distribution of activation energies [101].

Almost vanishing isotope eﬀects have been observed for Co diﬀusion in

various metallic glasses [102–106]. E was determined by measuring the si-

multaneous diﬀusion of the radiotracers

Co and

Co employing the serial

sectioning technique in conjunction with ion-beam sputtering. For illustra-

tion of the technique, which is described in Chap. 1, Fig. 6.3 depicts a typical

radiotracer proﬁle on a semilogarithmic scale. According to the thin ﬁlm so-

lution of Fick’s 2nd law (Chap. 1, (1.9)) a straight line ﬁtted to the proﬁle

has the slope 1/(4Dt) and thus yields the tracer diﬀusivity D if the annealing

time t is known. In order to obtain the isotope eﬀect one can easily derive

the following equation based on the thin ﬁlm solution

ln[c

(x, t)/c

(x, t)] = const. − (D

− 1) · ln[c

(x, t)] . (6.10)

Equation (6.10) shows that the slope of a straight line ﬁtted to the data in

Fig. 6.3b is given by D

−1. The isotope eﬀect E then follows from (6.9)

and the isotope masses. Representative isotope eﬀects for Co-Zr glasses are

shown in Fig. 6.4.

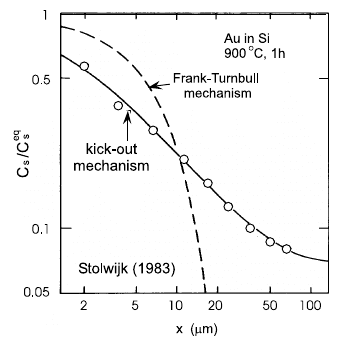
6 Diﬀusion in Metallic Glasses and Supercooled Melts 267

Fig. 6.4. Isotope eﬀect in Co

1−x

glasses for 0.31 <x<0.86. One

notes that the isotope eﬀect is very

close to zero in the whole concentra-

tion range [105].

The essentially vanishing isotope eﬀects measured in metallic glasses were

taken as strong evidence of a highly collective hopping mechanism, as sug-

gested by the notion of highly cooperative medium assisted hopping (see

Sect. 6.4) and molecular dynamics (MD) simulations [107–109]. In these terms

the extremely small isotope eﬀects are attributed to a strong dilution of the

mass dependence of diﬀusion due to the participation of a large number of

atoms in collective hopping processes.

In MD simulations mainly chain-like displacements have been observed

[88]. Both the computer simulations as well as the isotope eﬀect measurements

suggest the number of atoms participating in a cooperative hopping process

to be very high, typically well above ten [109, 110].

Computer simulations also indicate a connection between the low-fre-

quency excitations in glasses and long-range diﬀusion [88]. These low-fre-

quency excitations appear to be characteristic of topologically disordered

solids [111–114]. They give rise to extra speciﬁc heat at low temperatures and

to additional contributions to the low-frequency part of the vibrational den-

sity of states as obtained by Raman and inelastic scattering. Close to absolute

zero temperature the excitations can be attributed to tunneling (two-level)

systems and at somewhat higher temperatures to quasi-localized vibrational

states [113]. In addition to the periodic low frequency excitations aperiodic

thermally activated relaxations have been observed [115]. Fits of an extended

soft-potential model to experimental data resulted in eﬀective masses of 20 to

100 atomic masses for the entities moving in the eﬀective soft potentials. In a

soft-sphere glass, which is a ﬁrst approximation of a metallic glass, the local

relaxations turned out to be collective jumps of groups of atoms, predom-

inantly chains (sometimes with side-branches) along dense directions [115].

The eﬀective mass and the total jump length of the atoms were found to

increase with increasing temperature, and the possibility of fusion of isolated

268 Franz Faupel and Klaus R¨atzke

relaxations at high temperatures was demonstrated. It is not unlikely that

such processes lead to long-range diﬀusion. We emphasize, however, that our

isotope eﬀect measurements do not rule out other cooperative mechanisms,

e.g., those involving smeared out defects.

6.5.4 Pressure Dependence

Information on the role of thermal defects have been obtained from measure-

ments of the pressure dependence of diﬀusion. For a single-jump-type vacancy

mechanism in crystalline close packed metals the activation volume, given by

act

≈−k



∂ ln D

∂p



, (6.11)

is of the order of the size of the relaxed vacancy, i.e., somewhat smaller than

one atomic volume [98]. In (6.11) D, p, T ,andk

are diﬀusivity, pressure,

absolute temperature, and Boltzmann constant, respectively. For interstitial

diﬀusion, i.e. a direct mechanism without thermally generated defects, the

activation volume proved to be nearly vanishing [98]. Both observations sug-

gest the activation volume to be essentially given by the formation volume of

the defect and the migration volume for a single-jump mechanism to be very

small.

Several measurements, carried out by our group [116–118] and the M¨unster

group [119], have revealed an almost vanishing pressure dependence for

Co diﬀusion in the metallic glasses Co

76.7

14,3

,Fe

,and

. A recent example is shown in Fig. 6.5 [118]. This in conjunction

with the very small isotope eﬀects, measured in these systems, led us to the

conclusion that the migration volume can be close to zero even for a coop-

erative hopping process involving many atoms. Consequently, diﬀusion was

interpreted in terms of direct cooperative hopping process as observed in the

above mentioned MD simulations.

On the other hand, a pronounced pressure dependence, corresponding

to activation volumes between one half and two atomic volumes, expressed

in terms of the average atomic volume of the alloy, was reported for Au

diﬀusion in amorphous Pd

[120], Ni diﬀusion in Ni

100−x

(42 <

x<62) [121] and Co

glasses [122], Hf diﬀusion in Ni

[123], and

Zr diﬀusion in Co

[124]. Based on our aforementioned conclusion on

the possibility of very small migration volumes even for cooperative hopping

processes we tend to attribute the observed signiﬁcant activation volumes to

formation of thermal defects, which, however, may not necessarily be localized

like a vacancy in a crystal but are expected to be spread out. On the other

hand, recent results from MD simulations indicate that collective hopping

processes may have a signiﬁcant migration volume [109].

Of particular interest is the case of Co-rich Co-Zr glasses. Here Co seems

to diﬀuse by a cooperative hopping process without assistance of thermal

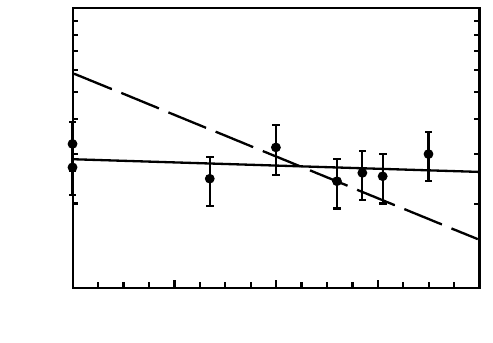
6 Diﬀusion in Metallic Glasses and Supercooled Melts 269

p (MPa)

0 200 400 600 800

D (m

-1

)

-21

-20

Fig. 6.5. Isothermal pressure dependence of

Co diﬀusion in structurally relaxed

amorphous Co

at 563 K [118]. The dashed line corresponds to an activation

volume of one atomic volume.

defects, judging from a vanishing isotope eﬀect [104] and an almost vanishing

pressure dependence [118]. Diﬀusion of Zr, in contrast, is characterize by a

large activation volume of the order of an atomic volume, which we attributed

to diﬀusion via thermal defects [124]. This points to the existence of an op-

posite Kirkendall eﬀect in interdiﬀusion in amorphous Co-Zr or Ni-Zr alloys

which should behave similarly. An opposite Kirkendall eﬀect, evidenced by

void formation on the side with a higher concentration of the slower com-

ponent, was indeed reported under conditions where both Ni and Zr were

mobile in interdiﬀusion experiments involving amorphous Ni-Zr couples of

diﬀerent composition. As expected no such eﬀect was seen when only Ni was

mobile (see Sect. 6.5.1) [81]. These experiments lend support to the existence

of thermal defects that mediate diﬀusion.

Mechanisms based on delocalized thermal defect have been proposed be-

fore [55, 97, 125, 126]. A well known example is the spread out free vol-

ume within the free-volume approach modiﬁed for glasses (see Sect. 6.5.2)

[55,94, 127].

6.5.5 Eﬀect of Excess Volume on Diﬀusion

So far, we have discussed diﬀusion in structurally relaxed metallic glasses.

Only in these systems the structure is stable during a diﬀusion experiment,

and reproducible diﬀusion measurements can be made, if the diﬀusion tem-

perature does not signiﬁcantly exceed the relaxation temperature and the

diﬀusion time in not too long. On the other hand, conventional metallic

glasses, and to some extend also bulk glasses [128, 129], contain excess vol-

270 Franz Faupel and Klaus R¨atzke

ume quenched in from the liquid state. This excess volume aﬀects nearly all

properties [130] and enhances diﬀusion [131,132]. Therefore, we have investi-

gated the inﬂuence of excess volume on the diﬀusion mechanism by measuring

both the diﬀusivity and the isotope eﬀect during structural relaxation of as

quenched glasses. First measurements were carried out for Co diﬀusion in

a Co-rich metal-metalloid glass [133]. The isotope eﬀect was found to be as

high as 0.5 in the as-quenched state and to drop to a very low value of 0.1 in

the relaxed state evidenced by a plateau region of the diﬀusivity. The high

isotope eﬀects in an as quenched state corroborates the notion of quenched-in

quasi-vacancies, which migrate to the outer sample surface during structural

relaxation [56].

On the other hand, our recent isotope eﬀect measurements in thin amor-

phous Co-Zr ﬁlms do not show any change in the diﬀusion mechanism during

structural relaxation and point to a collective hopping mechanism in the

relaxed and in the as-quenched state [132,134]. As shown in Fig. 6.6a the dif-

fusivity drops substantially during relaxation and ﬁnally reaches the expected

plateau value. The course of D(t) is well described by the Kohlrausch law

given in (6.7). The isotope eﬀect in very low in the whole range (Fig. 6.6b)

reﬂecting highly collective diﬀusion. Similar behavior was observed in thin

ﬁlms. Apparently, in the thin Co-Zr ﬁlms excess volume appears to

annihilate intrinsically, e.g., by recombination of regions of higher and lower

density on the nanoscopic scale as ﬁrst suggested by Egami et al. [130].

6.6 Diﬀusion in Supercooled and Equilibrium Melts

Most diﬀusion studies in the new bulk-glass formers where performed in the

amorphous ‘Johnson alloy’ Zr

7.5

27.5

also termed Vitrelloy 4.

An Arrhenius plot summarizing the results for the is shown in Fig. 6.7, for

example. One notes the aforementioned kinks shifting to lower temperatures

for slower diﬀusing species, which have often been interpreted as a change

in the diﬀusion mechanism at the caloric glass transition temperature. The

diﬀusivities follow the expected size dependence (for a detailed discussion

see, e. g., Chap. 5 in [5]) in both the glassy and the supercooled liquid state

except for the Be data reported by Geyer et al. (dashed line in Fig. 6.7). The

intersection of the Be diﬀusivity with those of substantially larger elements

is quite unusual and contradicts expectations on the size dependence of dif-

fusion as well as more general concepts of the convergence of the diﬀusivities

at higher temperatures (above T

) as predicted by the Stokes-Einstein equa-

tion. While Geyer at al. performed chemical diﬀusion experiments, which are

inﬂuenced by the thermodynamic factor [99] recent radiotracer experiments

of our group in cooperation with the group of Geyer demonstrated that the

behavior of Be is by no means exceptional. As shown in Fig. 6.7, the radio-

tracer data of Rehmet et al. [135] (solid line) nicely ﬁt in with the overall

picture. The diﬀerences between the tracer and the chemical diﬀusion data

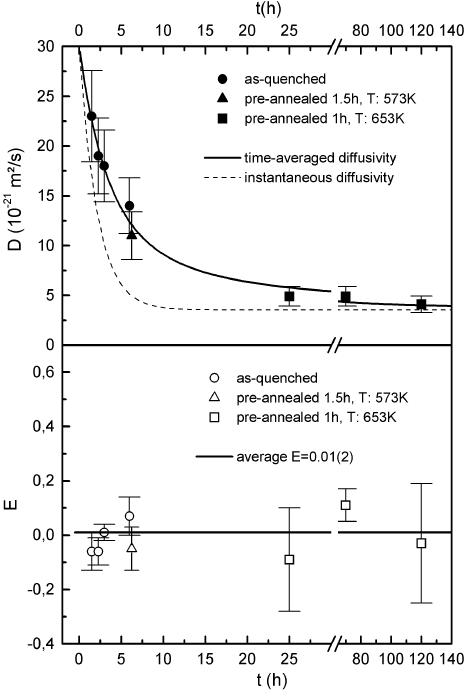
6 Diﬀusion in Metallic Glasses and Supercooled Melts 271

Fig. 6.6. (top) Time dependence of

Co diﬀusion in Co

during structural

relaxation at T = 300

◦

C. The squares indicate samples that have been preannealed

at T = 380

◦

C for 1 h. Circles indicate specimens that have not been preannealed

before diﬀusion treatment while the specimen described by a triangle was annealed

for 1.5 h and again for 4.75 h. The straight line represents the time-averaged dif-

fusivity, the dashed line shows the instantaneous diﬀusivity (for details see 132).

(bottom) Isotope eﬀect E in Co

during structural relaxation. The squares

indicate the preannealed samples while circles display the specimens which have

not been preannealed before diﬀusion annealing. The specimen represented by a

triangle was annealed for 1.5 h and again for 4.75 h.

are not yet understood. They cannot be attributed to the thermodynamic

factor, which goes to unity at high temperatures.

Recently, we have also carried out isotope eﬀect measurements involving

the isotopes

Co and

Co by means of the radiotracer technique in the