Hearn E.J. Mechanics of Materials. Volume 1
Подождите немного. Документ загружается.


59.4
Thin Cylinders
and
Shells
203
..
or
Fig.
9.4.
Half of
a
thin sphere subjected
to
internal pressure showing uniform hoop stresses
acting
on
a surface element.
nd
4
p
x
-
=
CTH
x
ndt
Pd
bH=-
4t
Pd
circumferential
or
hoop
stress
=
-
4t
i.e.
9.3.1.
Change in internal volume
As
for the cylinder,
change in volume
=
original volume
x
volumetric strain
but
volumetric strain
=
sum
of
three mutually perpendicular strains (in this case all equal)
=
3ED
=
3EH
..
3Pd
change
in
internal volume
=
-
[
1
-
v]
Y
4tE
(9.9)
9.4.
Vessels subjected to fluid pressure
If a fluid is used as the pressurisation medium the fluid itself will change in volume as
pressure is increased and this must
be
taken into account when calculating the amount
of
fluid which must be pumped into the cylinder in order to raise the pressure
by
a specified
amount, the cylinder being initially full
of
fluid
at atmospheric pressure.
Now the
bulk modulus
of
a fluid is defined as follows:
volumetric stress
volumetric strain
bulk modulus
K
=

204
Mechanics
of
Materials
59.5
where, in this case,volumetric stress
=
pressure
p
and volumetric strain
=
-
change in volume
6V
original volume
Y
-_
(9.10)
PV
i.e.
change in volume
of
fluid
under pressure
=
-
K
The extra fluid required to raise the pressure must, therefore, take up this volume together
with the increase in internal volume of the cylinder itself.
..
extra fluid required to raise
cylinder
pressure by
p
=
-[5-4v]
Pd
v+-
PV
4tE
K
Similarly, for
spheres,
the extra fluid required is
=
~
3Pd
[l
-
v]
v+
PV
-
4tE
K
(9.1 1)
(9.12)
9.5.
Cylindrical vessel with hemispherical ends
Consider now the vessel shown in Fig.
9.5
in which the wall thickness of the cylindrical and
hemispherical portions may
be
different (this is sometimes necessary since the hoop stress in
the cylinder is twice that in
a
sphere of the same radius and wall thickness). For the purpose of
the calculation the internal diameter of both portions is assumed equal.
From the preceding sections the following formulae are known
to
apply:
I
c
1
I
t
t
t
I
I
I
I
1
I
Fig.
9.5.
Cross-section
of
a
thin cylinder
with
hemispherical
ends.
(a)
For
the cylindrical portion
Pd
2tc
hoop or circumferential stress
=
bHc
=
-

$9.6
Thin Cylinders
and
Shells
205
..
Pd
longitudinal stress
=
aLc
=
-
44
1
E
hoop or circumferential strain
=
-
[gHc
-
vak]
Pd
=
-[2-v]
44
E
(b)
For
the hemispherical ends
Pd
4ts
hoop stress
=
oHs
=
-
1
E
hoop strain
=
-
[aHs
-
voHs]
Pd
=
-[1
-v]
4t,E
Thus equating the two strains in order that there shall
be
no distortion of the junction,
-[2-v]
Pd
=
-[1
Pd
-v]
4t,
E
4t,E
i.e.
(9.13)
With the normally accepted value of Poisson’s ratio for general steel work of
0.3,
the
t,
0.7
t,
1.7
thickness ratio becomes
-
=-
i.e. the thickness
of
the cylinder walls must
be
approximately
2.4
times that of the
hemispherical ends for no distortion of the junction to occur. In these circumstances, because
of the reduced wall thickness of the ends, the maximum stress will occur in the ends. For
equal
maximum stresses
in the two portions the thickness of the cylinder walls must
be
twice that in
the ends but some distortion at the junction will then occur.
9.6.
Effects
of
end plates and joints
The preceding sections have all assumed uniform material properties throughout the
components and have neglected the effects of endplates and joints which are necessary
requirements for their production. In general, the strength of the components will
be
reduced
by the presence
of,
for example, riveted joints, and this should
be
taken into account by the
introduction
of
a
joint eficiency factor
tf
into the equations previously derived.

206
Mechanics
of
Materials
59.7
Thus, for
thin cylinders:
Pd
2tq
L
hoop stress
=
~
where
q
is the efficiency of the longitudinal joints,
Pd
longitudinal stress
=
-
4tqc
where
qc
is the efficiency of the circumferential joints.
For
thin spheres:
Pd
hoop stress
=
-
4tV
Normally the joint efficiency is stated in percentage form and this must be converted into
equivalent decimal form before substitution into the above equations.
9.7.
Wire-wound thin cylinders
In order to increase the ability of thin cylinders to withstand high internal pressures
without excessive increases in
wall
thickness, and hence weight and associated material cost,
they are sometimes wound with high tensile steel tape or wire under tension.
This
subjects the
cylinder to an initial hoop, compressive, stress which must be overcome by the stresses owing
to internal pressure before the material is subjected to tension. There then remains at this
stage the normal pressure capacity of the cylinder before the maximum allowable stress in the
cylinder is exceeded.
It is normally required to determine the tension necessary in the tape during winding in
order to ensure that the maximum hoop stress in the cylinder will not exceed a certain value
when the internal pressure is applied.
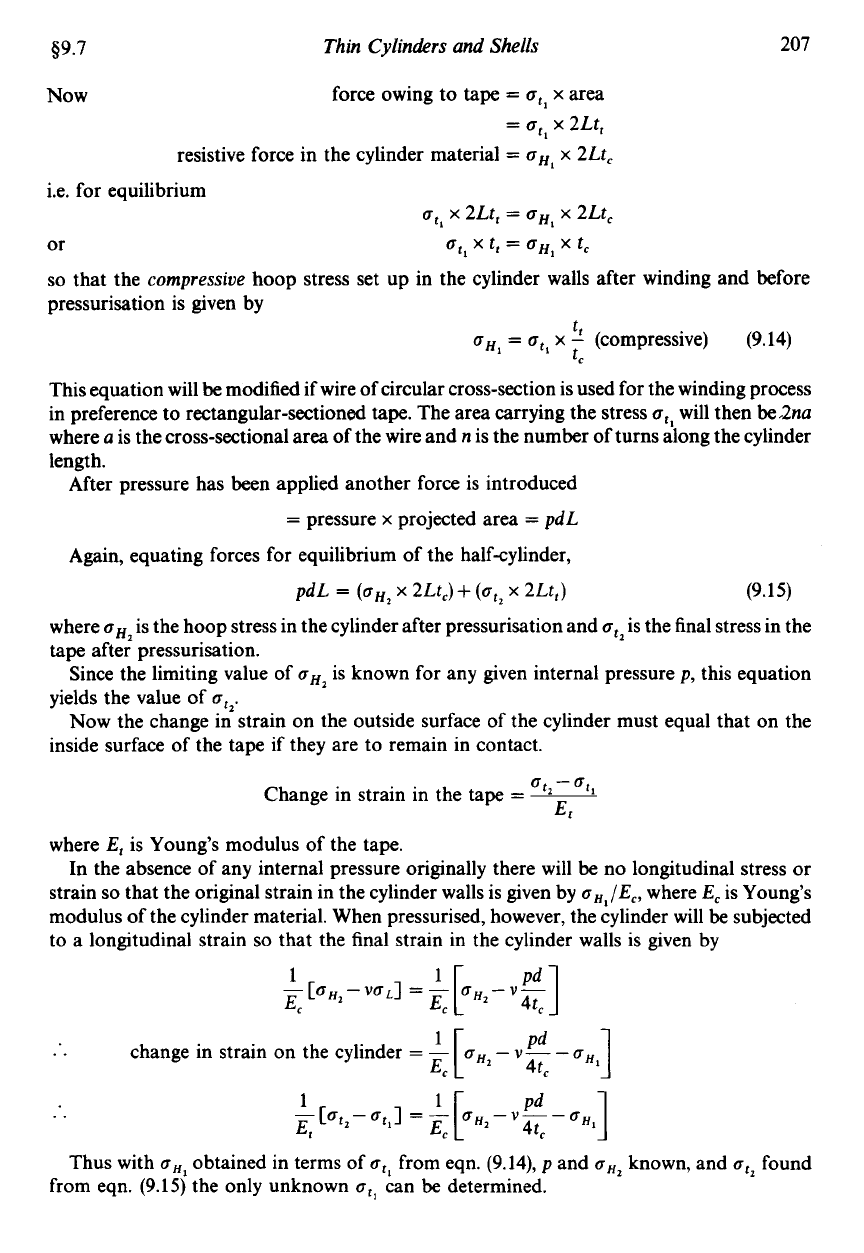
Consider, therefore, the half-cylinder
of
Fig.
9.6,
where
oH
denotes the hoop stress in the
cylinder walls and
o,
the stress in the rectangular-sectioned tape. Let conditions before
pressure is applied be denoted by suffix
1
and after pressure is applied by suffix
2.
Fig.
9.6.
Section ofa
Tope
thin cylinder with an external layer
of
tape
wound on with a tension.
$9.7
Thin Cylinders and Shells
207
Now
force owing to
tape
=
or]
x
area
=
ut,
x
2Lt,
ut,
x
2Lt,
=
OH,
x
2Ltc
bt
x
t,
=
OH]
x
t,
resistive force in the cylinder material
=
oH,
x
2Lt,
i.e. for equilibrium
or
so
that the
compressive
hoop stress set up in the cylinder walls after winding and before
pressurisation is given by
t
o,,,
=
crl
x
2
(compressive)
(9.14)
tc
This equation will
be
modified if wire of circular cross-section
is
used for the winding process
in preference to rectangular-sectioned
tape.
The area carrying the stress
ctl
will then
beans
where
a
is the cross-sectional area of the wire and
n
is the number of turns along the cylinder
length.
After pressure has been applied another force is introduced
=
pressure
x
projected area
=
pdL
Again, equating forces for equilibrium of the halfcylinder,
pdL
=
(oH,
x
2Ltc)
+
(or,
x
2Lt,)
where
o,,,
is the hoop stress in the cylinder after pressurisation and
otl
is the final stress in the
tape
after pressurisation.
Since the limiting value of
(iH,
is known for any given internal pressure
p,
this equation
yields the value of
or,.
Now the change in strain on the outside surface of the cylinder must equal that on the
inside surface of the tape if they are to remain in contact.
(9.15)
or,
-
or1
Change in strain in the tape
=
~
Et
where
E,
is Young’s modulus of the tape.
In the absence of any internal pressure originally there will
be
no longitudinal stress or
strain
so
that the original strain in the cylinder walls is given
by
oHl/Ec,
where
E,
is Young’s
modulus of the cylinder material. When pressurised, however, the cylinder will
be
subjected
to a longitudinal strain
so
that the final strain in the cylinder walls is given by
..
change in strain on the cylinder
=
Thus with
bH,
obtained in terms of
err,
from eqn.
(9.14),
p
and
bH,
known, and
or,
found
from eqn.
(9.15)
the only unknown
or,
can be determined.

208
Mechanics
of
Materials
Examples
Example
9.1
A
thin cylinder
75
mm internal diameter,
250
mm long with walls
2.5
mm thick is subjected
to an internal pressure
of
7
MN/mZ. Determine the change in internal diameter and the
change in length.
If,
in addition to the internal pressure, the cylinder is subjected to a torque
of
200
N m, find
the magnitude and nature
of
the principal stresses set up in the cylinder.
E
=
200
GN/m2.
v
=
0.3.
Solution
Pd2
(a) From eqn.
(93,
change in diameter
=
-
(2
-
v)
4tE
7
x
106
x
752
x
10-6
(2
-
0.3)
- -
4
x
2.5
x
10-3
x
200
109
=
33.4
x
m
=
33.4
pm
PdL
(b)
From eqn.
(9.3),
change in length
=
-
(1
-
2v)
4tE
7
io6
75
10-3
250
x
10-3
(1
-
0.6)
- -
4
2.5
x
10-3
x
200
x
109
=
26.2pm
pd
7
x
lo6
x
75
x
Hoop
stress
oH
=
-
=
2t
2
x
2.5
x
10-3
=
105MN/m2
pd
7
x
lo6
x
75
x
Longitudinal stress
oL
=
4t
=
2
x
2.5
10-3
=
52.5MN/m2
In addition to these stresses
a
shear stress
5
is set up.
From the torsion theory,
TT.
TR
JR
J
-_-
-
..
T=-
Now
Then
x
(804-754)
x
(41 -31.6)
J=-
=-
=
0.92
x
m4
32
1OI2
32
lo6
200
x
20
x
10-3
shear stress
T
=
-6
=
4.34
MN/m2
0.92
x
10-

Thin
Cylidrs
and
Shells
209
t
Or*
On
@
T
0,
=
crL
Enlorgod
vmw
oi
dement
an
wrfoce
of
cylinder subjected
to
torque ond internol
pressure
Fig.
9.7.
Enlarged view
of
the
stresses
acting
on
an
element
in
the surface
of
a
thin
cylinder
subjected
to
torque
and
internal pressure.
The stress system then acting on any element of the cylinder surface is as shown in Fig. 9.7.
The principal stresses are then given by eqn. (1 3.1
l),
.
u1
and
a’
=
*(a,
+
o,,)
i-
*J[
(a,
-
a,)’
+
42,,’]
=
$(lo5
+
52.5)ffJ[(lO5
-
52.5)’
+4(4.34)’]
=
3;
x
157.5 fiJ(2760
+
75.3)
=
78.75
f
26.6
Then
o1
=
105.35MN/m2 and
a2
=
52.15MN/m2
The principal stresses are
105.4
MN/mZ
and
52.2
MN/m2
both tensile.
Example
9.2
A
cylinder has an internal diameter of 230
mm,
has
walls
5
mm
thick and
is
1
m
long.
It is
m3 when filled with a
liquid
at a pressure
p.
found to change in internal volume by 12.0
x
If
E
=
200GN/m2 and
v
=
0.25, and assuming rigid end plates, determine:
(a) the values of hoop and longitudinal stresses;
(b) the modifications to these values
if
joint efficiencies of
45%
(hoop)
and
85%
(c)
the necessary change in pressure
p
to produce a further increase in internal volume
of
(longitudinal) are assumed;
15
%.
The liquid may
be
assumed incompressible.
Solution
(a) From eqn..
(9.6)
change in internal volume
=
-
pd
(5-4v)V
4tE

210
Mechanics
of
Materials
original volume
V
=
f
x
2302
x
x
1
=
41.6
x
m3
p
x
230
x x
41.6
x
(5
-
1)
4
x
5
x
10-3
x
200
x
109
Then change in volume
=
12
x
=
Thus
Hence,
12
10-6
x
4
x
5
x
10-3
x
200
x
109
=
230
x
10-3 41.6
x
10-3
x
4
=
1.25 MN/m2
pd
1.25
x
lo6
x
230
x
hoop stress
=
-
=
2t
Pd
2
x
5
x
10-3
=
28.8MN/m2
longitudinal stress
=
-
=
14.4 MN/m2
4t
(b) Hoop stress, acting on the longitudinal joints
($9.6)
pd
1.25
x
lo6
x
230
x
=--
-
2tVL
2
5
x
10-3
x
0.85
=
33.9 MN/mZ
Longitudinal stress (acting on the circumferential joints)
pd
1.25
x
lo6
x
230
x
1O-j
-
-
4tv,
4
x
5
x
10-3
x
0.45
=
32MN/m2
(c) Since the change in volume is directly proportional to the pressure, the necessary 15
%
increase in volume
is
achieved by increasing the pressure also by 15
%.
Necessary increase in
p
=
0.15
x
1.25
x
lo6
=
1.86 MN/mZ
Example
9.3
(a)
A
sphere, 1 m internal diameter and 6mm wall thickness, is to be pressure-tested for
safety purposes with water as the pressure medium. Assuming that the sphere is initially filled
with water at atmospheric pressure, what extra volume of water is required to
be
pumped in
to produce a pressure of 3 MN/m2 gauge? For water,
K
=
2.1 GN/m2.
(6)
The sphere is now placed in service and filled with gas until there is a volume change of
72
x
(c) To what value can the gas pressure be increased before failure occurs according to the
maximum principal stress theory
of
elastic failure?
For the material
of
the sphere
E
=
200 GN/mZ,
v
=
0.3 and the yield stress
0,
in simple
tension
=
280
MN/m2.
m3. Determine the pressure exerted by the gas on the walls of the sphere.

Thin Cylinders and Shells
Solution
21
1
volumetric stress
volumetric strain
(a) Bulk modulus
K
=
Now
and
volumetric stress
=
pressure
p
=
3
MN/mZ
volumetric strain
=
change in volume
+-
original volume
i.e.
P
K=-
6V/V
pv
3X1O6
48
K
2.1 x 109
3
change in volume
of
water
=
-
=
x
-
..
=
0.748
x
m3
(b)
From eqn.
(9.9)
the change in volume is given by
3Pd
6
v
=
-
(1
-
v)
v
4t
E
..
..
3p
x
1
x
$~(0.5)~(1
-0.3)
72
x
=
4
x
6
x
72 x
x
200
x
lo9
x
4
x
6 x 200
x
lo6
x
3
3
x 4n(0.5)3
x
0.7
P=
=
314 x lo3
N/m2
=
314
kN/mZ
(c) The maximum stress set up in the sphere will
be
the hoop stress,
i.e.
Pd
aI
=
aH
=
-
4t
Now, according to the maximum principal stress theory (see
915.2)
failure will occur when
the maximum principal stress equals the value of the yield stress of a specimen subjected to
simple tension,
i.e. when
o1
=
ay
=
280MN/m2
Thus
d
4t
280 x lo6
=
280
x
lo6 x 4
x
6
x
1
P'
=
6.72
x
lo6
N/m2
=
6.7
MN/mZ
The sphere would therefore yield at a pressure
of
6.7
MN/mZ.
Example
9.4
A
closed thin copper cylinder of
150
mm internal diameter having a wall thickness of
4
mm
is closely wound with a single layer
of
steel tape having a thickness of
1.5
mm, the tape being

212
Mechanics
of
Materials
wound on when the cylinder has no internal pressure. Estimate the tensile stress in the steel
tape
when it
is
being wound to ensure that when the cylinder is subjected to
an
internal
pressure of
3.5
MN/m2 the tensile hoop stress
in
the cylinder will not exceed
35
MN/m2. For
copper, Poisson’s ratio
v
=
0.3
and
E
=
100 GN/m2; for steel,
E
=
200 GN/m2.
Solution
Let
6,
be
the
stress
in
the
tape
and
let conditions before pressure is applied
be
denoted by
Consider the halfglider shown (before pressure is applied) in Fig.
9.6
(see
page 206):
sufb
1
and after pressure
is
applied
by
s&
2.
force owing to tension in tape
=
ut1
x
area
=
x
1.5
x
10-3
x
L
2
resistive force in the material of cylinder wall
=
on,
x
4
x
lo-’
x
L
x
2
..
2oH,
x
4
x
10-3
x
L
=
2ot1
x
1.5
x
10-3
x
L
1.5
4
..
oH,
=
-
or,
=
0.375
otI
(compressive)
After pressure is applied another force is introduced
=
pressure
x
projected area
=
PW)
Equating forces now acting on the half-cylinder,
pdL
=
(aH2
x
2
x
4
x
10-
but
p
=
3.5
x
lo6
N/mZ and
oH,
=
35
x
lo6
N/m2
x
L)
+
(ot,
x
2
x
1.5
x
10-
x
L)
:.
3.5
x
io6
x
150
x
10-3~
=
(35
x
io6
x
2
x
4
x
10-3
L)+
(ut,
x
2
x
1.5
x
10-3
x
L)
..
525
x
lo6
=
280
x
lo6
+
3ut2
lo6
(525
-
280)
3
ot,
=
..
or,
=
82MN/m2
The change in strain on the outside of the cylinder and on the inside of the
tape
must
be
equal:
or2
-
or1
change in strain in tape
=
___
E,
CHI
original strain in cylinder walls
=
-
E,
(Since there is no pressure in the cylinder in the original condition there
will
be
no
longitudinal stress.)
