Hearn E.J. Mechanics of Materials. Volume 1
Подождите немного. Документ загружается.


vi
Contents
2.2
Compound bars
-
‘<equivalent
”
or “combined” modulus
2.3
Compound bars subjected to temperature change
2.4
Compound bar (tube and rod)
2.5
Compound bars subjected
to
external load and temperature effects
2.6
Compound thick cylinders subjected
to
temperature changes
Examples
Problems
3
Shearing Force and Bending Moment Diagrams
Summary
3.1
Shearing force and bending moment
3.1.1
Shearing force
(S.F.)
sign convention
3.1.2
Bending moment (B.M.) sign convention
3.2
S.F.
and B.M. diagrams for beams carrying concentrated loads only
3.3
S.F.
and B.M. diagrams for uniformly distributed loads
3.4
S.F.
and B.M. diagrams for combined concentrated and uniformly
distributed loads
3.5
Points
of
contrafexure
3.6
Relationship between
S.F.
Q,
B.M.
M,
and intensity
of
loading
w
3.1
S.F.
and B.M. diagrams for an applied couple or moment
3.8
S.F.
and B.M. diagrams for inclined loa&
3.9
Graphical construction of
S.F.
and B.M. diagrams
3.10
S.F.
and B.M. diagrams for beams carrying distributed loads of
increasing value
3.1
1
S.F.
at points of application of concentrated loads
Examples
Problems
4
Bending
Summary
Introduction
4.1
Simple bending theory
4.2
Neutral axis
4.3
Section modulus
4.4
Second moment of area
4.5
4.6
4.1
Skew loading
4.8
Bending of composite or fitched beams
Reinforced concrete beams -simple tension reinforcement
Combined bending and direct stress -eccentric loading
29
30
32
34
34
34
39
41
41
41
42
42
43
46
47
48
49
50
52
54
55
55
56
59
62
62
63
64
66
68
68
70
71
73
74

Contents
vii
4.9
4.10
Shear stresses owing
to
bending
4.1
1
Strain energy in bending
4.12
Limitations of the simple bending theory
“Middle-quarter
”
and “middle-third
”
rules
Examples
Problems
5
Slope
and Deflection
of
Beams
Summary
Introduction
5.1
5.2
Direct integration method
5.3
MacaulayS method
5.4
Macaulay’s method for u.d.ls
5.5
Macaulay’s method for beams with u.d.1. applied over part of the beam
5.6
Macaulay’s method for couple applied at a point
5.7
Mohr’s “area-moment” method
5.8
Principle of superposition
5.9
Energy method
5.10
Maxwell’s theorem
of
reciprocal displacements
5.1
1
Continuous beams
-
CIapeyron’s “three-moment
”
equation
5.12
Finite difference method
5.13
Defections due
to
temperature effects
Relationship between loading, S.F., B.M., slope and akfection
Examples
Problems
6
Built-in Beams
Summary
Introduction
6.1
Built-in beam carrying central concentrated load
6.2
Built-in beam carrying uniformly distributed load across the span
6.3
Built-in beam carrying concentrated load offset from the centre
6.4
Built-in beam carrying a non-uniform distributed load
6.5
Advantages and disadvantages of built-in beams
6.6
Effect
of
movement of supports
Examples
Problems
76
77
78
78
79
88
92
92
94
94
97
102
105
106
106
108
112
112
112
115
118
119
123
138
140
140
141
141
142
143
145
146
146
147
152

Contents
...
Vlll
7
Shear
Stress
Distribution
Summary
Introduction
7.1
7.2
7.3
7.4
7.5
7.6
Distribution of shear stress due to bending
Application to rectangular sections
Application
to
I-section beams
7.3.1
Vertical shear in the web
7.3.2
Vertical shear in the flanges
7.3.3
Horizontal she& in the flanges
Application
to
circular sections
Limitation of shear stress distribution theory
Shear centre
Examples
Problems
8
Torsion
Summary
8.1
Simple torsion theory
8.2
Polar second moment of area
8.3
Shear stress and shear strain in shafts
8.4
Section modulus
8.5
Torsional rigidity
8.6
Torsion
of
hollow shafts
8.7
Torsion of thin-walled tubes
8.8
Composite shafts -series connection
8.9
Composite shafts -parallel connection
8.10
Principal stresses
8.1
1
Strain energy in torsion
8.12
Variation of data along shaft length -torsion of tapered shafts
8.13
Power transmitted by shafts
8,14
Combined stress systems -combined bending and torsion
8.15
Combined bending and torsion
-
equivalent bending moment
8.16
Combined bending and torsion -equivalent torque
8.17
Combined bending, torsion and direct thrust
8.18
Combined bending, torque and internal pressure
Examples
Problems
154
154
155
156
157
158
159
159
160
162
164
165
166
173
176
176
177
179
180
181
182
182
182
182
183
184
184
186
186
187
187
188
189
189
190
195

Contents
9
Thin Cylinders and Shells
ix
198
Summary
9.1
Thin cylinders under internal pressure
9.1.1
Hoop or circumferential stress
9.1.2
Longitudinal stress
9.1.3
Changes in dimensions
Thin rotating ring or cylinder
Thin spherical shell under internal pressure
9.3.1
Change in internal volume
Vessels subjected
to
JIuid pressure
Cylindrical vessel with hemispherical end
Effects
of
end plates and joints
Examples
Problems
9.2
9.3
9.4
9.5
9.6
9.7
Wire-wound thin cylinders
10 Thick cylinders
Summary
10.1
10.2
10.3
10.4
Longitudinal stress
10.5
Maximum shear stress
10.6
Change
of
cylinder dimensions
10.7
10.8
10.9
Compound cylinders
10.10
Compound cylinders -graphical treatment
10.1
1
Shrinkage or interference allowance
10.12
Hub on solid shaji
10.13
Force
fits
10.14
Compound cylinder -different materials
10.15
Uniform heating
of
compound cylinders
of
different materials
10.16
Failure theories -yield criteria
10.17
Plastic yielding
-
“auto-frettage”
10.18
Wire-wound thick cylinders
Difference in treatment between thin and thick cylinders -basic
assumptions
Development
of
the Lame theory
Thick cylinder
-
internal pressure only
Comparison with thin cylinder theory
Graphical treatment
-
Lame line
Examples
Problems
198
198
199
199
200
20
1
202
203
203
204
205
206
208
213
215
215
216
217
219
220
22
1
22
1
222
223
224
226
226
229
229
230
23
1
233
233
234
236
25
1
X
Contents
11
Strain Energy
254
Summary
Introduction
1
1.1
1
1.2
Strain energy -shear
1
1.3
Strain energy -bending
1
1.4
Strain energy
-
torsion
1
1.5
Strain energy of a three-dimensional principal stress system
1
1.6
Volumetric or dilatational strain energy
1
1.7
Shear or distortional strain energy
1
1.8
Suddenly applied loads
1
1.9
Impact loads -axial load application
1
1.10
Impact loads -bending applications
1
1.1
1
Castigliano’s first theorem for deflection
1
1.12
“Unit-load
”
method
1
1.13
Application of Castigliano
’s
theorem
to
angular movements
1
1.14
Shear deflection
Examples
Problems
Strain energy
-
tension or compression
12
Springs
Summary
Introduction
12.1
Close-coiled helical spring subjected to axial load
W
12.2
Close-coiled helical spring subjected
to
axial torque
T
12.3
Open-coiled helical spring subjected
to
axial load
W
12.4
Open-coiled helical spring subjected to axial torque
T
12.5
Springs in series
12.6
Springs in parallel
12.7
12.8
12.9
Allowable stresses
12.10
Leaf or carriage spring: semi-elliptic
12.1
1
Leaf or carriage spring: quarter-elliptic
12.12
Spiral spring
Limitations
of
the simple theory
Extension springs
-
initial tension
Examples
Problems
254
256
257
259
260
26
1
262
262
263
263
264
265
266
268
269
269
274
292
297
297
299
299
300
30
1
304
305
306
306
307
308
309
312
314
316
324

Con
tents
13
Complex Stresses
Summary
1
3.1
Stresses
on
oblique planes
13.2
Material subjected
to
pure shear
13.3
Material subjected
to
two mutually perpendicular direct stresses
13.4
Material subjected
to
combined direct and shear stresses
13.5
Principal plane inclination in terms of the associated principal stress
13.6
Graphical solution
-
Mohr
's
stress circle
13.7
Alternative representations
of
stress distributions at a point
1
3.8
Three-dimensional stresses -graphical representation
Examples
Problems
xi
326
326
326
327
329
329
33
1
332
334
338
342
358
14 Complex Strain and the Elastic Constants
Summary
14.1
Linear strain for tri-axial stress state
14.2
Principal strains in terms of stresses
14.3
Principal stresses in terms of strains -two-dimensional stress system
14.4
Bulk modulus
K
14.5
Volumetric strain
14.6
14.1
14.8
Effect of lateral restraint
14.9
14.10
Strains
on
an oblique plane
14.1
1
Principal strain
-
Mohr
s
strain circle
14.12
Mohr
's
strain circle -alternative derivation from the
14.13
Relationship between Mohr
's
stress and strain circles
14.14
Construction of strain circle from three known strains
14.15
Analytical determination of principal strains from rosette readings
14.16
Alternative representations of strain distributions at a point
14.1
I
Strain energy of three-dimensional stress system
Volumetric strain for unequal stresses
Change in volume of circular bar
Relationship between the elastic constants
E,
G,
K
and
v
general stress equations
(McClintock method) -rosette analysis
Examples
Problems
361
36
1
36
1
362
363
363
363
364
365
366
361
310
312
3 74
375
318
38
1
383
385
387
397
15
Theories
of
Elastic Failure
401
Summary
Introduction
401
40
1

xii
Contents
15.1
15.2
15.3
15.4
15.5
15.6
15.7
15.8
15.9
Maximum principal stress theory
Maximum shear stress theory
Maximum principal strain theory
Maximum total strain energy per unit volume theory
Maximum shear strain energy per unit volume (or distortion energy)
theory
Mohr
's
modijied shear stress theory for brittle materials
Graphical representation of failure theories for two-dimensional
stress systems (one principal stress zero)
Graphical solution of two-dimensional theory of failure problems
Graphical representation of the failure theories for three-dimensional
stress systems
15.9.1
Ductile materials
15.9.2
Brittle materials
15.10
Limitations of the failure theories
15.1
1
Eflect of stress concentrations
15.12
Safety factors
15.13
Modes of failure
Examples
Problems
16
Experimental
Stress
Analysis
Introduction
16.1
Brittle lacquers
16.2
Strain gauges
16.3
Unbalanced bridge circuit
16.4
Null balance or balanced bridge circuit
16.5
Gauge construction
16.6
Gauge selection
16.7
Temperature compensation
16.8
Installation procedure
16.9
Basic measurement systems
16.10
D.C.
and
A.C.
systems
16.11
Other types of strain gauge
16.12
Photoelasticity
16.13
Plane-polarised light
-
basic polariscope arrangements
16.14
Temporary birefringence
16.15
Production of fringe patterns
16.16
Interpretation
of
fringe patterns
16.17
Calibration
402
403
403
403
403
404
406
410
41
1
41
1
412
41 3
414
414
41
6
417
427
430
430
43 1
43
5
437
437
437
438
439
440
441
443
444
445
446
446
448
449
450
Contents
xiii
16.18
Fractional fringe order determination
-
compensation techniques
16.19
Isoclinics
-
circular polarisation
16.20
Stress separation procedures
16.21
Three-dimensional photoelasticity
16.22
Reflective coating technique
16.23
Other methods
of
strain measurement
Bibliography
Appendix
1.
Typical mechanical and physical pro'prties
for
engineering
materials
Appendix
2.
Typical mechanical properties
of
non-metals
Appendix
3.
Other properties
of
non-metals
45
1
452
454
454
454
456
456
xxi
xxii
xxiii
Index
xxv
CHAPTER
1
SIMPLE
STRESS
AND
STRAIN
1.1.
Load
In any engineering structure or mechanism the individual components will be subjected to
external forces arising from the service conditions or environment in which the component
works. If the component or member is in equilibrium, the resultant of the external forces will
be
zero but, nevertheless, they together place a load on the member which tends to deform
that member and which must be reacted by internal forces which are set up within the
material.
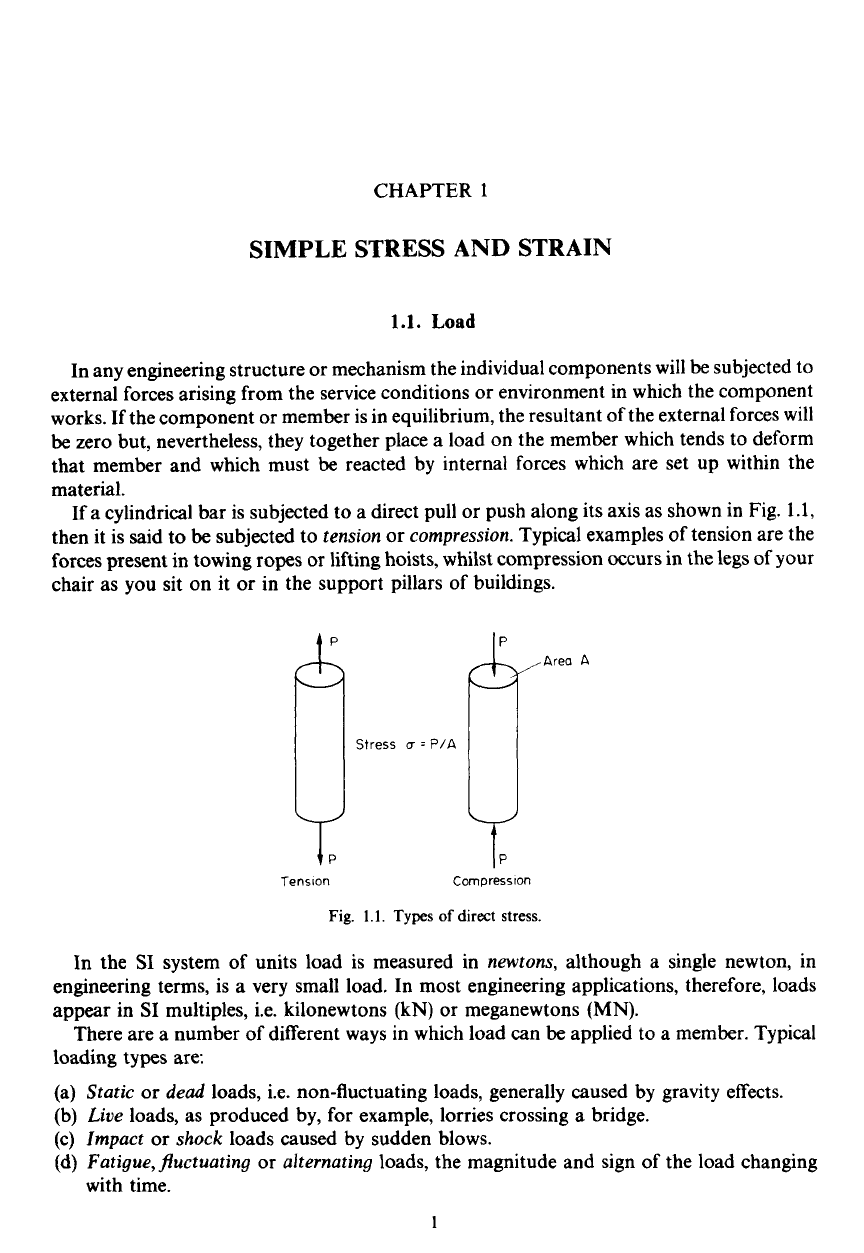
If a cylindrical bar
is
subjected to a direct pull or push along its axis as shown in Fig.
1.1,
then it is said to
be
subjected to
tension
or
compression.
Typical examples of tension are the
forces present in towing ropes or lifting hoists, whilst compression occurs in the legs of your
chair as you sit on it or in the support pillars of buildings.
,Are0
A
Tension
Compression
Fig.
1.1.
Types
of
direct
stress.
In the
SI
system
of
units load is measured in
newtons,
although a single newton, in
engineering terms, is a very small load. In most engineering applications, therefore, loads
appear in SI multiples, i.e. kilonewtons (kN) or meganewtons
(MN).
There are a number of different ways in which load can
be
applied to a member. Typical
loading
types
are:
(a)
Static
or
dead
loads, i.e. non-fluctuating loads, generally caused
by
gravity effects.
(b)
Liue
loads, as produced by, for example, lorries crossing a bridge.
(c)
Impact
or
shock
loads caused
by
sudden blows.
(d)
Fatigue,fluctuating
or
alternating
loads, the magnitude and sign of the load changing
with time.
1

2
Mechanics
of
Materials
$1.2
1.2. Direct or normal stress
(a)
It has been noted above that external force applied to a body in equilibrium is reacted by
internal forces set up within the material. If, therefore, a bar is subjected to a uniform tension
or compression, i.e. a direct force, which is uniformly or equally applied across the cross-
section, then the internal forces set up are also distributed uniformly and the bar is said to
be
subjected to
a
uniform
direct or normal stress,
the stress being defined
as
load
P
stress
(a)
=
-
=
-
area
A
Stress
CT
may thus be compressive or tensile depending on the nature of the load and will
be
measured in units of newtons per square metre (N/mZ) or multiples of this.
In some cases the loading situation is such that the stress will vary across any given section,
and in such cases the stress at any point is given by the limiting value
of
6P/6A
as
6A
tends to
zero.
1.3.
Direct strain
(E)
If a bar is subjected to a direct load, and hence a stress, the bar will change in length. If the
bar has an original length
L
and changes in length by an amount
6L,
the
strain
produced is
defined as follows:
change
in
length
6L
strain
(E)
= =-
original length L
Strain is thus a measure of the deformation of the material and is non-dimensional, Le. it has
no units; it is simply a ratio of two quantities with the same unit (Fig.
1.2).
Strain
C=GL/L
Fig.
1.2.
Since, in practice, the extensions of materials under load are very small, it is often
i.e.
microstrain,
when the
convenient to measure the strains in the form of strain
x
symbol used becomes
/ALE.
Alternatively, strain can be expressed
as
a
percentage strain
6L
L
strain
(E)
=
-
x
100%
i.e.
1.4.
Sign convention for direct
stress
and strain
Tensile stresses and strains are considered POSITIVE in sense producing an
increase
in
length. Compressive stresses and strains are considered NEGATIVE in sense producing a
decrease
in length.
