Haug H., Koch S. Quantum theory of the optical and electronic properties of semiconductors

Подождите немного. Документ загружается.

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2

Polaritons 199

meaning. Instead, the Poynting vector has to be calculated, from which one

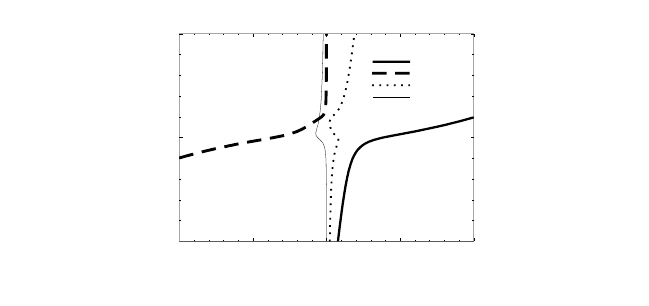
gets an energy velocity. At still lower frequency the branch ω

continues

as a Tscherenkov mode with c

>c/n

. But again this has no physical

signiﬁcance due to the very large damping. The mode ω

is damped in

the range of the LT-splitting and becomes photon-like with little damping

below the exciton resonance, ω/ω

=1.

0.5

1.5

-10

-5 0 5 10

-Im k

Re k

Re w

Im w

Re w

Im w

Fig. 11.3 Polariton dispersion with spatial dispersion and damping for ∆/ω

=0.1,

γ/ω

=0.01, ω



/2Mc

=0.001. The frequency ω is in units of ω

and k is in units

of c/ω

, respectively.

If one considers the inﬂuence of the interface between the crystal and,

e.g., vacuum, the presence of two propagating modes requires an additional

boundary condition (ABC). Often Pekar’s additional boundary condition

is chosen which states that the normal component of the polarization has

to be zero at the interface. The need for the additional boundary con-

dition stems from the fact that one has used a three dimensional spatial

Fourier transform, which is not allowed for a crystal with a surface. To

avoid this problem, one has to calculate the two-point polarization func-

tion P

(r, r



,t) for a spatially inhomogeneous system.

11.2 Hamiltonian Theory of Polaritons

Polaritons can also be discussed directly as mixed exciton–photon excita-

tions in a semiconductor. For this end, we will introduce exciton creation

and destruction operators, which, as we will show, obey Bose commuta-

tion relations in the linear regime. For the subsequent calculations, it is

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2

200 Quantum Theory of the Optical and Electronic Properties of Semiconductors

convenient to introduce a hole operator

†

−k,−s

≡ a

v,k,s

(11.21)

indicating that the annihilation of an electron with (k, s) in the valence

band corresponds to the creation of a hole with the opposite momentum

and spin. To keep the notation symmetrical, we deﬁne

†

k,s

≡ a

†

c,k,s

, (11.22)

where the electron operators α, α

†

operate exclusively in the conduction

band and the hole operators β, β

†

operate in the valence band, respectively.

Suppressing the spin index, and using the electron–hole pair operator

†

−k



, (11.23)

the operator describing generation of an exciton in the state ν with total

momentum K can be written as

†

ν,K



k,k





K − (k − k



)





k + k



†

−k





(k − K/2)α

†

K−k

. (11.24)

The derivation of this relation is best obtained in the Dirac representation,

where

†

ν,K

= |νK0| . (11.25)

Multiplying Eq. (11.25) with the completeness relation in terms of the

electron–hole pairs



k,k



|k, −k



k, −k



| =1 , (11.26)

one gets

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2

Polaritons 201

†

ν,K



k,k



|k, −k



k, −k



|νK0|



k,k



k, −k



|νK|k, −k



0|



k,k



k, −k



|νKα

†

−k



. (11.27)

Furthermore,

k, −k



|νK =





k, −k



|r, r



r, r



|νK





−ik·r



·r



iK·(r+r



)/2

(r − r



)

= δ[K − (k − k



)]ψ



k + k



, (11.28)

where ψ

(k) is the Fourier transform of the exciton wave function for the

relative motion. Explicitly, the Fourier transforms for the ground-state

wave functions are

(k)=8



πa

[1 + (ka

)

]

in 3D (11.29)

and

(k)=

√

2πa

[1 + (ka

/2)

]

3/2

in 2D. (11.30)

We see that ψ

(k) is roughly constant for 0 <k<1/a

or 2/a

, and falls

oﬀ rapidly for large k values.

The commutator for the exciton operators is

0,0

†

0,0



k,k



(k)ψ

∗



)



−k

,α

†



†

−k







|ψ

(k)|

(1 − α

†

− β

†

−k

) (11.31)

so that

[B

0,0

†

0,0

] =1−O(na

). (11.32)

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2

202 Quantum Theory of the Optical and Electronic Properties of Semiconductors

This is a Bosonic commutator relation for a vanishing number of electrons

and holes, i.e. in the linear regime. At elevated densities, however, one has

to take the deviations from the Bosonic nature into account.

The Hamiltonian for free excitons can be written as



e

νk

†

ν,k

. (11.33)

In order to express the interaction Hamiltonian H

of the electron system

with the light ﬁeld in terms of exciton operators, we multiply Eq. (11.24)

with ψ

∗

(κ) and sum over ν to get



∗

(κ)B

†

ν,K



νk

∗

(κ)ψ

(k − K/2)α

†

K−k



κ,k−K/2

†

K−k

= α

†

K+κ

†

K−κ

. (11.34)

Taking the ﬁnite wave number q of the light explicitly into account, H

can

then be written as

= −



k,q



†

q+k

†

q−k

E(q)e

−iω

+h.c.



= −



k,q,ν



(k)B

†

ν,q

E(q)e

−iω

+h.c.



. (11.35)

Using

E(q,t)=−

∂

∂t

A(q,t) , (11.36)

we obtain

E(q,t)=E(q)e

−iω

= i



2πω

− h.c.) , (11.37)

where we denote the photon annihilation operator by b

(see Appendix A),

and

√



. (11.38)

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2

Polaritons 203

The exciton–photon interaction Hamiltonian in the resonant approximation

is thus

= −i



q,ν

νq

†

νq

− h.c.) (11.39)

with the optical matrix element

g

νq

= d

∗

(r =0)



πω

/2 . (11.40)

The total exciton–photon Hamiltonian is now

H=







νq

†

νq

+ ω

†

−i



νq

†

νq

−h.c.)



. (11.41)

In the following, we consider only the lowest exciton level. The bilinear

Hamiltonian (11.41) can be diagonalized by introducing polariton operators

as linear combination of exciton and photon operators :

= u

+ v

. (11.42)

We demand that the polariton operators obey the Bose commutation rela-

tions

†

]=|u

+ |v

=1 . (11.43)

We now choose the unknown coeﬃcients u

and v

so that the Hamiltonian

(11.41) becomes diagonal in the polariton operators

H = 



Ω

†

. (11.44)

It turns out that the transformation coeﬃcients u

and v

and the polariton

spectrum Ω

can best be found by evaluating the commutator [p, H]/ once

directly using the polariton Hamiltonian (11.44) and once using Eq. (11.42)

together with the exciton–photon Hamiltonian. We obtain

Ω

=Ω

+ v

)

= u

− ig

)+v

(ω

+ ig

) . (11.45)

Comparing the coeﬃcients of B

and b

we ﬁnd

0=(Ω

− e

+ ig

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2

204 Quantum Theory of the Optical and Electronic Properties of Semiconductors

and

0=−ig

+(Ω

− ω

, (11.46)

respectively. The determinant of the coeﬃcients of u

and v

has to vanish,

i.e,

(Ω

− e

)(Ω

− ω

) − g

=0 ,

Ω

q,1,2

+ ω

) ±



− ω

)

+4g

. (11.47)

polariton spectrum

We see immediately that Ω

1,2

are the frequencies of the upper and lower

polariton branches which we found already in Sec. 11.1. For q → 0,the

upper branch approaches e

+ g

showing that the LT splitting in this

formulation is given by g

, which vanishes for g from Eq. (11.40).

Using Eqs. (11.43) and (11.46), we ﬁnd for the upper polariton branch

q,1



Ω

q,1

− ω

2Ω

q,1

− e

− ω

and v

q,1

= i



Ω

q,1

− e

2Ω

q,1

− e

− ω

(11.48)

andforthelowerpolaritonbranchwithΩ

<e,ωthe coeﬃcients

q,2



Ω

q,2

− ω

2Ω

q,2

− e

− ω

and v

q,2

= −i



Ω

q,2

− e

2Ω

q,2

− e

− ω

. (11.49)

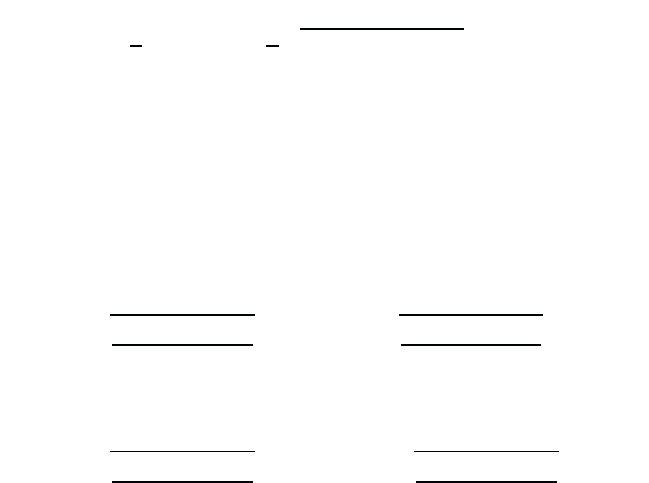
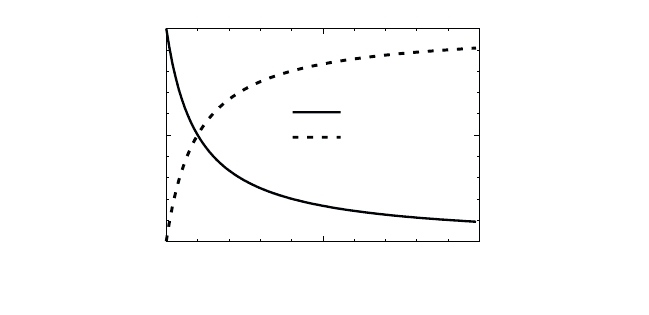
Fig. (11.4) shows the wave number dependence of |u

and |v

for the up-

per polariton branch according to Eq. (11.48), for simplicity without spatial

dispersion, i.e., with e

= e

. The upper branch polariton is exciton-like

for small q-values, (|u

 1), and changes around the exciton resonance

successively into a photon-like excitation (|v

 1). The lower branch

polariton shows the reverse properties.

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2

Polaritons 205

0.5

5 10

|u |

|v |

qc/e n

Fig. 11.4 Dispersion of |u

and |v

for the upper polariton branch.

The resulting polariton Hamiltonian is

H = 



q,i=1,2

Ω

i,q

†

i,q

. (11.50)

All formulas derived in this chapter hold only if the nonresonant interaction

terms are small. The full interaction Hamiltonian with nonresonant terms

has the form

= i



+ B

†

−q

)(b

−q

− b

†

) . (11.51)

The total Hamiltonian can still be diagonalized by a transformation which

mixes B

, b

and B

†

−q

, b

†

−q

operators linearly :

i,q

= u

i,1,q

+ u

i,2,q

+ u

i,3,q

†

−q

+ u

i,4,q

†

−q

. (11.52)

This slightly more general transformation is called the Hopﬁeld (1958) po-

lariton transformation.

If we compare the dielectric formalism used at the beginning of this

chapter with the diagonalization procedure of this section, we see that an

advantage of the ﬁrst approach is that it allows us quite naturally to include

the damping of the modes.

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2

206 Quantum Theory of the Optical and Electronic Properties of Semiconductors

11.3 Microcavity Polaritons

Even though we did not specify it explicitly, the polariton properties dis-

cussed so far are valid for inﬁnitely extended bulk materials. Modiﬁcations

are necessary when we deal with quantum conﬁned systems, such as quan-

tum wells and wires. In these cases, free propagation is possible only in the

unconﬁned directions, leading to two- or one-dimensional polariton modes.

More interesting are conﬁgurations with quantum conﬁnement not only

for the electrons but also for the photons. Here, the conﬁnement for the

photons can be provided by converting two parallel end faces of a semi-

conductor crystal into mirrors of very high reﬂectivity. This generates an

optical resonator, i.e., a Fabry Perot cavity, which has eigenmodes deter-

mined by the length L of the cavity and the quality of the mirrors. In the

simplest case, these mirrors could be made with metallic ﬁlms, but often

one uses so-called distributed Bragg reﬂectors (DBR mirrors) that are made

of alternating layers of low and high refractive index material with a layer

thickness of a quarter of the wave length of light.

If L equals half a wave length of light, one has a one-dimensional mi-

crocavity where only one longitudinal optical mode is allowed. This mode

is just a standing wave with nodes at both mirrors and a single maximum

in the middle of the sample, while parallel to the mirrors one has still a

continuum of transverse running modes with a wave vector k



To determine the microcavity mode, we start with the wave equation

(1.43) in the form

∆E(r,ω)+

(ω)E(r,ω)=0 , (11.53)

where we assume a situation without any resonances in the spectral range of

interest, so that the dielectric function is purely real,  = n

.Thesolution

for the lowest mode of the microresonator is

E(r,ω)=E

sin(

πz



·r

. (11.54)

Inserting (11.54) into the wave equation (11.53), we ﬁnd the eigenfrequen-

cies

0,k





+ k



. (11.55)

Note, that in contrast to the dispersion of bulk photons, the dispersion of

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2

Polaritons 207

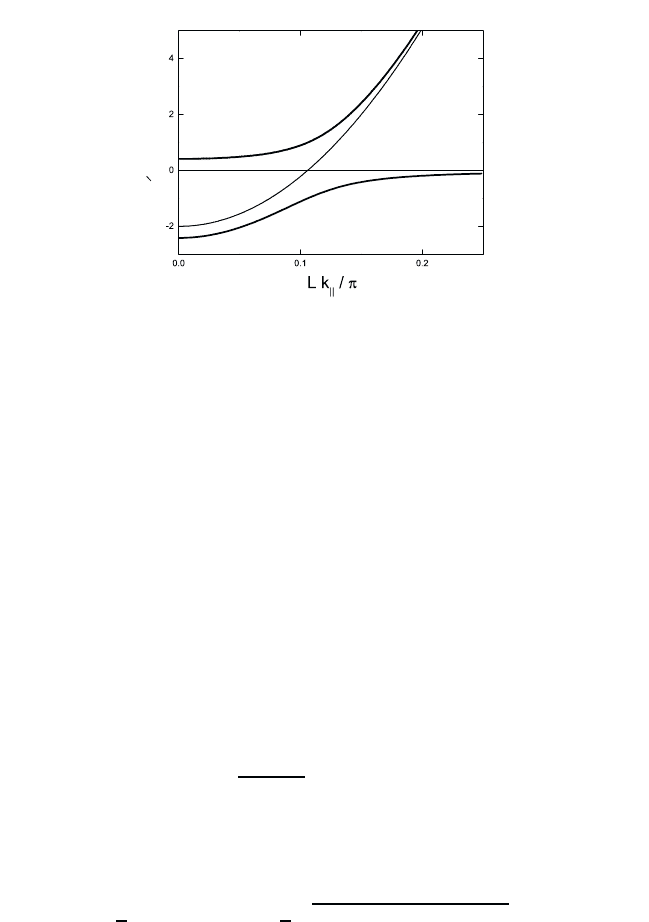
h( - e )/EW

Fig. 11.5 Exciton polariton dispersion of a planar microcavity versus parallel momen-

tum k



. The thin lines show the bare caving photon and exciton dispersions.

the microcavity polaritons (11.55) approaches a ﬁnite frequency in the long

wave-length limit k



→ 0.

Next, we consider the interaction of this light mode with a 2D quan-

tum well placed in the middle of the microcavity, i.e. at z = L/2,where

the optical mode has its maximum. If one couples the 1s-excitons of this

quantum well to the photons of the microcavity mode, one gets in analogy

with (11.41) the following resonant exciton–photon Hamiltonian

H = 







0,k



†

0,k



0,k



+ ω

0,k



†

0,k



0,k



− ig



†

0,k



0,k



− h.c.)



(11.56)

Here, in analogy with (11.40), the interaction matrix element is given by

g



= d

(r =0)



πω

0,k



. (11.57)

Applying the same diagonalization procedure as discussed in the last sec-

tion, we get the following microcavity polariton spectrum

Ω



,1,2

0,k



+ ω

0,k



) ±



0,k



− ω

0,k



)

+4g



. (11.58)

A sketch of the resulting microcavity polariton is given in Fig. 11.5 for a

cavity mode which is degenerate at k



=0with the 1s-exciton. One sees

January 26, 2004 16:26 WSPC/Book Trim Size for 9in x 6in b ook2

208 Quantum Theory of the Optical and Electronic Properties of Semiconductors

that in contrast to the bulk polariton both branches of the microresonator

polariton tend to a ﬁnite frequency at k



→ 0 which gives these structures

unique optical properties.

REFERENCES

The polariton concept has been introduced by:

J.J. Hopﬁeld, Phys. Rev. 112, 1555 (1958)

For a discussion of the ABC problem see:

V.M. Agranovich and V.L. Ginzburg, Crystal Optics with Spatial Dispersion

and Excitons, Springer Verlag, Berlin (1984)

K. Cho, Excitons, Topics in Current Physics, Vol. 14, Springer Verlag,

Berlin (1979)

A.StahlandI.Balslev,Electrodynamics of the Semiconductor Band Edge,

Springer Tracts in Modern Physics 110, Springer Verlag, Berlin (1987)

For a full numerical solution of the ABC problem and detailed comparisons

to experiments see:

H.C. Schneider, F. Jahnke, S.W. Koch, J. Tignon, T. Hasche, and D.S.

Chemla, “Polariton Propagation in High-Quality Semiconductors — Micro-

scopic Theory and Experiment versus Additional Boundary Conditions”,

Phys. Rev. B63, 045202 (2001)

For a discussion of many aspects of semiconductor microcavity systems and

a detailed list of references see:

G. Khitrova, H.M. Gibbs, F. Jahnke, M. Kira, and S.W. Koch, “Semicon-

ductor Physics of Quantum Wells and Normal-Mode Coupling Microcavi-

ties”, Rev. Mod. Phys. 71, 1591 (1999)

PROBLEMS

Problem 11.1: Generalize Eq. (11.20) to include the energetically higher

(n =2, 3,...) exciton levels.

Problem 11.2: Compute the Fourier transform of the 1s-exciton wave