Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.

Theorem
for
regular
Sturm-Liouville
systems
16.2 STURM--L1DUVILLE
SYSTEMS
AND
SDLDES
513
Hence, zero is not an eigenvalue of K, i.e., Mo = {D). Since eigenvectors
of
K = L;
1
coincidewith eigenvectors of
Lx,
andeigenvalues of
Lx
arethe
reciprocals
of the eigenvalues of K,we have the following result.
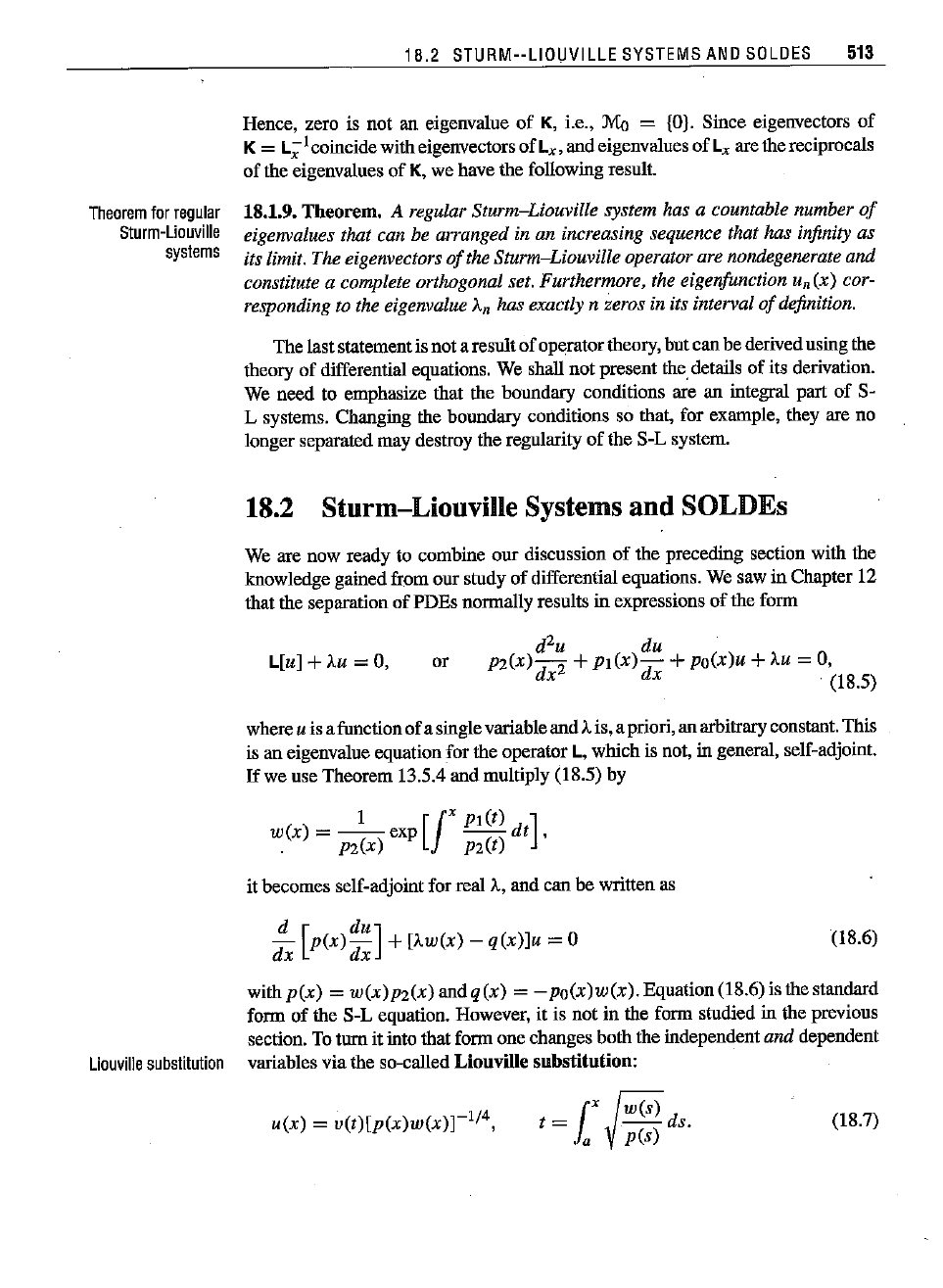
18.1.9.
Theorem.
A regular Sturm-Liouville system has a countable number
of
eigenvalues that can be arranged in an increasing sequence that has infinityas
its limit. The eigenvectors
of
the Sturm-Liouville operator are nondegenerate and
constitute a complete orthogonal set. Furthermore, the eigenfunction
Un
(x) cor-
responding to the eigenvalue
An
has exactly n zeros in its interval
of
definition.
The laststatementis not a resultof operatortheory, but can be derivedusing the
theory of differential equations. We shall not present the,details of its derivation.
We need to emphasize that the boundary conditions are an integral part of S-
L systems. Changing the boundary conditions so that, for example, they are no
longer separated may destroy the regularity of the SoL system.
18.2 Sturm-LiouvilleSystems andSOLDEs
We are now ready to combine
our
discussion of the preceding section with the
knowledge gained from our study of differential equations. We saw in Chapter
12
that the separation
of
PDEs normally results in expressions
of
the form
L[u]+
AU
= 0, or
d
2u
du
P2(x)d
2 +
PI(x)-d
+
po(x)u
+
AU
= 0,
x x (18.5)
(18.6)
where
uis afunctionof a single variableand Ais, apriori, an arbitraryconstant. This
is an eigenvalue equation for the operator L,which is not, in general, self-adjoint.
lfwe
use Theorem 13.5.4 and multiply (18.5) by
1
[fX
PI(t)
]
w(x)
=
--exp
--dt,
. P2(X) P2(t)
it becomes self-adjoint for real A,and can be written as
d [ dU]
dx
p(x)
dx
+
[AW(X)
-
q(x)]u
= 0
withp(x)
=
w(x)p2(x)andq(x)
=
-po(x)W(x).
Equation(18.6) is the standard
form of the SoL equation. However, it is not in the form studied in the previous
section. Toturn it into that form one changes both the independent
and dependent
Liouville
substitution
variables
via
the so-called Lionville
substitntion:
u(x)
=
v(t)[p(x)w(x)r
1/4,
_lX~(S)d
t - . s.
a pes)
(18.7)

514 18. STURM-LIOUVILLE SYSTEMS: FORMALISM
(18.8)
It
is
then
a
mailer
of
chain-ruledifferentiationto
show
that
Equation
(18.6)
hecomes
d
2
v
dt
2
+
[J.
-
Q(t)]v
= 0,
where
Q(t)
=
q(x(t))
+
[P(X(t))W(X(t))]-1/4
d
d:
[(pw)I/4].
w(x(t))
t
Therefore,
Theorem
18.1.9 still holds.
JosephLiouville (1809-1882) was a highly respecled professor
at the College de France, in Paris,
and
the
founder and editor
of
theJournaldes Mathbnatiques Pures et Appliquees, afamouspe-
riodical that
played
an importantrole in Frenchmathematicallife
through the latterpart
of
the nineteenth century. His
own
remark-
ableachievements as acreativemathematicianhaveonlyrecently
received the appreciation they deserve.
He was the first to solve a
boundary
value
problem
by solving
an equivalentintegral equation. His ingenious theory
of
fractional
differentiation answered the long-standing question
of
what
rea-
sonable meaning
can
be assigned to
the
symbol any/dx
n
when n is
not
a positive integer.
He discovered the fundamental result
in
complex
analysis that a
bounded
entire function
is necessarily a constant
and
used
it as
the
basis for his own theory
of
elliptic functions.
Thereis also a well-known
Liouvilletheoremin Hamiltonianmechanics,
which
states
that
volume integrals are time-invariant in
phase
space. In collaboration
with
Sturm, he also
investigated
the
eigenvalue
problem
of
second-orderdifferential equations.
The theory
of
transcendental numbers is anotherbranch
of
mathematics that originated
in Liouville's work. The irrationality
of
1f
and
e (the fact
that
they are
not
solutions
of
any
linear equations)
had
been
proved in
the
eighteenth century by Lambert
and
Euler.In 1844
Liouville showed
that
e is not a
root
of
any quadratic equation
with
integral coefficients
as well. This
led
him
to conjecture
that
e is transcendental, which means
that
it does not
satisfy any polynomial equation with integral coefficients.
18.2.1.
Example.
TheLiouville substitutiou [Equatiou
(18.7)1
transformsthe BesselDE
(xu')' +
(Px
- v
2/x)u
= 0 iuto
d
2
v
[2
v
2
-
1
/4]
-+
k -
v=O,
dt
2
t
2
coskt
Jl/2(kt)
= B .,ji ,
or
from
which
we can obtainaninterestingresult
when
v = !.In
that
case
we
have
v+k
2
v =0,
whose solutious are of the form
coskt
aud
siukt.
Noting that u(x) = JI/2(X), Equatiou
(18.7)gives
sinkt
JI/2(kt) = A .,ji

(18.9)
18.2 STURM--LIOUVILLESYSTEMS
ANO
SOLDES
515
andsince
h/2(X)
isanalytic
atx
= 0, wernnst have
h/2(kt)
= A
sinkt/,f!,
whichisthe
resultobtainedin
Chapter
14.
..
The appearanceof W is the resultof our desireto renderthe differential nperator
self-adjoint.
It
also appears in another context. Recall the Lagrange identity for a
self-adjoint differential operator
L: .
d , ,
uL[v]
-
vL[u]
=
-{p(x)[u(x)v
(x)
-
v(x)u
(x)]).
dx
If
we specialize this identity to the S-Lequationof(18.6) with u = Uj correspond-
ing to the eigenvalue
Al and v =
U2
corresponding to the eigenvalue A2,we obtain
for the LHS
uIL[U2] -
u2L[uIl
= Uj(-A2WU2) +U20.IWUI) = (AI - A2)WUIU2.
Integrating both sides of (18.9) then yields
(Aj - A2)
lab
WUjU2dx =
{p(x)[Uj(x)u~(x)
-
U2(X)U~
(x)])~.
(18.10)
A desired property of the solutions of a self-adjoint DE is their orthogonality
when they belong to different eigenvalues. This property will be satisfied if we
assume an inner product integral with weight function
W
(x),
and
if
the RHS
of
Equation (18.10) vanishes. There are various boundary conditions that fulfill the
latter requirement. For example,
Uj and
U2
could satisfy the boundary conditions
of Equation (18.2). Another set of appropriate boundary conditions (BC) is the
periodic
boundary
periodic
BC given by
conditions
ural = u(b) and
u'(a) = u'(b). (18.11)
However, as the following example shows, the latter BCs do
not
lead to a regular
S-L system.
18.2.2.
Example.
(a)TheS-Lsysternconsisting oftheS-Lequationd
2u/dt
2
+",2
u = 0
intheinterval[0,
T] withtheseparatedBCsu(O)= 0 andu(T) = ohastheeigenfunctions
un(t) = sin
n;
t with n =
1,2,
...
and theeigenvalues
An
=
w~
=
(nx
/T)2
with
n = 1,2,
....
(b) Letthe S-Leqnationbethesameasinpart(a)bntchange theintervalto
[-T,
+T]
and
theBCstoaperiodiconesuchas
u(
-T)
= u(T)
andu'(-T)
=
u'(T).
The
eigenvalues
are
thesameasbefore,but the eigenfunctions are1,
sin(mrt/T),
andcos(mrt/T),wheren isa
positive
integer.
Note
that
there
is a degeneracy hereinthesensethattherearetwolinearly
independent
eigenfunctions havingthesameeigenvalue(mr/
T)2.
By Theorem18.1.9,the
S-L systemis not
regular.
.
(c)The
Besselequationforagivenfixed
1)2
is
1 ( v
2
)
u"+
-;.u'
+ k
2
- x
2
u = 0,
where a
:S:X
:s:b,
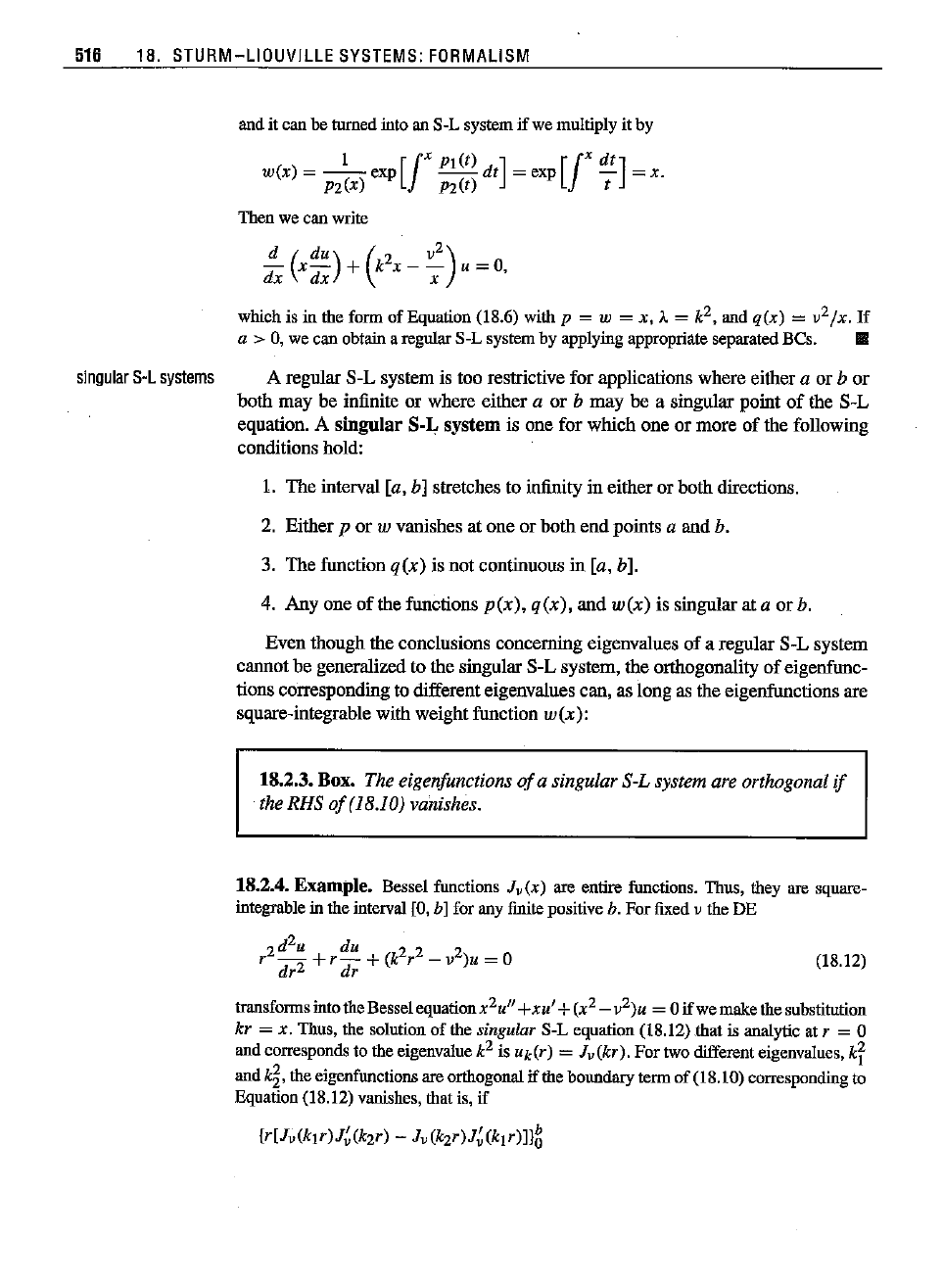
(18.12)
516 18. STURM-LIOUVILLE
SYSTEMS:
FORMALISM
anditcanbe
turned
intoanS-Lsystem
if
we
multiply
itby
I
[IX
Pl(t)]
[IX
dt]
w(x)=--exp
--dt=exp
-=x.
P2(X)
P2(t) t
Thenwe canwrite
d
(dU)
(2
v
2
)
-
x-
+ k x - - u =0,
dx dx x
whichis in theform of Equation(18.6) with P = w = x, A= k
2,
and
q(x)
= v
2/x.
If
a > 0, wecan obtainaregular
SoL
systemhy applyingappropriateseparatedBes.
III
singular
S·L
systems
A regular SoLsystem is too restrictive for applications where either a or b or
both may be infinite or where either
a or b
may
be a singular point
of
the SoL
equation. A
singular
S·L
system
is one for which one
or
more
of
the following
conditions hold:
1.
The
interval [a, b] stretches to infinity in either or both directions.
2. Either
p or w vanishes at one or both
end
points a and b.
3. The function
q(x)
is not continuous in [a, b].
4.
Anyone
of
the functions
ptx),
q(x),
and
w(x)
is singular at a
or
b.
Even though the conclusions concenting eigenvalues
of
a regular SoLsystem
cannot be generalized
to the singular SoLsystem, the orthogonality
of
eigenfunc-
tions correspondiug to different eigenvalues can, as long as the eigenfunctions are
square-integrable with weightfunction
w(x):
18.2.3. Box. The eigenfunctions
of
a singularSoLsystem are orthogonal if
the RHS of(18.10) vanishes.
18.2.4.
Example.
Bessel functions Jvex) are entire functions. Thus, they are square-
integrable in the interval[0,b] for any finitepositiveb. For fixedv the DE
2
d2u
du 2 2 2
r dr
2
+r dr +(k r - v )u = 0
transformsinto the Bessel
equationx-e"
+xu'
+(x
1_v
2)u
= o
ifwe
make the substitution
kr = x.
Thus,
the
solution
of thesingular S-L
equation
(18.12)thatis
analytic
atr = 0
and
corresponds
tothe
eigenvalue
k
2
is
uk(r)
=
JvCkr).
Fortwo
different
eigenvalues,
kr
and
k~,
theeigenfunctions areorthogonal
if
theboundarytermof (18.10)corresponding to
Equation(18.12)vanishes, thatis,
if
(r[Jv(klr)J~(k2r)
-
Jv(k2r)J~(klr)]Jg

where
- 1 < x < 1,
18.3
OTHER
PROPERTIES
OF
STURM--L10UVILLE
SYSTEMS
517
vanishes,whichwilloccurifandonlyif
Jv(kj
b)J~(k2b)
-Jv(k2b)J~(kj
b) =
O.
Acornmon
choiceis to take Jv(k]b) = 0 = Jv(k2b), that is, to take both
k]b
and k2b as (different)
rootsof the Bessel
function
of
order
v. WethushaveItr
Jv(kir)Jv(kjr)
dr
= 0
if
ki and
kj are
different
roots
of Jp(kb) =
O.
The
Legendre
equation
d [ 2
dU]
-
(I-x)-
+AU=O,
dx
dx
is aheady self-adjoint. Thus,
w(x)
= I, and
p(x)
= 1 - x
2.
The eigenfnnctionsof this
singularS-I. system[singularbecanse
p(I)
=
p(-I)
= 0] areregularattheendpoints x =
±I
andaretheLegendrepolynomialsPn(x) correspondingtoA= n(n + 1).The bonndary
termof(I8.1O) clearlyvanishes
ata
=
-1
and b = +1. SincePn(x) are square-integrable
on
[-I,
+1], we obtain the familiar orthogonality relation:
J~l
Pn(x)Pm(x)dx
= 0 if
m
i'
n.
TheHermite DEis
u"- 2xu' +
AU
=
O.
(18.13)
(18.14)
2
It is transformedinto an
SoL
systemif we multiplyit by
w(x)
=
e-
x
.
The resultingSoL
equation
is
d [ 2
dU]
2
-
e-
x
_
+
Ae-
x
u
=
O.
dx
dx
The
boundary
tenn
corresponding
to the twoeigenfunctions u1
(x)
andU2
(x)
having
the
respective
eigenvalues A1
and)..2
=1=
Al is
(e-
X2
[U](x)u2
(x)-
U2
(x)u!
(x)]J~.
This
vanishes
for
arbitrary
Uland U2
(because
theyare
Hermite
polynomials) if a =
-00
andb =
+00.
The
function
u is an eigenfunction of (18.14)
corresponding
to the eigenvalue x if
and only if it is a solution of (18.13). Solutions of this DE corresponding to A = 2n
are the Hermite polynomials H
n
(x) discussed in Chapter 7. We can therefore write
J~::
e-
x 2
Hn(x)Hm(x)
dx
= 0
ifm
i'
n. This orthogonalityrelationwasalso derivedin
Chapter7.
III
18.3 OtherPropertiesof Sturm-Liouville Systems
The
S-L
problem is central to the solution
of
many
DEs
in mathematical physics.
In
some
cases the S-L equation has a direct bearing on the physics.
For
example,
the eigenvalueAmay correspondto the orbitalangularmomentum
of
an electronin
an atom (see the treatment
of
spherical harmonics in Chapter 12) or to the energy
levels
of
a particle in a potential (see Example 14.5.2).
In
many cases, then, it
is worthwhile to gain some knowledge
of
the behavior
of
an
S-L
system in the
limit
of
large
A-high
angular momentum or
high
energy. Similarly, it is useful to
understand the behavior
of
the solutions for large values
of
their arguments. We
therefore devote this section to a discnssion
of
the behavior
of
solutions
of
an
S-L
system in the limit of large eigenvalues
and
large independent variable.

518 18. STURM-LIOUVILLE
SYSTEMS:
FORMALISM
18.3.1 Asymptotic Behavior for Large Eigenvalues
We assume that the SoLoperator has the form given in Equation (18.1). This can
always be done for an arbitrary second-order linear DE by multiplying it by a
proper function (to make it self-adjoint) followed by a Liouville substitution. So,
consider an SoL systems of the following form:
u"
+[A -
q(x)]u
==
u"
+
Q(x)u
= 0
whereQ=A-q
(18.15)
with separated
Bes
of
(18.2).
Let
us assume that
Q(x)
> 0 for all x E [a, b], that
is,
A>
q(x).
This is reasonable, since we are interested in very large
A.
The study of the system
of
(18.15)
and
(18.2) is simplified
if
we make the
PrOfer
substitution
Priifer
snbstitution:
(18.16)
(18.17)
where
R(x,
A)and
</>(x,
A)are A-dependentfunctions ofx. This substitutiontrans-
forms the SoLequation of (18.15) into a pair
of
equations (see Problem 18.3):
d</>
q'
dx = .vA -
q(x)
- 4[A _
q(x)]
sin2</>,
dR =
Rq'
cos2</>.
dx 4[A -
q(x)]
The function
R(x,
A)is assumedto be positive because any negativity
ofu
can
be transferred to the phase
</>(x,
A).Also, R carmot be zero at any point of [a, b],
because both
u and u' would vanish at that point, and, by Lemma13.3.3,
u(x)
=
O.
Equation (18.17) is very useful in discussing the asymptotic behaviorof solutions
of SoL systems both when A
-->
00
and when x -->
00.
Before we discuss such
asymptotics, we need to make a digression.
It
is often useful to have a notation for the behavior of a function j (x, A)for
large Aand all values of
x.
If
the function remains bounded for all values
of
x as
A-->
00,
we write
j(x,
A) =
0(1).
Intuitively, this means that as Agets larger and
larger, the magnitude of the function
j(x,
A)remains of order 1.
In
other words,
for no value of x is lim"....co
j(x,
A) infinite.
If
A"
j(x,
A) =
0(1),
then we can
write
j(x,
A)= O(1)/A". This means that as Atends to infinity,
j(x,
A)
goes to
zero as fast as I/A" does. Sometimes this is written as
j(x,
A) = OrA
-").
Some
properties of
0(1)
are as follows:
1.
If
a is a finite real number, then
0(1)
+a =
0(1).
2.
0(1)
+
0(1)
=
0(1),
and
0(1)0(1)
=
0(1).
3. For finite a and b,
J:
0(1)
dx =
0(1).
4.
If
r and s are real numbers with r
::s
s, then
O(I)A'
+
O(I)A'
= o(1)A'.
.'

18.3
OTHER
PROPERTIES
OF
STURM--L10UVILLE
SYSTEMS
519
5.
If
g(x)
is any bounded function
of
x,
then
a Taylor series expansion yields
[A
+
g(x)]'
= A'
[I
+
g~)
r
, {
g(x)
r(r
- I)
[g(X)]2
O(I)}
=A
I+r-+
-
+--
A 2 A A
3
= A' +
rg(x)A,-1
+
O(l)A,-2
= A' +
O(l)A,-1
=
0(1)).'.
Returning to Equation (18.17)
and
expanding its RHSs using property 5, we
obtain
dR
0(1)
=
dx
A
Taylor series expansion
of
q,(x, A) and
R(x,
A) about x = a then yields
0(1)
q,(x, A) = q,(a, A) +(x -
a)Vi
+
Vi'
0(1)
R(x,
A) = R(a, A)+
-A-
(18.18)
for A
-+
00.
These resnlts are useful in determining the behavior
of
Anfor large
n. As an example, we use (18.2)
and
(18.16) to write
_
al
=
u'(a)
= R(a,
A)Ql/4(a,
A)cos[q,(a, A)] =
Ql/2(a,
A)cot[q,(a, A)],
Ih
u(a)
R(a,
A)Q
1/4(a, A)sin[q,(a, A)l
where we have assumedthat,81 f'
O.
If,81 = 0, we can take the ratio ,81/al, which
is finite because at least one
of
the two constants
must
be different from zero.
Let
A =
-al/,81
and write cot[q,(a, A)] =
A/.JA
q(a).
Similarly, cot[q,(b, A)] =
B/
.JA -
q(b),
where B =
-a2/.82.
Let
us concentrate on the
nth
eigenvalue and
write
-1
A
</!(a,
An) =
cot
"fAn -
q(a)
A
q,(b, An) =
coC
1
--r.;'==T,[i'
.JAn
q(b)
For
large Anthe argument
of
cot"
1
is small. Therefore, we can expandthe RHS in
a Taylor series about zero:
1 1
1f
1f
0(1)
cot"
e
ee
ccf"
(0)-£+···=--£+···=-+--
2 2
A.
for s = o
(I)/A..
It
follows that
1f
0(1)
q,(a, An) =
"2
+
A.'
1f
0(1)
q,(b, An) = - +
nit
+
1'>'
2 vAn
(18.19)

520
18. STURM-LIOUVILLE
SYSTEMS:
FORMALISM
The
term
nit
appears in (18.19)
because,
by
Theorem
18.1.9,
the
nth
eigenfunction
has
n
zeros
between
a
and
b.
Since
u = R
Q~
1/4
sin
tP,
this
means
that
sin
tP
must
go
through
n
zeros
as x
goes
from
a to b.
Thus,
at x = b
the
phase
tP
must
be
nn
largerthanatx = a.
Substituting x = b
in
the
first
equation
of
(18.18),
with
A --> An,
and
using
(18.19),
we
obtain
~
+n1l' +
0(1)
=
~
+
0(1)
+(b
-a)J):;;
+
0(1),
2
.n;
2
.;r;;
.n;
or
0(1)
(b - a)J):;; =
nn
+
1'>'
vAn
(18.20)
(18.21)
One
consequence
of
this
result
is that,
limn-->oo
nA;I/2
=
(b-a)I1l'.
Thus,.;r;;
=
Csn,
where
limn-->oo
C
n
=
1l'1(b
- a),
and
Equation
(18.20)
can
be
rewritten as
J):;;
=
~
+
0(1)
=
~
+
0(1).
b
-a
Cnn b
-a
n
00,
This
equation
describes
the
asymptotic
behavior
of
eigenvalues.
The
following
theorem,
stated
without
proof,
describes
the
asymptotic
behavior
of
eigenfunctions.
18.3.1.
Theorem.
Let
{un(x)}
~o
be the normalizedeigenfunctions
of
the regular
S-L system given by Equations
(18.15)
and
(18.2)with fhfh
i'
O.
Then.for n -->
(18.22)
where An =
n(n
+
I).
_~
n1l'(x-a)
0(1)
unix)
-
--cos
+
--.
b-a
b-a
n
18.3.2. Example. Let us derive an asymptotic formula for the Legeudre polynomials
Pn
(x). WefirstmaketheLiouvillesubstitution totransform theLegendre DE
[(I-x
2)
pI]'
+
n(n
+ I)P
n
= 0ioto
d
2v
dt
2
+
[An
-
Q(t)]v
= 0,
asymptotic
behavior
ofsolutions of
large
order
(18.23)
Here p(x) =
1-
x
2
and w(x) =
I,
so t = r
ds/~
=
cos-I
x, or x(t) =
cost,
and
Pn(x(t)) =
v(t)[I-x2(t)]-1/4
=
;(1)
.
smr
10Equation (18.22)
16.3
OTHER
PROPERTIES
OF
STURM--LIOUVILLE
SYSTEMS
521
For large n we can neglect
Q(t),
make the approximation
An
~
(n +
!)2,
and write
v+
en
+!)2
v
= 0, whose general solution is
v(t)
= A cos[(n+
~)t
+"J,
where
A and a are arbitrary constants. Substituting this solution in
(18.23)
yields
Pn(cost) = A cos[(n+
~)t
+
"J/v'sint.
To determine" we note that Pn(O) = 0
if
n
is odd.Thus,
if
we lett = 1r/2,thecosine
term
vanishesforoddn
if
a = -JC/4.Thus,the
general
asymptotic
formula for
Legendre
polynomials is
A [ I
"]
Pn(cost) =
v"
cos (n +
-)t
- - .
smt
2 4
18.3.2 Asymptotic Behaviorfor Largex
III
Liouville and Pnifer substitutions are usefulin investigating the behavior of the
solutions of S-L systems for large x as well. The generalprocedure is to transform
the DE into the form
of
Eqnation (18.8) by the Liouville substitution; then make
the Pnifersubstitution
of
(18.16) to obtain two DEs in the form
of
(18.17). Solving
Equation (18.17) when x
-+
00
determines the behavior
of
</J
and R and, subse-
quently, of
u, the solution. Problem 18.4 illustrates this procedure for the Bessel
functions. We simply quote the results:
(2
[ (
1)](
v
2
-
1/4]
0(1)
Jv(x)=y;;;;COS
x-
v+2:
2+
2x +
x5/2'
(2
. [ (
1)](
v
2
-1/4]
0(1)
Y
v
(x) = y
;;;;
sm x - v +
2:
2 + 2x + x5/2 .
These two relations easily yield the asymptotic expressions for the Hankel func-
tions:
HP)(x)
= Jv(x) +iYv(x)
{!;
{[
(
1)](
v
2
-1/4]}
0(1)
=
-exp
i
x-
v+-
-+
+--,
](X
2 2 2x x
5
/
2
H~2)(x)
= Jv(x) - iYv(x)
=
(2
exp
{-i
[x
_
(v
+
~)
::.
+ v
2
-
1/4]}
+
0(1).
Y
;;;;
2 2 2x x
5/
2
If the last term in the
exponent-which
vanishes as x
-+
oo-is
ignored, the
asymptotic expressionfor HPJ
(x)
matches what was obtainedin Chapter15using
the method of steepest descent.

522
18. STURM-LIOUVILLE
SYSTEMS:
FORMALISM
18.4 Problems
18.1. Show that the Liouville substitutiou trausfonns regular S-L systems into
regular S-L systems aud separated aud periodic BCs into separated aud periodic
BCs, respectively.
18.2. Let UI(x) aud U2(X) be trausfonned, respectively into VI(t) aud V2(t) by the
Liouville substitutiou. Show that the inner productou
[a, b] with weight functiou
w(x)
is trausfonned into the innerproduct ou [0, c] with unit weight, where c =
J:
../w/pdx.
18.3. Derive Equatiou (18.17) from (18.15) using Priifer substitution.
18.4. (a) Show thatthe Liouville substitution trausfonns the Bessel DE into
d
2
v
(2
v
2
-
1/4)
_
-2
+ k - 2
v-D.
dt t
(b) Findthe the equations obtained from the Priifer substitution, aud show that for
large
x these equations reduce to
, (
a)
0(1)
</>
= k I - 2k
2x
2
+
~
R'
0(1)
=
R
~'
where a = v
2
-
t.
(c) Integrate these equations from x to b > x aud take the limit as b --+
00
to get
a
0(1)
</>(x)
=
</>00
+kx+
2kx
+
7'
0(1)
R(x)
= Roo+
-2-'
x
where
</>00
=
limb-->oo(</>
(b) - kb) aud Roo =
limb-->oo
R(b).
(d) Substitute these aud the appropriate expression for
Q-I/4
in Equation (18.16)
aud show that
Roo (
v
2
-
1/4)
0(1)
vex) =
Ii:
cos
kx
- kxoo + +
-2-'
-Jk 2kx x
where kxoo ea n
/2
-
</>00'
(e) Choose Roo =
../2/:n:
for all solutions
of
the Bessel DE, aud let
aud
for the Besselfunctions
Jv(x) aud the Neumannfunctions Y
v
(x),
respectively, aud
find the asymptotic behavior
of
these two functions.
