Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


19.4
SEPARATION
IN SPHERICAL
COOROINATES
543
where
Zv(x)
is a solution of the Bessel eqnation
of
order v.
A general solution
of
(19.20) can therefore be written as
If
the originis included in the region of interest, then we must set B =
O.
For such
a case, the solution to the Helmholtz equation is
00
Z
'h(r,
IJ,
'P) = L L Azmjz(kr)Yzm(lJ, 'P).
I=Om=-l
(19.22)
forI = 0, 1,
...
, n = 1,2,
....
particle
Ina
hard
sphere
The subscript k indicates
that'"
is a solution of the Helmholtz equation with k
2
as
its constant.
19.4.1. Example. PARTICLE IN A HARD SPHERE
The time-independent Schrodinger equation for a particle in a sphere of radius a is
,,}
- 2jLv
2t
=
Et
with the
Be
t(o,
e,
IfJ)
=
O.
Here E is the energy of the particle
and
jL is its mass.We rewritethe Schrodinger equationas
V2t
+ 2jLE
jn
2
=
O.
With
k
2
=
2f.LE
/;,,2,we can
immediately
writethe
radial
solution
Rz(r) = Ajz(kr) =
Ajz(,j2jLErjn).
Thevanishing of
tat
0 impliesthat jZ(.,f2jLE
ojn)
= 0, or
.,f2jLE0
11,
- = Xin for n = 1,2,
"',
where
KIn is thenthzeroof
hex),
whichis thesameas thezeroof Jl+l/2(X).
Thus,
the
energy
is
quantized
as
El
n
=
n2x~
2jLo
The
general
solution to theSchrodlnger
equation
is
00
00
I
r)
t(r,e,IfJ)
=
LL
L Anzmjz(Xz
n
ti
YZm(e,'P).
n=11=Om=-1
III
A particularly useful consequence
of
Equation (19.22) is the expansion of a
plane wave in terms
of
spherical Bessel functions.
It
is easily verified that
if
k
is a vector, with k . k
= k
2
,
then e
ik
.
r
is a solution
of
the Helmholtz equation.
Thus,
e
ik
.
r
can be expanded as in Equation (19.22). Assuming that k is along the
z-axis, we get
k·
r =
krcoslJ,
which is independent of 'P. Only the terms of
Equation (19.22) for which
m = 0 will survive in such a case, and we may write
eik"osO =
L~o
A/j/(kr)Pz(cos
IJ).To find Az,
let
u =cos
IJ,
mnltiply both sides
by
Pn(u),andintegratefrom-I
to I:
1
1 .
00
11
2
Pn(u)e'krUdu = L
Azjz(kr)
Pn(u)Pz(u) du =
Anjn(kr)--.
-1
/=0
-1
2n +I
544 19. STURM-LIOUVILLE
SYSTEMS:
EXAMPLES
Thus
. 2n +
11
1
ikru
Anln(kr)
=
--
Pn(u)e
du
2
-1
= 2n +1 f (ikr)m 1
1
Pn(u)umdu.
2 m=O m!
-1
(19.23)
expansion
ofe
ik
.
r
in
spherical
harmonics
This equality holds for all values
of
kr.
In particular, both sides should give the
same resultin the limit
of
small
kr.
Fromthe definition
of
jn
(kr)
and the expansion
of
I
n
(kr),
we obtain
.Jii
(kr)n
1
jn(kr)
~
2 2
['(n
+
3/2)
On the other hand, the first nonvanishing term
of
the RHS
of
Equation (19.23)
occurs when
m = n. Eqnating these terms on both sides, we get
.Jii
(kr)n
2
1..+1
n!
= 2n +1 ;n(kr)n 2
n+1(nl)2
An
2 2 (2n +
1)!.Jii
2 n! (2n +I)! '
wherewe haveused
(19.24)
(
~)
_ (2n +1)1.Jii
I'
n + 2 - 21..+1n!
and
1
1 n 2
n
+
1
(nl)2
-1
Pn(U)U
du = (2n +1)1'
Equation (19.24) yields An = ;n(2n +1).
With An thns calculated, we
can
now write
00
elkroo'B =
L(21
+
l);l
jl
(kr) PI(cos
6).
I~O
(19.25)
For an arbitrary direction
of
k, k . r =
kr
cos y, where y is the angle between k
and
r.
Thus, we may write elk.• =
I:Z:;o(21
+1);1jl (kr)PI(cos V), and using the
addition theorem for spherical harmonics, we finally obtain
00
1
elk-r = 4n L L ;1h(kr)Y/;n (6', ,P')Ylm(6,
<p),
1=0
m=-l
(19.26)
where
6'
and ip' are the spherical angles
of
k and 6 and
<p
are those
of
r. Such
a decomposition
of
plane waves into components with definite orbital angular
momenta is extremely useful
when
working with scattering theory for waves and
particles.
19.5
PROBLEMS
545
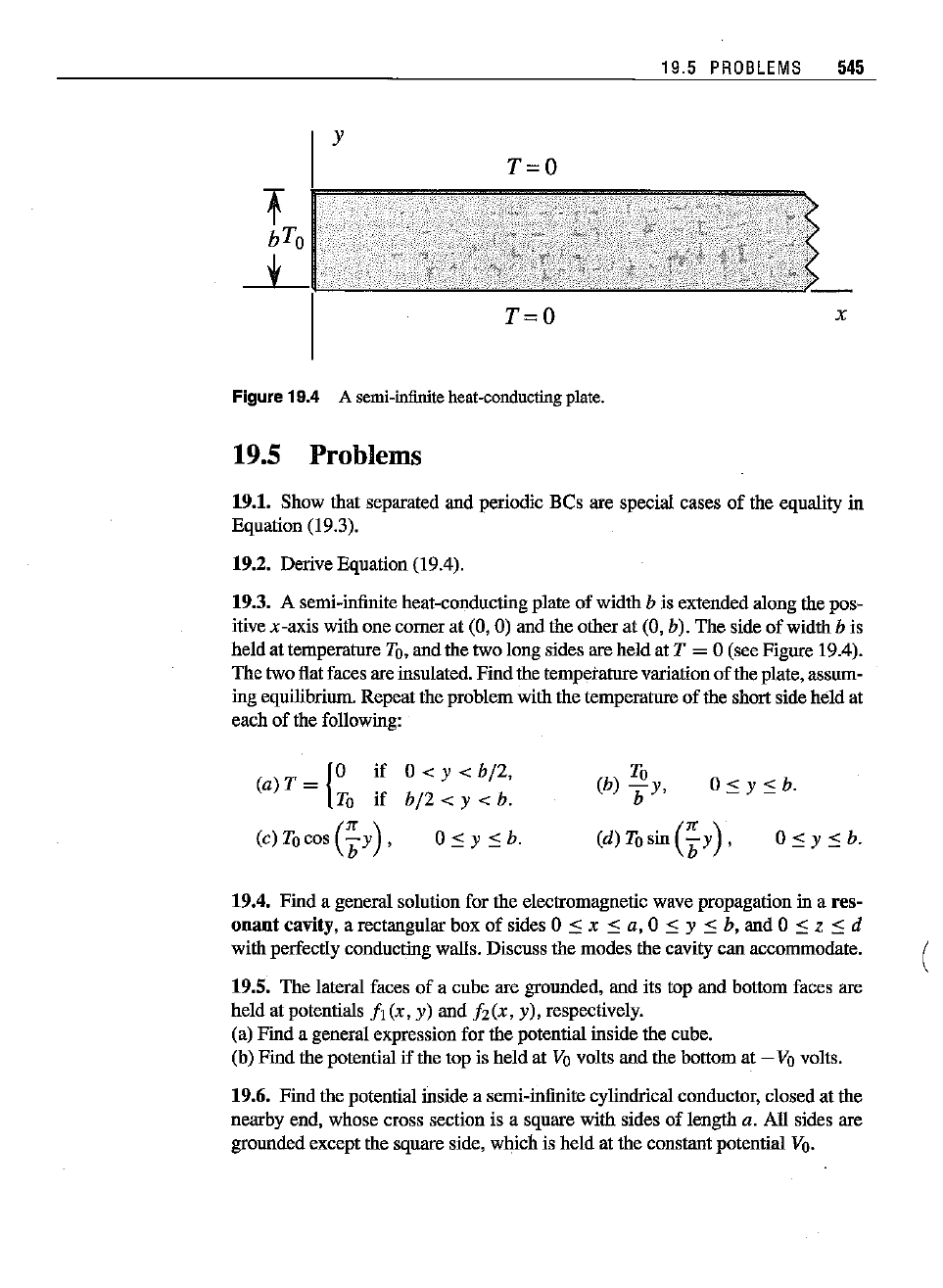
T=O
T=O
Figure 19.4 A semi-infiniteheat-conductingplate.
19.5 Problems
x
19.1. Show that separated and periodic
Bes
are special cases
of
the equality in
Equation (19.3).
19.2. Derive Equation (19.4).
19.3. A semi-infinite heat-conducting plate of width b is extended along the pos-
itive x-axis with one comer at (0, 0) and the other at (0, b). The side of width b is
held at temperature
To,and the two long sides are held at T = 0 (see Figure 19.4).
The two flat faces are insulated. Find the temperature variation
of
the plate, assum-
ing equilibrium. Repeat the problem with the temperature of the short side held at
each of the following:
Ca)T={O
~f
To If
(c) Tocos
Gy)
,
0<
y <
biZ,
biZ
< y < b.
o
:'0
y
:'0
b.
(b)
;y,
0:'0
y:'O
b.
(d) Tosin
(~y)
, 0:'0 y
:'0
b.
19.4. Find a general solution for the electromagnetic wave propagation in a res-
onant
cavity, a rectangular box
of
sides 0
:'0
x
:'0
a, 0
:'0
Y
:'0
b, and 0
:'0
z
:'0
d
with perfectly conducting walls. Discuss the modes the cavity can accommodate. (
19.5. The lateral faces
of
a cube are grounded, and its top and bottom faces are
held at potentials
11
(x, y) and
fz(x,
y), respectively.
(a) Find a general expression for the potential inside the cube.
(b) Find the potential
if
the top is held at
Vo
volts and the bottom at -
Vo
volts.
19.6. Find the potential inside a semi-infinite cylindrical conductor, closed at the
nearby end, whose cross section is a square with sides
oflength
a. All sides are
grounded except the square side, which is held at the constant potential
Vo.

546 19. STURM-LIOUVILLE
SYSTEMS:
EXAMPLES
19.7. Find the temperature distribution
of
a rectangular plate (see Figure 19.2)
with sides
of
lengths a and b
if
three sides are held at T = 0
and
the fourth side
has a temperature variation given by
0::;
x < a.
To
(a)
-x,
a
To
I a I
(e)
-;
x -"2 '
o
:::
x < a.
To
(b)
2"x(x
- a),
0:"
x
:"
a.
a
(d)
T = 0, 0 :" x :" a.
19.8. Consider a thin heat-conducting
bar
of
length b along the x-axis with one
end
at x = 0 heldat temperature
To
and
the other
end
at x = b heldattemperature
-
To.
The lateral surface
of
the
bar
is thermally insulated.
Find
the temperature
distribution at all times if initially it is given by
2To
(a) T(O,x) =
-bx
+
To,
where
0:"
x:"
b.
2
T
o 2
(b) T(O,x) =
-z;zx
+To, where
0:"
x :" b.
To
(e) T(O,x) =
-,;x
+
To,
where
0:"
x < b.
(d)
T(O,x)
=
Tocosej;x),
where
O:"x:"b.
Hint: The solution corresponding to the zero eigenvalue is essential and cannotbe
excluded.
19.9. Determine
T(x,
y, r) for the rectangularplate
of
Example 19.2.3
if
initially
the lowerleft quarter is held at
To
and
the rest
of
the plate is held at T =
O.
19.10. All sides
of
the plate
of
Example 19.2.3 are held at T = O.
Find
the
temperature distribution for all time if the initial temperature distribution is given
by
if
"a
< x <
~a
and
Ib
< y <
~b
4--44--4'
otherwise.
{
To
(a)
T(x,
y, 0) = 0
To
(b)
T(x,
y, 0) =
-xy,
ab
To
(e)
T(x,
y, 0) =
-x,
a
where
0:"
x < a and 0 :" y < b.
where
0:"
x < a and 0 < y < b.
(
19.11. Repeat Example 19.2.3 with the temperatures
of
the sides equal to Tl,
T2,
T3,
and
T4.Hint: You
must
include solutions corresponding to the zero eigenvalue.
19.12. A string
of
length a is fixed at the
left
end, and the right
end
moves with
displacement A
sinmt.
Find
1{f(x,t) and a consistent set
of
initial conditions for
the displacement and the velocity.

19.5
PROBLEMS
547
19.13. Find the equation for a vibrating rectangular membrane with sides of
lengths a and b rigidly fastened on
all sides.
For
a = b, show that a given mode
frequency
mayhavemore
than
onesolution.
19.14. Repeat Example 19.3.1
if
the can has semi-infinite length, the lateral sur-
face is grounded, and:
(a) the base is held at the potential
V(p,
<fI).
Specialize to the case where the potential of the base is
given-in
Cartesian co-
ordinates-s-oy
Vo
(b) V =
-yo
a
Vo
(c) V =
-x.
a
Vo
(d) V =
'2xy.
a
Hint: Use the integral identity
f zV+IJv(z)
dz
=
Zv+1
Jv+1
(z).
19.15.
Findthe steady-state temperature distribution T
(p,
tp, z) in a semi-infinite
solid cylinder
of
radius a if the temperature distribution
of
the base is
f(p,
<fI)
and
the lateral surface is held at
T =
O.
19.16. Find the steady-state temperature distribution of a solid cylinder with a
height and radius of 10, assuutingthat the baseand the lateral surface are at
T = 0
and the top is at T
= 100.
19.17. The circumference
of
a flat circularplate
of
radius a, lyingin the xy-plane,
is held at T =
O.
Find the temperature distribution for all time if the temperature
distribution
att = 0 is
given-in
Cartesian
coordinates-by
To
(a)
-yo
a
To
(b)
-x.
a
To
(c)
'2xy.
a
(d)
To.
19.18. Findthe temperature
of
a circularconducting plate
of
radius a at allpoints
of its surface for
alltime t > 0, assuming that its edge is held at T = 0 and initially
its surface from the ceuter to a
/2
is in contact with a heat bath
of
temperature
To.
19.19. Fiud the potential of a cylindrical conducting can of radius a and height h
whose top is held at a constant potential
Vo
while the rest is grounded.
19.20. Findthe modes and the correspondingfields of acylindricalresonantcavity
of length
L and radius a. Discuss the lowest TM mode.
19.21. Two identical long conducting half-cylindrical shells (cross sectious are
half-circles)
of
radius a are gluedtogetherin such away that they are insulatedfrom
one another. One half-cylinder is held at potential
Vo
and the other is grouuded.
Findthe potentialat anypointinsidetheresultingcylinder. Hint: SeparateLaplace's
equation
intwo dimensions.
(

548
19. STURM-LIOUVILLE
SYSTEMS:
EXAMPLES
19.22. A linear charge distribution of uniform dcnsity x extends along the z-axis
from z
=
-b
to z = b. Show that the electrostatic potential at any point r > b is
given by
00
(b/r)Zk+l
<P(r,
e, rp) = 2)"
I:
PZk(COSe).
k~O
2k+
I
Hiut: Considera point on the z-axis at a distance r > b from the origin. Solve the
simple problem by integration and compare the result with the infinite series to
obtain the unknown coefficients.
19.23. The upper
half
of a heat-conducting sphere
of
radius a has T = 100;the .
lower
half
is maintainedat T =
-100.
The wholesphereis insidean infinitelylarge
mass of heat-conducting material. Find the steady-state temperature distribution
inside and outside the sphere.
19.24. Find the steady-state temperature distribution inside a sphere of radius a
when the surface temperature is given by:
(a)
To
cos
z
e.
(d)
To
(cos e- cos
3
e).
(b)
To
cos"
e.
(e) To
sm2
e.
(c) Tolcos e].
(f)
To
sin
4
e.
19.25. Findthe electrostatic potentialbothinside and outside a conducting sphere
of radius
a when the sphere is maintained at a potential given by
(a) Vo(cose -
3sin
z
e).
{
Vocos e for the upper hemisphere,
(c) .
o for the lower hemisphere.
19.26. Find the steady-state temperature distribution inside a solid hemisphere
of radius
a
if
the curved surface is held at To and the flat surface at T =
O.
Hint: Imagine completing the sphere and maintaining the lower hemisphere at a
temperature such that the overall surface temperaturedistributionis an
odd
function
about
e= n
/2.
19.27. Find the steady-state temperature distribution in a spherical shell
of
inner (
radius
R1 and outerradius Rz when the innersurface has a temperature Tl and the
outer surface a temperature
Tz-
Additional Reading
1. Jackson, J. Classical Electrodynamics, 2nd ed., Wiley, 1975. The classic
textbook on electromagnetism with many examples and problems on the
solutions of Laplace's equation in different coordinate systems.

19.5
PROBLEMS
549
2. Mathews, J. and Walker, R. Mathematical Methods
of
Physics, 2nd ed.,
Benjamin, 1970.
3. Morse, P. and Feshbach, M. Methods
of
Theoretical Physics, McGraw-Hill,
1953.
(
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
( 1
1
1
1
1
1
1
1
1

Part VI _
Green's Functions
(
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
1
( 1
1
1
1
1
1
1
1
1
1
1
