Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


16.7
SPECTRAL
THEOREM
FOR
COMPACT
OPERATORS
473
o
Figure 16.1 The
shaded
area
represents
a convexsubsetof the
vector
space.
It
consists
of
vectors
whosetips lie in the
shaded
region.
It
is
clear
that
there
is a
(unique)
vector
belongingtothe subsetwhose lengthis
minimum.
Proof
(I)
was proved in Proposition 16.6.1. (2) was shown in the lemma above.
(3) Let
<T,,(K)
sa
{A
E
<T(K)
IIAI
~
lin}.
Clearly,
<Tn(K)
must be a finite set,
becauseotherwisethe infinite set wouldconstitute a sequencethat by compactuess
of
<T"(K) would have to have (at least) a limitpoint. By part (2), this limit must be
zero, which is not included in
<Tn
(K). Let
<T1
(K) =
{Ai}7~1,arranged
in order of
decreasing absolute value. Next, let
Ak+l'
Ak+2,
...
label the elements of
<T2(K)
not accounted for in
<T1
(K), again arranged in decreasing absolute value.
If
this
process stops after a finite number
of
steps,
<T
(K) is finite. Otherwise, continue the
process to construct a sequence whose limit by necessity is zero. D
16.7 Spectral Theorem for Compact Operators
The finite-dimensional spectral decompositiontheorem of Chapter4 was basedon
the existence of eigenvalues, eigenspaces, and projectionoperators. Such existence
was guaranteedby the existence of an innerproductfor any finite-dimensional vec-
tor space. The task of establishing spectral decompositionfor infinite-dimensional
vector spaces is complicated not only by the possibility of the absence
of
an inner
product, but also by the questions
of
completeness, closure, and convergence. One
can elintinate the first two hindrances by restticting oneself to a Hilbert space.
However,
evenso,onehastodealwith
other
complications of
infinite
dimensions.
As an example, consider the relation V = W 6) W.L,which is ttivially true for
any subspace W in finite dimensions once an orthonormal basis is chosen. Recall
that the procedure for establishing this relation is to complement a basis of W
to produce a basis for the whole space.
In an infinite-dimensional Hilbert space,
we do not know a priori how to complement the basis of a subspace (which may
be infinite-dimensional). Thus, one has to prove the existence
of
the orthogonal
complement of a subspace. Without going into details, we sketch the proof. First
a definition:

474 16. AN
INTRODUCTION
TO
OPERATOR
THEORY
convex
subset 16.7.1. Definition. A convexsubset E
of
a vector space is a collection
of
vectors
such that
iflu)
and Iv)are in E, then lu)
-t(lu)
-Iv»
is also in
Efor
all 0
:0:
t
:0:
1.
Intuitively, any two points
of
a convex subset
can
be connected by a straigbt line
segmentlying entirely in
the subset.
Let
E be a convex subset (nota subspace)
of
a Hilbert space Ji:.
One
can show
that there exists a unique vector in
E with
minimal
nonn
(see Figure 16.1). Now
let
M be a subspace
of
Ji:.
For
an arbitrary vector lu) in Ji:, consider the subset
E = lu) - M, i.e., all vectors
of
the
form
lu) - 1m) with 1m) E M. Denote
the unique vector
of
minimal
nonn
of
lu) - M by lu) - IPu) with IPu)
EM.
One
can
show that lu) - IPu) is orthogonal to lu), i.e., (Iu) - IPu» E M.L (see
Figure 16.2). Obviously,
only
the
zero vector
can
be simultaneouslyin M and M.L.
Furthermore, any vector lu) in Ji:
can
be written as lu) = IPu) +(Iu)
-IPu»
with
IPu)
EM
and
(Iu)
-IPu»
E M.L. This shows that Ji: = M
Ell
M.L. In words,
a Hilbert space is
the direct Sum
of
anyone
of
its subspaces
and
the orthogonal
complement
of
that subspace. The vector I
Pu)
so constructed is the projection
of
lu)
inM.
A projection operator P
can
be defined as a linear operator with
the
property
that p
2
= P.
One
can
then
show
the
following.
16.7.2.
Theorem.
The kernel
ker
P
of
a projection operator is the orthogonal
complement
of
the range P(Ji:)
of
P in Ji: iffP is hermitian.
This
is the reason for demanding
henniticity
of
the projection operators in our
treatruent
of
the
finite-dimensional case.
We
now
concentrate
on
the compact operators, and first
look
at hermitian
compact operators. We
need
two lemmas:
16.7.3.
Lemma.
Let H E :B(Ji:) be a bounded hermitian operator on the Hilbert
space
K Then
[H]
=maxl] (H»] x) II1Ixll =
II.
Proof
Let
M denote
the
positive
number
on
the
RHS.
From
the
definition
of
the
norm
of
an operator, we easilyobtain I
(Hxlx)
1:0:
lIHlllIx1l
2
= lIHlI,orM:o:
lIHII.
For
the reverse inequality, see Problem 16.7. D
16.7.4.
Lemma.
Let K E :B(Ji:) be a hermitian compact operator. Then there is
an eigenvalue}"
ofKsuch
that
IAI
=
lIKll.
Proof
Let
(Ix
n)
I be a sequence
of
unit vectors such
lbat
Thisis always possible, as the following argument shows.
Let
E
be
a smallpositive
number. There
must
exist a unit vector IXI) E Ji: such that

16.7
SPECTRAL
THEOREM
FOR
COMPACT
OPERATORS
475
Figure 16.2 The
shaded
area
represents
the
subspace
Jy(of thevector
space.
Theconvex
subset
E
consists
ofall
vectors
connecting
points
ofM tothetipof lu}. It isclear
that
there
is a
(unique)
vectorbelonging to E whose lengthis
minimum.
The
figure
shows
that
this
vectoris orthogonal to M,
because otherwise,
IIKII
- E would be greater thau or equal to the
uonn
of
the
operator (see
Lemma
16.7.3). Similarly, there
must
exist auother (differeut) unit
vector
IX2}
E
1f
such that
II
KII
- E
12
= I
(Kx21
X2)
I. Continuing this way, we
constructau infinite sequence
of
unitvectors (Ix
n))
with the property
IIKII-Eln
=
I(Kx
n
Ix
n)
I·This coustructionclearlyproduces the desired sequence. Notethat the
argumeutholds for
anyhennitiauboundedoperator; compactness is
uot
uecessary.
Now define
an sa (Kxnl x
n
)
aud
leta
= lim an, so that lal =
IIKII.
Compact-
ness
of
Kimplies that(IKx
n))
converges.
Let
Iy} E
1fbe
the limit
of
{IKx
n}}.
Then
lIyll
= lim
IIKx
nll
~
IIKllllx
nll
=
IIKII.
On the
otherhaud,
o
~
IIKx
n
-
axnll =
IIKx
nll
2
-
2a (Kxnl x
n)
+lal
2.
Taking the limit aud noting that
an aud a are real, we get
It
follows from these two inequalities that
lIyll
=
IIKII
aud that lim Ix
n
}
= Iy} [tx,
Furthermore,
(K-
al)(ly}
la)
=(K -
al)(lim
Ix
n})
=lim(K -
al)
Ix
n}
=0
Therefore,
a is au eigeuvalue
of
Kwith eigenvector Iy} Ia. o
Let us orderall the eigeuvalues
of
Theorem16.6.9 in decreasing absolutevalue.
Let
M
n
denote the (finite-dimensional) eigeuspace corresponding to eigenvalue
An, aud P
n
the projection to M
n.
The
eigenspaces are pairwise orthogonal aud
PnP
m
= 0 for m
i'
n. This follows in exact aualogy with the finite-dimensional
case.
First assume that K has only finitely mauy eigenvalues,

476 16. AN INTRODUCTION
TO
OPERATOR
THEORY
Let M ""
Ml
Ell
Mz
Ell
...
Ell
M,. =
I:'i=l
EIlMj, and let Mo be the orthogonal
complement
of
M. Sinceeacheigenspaceis
invariant
under
K,
sois
JvC,
Therefore,
by Theorem
4.2.3-which
holds for finite- as well as infinite-dimensional vector
spaces-and
the fact that Kis henmitian, Mo is also invariant. Let
Ko
be the restric-
tion of Kto Mo. By Lemma
16.7.4,
Ko
has an eigenvalne Asnch that
IAI
=
IIKolI.·
If
A i" 0, it mnst be one of the eigenvalnes already accounted for, because any
eigenvalue of
Ko
is also an eigenvalue
of
K. This is impossible, because Mo is
orthogonal to all the eigenspaces. So, A
= 0, or
IAI
=
IIKoll
= 0, or
Ko
= 0, i.e.,
Kacts as the zero operator on
Mo.
Let Po be the orthogonal projection on Mo. Then
JC
=
I:'i=o
EIlM
i- and we
have 1
=
I:'i=o
Pi- and for an arbitrary [x) E
JC,
we have
spectral
theorem
for
compact
hermitian
operators
K[x) = K
(~Pj
IX)) =
~K(Pj
Ix)) =
tAj(Pj
Ix)).
It
follows that K =
I:'i=l
AjPj.
Notice that the range
ofKis
I:'i=l
EIlMj,which is
finite-dimensional. Thus, Khas finite rank. Barring some technical details, which
we shall notreproducehere, the case
of
a compacthenmitianoperatorwith infinitely
many eigenvalues goes through in the sanneway (see [DeVi
90, pp. 179-180]):
16.7.5.
Theorem.
(spectral theorem: compact henmitian operators) Let K be a
compact hermitian operator on a Hilbert space
JC.
Let
{A
j IN=l be the distinct
nonzero eigenvalues
of
K arranged in decreasing order
of
ahsolute values. For
each j let
Mj
be the eigenspace
ofK
corresponding to eigenvalue Aj and Pj its
projection operator with the property
PiPj = 0 for i i"
j.
Then:
1.
If
N <
00,
then K is an operator
of
finite rank, K =
I:
J
= l
AjPj,
and
JC
= Mo
Ell
Ml
Ell···
Ell
MN, or 1 =
I:J=oPj,
where Mo is infinite-
dimensional.
2.
If
N =
00,
then Aj
-+
0 as j
-+
00,
K =
I:i=l
AjPj,
and
JC
=
Mo
Ell
I:i=l
EIlM
l» or 1 =
I:i=o
P[, where
Mo
could be finite- or infinite-
dimensional.
Furthermore,
which shows that the infinite series above convergesfor an operator norm.
The eigenspaces of a compact hermitian operator are orthogonal and, by (2)
of Theorem
16.7.5,span the entire space. By the Grann-Schrnidt process, one can
select an orthonormal basis for each eigenspace. We therefore have the following
corollary.
16.7.6. Corollary.
If
K is a compact hermitian operator on a Hilbert space
JC,
then the eigenvectors
ofK
constitute an orthonormal basis for
JC.

spectral
theorem
for
compact
normal
operators
16.7
SPECTRAL
THEOREM
FOR
COMPACT
OPERATORS
477
16.7.7.
Theorem.
Let
Kbe a compact hermitian operator on a Hilbert space
Jf
and let K =
'£7~1
AjPj,
where N couldbe infinite. A boundedlinear operator on
Jf
commutes with Kif
and
only ifit commutes with every Pi-
Proof The
"if"
part is straightforward. So assume that the bounded operator T
commutes with K. For
[x) E
Mj,wehave(K-Aj)Tlx)
=
T(K-Aj)
Ix)
=0.
Similarly, (K- Aj)Tt [x) = Tt(K - Aj) Ix) = 0, because 0 = [T, K]t =
rr'.
K].
These equatious show that both T aud
rt
leave Mj invariaut. This meaus thatMj
reduces T, aud by Theorem 4.2.5, TPj = PjT. 0
Next we prove the spectral theorem for a uormal operator. Recall that auy
operatorTcaubewrittenasT=T,+iTiwhereT,
=
!(T+Tt)audTi
=
lICT-Tt)
are henntitiau, aud since both T aud
rt
are compact, T, aud Ti are compact as well.
For normal operators, we have the extra condition that
[T"
Til = [T,Tt] =
O.
Let
T, =
'£7~1
AjPj
aud r, =
,£f=ll-'k
Qk
be the spectral decompositions err, aud
Ti. Using Theorem 16.7.7, it is strailfhtforward to show that
if
[T"
Til = 0 then
[Pj,Qk]
=
O.Now,sinceJf
=
'£j~oEllMj
=
'£~oEll:Nk.whereMj
are the
eigenspaces
ofT,
aud:Nk those
ofTi,
we have, for auy Ix) E
Jf,
T, [x) =
(~AjPj)
(~Qk
IX)) =
~~AjPjQk
Ix).
Sintilarly,
r, [x) =
Ti('£7~oPj
Ix) =
'£f=1
,£7=0I-'kQkPj [x), Combining
these two relations aud noting that
QkPj = Pj Qk gives
N N
T [x) = (T, +
iTi)
[x) = L
L(Aj
+il-'k)PjQk
[z}.
j~Ok~O
The projection operators Pj Qk project onto the intersection of Mj aud :Nk.There-
fore, Mj
n :Nk are the eigenspaces of T. Only those terms in the sum for which
Mj
n:Nk
f=
0 contribute. As before, we cau order the eigenvalues according to
their absolute values.
16.7.8.
Theorem.
(spectral theorem: compact normal operators)
LetT
be a com-
pact normal operator on a Hilbert space
Jf.
Let
{A
j
17=1
(where N can be 00)
be the distinct nonzero eigenvalues
ofT
arranged in decreasing order
of
absolute
values. For each n let M
n
be the eigenspace
ofT
corresponding to eigenvalue An
and P
n
its projection operator with the property PmP
n
=
Of
or m
f=
n. Then:
1.
If
N <
00,
then T is an operator
of
finite rank T =
'£~~1
Aj Pi-
and
Jf
= Mo
Ell
Ml
Ell···
Ell
MN,
or 1 = ,£7=0
Pj,
where Mo is infinite-
dimensional.
2.
If
N =
00,
then An --> 0 as n -->
00,
T =
'£~1
AnP
no
and
Jf
=
Mo
Ell
'£~1
EIlM
n,
or 1 =
'£1=0
Pj,
where Mo could be finite- or infinite-
dimensional.

478 16.
AN
INTRODUCTION
TO
OPERATOR
THEORY
As in the case
of
a compacthermitianoperator, by the Gram-Schmidtprocess,
one can select an orthonormal basis for each eigenspace
of
a normal operator, in
which case we have the following:
16.7.9. Corollary.
lfT
is a compact normal operator on a Hilbert space
Jf,
then
the eigenvectors
ofT
constitute an orthonormal basis for Jf.
One can use Theorem 16.7.8 to write any function
of
a normal operator T as
an expansion in terms
of
the projection operators
of
T. First we note that T
k
has
A~
as its expansion coefficients. Next, we add various powers
of
T in the form
of
a polynomial and conclude that the expansion coefficients for a polynomial p(T)
are pO.n). Finally, for any function
f(T)
we have
00
f(T)
=
Lf(An)P
n.
n=l
(16.8)
Johann
(John)von Neumann,(1903-1957), theeldest ofthree
sons of Max von Neumann, a well-to-do Jewish banker, was
privatelyeducateduntilhe entered the gymnasium in 1914. His
unusual mathematical abilities soon came to the attention of
his teachers, who pointed out to his father that teaching him
conventional school mathematics would be a waste
of
time;
he was therefore tutored in mathematics under the guidance of
university professors, and by the age
of
nineteenhe was already
recognized as a professional mathematician and had published
his first paper.
VonNeumannwas Privatdozentat Berlinfrom 1927 to 1929
and at Hamburg in 1929-1930, then went to Princeton.University
forthree
years; in 1933
he was invited to
join
the newly opened Institute for Advanced Study, of which he was the
youngest permanent member at that time. At the outbreak of World War Il, von Neumann
was called upon to participate in various scientific projects related to the war effort:
In
particular, from 1943 he was a consultant on the construction
of
the
atomic bomb at Los
Alamos. After the war he retained his membership on numerous government boards and
committees, and in 1954 he became a member
of
the Atomic Energy Commission. His
health began to fail in 1955, and he died of cancer two years later.
It
is only in comparison with the greatest mathematical geniuses of history that von
Neumann's scope in pure mathematics may appear somewhatrestricted;
it
was far beyond
the range
of
most
of
his contemporaries, and his extraordinary work in appliedmathematics,
in which he certainly equals Gauss, Cauchy, or Poincare, more than compensates for its
limitations. Von Neumann's work in pure mathematics was accomplished between 1925
and 1940, when he seemed to be advancing at a breathless speed on all fronts of logic
and analysis at once, not to speak
of
mathematical physics. The dominant theme in von
Neumann's work is by far his work on the
spectraltheoryofoperatorsin Hilbert spaces.
For twenty years he was the undisputed master in this area, which contains what is now
considered his most profound and most original creation, the theory
of
rings of operators.

16.7
SPECTRAL
THEOREM
FOR
COMPACT
OPERATORS
479
The
first papers (1927) in
which
Hilbert space
theory
appears are
those
on the foundations
of
quantum mechanics.
These
investigations
later
led
von
Neumann
to a systematic study
of
unbounded
hermitian operators.
Von
Neumann's
most
famous
work
in theoretical
physics
is his axiomatization
of
quan-
tum mechanics.
When
he
began
work
in
that
field in 1927,' the
methods
used
by its founders
were hard to formulate in precise mathematical tenus: "Operators" on "functions" were
handled
without
much
consideration
of
their
domain
of
definition or
their
topological
prop-
erties,
and
it was blithely
assumed
that
such
"operators,"
when
self-adjoint,
could
always
be "diagonalized" (as in the finite dimensional case), at the expense of introducing Dirac
delta functions as "eigenvectors." Von
Neumann
showed
that
mathematical
rigor
could be
restored by taking as basic axioms the assumptions
that
the states
of
a physical system
werepoints
of
a Hilbertspace and
that
the
measurable
quantities
were
Hermitian (generally
unbounded) operators densely defined in
that
space.
After
1927
von
Neumann
also devoted
much
effort
to
more
specific problems
of
quan-
tum mechanics,
such
as the
problem
of
measurement
and
the foundation of
quantum
statis-
tics and
quantum
thermodynamics, proving in particular an ergodic
theorem
for quantum
systems.
All
this work was developed and
expanded
in Mathematische Grundlagen del"
Quantenmechanik
(1932),in whichhe
also
discussedthe
much-debated
question
of
"causal-
ity" versus "indeterminacy" and concluded
that
no introduction
of
"hidden
parameters"
could
keep the basic structure
of
quantum
theory
and
restore "causality."
Von
Neumann's
uncommon
grasp
of
applied mathematics, treated as a whole without
divorcing
theory
from experimental realization, was nowhere
more
apparent than in his
work on computers. He
became
interested in numerical computations in connection
with
the
need
for
quick
estimates and approximate results that developed
with
the technology
used for the
war
effort-particularly
the
complex
problems
of
hydrodynamics-and
the
completely
new
problems presented by the harnessing
of
nuclear
energy,
for
which no
ready-made theoretical solutions
were
available. Von
Neumann's
extraordinary ability
for
rapid
mental calculation was legendary.
The
story is
told
of
a friend
who
brought
him
a
simple kinematics problem. Two trains, a certain given distance apart,
move
toward
each
other
at a given speed. A fly, initially on the windshield
of
one
of
the trains, flies back
and
forth
between
them, again at a
known
constant speed.
When
the trains collide, how far has
the fly traveled? One way to solve the
problem
is to
add
up all the successively smaller
distances in
each
individual flight.
(The
easy
way
is to multiply the
Ily'sspeed
by the
time
elapsed until the crash.)
After
a few seconds
of
thought, von
Neumann
quickly gave the
correct answer.
"That's
strange," remarked his friend,
"Most
people try to
sum
the infinite series."
"What's
strange
about
that?"
von
Neumann
replied.
"That's
what
I did."
In closing this section, let ns remark that the paradigm of compact operators,
namely the Hilbert-Schmidt operator, is such because it is defined on the
finite
rectangle [a, b) x [a, b).
If
this rectangle grows beyond limit, or equivalently,
if
the Hilbert space is
r:..
2
(R
oo
), where Roo is some infinite region
of
the real line,
then the compactness property breaks down, as the following example illustrates.
16.7.10. Example.
Consider
thetwo
kernels
Kj(x.
t) =
e-
I
x-
II
and K2(X, t) =
sinxt
480 16. AN
INTROOUCTION
TO
OPERATOR
THEORY
wherethe firstone actson
,(,2(_00,
00) andthe second oneon ,(,2(0, 00). Onecan show
(see Problem 16.8) thatthese two kernels have, respectively, the two eigenfunctions
e
iat•
a E
JR.,
and
a
>0,
corresponding
to thetwoeigenvalues
2
),,=--2'
o<EIR,
1+0<
and
resolvent
ofan
operator
Wesee
that
in the
first
case,all real
numbers
between0 and2 areeigenvalues,
rendering
this set
uncountable.
In the secondcase,
there
areinfinitely (in fact,
uncountably)
many
eigenvectors.
(oneforeacha)
corresponding
tothesingleeigenvalue
JJr
/2. Note,
however,
that
in the
first
case the eigenfunctions andin the second case the
kernel
have
infinite
norms.
II
16.8 Resolvents
The discussion
of
the preceding section showed that the spectrum
of
a normal
compactoperatoriscountable. Removing the compactness property in general will
removecountability, as
showninExample
16.7.10.Wehave also seen that the right-
shiftoperator, aboundedoperator,has uncountablymany pointsin its spectrum. We
therefore expect that the sums in Theorem 16.7.8 should be replaced by integrals
in the spectral decomposition theorem for (noncompact) bounded operators. We
shall not discuss the spectral theorem for general operators. However, one special
class
of
noncompact operators is essential for the treatroent
of
Stnrrn-Liouville
theory (to be stndied in Chapters 18 and 19).
For
these operators, the concept
of
resolventwill be used, whichwe develop in this section. This conceptalso makesa
connectionbetween
the
countable(algebraic) and the uncountable (analytic) cases.
16.8.1. Definition. Let T be an operator
and
A E P(T). The operator Ri.(T) ea
(T - A1)-1 is called the resolvent
ofT
at A.
Thereare two importantproperties
of
the resolventthat are useful in analyzing
the .spectrum
of
operators. Let us assume that A,fJ, E P(T), A rf
fJ"
and take
the difference between their resolvents. Problem 16.9 shows how to obtain the
following relation:
(16.9)
To obtain the second property
of
the resolvent, we formally (and indefinitely)
differentiate Ri.
(T) with respect to Aand evaluate the result at A= W

16.8
RESOLVENTS
481
Differentiating both sides
of
this equation. we get
2Ri
(T), and in general.
d
n
d
n
-R,(T)
=
n!R~+l(T)
=}
-R,(T)
I =
n!R~+l(T).
d)..n d)..n
,~"
Assuming that the Taylor series expansion exists,
we
may write
(16.10)
(16.11)
forn
=0.1
•...
which is the second property
of
the resolvent.
We now
look
into the spectral decomposition from an analytical viewpoint.
For convenience, we concentrate on the finite-dimensional case and let A be an
arbitrary (not necessarilyhermitian) N x N matrix.
Let
Abe acomplexnumberthat
is larger (in absolute value) than any
of
the eigenvalues
of
A. Since all operators
on finite-dimensional vector spaces are compact, Lemma 16.7.4 assures us that
1)..1
>
II
T
II.
and it is then possible to expand R, (T) in a
convergent
power series as
follows:
1
00
A n
R,(A) =
(A_)..l)-l
=
--
L:(-)
)..
n~O
)..
This is the Laurent expansion
of
R, (A). We can immediately read off the residue
of
R,(A) (the coefficient
of
1/)..):
Res[R,(A)] =
-1
=}
----.!...,
1 R,(A)d)" = 1.
2m
fr.
where
I'
is a circle with its center at the origin and a radius large enongh to
encompass all the eigenvalues
of
A[see Figure l6.3(a)]. A similar argument shows
that
----.!...,
1 )..R,(A)d)" = A.
21n
fr.
and in general.
1
inn
--.
)..R,(A)d)..=A
21l"1 r
Using this and assuming that we can expand the function
f(A)
in a power series.
we get
-
~
1
f()..)R,(A)d)"
=
f(A).
2m
fr.
Writing this equation in the form
_1_1
f()..) d)..=
f(A)
2rri
fr.
)"l-A
(16.12)
482
16. AN
INTRODUCTION
TO
OPERATOR
THEORY
•
•
0
0
•
0
0
•
0
•
•
0
(a)
(b)
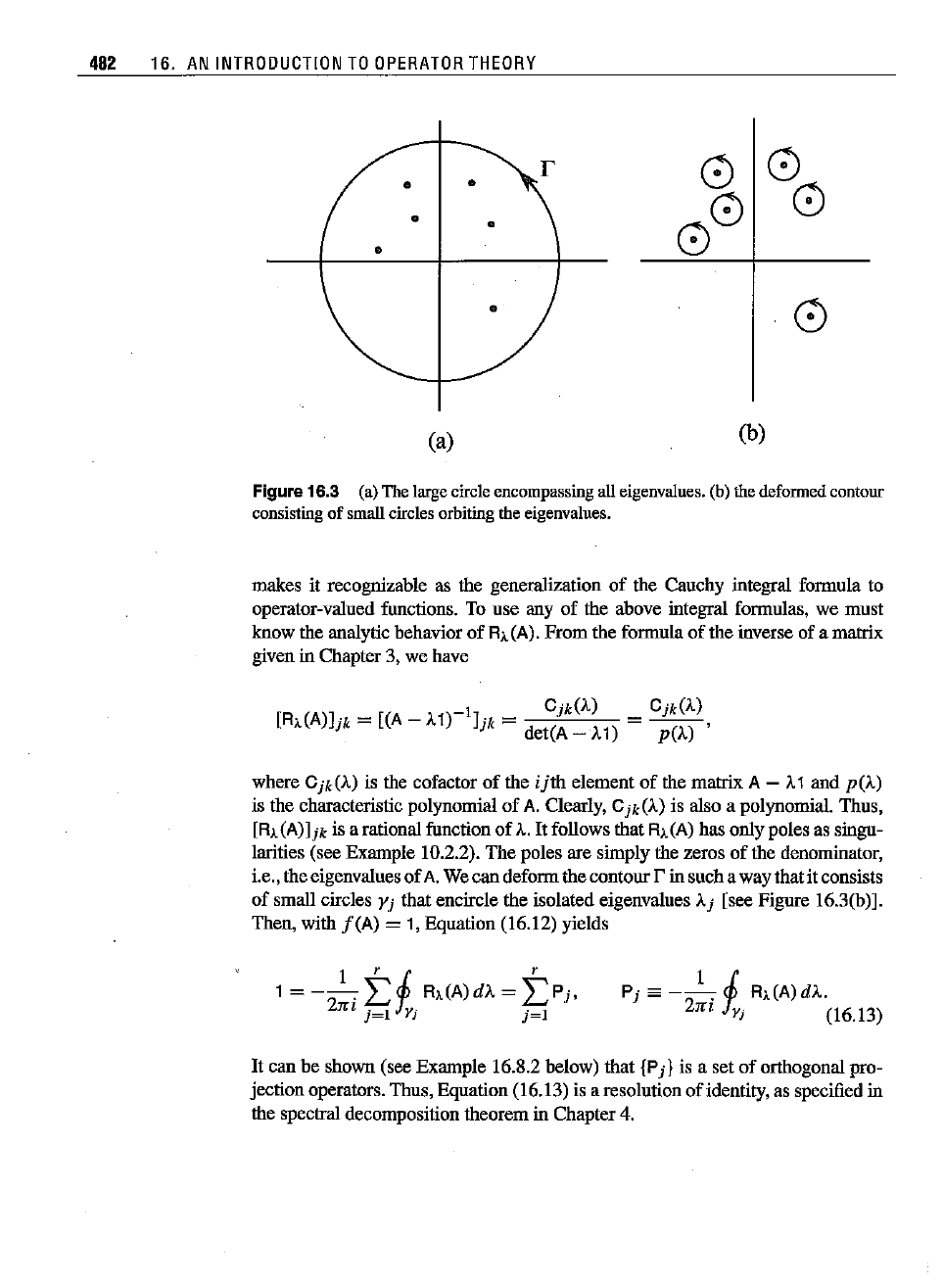
Figure 16.3 (a)Thelargecircleencompassing alleigenvalues. (b)the
deformed
contour
consisting of smallcircles
orbiting
theeigenvalues.
makes it recognizable as the generalization
of
the Cauchy integral formula to
operator-valued functions. To use any of the above integral formulas, we must
know the analytic behavior
of
RJ.(A).
From
the formula
of
the inverse of a matrix
given in Chapter 3, we have
where
Cjk(J..) is the cofactor
of
the
ijth
element
of
the matrix A -
J..
1 and p(J..)
is the characteristic polynomial of A. Clearly, Cjk(J..) is also a polynomial. Thus,
[RJ.
(A)]jk is a rational function
of
J...
It
follows that
RJ.
(A)has ouly poles as singu-
larities (see Example 10.2.2). The poles are simply the zeros
of
the denominator,
i.e., the eigenvalues
of
A.We
can
deformthe contourr in such a way that itconsists
of small circles
Yj that encircle the isolated eigenvalues
J..
j [see Figure 16.3(b)].
Then, with
f(A)
= 1, Equation (16.12) yields
Pj
sa
-~
J RJ.(A)dJ...
2n,
r"j
(16.13)
It can be shown (see Example 16.8.2 below) that {P
j)
is a set of orthogonal pro-
jectionoperators. Thus, Equation (16.13) is a resolution
of
identity, as specified in
the spectral decomposition theorem in Chapter 4.
