Hall A.S., Archer F.E., Gilbert R.I. Engineering Statics

This Page Intentionally Left Blank

d

physics require a howled

book, it is appropriate to consider

istributed load acting on a plane surface. The

fluid pressure on submer

be applicable to problems involving stresses, and to more

ab

r

first the case

of

a

lo

of

uniform intensity

W

(

The load acting on an elemental area

dA

is:

dW=

W

and the total load

is:

W=

IWdA

=

W~dA

=

WA

where

A

is the area

of

the figure. For figures of simple shape, such as those occurring in

engineerillg, the value

of

A

is known from earlier studies. nly for unusual shapes is

integration required.

W

is the resultant of the parallel forces acting on the elemental

areas. It may be thought

of

as the volume

of

the

~~ess~~e

~~uc~

(Figure

A.

1)

of area

A

and

thickness

W,

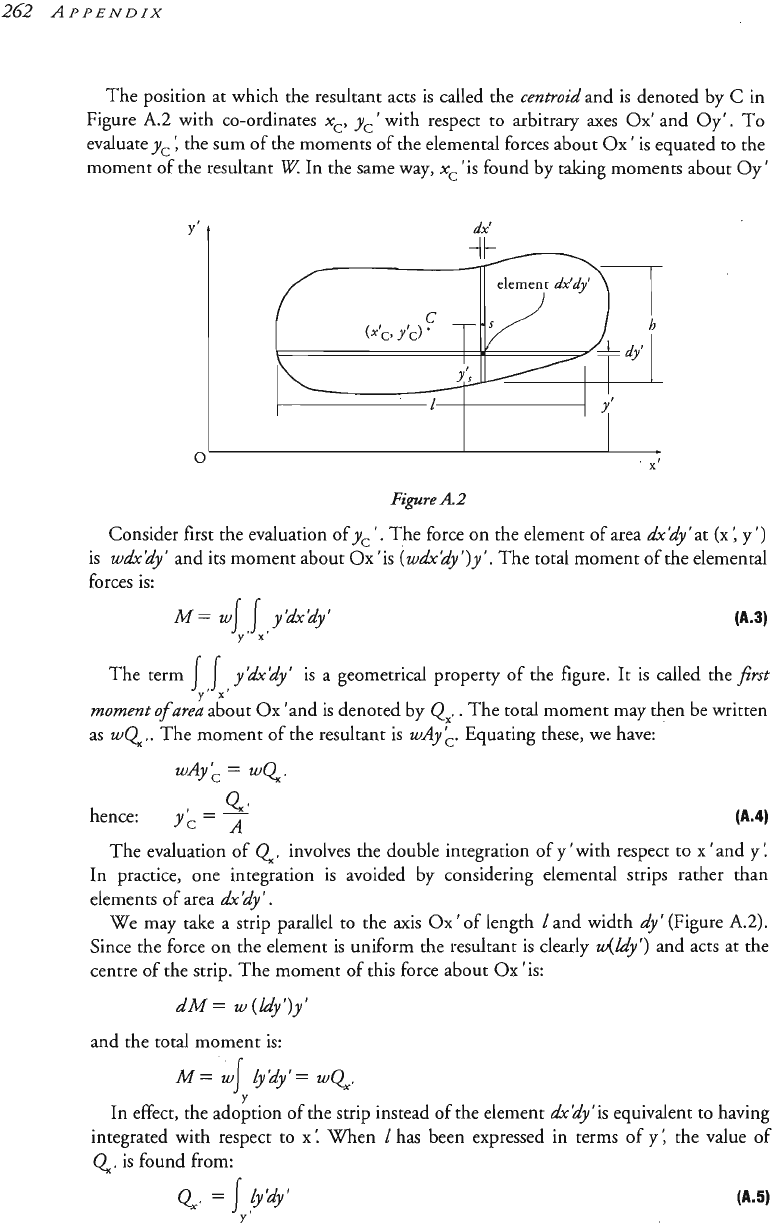
The position at which the resultant acts is calle

re

A.2

with co-ordinates

xc,

yc

'

with respect to arbit

e

yc

',

the sum of the moments of the elemental forces

t

of the resultant

CEi:

In the same way,

xc

'is

found by

Y'

'

dX'

U

.

X'

er first the evaluation

of

yc

'

(I

on

the element

of

area

is

'

and its moment about

Ox

'i

y

'.

The total moment

forces is:

M=

W/

J

,y~~yf

YX

Zy'

is a geometrical property of the figure. It

is

called the

st

and

is

denoted by

Q),

.

The total moment may then be written

,

The moment of the resultant

is

WAY

L.

Equating these, we have:

,

involves the double in ation of y 'with respect to

x

'

and y

'.

ation is avoided by c ering elemental strips rather than

We may take a strip parallel to the axis

Ox

'

of length

I

and width

dy

'

(Figure

A.2).

ince the force on the element

is

uniform the resultant is clearly

~~y')

and acts at the

elements of area

centre of the strip. The moment of this force about

Ox

'is:

dM

=

W

(~y')y'

and the total moment is:

strip instead

of

the element

d3c'dy

'is equiv~e~t to having

en

I

has been expressed in terms of

y;

the value of

GEOMETRICAL PROPERTIES

OF

PLANE FIGURES

263

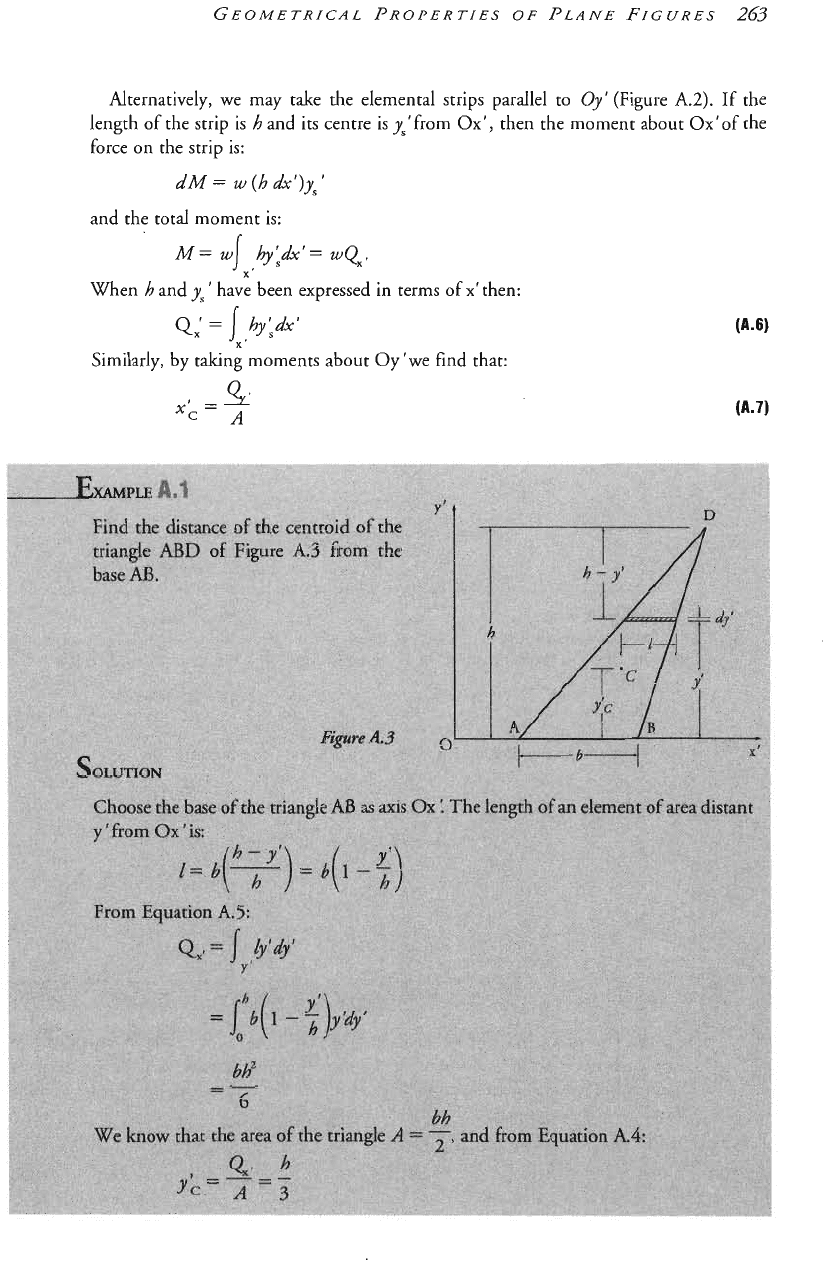
~ternatively,

we

may take the elemental strips parallel to

Oy'

(Figure

A.2).

If

the

length

of

the strip

is

h

and its centre

is

ys'from Ox', then the moment about Ox'of the

force

on

the strip

is:

dM

=

W

(h

&')&

'

and the total moment is:

M==

W/

by:&'=

wQ,

X(

VUhen

h

and

ys

'

have been expressed in terms

of

x' then:

cr:

=

J"

,h&&'

X

Similarly, by taking moments about Oy

'we

find that:

1".

%

.xc

-

A

It

is

usual to use the symbols Cx, Cy for axes with origin at the centroid. The initial

convenient axes are therefore called Ox ‘and Oy

‘.

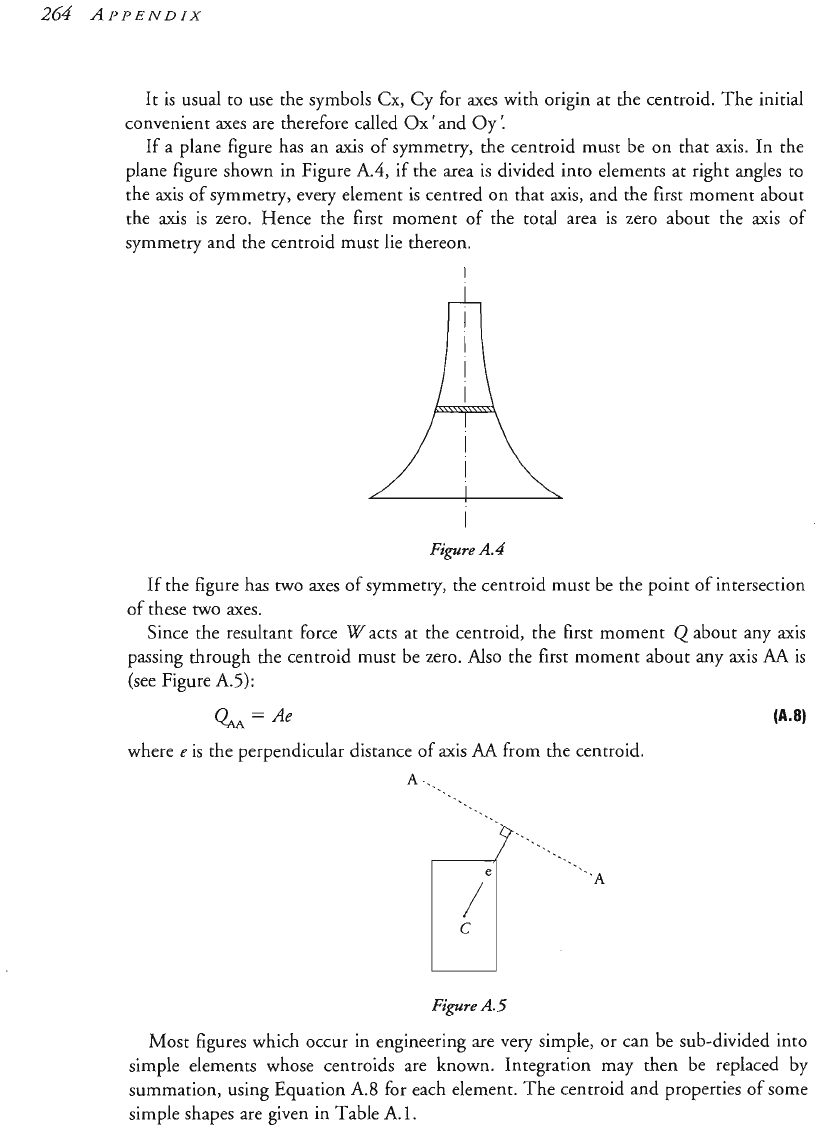
If a plane figure has an axis of symmetry, the centroid must be on that axis. In the

plane figure shown in Figure

A.4,

if the area is divided into elements at right angles to

the axis of symmetry, every element is centred on that axis, and the first moment about

the axis is zero. Hence the first moment of the total area is zero about the axis of

symmetry and the centroid must lie thereon.

1

If the figure has

two

axes of symmetry, the centroid must be the point

of

intersection

of

these

two

axes.

Since the resultant force Wacts at the centroid, the first moment

Q

about any axis

passing through the centroid must be zero.

Also

the first moment about any axis

AA

is

(see Figure

A.5):

Q,

=

Ae

where

e

is the perpendicular

distance of axis

AA

from

A

’..

7..

..

the centroid.

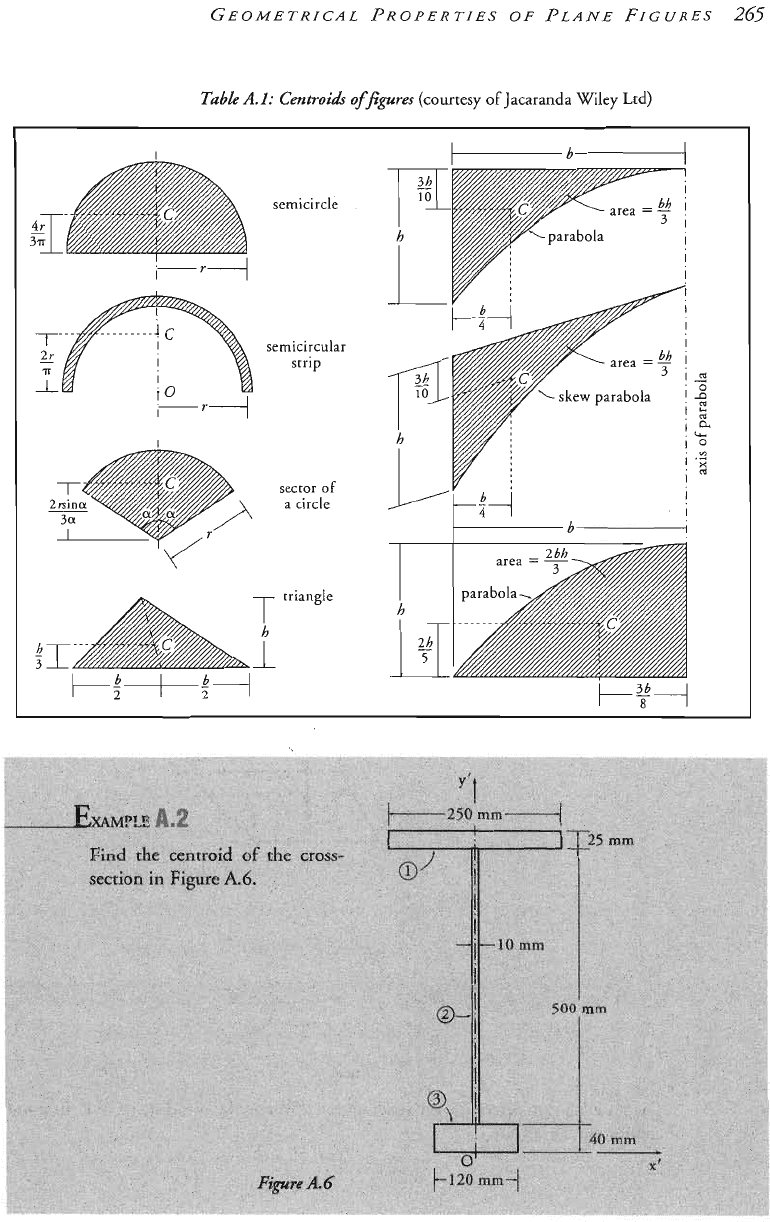
Most figures which occur in engineering are very simple,

or

can be sub-divided into

simplie elements whose centroids are known. Integration may then be replaced by

summation, using Equation

A.8

for each element. The centroid and properties of some

simple shapes are given in Table

A.

1.

Table

A,

I:

~~~oi~

o~~~~es

(courtesy

of

Jacaranda Wiley Ltd)

I

semicircle

semicircular

strip

sector of

a

circle

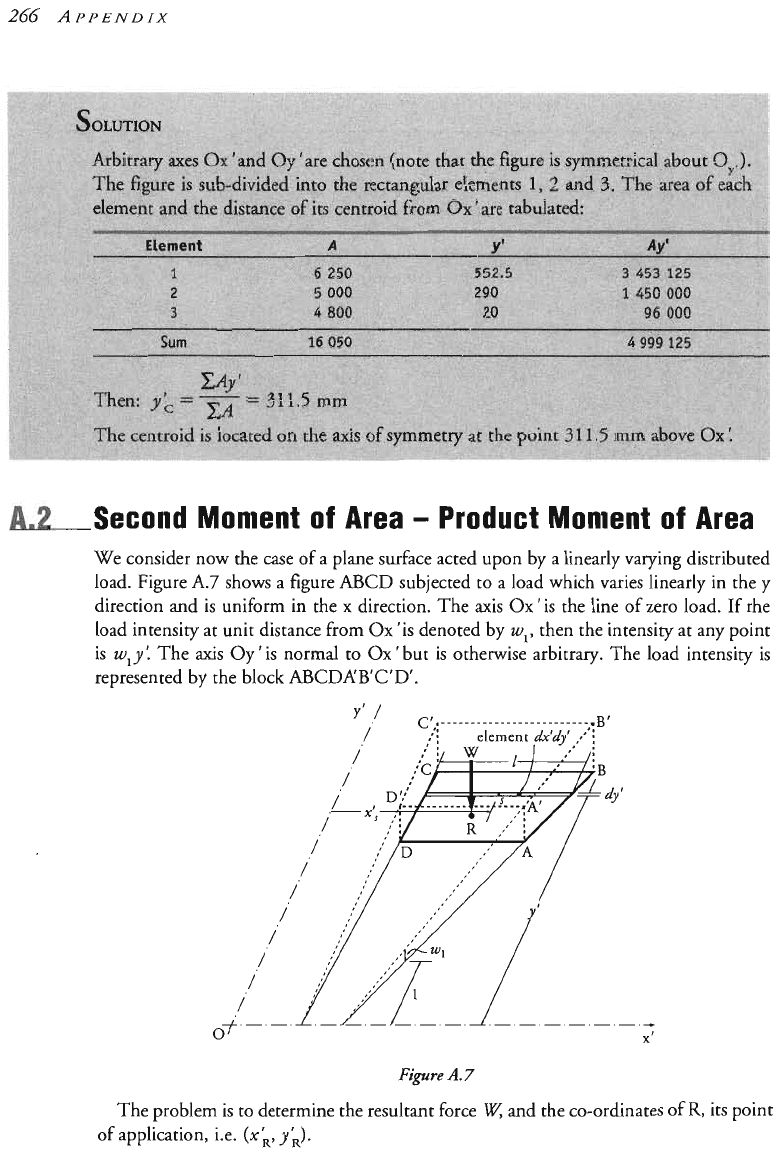
We consider now the

case

of a plane surface acted upon by a linearly varying distributed

load. Figure

A.7

shows a figure

ABCD

subjected to a load which varies linearly in the y

direction and is uniform in the

x

direction, The

axis

Ox

'

is the line

of

zero load.

If

the

load intensity at unit distance from

Ox

'is denoted by

wl,

then the intensity at any point

is

wlyl

The

axis

Oy

'

is normal to

Ox

'

but is otherwise arbitrary. The load intensity

is

represented by the block

ABCDAB'C'D'.

Y'

!

~~~""""""""""-----

I'

,

element

$x'd

I

,+'

E

*:B'

I

I'

Pipre

R

7

The problem is to determine the resultant force

K

and the co-ordinates of

R,

its point

of application, i.e.

(xi,

y

L).

Consider an elemental area distant

y

'from

Ox

The load intensity here is

wly

t

The force

on the element

is:

dW=

(~,y')~'dy'

and the total load is:

'dy'= wlIIy~~y'

The integral

I~y~'dy'

is the first moment of area about

Ox

'.

Hence:

W=

WIQ,

If the distance from the centroid to axis

Ox'

is

y:,

then:

Q,=yLA

and

W=

w,y',A

But

wtyc'is

the intensity of loading at the centroid, which may be denoted by

wc.

So

the total load is the intensity of load at the centroid times the area of the figure:

W=

R

Since the load intensity varies in one direction and not in the other, the ~etermination

of

.xk

and

y

will be different. To find the distance of

R

from

Ox

we take moments

about

Ox

t

The moment of the element force

(

wly

')

dx

U''

about

Ox

'

is:

~~',

=

(w1yfdx'dy')yf

=

~~y'2~'dyf

Hence the

sum

of the ~o~ents of

all

the element forces about

Ox

'is:

The term

ff

y

'2&'dy'

is a geometrical property of the figure.

It

is called the

second

~u~ent

ofarea

about

Ox

'and is denoted by

I'

j,,

That is:

rxk,

=

ffy~2~'dyf

and:

IW~,

=

wsrxk,

The moment

of

the resultant about

Ox

'is

Wy

K

and from Equation

A.

1

:

Wy~

=

(w~y~A)y~

'x'

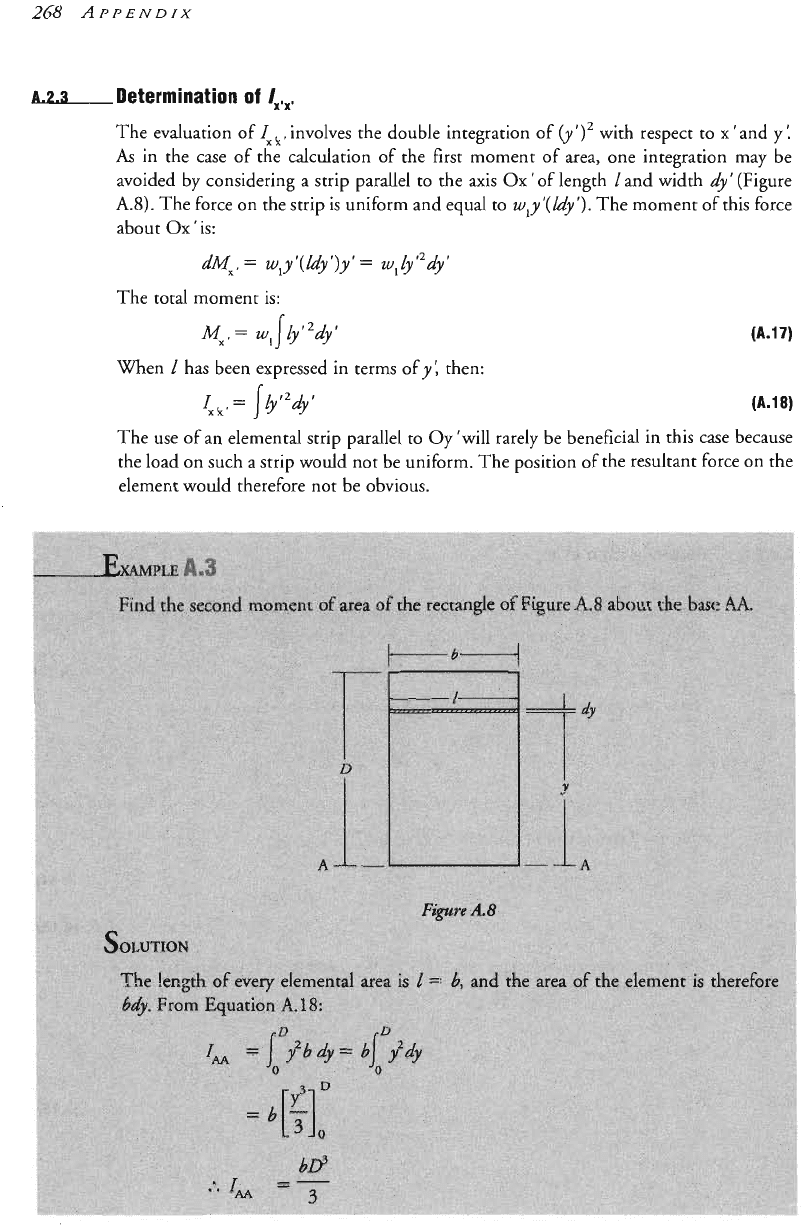
The evaluation of

L

k,

involves the double integration of

(J

')'

with respect to x

'

and y

As

in the case of the calculation of the first moment of area, one integration may be

avoided by considering a strip parallel to the

axis

Ox

of length

I

and width

dy

'

(Figure

AS).

The force on the strip is uniform and equal to

wly

'(

Uy

').

The moment of this force

about Ox 'is:

dMx,

=

~~y~(~yf)yf

=

w,~f2dyf

The total moment is:

M',

=

~1f~~2dyf

Ix,.

=

f~f2dy'

When

I

has been expressed in terms of

y

:

then:

The use of

an

elemental strip parallel to Oy will rarely be beneficial in this case because

the load on such a strip would not be uniform. The position of the resultant force on the

element would therefore not be obvious.

f

the resultant W from Oy

’

in Figure

12.7

(page

266),

we take

moments about Oy The force on an element

(dx

Uy

’)

is

WJ

’(dx

Uy

‘)

and the moment

of this force about Oy

‘

is:

~~,,

=

W~y’(~Uy‘)x‘

The total moment is:

IMy’

=

w~~~yj,~~y‘

‘xt.

=

J”

yj,~Uy’

The term

J”/y

j,

Xy

Uy

’

is a geometrical property called the

~rud~ct

~u~e~t

ufdrea

and

is denoted by

IX,,,

Y

x*

and:

M,,

=

wlrx,,,

The moment of the resultant about Oy ‘is:

WXC,

=

(W1y~A)xC,

Hence:

(w,y

&4)x

C,

=

w1

I’

‘Y’

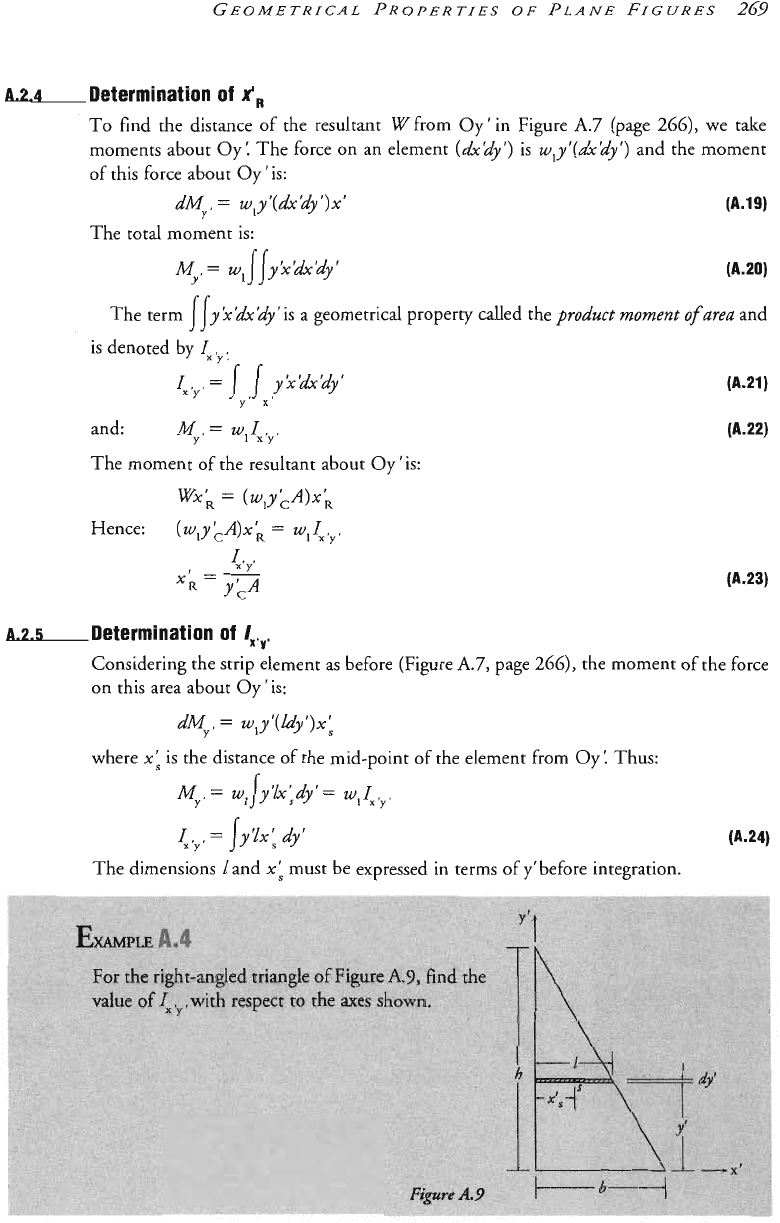
Considering the strip element

as

before (Figure

12.7,

page

266),

the moment of the force

on this area about Oy

‘

is:

dMy

,

=

WJ

’(

Ldy

‘)x

6

where x: is the distance of the mid-point of the element from

Oy

Thus:

My.

=

w,J”~~~d~f=

WIIxp.

<‘,,

=

/yYx:

dy’

The dimensions Land x: must be expressed in terms of y’before integration,

Hall A.S., Archer F.E., Gilbert R.I. Engineering Statics

Подождите немного. Документ загружается.