Greiner W., Schrammm S., Stein E. Quantum Chromodynamics

Подождите немного. Документ загружается.

16 1. The Introduction of Quarks

Exercise 1.2

Table 1.3. The eigenvalues of the Casimir

operator

for the regular representation



ijm

12f

123

+2 f

147

+2 f

156

22f

123

+2 f

246

+2 f

257

32f

123

+2 f

345

+2 f

367

42f

246

+2 f

345

+2 f

147

+2 f

458

52f

156

+2 f

257

+2 f

345

+2 f

458

62f

156

+2 f

246

+2 f

367

+2 f

678

72f

147

+2 f

257

+2 f

367

+2 f

678

82f

458

+2 f

678

Solution. Each irreducible representation of SU(3) is uniquely determined by

the eigenvalues of its Casimir operators. Each state in a multiplet has the same

eignvalues with respect to

. Thus this operator must be proportional to the unit

matrix. This is checked here using an example. Using (1) it follows for

that

(

)

=−



i, j

ilj

ijm

. (3)

From the Table 1.2 on page 6 of the f

ijk

, one recognizes that f

ilj

= 0and f

ijm

=

0, which implies that l = m:

(

)



i, j

ijm

= 3δ

. (4)

This proves (2).

2. Review of Relativistic Field Theory

2.1 Spinor Quantum Electrodynamics

As a general introduction, this section reviews the basics of spinor quantum elec-

trodynamics that are referred to in the following text.

Section 2.2 will give

a similar review of scalar quantum electrodynamics. Readers who are familiar

with this material should continue with Chap. 3.

2.1.1 The Free Dirac Equation and Its Solution

The equation of motion for the free spinor ﬁeld Ψ is the free Dirac equation (we

use natural units,

 = c = 1):

∂

∂t

Ψ =(−i

α ·∇ −

βm

)Ψ ;







110

0 −



. (2.1)

The components

α and

β are Hermitian 4 ×4 matrices, i. e.

†

and

†

β. The solutions of (2.1) are of the form

Ψ =we

−i p·x

, (2.2)

where

p ≡ p

=( p

, p)



note: p

= ( p

, −p)



(2.3)

is the momentum four-vector and w a four-component Dirac spinor. The

spinor w is usually decomposed into the two two-component spinors ϕ and χ:

w =





. (2.4)

With this, the Dirac equation becomes a coupled system of equations for ϕ

and χ:







σ · p

σ · p −m





, (2.5)

For a detailed discussion see W. Greiner: Relativistic Quantum Mechanics – Wave

Equations, 3rd ed. (Springer, Berlin, Heidelberg 2000)

18 2. Review of Relativistic Field Theory

where 11 =





is the 2 ×2 unit matrix and

σ the vector of the 2 ×2Pauli

matrices. Equation (2.5) is a homogeneous system of equations for ϕ and χ.The

coefﬁcient determinant has to vanish, i. e.

det



( p

−m

)11 −

σ · p

−

σ · p ( p

)11



=( p

)

−m

−



σ · p



=0 . (2.6)

Using the well-known relation



σ · A



σ ·B



= A·B 11+i

σ ·

(

A× B

)

(2.7)

yields

( p

)

= p

. (2.8)

It possesses solutions of positive and negative energy.

Plane Waves of Positive Energy. In this case

≡ E =+



> 0 . (2.9)

Exploiting the covariance of the Dirac equation, we ﬁrst give the solutions for

a particle at rest for which

, p = 0 (2.10)

holds. The system of equations (2.5) then has the form







110

0 −m





(2.11)

and leads to

χ = 0 (2.12)

and

w( p

) =





. (2.13)

The two linearly independent solutions for the two-spinor ϕ are





(spin ↑),





(spin ↓), (2.14)

see W. Greiner: Quantum Mechanics – An Introduction, 4th ed. (Springer, Berlin,

Heidelberg, 2000), Exercise 13.2.

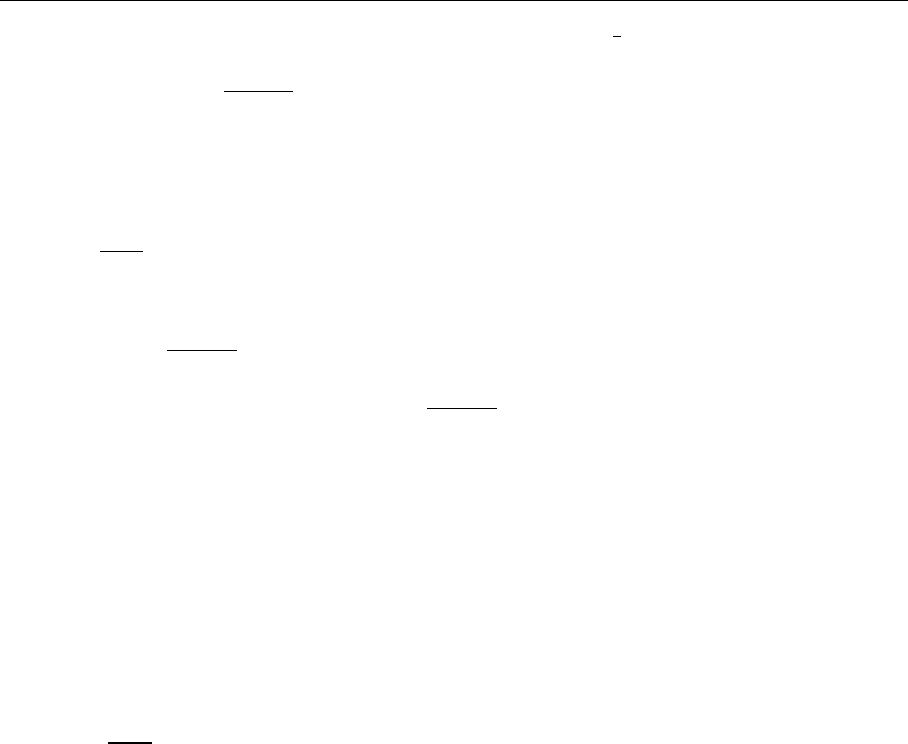
2.1 Spinor Quantum Electrodynamics 19

which clearly shows that the Dirac equation describes particles of spin

.For

nonvanishing spatial momentum

p =0 , p

= E =



, (2.15)

χ can be expressed by ϕ using (2.5), and one obtains the spinors of positive

energy in the form



σ·p

E+m



, s = 1, 2 . (2.16)

Plane Waves of Negative Energy. These are characterized by

≡−E =−



, (2.17)

where E always indicates the positive square root



, i.e., in this notation

E > 0. To construct the solutions we proceed as above. For a particle at rest with

=( p

=−m

, p = 0) a system analogous to (2.11) leads to

ϕ = 0 (2.18)

and to the four-spinor

w( p

=−m

) =





, (2.19)

respectively. For nonvanishing spatial momentum, i.e., for the four-momentum

=(−E, p), ϕ can now be eliminated and one obtains

w =



−

σ·p

E+m



. (2.20)

We give the following important deﬁnition, which can be understood from hole

theory. A particle (electron) is identiﬁed with a solution of positive energy and

positive momentum p, i.e.,

Ψ ∼e

−i p·x

, p = (E, p), (2.21)

and an antiparticle (positron) with the solution of negative energy and negative

momentum, i.e.,

Ψ ∼e

i p·x

= e

−i(−p·x)

≡ e

−i p



·x

, p



= (−E, −p). (2.22)

The particle and antiparticle solutions are therefore connected by the transform-

ation

→−p

. (2.23)

20 2. Review of Relativistic Field Theory

Similarly, one expects for the spin that a missing particle of spin ↑ corresponds

to an antiparticle of spin ↓. In other words, electron solutions of negative energy,

negative momentum, and spin ↓ correspond to positron solutions of positive

energy, positive momentum, and spin ↑. For this reason one puts





and χ





. (2.24)

The four-spinors w representing particles and antiparticles are now



−

σ·p

E+m



, s = 1, 2 . (2.25)

With deﬁnitions (2.21)–(2.24) it is guaranteed that the quantities E and p,as

well as the basis spinors χ

and χ

that appear in the solutions (2.25), always

denote energy, momentum, and spin ↑ or spin ↓ of the (physically observed)

antiparticle.

2.1.2 Density and Current Density

The density  and current density j of the Dirac ﬁeld are, independent of the sign

of the energy, given by

 = Ψ

†

Ψ, (2.26a)

j = Ψ

†

αΨ, (2.26b)

and satisfy the continuity equation

∂

∂t

 +∇ · j =0 . (2.27)

For any spinor of the form

Ψ =w u(x, t) (2.28)

it follows that

 = w

†

w|u(x, t)|

, (2.29a)

j = w

†

αw|u(x, t)|

. (2.29b)

Obviously,  ≥ 0 always holds, and this is independent of (2.28) designating

a particle or an antiparticle solution. Similarly, j does not change its sign when

moving from particle to antiparticle solutions. This is most quickly veriﬁed for

2.1 Spinor Quantum Electrodynamics 21

the z component:

( j

)

−

↑

⎛

⎜

⎝





E+m





⎞

⎟

⎠

†





⎛

⎜

⎝





E+m





⎞

⎟

⎠

E +m

( j

)

↑

⎛

⎜

⎝

−

E+m









⎞

⎟

⎠

†





⎛

⎜

⎝

−

E+m









⎞

⎟

⎠

E +m

(2.30)

A sign change of charge and current density is, however, desired. To put it in by

hand one inserts an extra minus sign whenever an electron (fermion) of nega-

tive energy appears in a ﬁnal state. This rule is very naturally included in the

deﬁnition of the Feynman propagator.

2.1.3 Covariant Notation

It is customary to introduce γ matrices, which replace

α and

β (we leave out the

operator hats for γ matrices in the following):

β, (γ

)

= 11 ,

,(γ

)

=−11 , i = 1, 2, 3 ,

+γ

= g

µν

11 ,(γ

)

†

= γ

. (2.31)

Here 11 designates the unit matrix. The free Dirac equation (2.1) takes the form



iγ

∂

∂x

−m



Ψ =0 ,

(i∂/ −m

)Ψ =0 , (2.32)

using the (Feynman) dagger notation (a/ ≡ γ

). Density  and current dens-

ity j can be combined to form a four-current density:

Ψγ

Ψ, (2.33)

and the continuity equation (2.27) can be written as a four-divergence:

∂

∂x

=0 . (2.34)

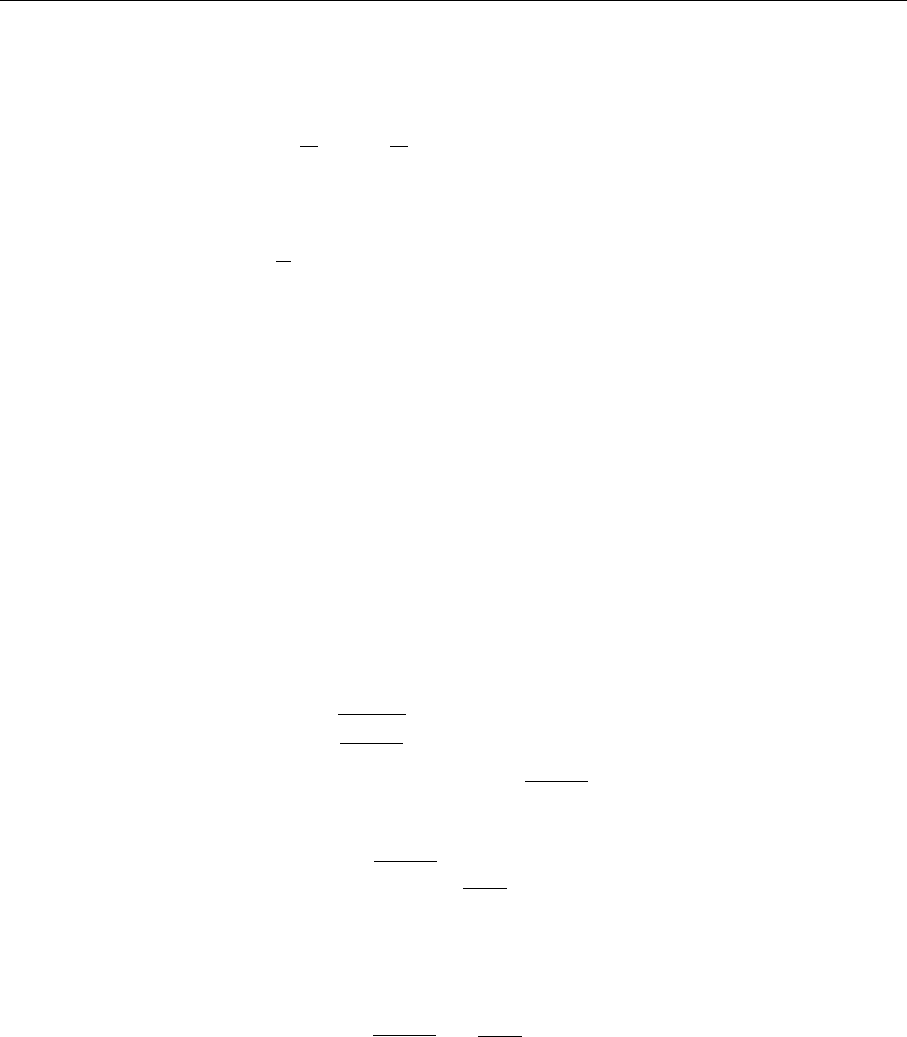
22 2. Review of Relativistic Field Theory

Here

Ψ =Ψ

†

(2.35)

designates the adjoint spinor. It obeys the equation

i∂

Ψγ

Ψ = 0 . (2.36)

For plane waves (2.25), equations (2.32) and (2.36) become

( p/ −m

)w = 0 ,

w( p/ −m

) = 0 ,

(2.37)

respectively.

2.1.4 Normalization of Dirac Spinors

It is useful to consider again the normalization of spinor wave functions. Let us

ﬁrst consider plane waves of positive energy,

1,2

= N



1,2

−i p·x

. (2.38)

We normalize such a wave in a box of volume V in such a way that

x  = 2E (2.39)

holds. This normalization differs from the usual normalization to unity of quan-

tum mechanics but is often used in ﬁeld theory. Using the explicit form of the

spinors ω

1,2

of positive energy yields the normalization factor



E +m

. (2.40)

Usually one absorbs the factor

√

E +m

in the deﬁnition of the spinor w

and

designates the spinors for positive energy by u( p, s):

u(p, s) =

E +m



σ·p

E+m



, s = 1, 2 ,





,ϕ





. (2.41)

One similarly introduces spinors v( p, s) for negative energy:

v( p, s) =

E +m



−

σ·p

E+m



, s = 1, 2 ,





,χ





. (2.42)

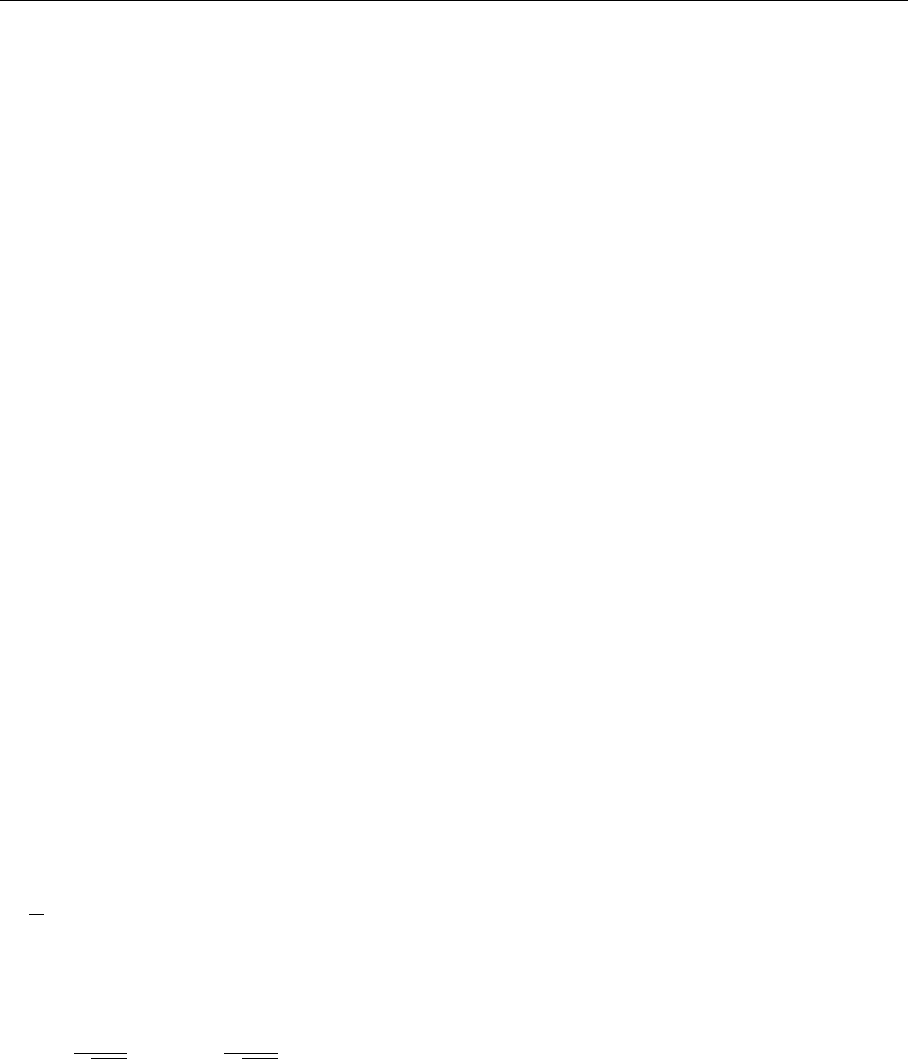
2.1 Spinor Quantum Electrodynamics 23

The plane waves for electrons and positrons now read

Ψ( e

−

) = u( p, s) e

−i p·x

Ψ( e

) = v( p, s) e

+i p·x

(2.43)

respectively. We again emphasize that the spinors v( p, s) are constructed such

that E, p,ands = 1, 2 in (2.42) correspond to energy, momentum, and spin

projection ↑ or ↓ of the positron. It is easy to check that

†

u = v

†

v = 2E (2.44)

and, utilizing (2.37) and (2.43),

( p/ −m

)u = 0 , (2.45a)

( p/ +m

)v = 0 (2.45b)

hold. Equations (2.45) are the momentum-space Dirac equation for the (free) so-

lutions of positive and negative energy, respectively. Correspondingly one ﬁnds

the Dirac equations for the adjoint spinors

u and

u(p/ −m

) = 0 , (2.46a)

v(p/ +m

) = 0 . (2.46b)

The normalization conditions for the spinors can be summarized as

u(p, s)u( p



, s



) = 2m



, (2.47a)

v(p, s)v( p



, s



) =−2m



. (2.47b)

It is customary to unify the spinors u and v by deﬁning

( p) = u( p, 1),

( p) = u( p, 2),

( p) = v( p, 1),

( p) = v( p, 2),

(2.48)

so that equations (2.47a) and (2.47b) can be summarized as

( p)w

( p



) = 2m



,ε

1forr = 1, 2

−1for r = 3, 4

. (2.49)

We want to emphasize that another normalization of u( p, s) and v( p, s) can also

quite often be found:



√

u ,v



√

v. (2.50)

The advantage of the normalization used here is its covariance. This is un-

derstandable from (2.44): the densities u

u and v

v are proportional to the

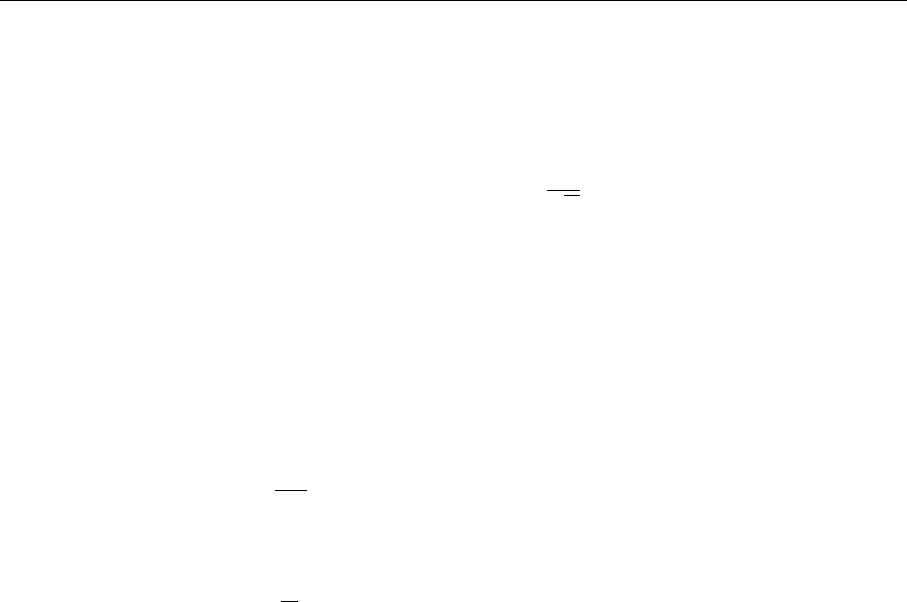
24 2. Review of Relativistic Field Theory

energy E and transform as 0 components of a four-vector. When we compute

cross sections, this and the corresponding transformation properties of phase

space and ﬂow factors make Lorentz invariance obvious.

With these conventions we can write electron and positron wave functions as

Ψ(e

−

) = Nu ( p, s)e

−i p·x

, (2.51a)

Ψ(e

) = Nv( p, s)e

i p·x

, N =

√

. (2.51b)

These explicit expressions enable us to write down directly the transition cur-

rents, as we shall see below.

2.1.5 Interaction with a Four-Potential A

The interaction of the ﬁeld with an electromagnetic potential A

is introduced by

the so-called “minimal” coupling to preserve gauge invariance. For an electron

(of charge −e), the minimal substitution is

∂

∂x

≡∂

→∂

−ieA

. (2.52)

Thus the free Dirac equation (2.1) is changed into (written in noncovariant form)

∂

∂t

ψ = (−i

α ·∇ +

βm

V )ψ , (2.53)

where the interaction

V is given by

V =−eA

11 +e

α · A . (2.54)

In covariant form, the Dirac equation with interaction (substitution of (2.57) into

(2.32)) reads



iγ

(∂

−ieA

) −m



Ψ =0 (2.55)



iγ

∂

−m



Ψ =−eγ

Ψ ≡+γ

V Ψ, (2.56)

where the interaction is written as

V =−eγ

. (2.57)

2.1 Spinor Quantum Electrodynamics 25

2.1.6 Transition Amplitudes

The transition amplitude (S-matrix element) of an initial electron state

−

; p, s) with four-momentum p andspinprojections into a ﬁnal electron

state Ψ

−

; p



, s



) characterized by momentum p



and spin s



is, in ﬁrst-order

perturbation theory,

(1)

=−i

x Ψ

†



−

; p



, s





V Ψ



−

; p, s



=−i

x Ψ

†



−

; p



, s





V Ψ



−

; p, s



=−i



−

; p



, s





−eγ





−

; p, s



=−i



−



(2.58)

where



−



= (−e)



−

; p



, s







−

; p, s



(2.59)

are the electron (fermion) transition current densities. Using the plane waves

(2.51a) this becomes explicitly

−

) =

(−e)

( p



, s



)γ

( p, s) e

i( p



−p)·x

, N

= N

√

(2.60)

and now allows the calculation of scattering process in lowest order according

to (2.58).

2.1.7 Discrete Symmetries

We restrict ourselves here to a summarizing and tabulating the properties of the

discrete symmetry transformations parity

P, charge conjugation

C, and time

reversal

T .

Dirac ﬁelds can be combined into the following bilinear forms (currents),

distinguished by their tensor character:

S(x) =

Ψ(x)Ψ(x) scalar , (2.61a)

(x) =

Ψ(x)γ

Ψ(x) vector , (2.61b)

µν

(x) =

Ψ(x)σ

µν

Ψ(x) tensor , (2.61c)

P(x) = i

Ψ(x)γ

Ψ(x) pseudoscalar , (2.61d)

(x) =

Ψ(x)γ

Ψ(x) pseudovector . (2.61e)

The behavior of these currents under the transformations

T ,aswellas

under the mixed symmetry

O =

T , is given in Table 2.1 where

x = (t, −x).