Gregersen E. (editor) The Britannica Guide to Statistics and Probability
Подождите немного. Документ загружается.

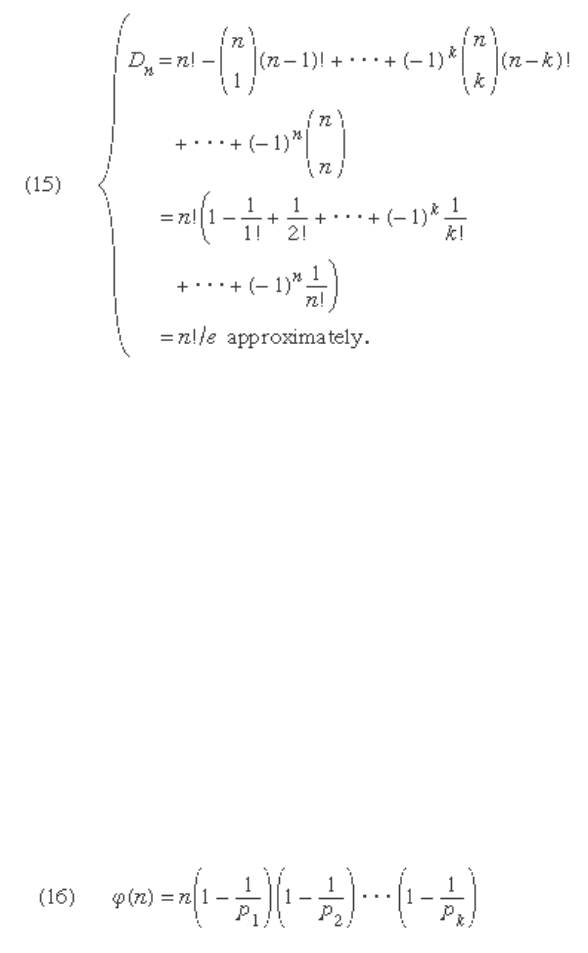
7 The Britannica Guide to Statistics and Probability 7
210
example. Hence the number D
n
of derangements can be
shown to be approximated by n !/ e
This number was fi rst obtained by Euler. If n persons
check their hats in a restaurant, and the waiter loses the
checks and returns the hats at random, then the chance
that no one receives his own hat is D
n
/ n ! = e
-1
approximately.
It is surprising that the approximate answer is indepen-
dent of n . To six places of decimals e
-1
= 0.367879. When n =
6 the error of approximation is less than 0.0002.
If n is expressed as the product of powers of its prime
factors p
1
, p
2
, · · · p
k
, if the objects are the integers less than
or equal to n , and if A
i
is the property of being divisible
by p
i
, then Sylvester’s formula gives, as the number of
integers less than n and prime to it, a function of n , writ-
ten ϕ( n ), composed of a product of n and k factors of the
type (1 - 1/ p
i
)
.
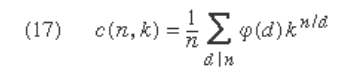
211
7
Combinatorics 7
The function ϕ( n ) is the Euler function.
Polya’s Theorem
It is required to make a necklace of n beads out of an infi -
nite supply of beads of k different colours. The number of
different necklaces, c ( n , k ), that can be made is given by
the reciprocal of n times a sum of terms of the type ϕ( n )
k
n / d
, in which the summation is over all divisors d of n and ϕ
is the Euler function
.
Though the problem of the necklaces appears to be
frivolous, the formula given above can be used to solve a
diffi cult problem in the theory of Lie algebras, of some
importance in modern physics.
The general problem of which the necklace problem is
a special case was solved by the Hungarian-born American
mathematician George Polya in a famous 1937 memoir in
which he established connections between groups, graphs,
and chemical bonds. It has been applied to enumeration
problems in physics, chemistry, and mathematics.
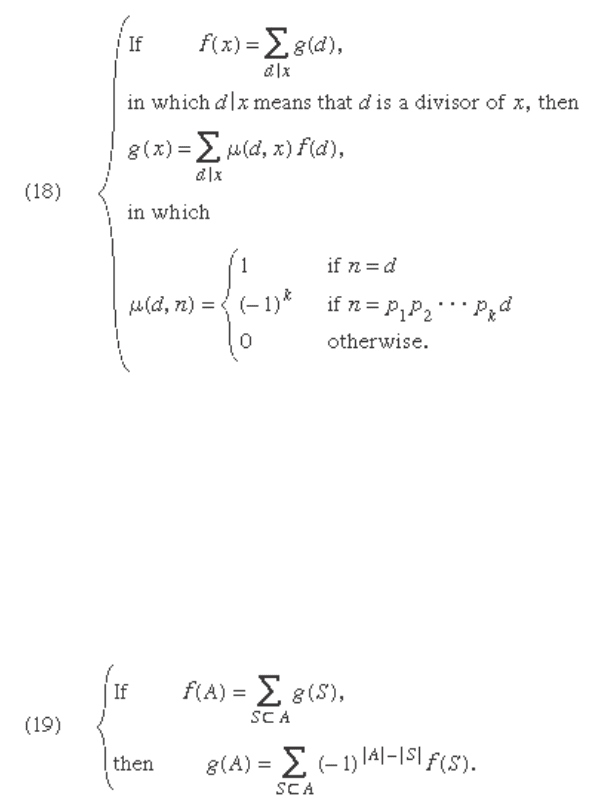
The Möbius Inversion Theorem
In 1832 the German astronomer and mathematician
August Ferdinand Möbius proved that if f and g are func-
tions defi ned on the set of positive integers, such that f
evaluated at x is a sum of values of g evaluated at divisors of
x , then inversely g at x can be evaluated as a sum involving
f evaluated at divisors of x
7 The Britannica Guide to Statistics and Probability 7
212
In 1964 the American mathematician Gian-Carlo
Rota obtained a powerful generalization of this theorem,
providing a fundamental unifying principle of enumera-
tion. One consequence of Rota’s theorem is the following:
If f and g are functions defi ned on subsets of a fi nite set A ,
such that f ( A ) is a sum of terms g ( S ), in which S is a subset
of A , then g ( A ) can be expressed in terms of f
Special Problems
Despite the general methods of enumeration already
described, there are many problems in which they do not
apply and therefore require special treatment. Two such
problems include the Ising problem and the self-avoiding
random walk.

213
7
Combinatorics 7
The Ising Problem
A rectangular m × n grid is made up of unit squares, each
coloured either red or green. How many different colour
patterns are there if the number of boundary edges
between red squares and green squares is prescribed?
This problem, although easy to state, proved diffi-
cult to solve. A complete and rigorous solution was not
achieved until the early 1960s. The importance of the
problem lies in the fact that it is the simplest model that
exhibits the macroscopic behaviour expected from cer-
tain natural assumptions made at the microscopic level.
Historically, the problem arose from an early attempt,
made in 1925, to formulate the statistical mechanics of
ferromagnetism. The three-dimensional analogue of the
Ising problem remains unsolved in spite of persistent
attacks.
Self-Avoiding Random Walk
A random walk consists of a sequence of n steps of unit
length on a flat rectangular grid, taken at random either in
the x- or the y-direction, with equal probability in each of
the four directions. What is the number R
n
of random
walks that do not touch the same vertex twice? This prob-
lem has defied solution, except for small values of n,
though a large amount of numerical data has been amassed.
pRobleMs of choice
Systems of Distinct Representatives
Subsets S
1
, S
2
, · · · , Sn of a finite set S are said to possess a set
of distinct representatives if x
1
, x
2
, · · · , x
n
can be found, such
that x
i
∊ S
i
, i = 1, 2, · · · , n, x
i
≠ x
j
for i ≠ j. It is possible that S
i
and S
j
, i ≠ j, may have exactly the same elements and are
7 The Britannica Guide to Statistics and Probability 7
214
distinguished only by the indices i, j. In 1935 American
mathematician, M. Hall, Jr., proved that a necessary and
sufficient condition for S
1
, S
2
, · · · , S
n
to possess a system of
distinct representatives is that, for every kn, any k of the n
subsets contain between them at least k distinct elements.
For example, the sets S
1
= (1, 2, 2), S
2
= (1, 2, 4), S
3
= (1, 2,
5), S
4
= (3, 4, 5, 6), S
5
= (3, 4, 5, 6) satisfy the conditions of the
theorem, and a set of distinct representatives is x
1
= 1, x
2
=
2, x
3
= 5, x
4
= 3, x
5
= 4. Conversely, the sets T
1
= (1, 2), T
2
= (1,
3), T
3
= (1, 4), T
4
= (2, 3), T
5
= (2, 4), T
6
= (1, 2, 5) do not possess
a system of distinct representatives because T
1
, T
2
, T
3
, T
4
,
T
5
possess between them only four elements.
The following theorem resulting from König is closely
related to Hall’s theorem and can be easily deduced from
it. Conversely, Hall’s theorem can be deduced from
König’s: If the elements of rectangular matrix are 0s and
1s, then the minimum number of lines that contain all of
the 1s is equal to the maximum number of 1s that can be
chosen with no two on a line.
Ramsey’s Numbers
If X = {1, 2, . . . , n}, and if T, the family of all subsets of
X containing exactly r distinct elements, is divided into
two mutually exclusive families α and β, the following
conclusion that was originally obtained by the British
mathematician Frank Plumpton Ramsey follows. He
proved that for r ≥ 1, p ≤ r, q ≤ r there exists a number N
r
(p,
q) depending solely on p, q, r such that if n > N
r
(p, q), there
is either a subset A of p elements all of the r subsets of
which are in the family α or there is a subset B of q ele-
ments all of the r subsets of which are in the family β.
The set X can be a set of n persons. For r = 2, T is the
family of all pairs. If two persons have met each other,
then the pair can belong to the family α. If two persons

215
7
Combinatorics 7
have not met, then the pair can belong to the family β. If
these things are assumed, then, by Ramsey’s theorem, for
any given p ≥ 2, q ≥ 2 there exists a number N
2
(p, q) such
that if n > N
2
(p, q), then among n persons invited to a party
there will be either a set of p persons all of whom have met
each other or a set of q persons no two of whom have met.
Although the existence of N
r
(p, q) is known, actual val-
ues are known only for a few cases. Because N
r
(p, q) = N
r
(q,
p), it is possible to take p ≤ q. It is known that N
2
(3, 3) = 6,
N
2
(3, 4) = 9, N
2
(3, 5) = 14, N
2
(3, 6) = 18, N
2
(4, 4) = 18. Some
bounds are also known; for example, 35 ≤ N
2
(4, 6) ≤ 41.
A consequence of Ramsey’s theorem is the following
result obtained in 1935 by the Hungarian mathematicians
Paul Erdös and George Szekeres. For a given integer n
there exists an integer N = N(n), such that a set of any N
points on a plane, no three on a line, contains n points
forming a convex n-gon.
design TheoRy
BIB (Balanced Incomplete Block) Designs
A design is a set of T = {1, 2, . . . , υ} objects called treatments
and a family of subsets B
1
, B
2
, . . . , B
b
of T, called blocks,
such that the block B
i
contains exactly k
i
treatments, all
distinct. The number k
i
is called the size of the block B
i
,
and the ith treatment is said to be replicated r
i
times if it
occurs in exactly r
i
blocks. Specific designs are subject to
further constraints. The name design comes from statisti-
cal theory in which designs are used to estimate effects of
treatments applied to experimental units.
A BIB design is a design with υ treatments and b blocks
in which each block is of size k, each treatment is repli-
cated r times, and every pair of distinct treatments occurs
together in λ blocks. The design is said to have the

7 The Britannica Guide to Statistics and Probability 7
216
parameters (υ, b , r , k , λ). Some basic relations are easy to
establish
.
These conditions are necessary but not suffi cient for
the existence of the design. The design is said to be proper if
k < υ—that is, the blocks are incomplete. For a proper BIB
design Fisher’s inequality b ≥ υ, or equivalently r ≥ k , holds.
A BIB design is said to be symmetric if υ = b , and con-
sequently r = k . Such a design is called a symmetric (υ, k , λ)
design, and λ(υ − 1) = k ( k − 1). A necessary condition for the
existence of a symmetric (υ, k , λ) design is given by the
following:
A. If υ is even, k − λ is a perfect square.
B. If υ is odd, a certain Diophantine equation
has a solution in integers not all zero.
For example, the designs (υ, k , λ) = (22, 7, 2) and (46, 10,
2) are ruled out by (A) and the design (29, 8, 2) by (B).
Because necessary and suffi cient conditions for the exis-
tence of a BIB design with given parameters are unknown,
it is often a diffi cult problem to decide whether a design
with given parameters (satisfying the known necessary
conditions) really exists.
Methods of constructing BIB designs depend on the
use of fi nite fi elds, fi nite geometries, and number theory.
Some general methods were given in 1939 by the Indian
mathematician Raj Chandra Bose, who has since emi-
grated to the United States.
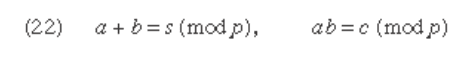
217
7
Combinatorics 7
A fi nite fi eld is a fi nite set of marks with two opera-
tions, addition and multiplication, subject to the usual
nine laws of addition and multiplication obeyed by ratio-
nal numbers. In particular the marks may be taken to be
the set X of non-negative integers less than a prime p . If
this is so, then addition and multiplication are defi ned by
modifi ed addition and multiplication laws
in which a , b , r , and p belong to X . For example, if p = 7,
then 5 + 4 = 2, 5 · 4 = 6. There exist more general fi nite fi elds
in which the number of elements is p
n
, p a prime. There is
essentially one fi eld with p
n
elements, with given p and n .
It is denoted by G F ( p
n
).
Finite geometries can be obtained from fi nite fi elds in
which the coordinates of points are now elements of a
fi nite fi eld. A set of k + 1 non-negative integers d
0
, d
1
, · · · , d
k
,
is said to form a perfect difference set mod υ, if among the
k ( k − 1) differences d
i
− d
j
, i ≠ j , i , j = 0, 1, · · · , k , reduced mod
υ, each nonzero positive integer less than υ occurs exactly
the same number of times λ. For example, 1, 4, 5, 9, 3 is a
difference set mod 11, with λ = 2. From a perfect difference
set can be obtained the symmetric (υ, k , λ) design using the
integers 0, 1, 2, · · · , υ − 1. The j th block contains the treat-
ments obtained by reducing mod υ the numbers d
0
+ j , j
1
+
j , · · · , d
i
+ j , j = 0, 1, · · · , υ − 1.
It can be shown that any two blocks of a symmetric (υ, k ,
λ) design intersect in exactly k treatments. By deleting one
block and all the treatments contained in it, it is possible to
obtain from the symmetric design its residual, which is a
BIB design (unsymmetric) with parameters υ* = υ − k , b * = υ
− 1, r * = k , k * = k − λ, λ* = λ. One may ask whether it is true that
a BIB design with the parameters of a residual can be
7 The Britannica Guide to Statistics and Probability 7
218
embedded in a symmetric BIB design. The truth of this is
rather easy to demonstrate when λ = 1. Hall and W.S. Connor
in 1953 showed that it is also true for λ = 2. The Indian math-
ematician K.N. Bhattacharya in 1944, however, gave a
counterexample for λ = 3 by exhibiting a BIB design with
parameters υ = 16, b = 24, r = 9, k = 6, λ = 3 for which two par-
ticular blocks intersect in four treatments and which for
that reason cannot be embedded in a symmetric BIB design.
A BIB design is said to be resolvable if the set of blocks
can be partitioned into subsets, such that the blocks in
any subset contain every treatment exactly once. For the
case k = 3, this problem was first posed during the 19th
century by the British mathematician T.P. Kirkman as a
recreational problem. There are υ girls in a class. Their
teacher wants to take the class out for a walk for a number
of days, the girls marching abreast in triplets. It is required
to arrange the walk so that any two girls march abreast in
the same triplet exactly once. It is easily shown that this is
equivalent to the construction of a resolvable BIB design
with υ = 6t + 3, b = (2t + 1)(3t + 1), r = 3t + 1, k = 3, λ = 1. Solutions
were known for only a large number of special values of t
until a completely general solution was finally given by the
Indian and American mathematicians Dwijendra K. Ray-
Chaudhuri and R.M. Wilson in 1970.
Pbib (Partially Balanced Incomplete
Block) Designs
Given υ objects 1, 2, · · · , υ, a relation satisfying the follow-
ing conditions is said to be an m-class partially balanced
association scheme:
A. Any two objects are either 1st, or 2nd, · · · , or
mth associates, the relation of association being
symmetrical.
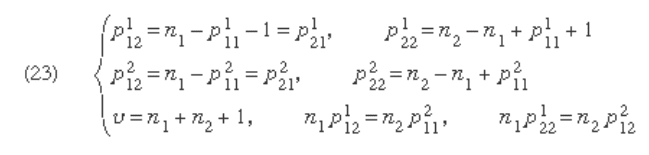
219
7
Combinatorics 7
B. Each object α has n
i
i th associates, the number
n
i
being independent of α.
C. If any two objects α and β are i th associates, then
the number of objects that are j th associates of
α and k th associates of β is p
j
k
i
and is indepen-
dent of the pair of i th associates α and β.
The constants υ, n
i
, p
j k
i
are the parameters of the asso-
ciation scheme. A number of identities connecting these
parameters were given by the Indian mathematicians Bose
and K.R. Nair in 1939, but Bose and the American math-
ematician D.M. Mesner in 1959 discovered new identities
when m > 2.
A PBIB design is obtained by identifying the υ treat-
ments with the υ objects of an association scheme and
arranging them into b blocks satisfying the following
conditions:
A. Each contains k treatments.
B. Each treatment occurs in r blocks.
C. If two treatments are i th associates, they occur
together in λ
i
blocks.
Two-class association schemes and the corresponding
designs are especially important both from the mathe-
matical point of view and because of statistical applications.
For a two-class association scheme the constancy of υ, n
i
,
p
11
1
, and p
11
2
ensures the constancy of the other parameters.
Seven relations hold :
.
