Graebel W.P. Advanced Fluid Mechanics
Подождите немного. Документ загружается.


164 Exact Solutions of the Navier-Stokes Equations
These equations can be considerably simplified by recognizing for this problem that
from the form of the stream function, the following holds:
v
R
R
=−
v
R
R
v
R
=−
v
R
Using these and some tedious but straightforward calculus, the Navier-Stokes equations
become
−
v
2
R
+v
2
R
−
v
sin
R
v
R
=−
p
R
+
R
2
2
v
R
2
−v
v
=−
p
−
R
v
R
(5.4.28)
The second of these can be integrated to obtain
p −p
0
=
−
v
2
2
+
v
R
v
R
+
c
1
R
2
(5.4.29)
Here, p
0
is the constant pressure at infinity. Since R was held constant during the
integration, the “constant of integration” c
1
is in fact a function of R. The radial
momentum equation dictates that it be proportional to R
−2
.
Using the form for the pressure, inserting it into the radial momentum equation,
and using the stream function, the result is
f
2
+ff
=2f
+
1−
2
f
−2c
1
Primes here denote differentiation with respect to . Integrating this once gives
ff
=2f +1−
2
f
−2c
1
−c
2
and once more gives
1
2
f
2
=2f +1−
2
f
−c
1
2
−c
2
−c
3
(5.4.30)
with boundary conditions f0 = f = 0.
Squire solved this for the special case c
1
=c
2
=c
3
=0, obtaining
f =
21 −
2
a +1−
, (5.4.31)
where a is a parameter representing the strength of the jet.
The momentum flux M in the x direction that passes through the surface of a sphere
centered at the origin is
M =
0
v
R
v
R
cos −v
sin
2R
2
sin d
=2
2
32a +1
3aa +2
−16a +1 +8aa +2 ln
a +2
a
(5.4.32)

5.4 Steady Flows When Convective Acceleration Is Present 165
and the force component for the same sphere is
F
z
=−
0
!
−p +2
v
R
R
cos −
R
R
v
R
+
1
R
v
R
sin
"
2R
2
sin d
(5.4.33)
=
2
2
24a +1 −12a
2
+24a +4 ln
a +2
a
The ratio
M +F
z
2
/
=2
32a +1
3aa +2
+8a +1 −4a +1
2
ln
a +2
a
(5.4.34)
gives a measure of the momentum strength of the jet. For values of a =1, 0.1, and 0.01,
this ratio takes on the values 34.76, 314.0, and 3,282. Whether there are other important
combinations of the three constants of integration is not resolved by this analysis.
5.4.6 Flow Due to a Rotating Disk
One of the very few similarity solutions that involve all three velocity components was
found by von Kármán in 1921 for flow caused by a large rotating disk in a stagnant
fluid. We alter our previous “general” form for the stream function by replacing U
by r, where is the angular speed of the disk. Following our form for the general
similarity solution and remembering that for axisymmetric flows the stream function
has dimensions of length cubed over time, one length dimension higher than in the
two-dimensional case, we have
=−r
rU/f =−r
2
/f (5.4.35)
where
=zU//
rU/ =z
/ (5.4.36)
(We have also altered our general solution in one other trivial detail: We have put a
minus sign in because we expect to have a downward vertical velocity component
directed toward the disk, and it is convenient to have f positive.) The appropriate form
for the swirl velocity component to accompany the stream function was found by von
Kármán to be
v
=rg (5.4.37)
Differentiation of the velocity components gives
v
r
=rf
v
=rg v
z
=−2
/f
v
r
r
=f
v
r
=g
v
z
r
=0
2
v
r
r
2
=0
2
v
r
2
=0
2
v
z
r
2
=0
v
r
z
=r
/f
v
z
=r
/g
v
z
z
=−2f
2
v
r
z
2
=
r
2
f
2
v
z
2
=
r
2
g
2
v
z
z
2
=−2
/f
166 Exact Solutions of the Navier-Stokes Equations
Substituting the preceding results into the Navier-Stokes equations in cylindrical polar
form gives
p
r
=r
2
−f
2
+2ff
+g
2
+f
0 =−2f
g +2fg
+g
p
z
=
1
p
=−2
/
2ff
+f
(5.4.38)
The no-slip boundary conditions at the wall give v
z
0 = v
r
0 = 0, or equivalently
f0 = f
0 = 0g0 =1. As becomes large (far away from the wall), the viscous
solution must approach the inviscid flow in having v
r
and v
approach zero (equivalently,
f
and g approach zero). Notice that the relative scalings of the various velocities and
the velocity and pressure gradients are as previously noticed. Since the flow is entirely
driven by the disk, the flow away from the disk is very weak. The disk is in fact acting
as a rather inefficient centrifugal pump.
Elimination of the pressure in the preceding forms results in
f
+2ff
−f
2
+g
2
=0 (5.4.39)
g
+2fg
−2gf
=0 (5.4.40)
and
p =−2f
2
+f
(5.4.41)
The numerical solution of this system of equations (5.4.39) and (5.4.40) subject
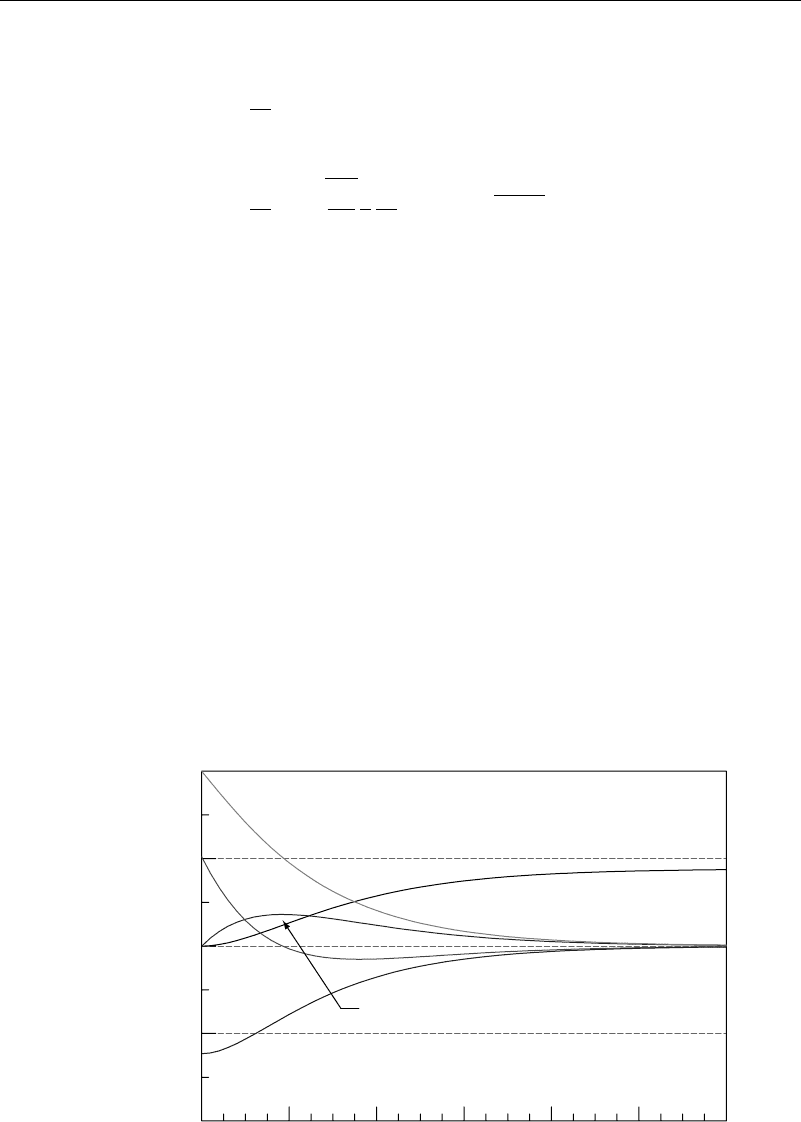
to the boundary conditions is shown in Figure 5.4.6. and Table 5.4.3 The unknown
derivatives at the wall are found to have the values g
0 =−06159 and f
0 =0510.
0.5
1.0
0.0
–0.5
–1.0
01
f
f
′
f
″
g
′
g
4563
η
2
Figure 5.4.6 Von K
´
arm
´
an’s solution for a rotating disk

5.4 Steady Flows When Convective Acceleration Is Present 167
TABLE 5.4.3 Rotating disk boundary layer (von Kármán)
f f
f
g −g
0000000 00000 05102 10000 06159
0100024 00462 04163 09386 06112
0200090 00836 03338 08780 05987
0300189 01133 02620 08190 05803
0400314 01364 01999 07621 05577
0500459 01536 01467 07076 05321
0600620 01660 01015 06557 05047
0700790 01742 00635 06067 04763
0800967 01789 00317 05605 04476
0901147 01807 00056 05171 04191
1001327 01802 −00157 04766 03911
1101506 01777 −00327 04389 03641
1201682 01737 −00461 04038 03381
1301853 01686 −00564 03712 03133
1402019 01625 −00640 03411 02898
1502178 01559 −00693 03132 02677
1602331 01487 −00728 02875 02470
1702476 01413 −00747 02638 02276
1802613 01338 −00754 02419 02095
1902743 01263 −00751 02218 01927
2002866 01188 −00739 02033 01771
2203089 01044 −00698 01708 01494
2403284 00910 −00643 01433 01258
2603454 00788 −00580 01202 01057
2803600 00678 −00517 01008 00888
3003726 00581 −00455 00845 00745
3003726 00581 −00455 00845 00745
3203834 00496 −00397 00708 00625
3403925 00422 −00343 00594 00525
3604003 00358 −00296 00498 00440
3804069 00303 −00253 00417 00369
4004125 00257 −00216 00349 00309
4204172 00217 −00184 00293 00259
4404212 00183 −00156 00245 00217
4604246 00154 −00132 00205 00182
4804274 00129 −00112 00172 00152
5004298 00109 −00095 00144 00128
Since f =04422, the flow far above the plate is v
z
=−08845
√
. The boundary
layer thickness, again constant since is independent of r, is approximately
=5
/ (5.4.42)

168 Exact Solutions of the Navier-Stokes Equations
Even though our result is for an infinite disk, we can find the moment over a finite
radius R of one side of the disk by
M =−
R
0
r
z
z =0
2r dr =−2
/g
0
R
0
r
3
dr =−05R
4
/g
0
Since −g
0 = 1935, we have for a moment coefficient
C
M
=
M
1
2
2
R
5
=
1935
R
2
/
(5.4.43)
In practice, the flow is found to be laminar for values of the Reynolds number
R
2
/ less than 10
5
, and turbulent for larger Reynolds numbers.
Problems—Chapter 5
5.1 Two fluids of different densities and viscosities are flowing down a plane with
slope . The lower layer of fluid occupies the region 0 ≤ y ≤ a and has density and
viscosity
1
and
1
, while the upper fluid occupies the region a ≤y ≤b and has density
and viscosity
2
and
2
. The lower fluid has the higher density. The surface at y =b is
open to the atmosphere. Find the pressure and velocity distributions.
5.2 A layer of viscous fluid flows down a vertical plate under the action of gravity.
The density of the fluid varies linearly from
1
at the wall to
2
<
1
at y = b, the free
surface. Find the velocity distribution and the velocity at the free surface.
5.3 Find the flow in a rotating pipe of radius a. A pressure gradient is present.
Take the flow to be axially symmetric and steady.
5.4 Verify that the velocity in a conduit with an elliptic cross-section with semimajor
and semiminor axes a and b is given by
v
x
=K
y
2
a
2
+
z
2
b
2
−1
where K =
1
2
p
x
a
2
b
2
a
2
+b
2
5.5 For flow in the conduit with an elliptic cross-section (problem 5.4), find the ratio
b/a that gives the maximum flow rate for a given pressure gradient and cross-sectional
area.
5.6 Verify that the laminar velocity in a pipe with the cross-section of an equilateral
triangular of side b is given by
v
x
=−
√
3
6b
p
x
z
z +
√
3y −
√
3b
2
z −
√
3y −
√
3b
2
5.7 Using the solution for flow between concentric rotating cylinders, find the
velocity distribution caused by a circular cylinder that is rotating in an infinite fluid.
Show that this is the same as that due to a line vortex along the z-axis. What is the
strength of this vortex?
5.8 Find the flow of a layer of viscous fluid on an oscillating plate U
0
cost at
y =0. The fluid is of constant thickness a; the surface at y = a is stress-free.
5.9 A large disk is rotated sinusoidally at a frequency in a semi-infinite fluid.
Find the steady-state fluid velocity, assuming vr z t =
0 rfz t 0
.

Problems—Chapter 5 169
5.10 A concentrated line vortex of the type discussed in Chapter 2 is suddenly
introduced into a viscous fluid. The action of viscosity is to diffuse the vorticity. The
problem to be solved for is thus the following:
t
=
2
r
2
+
1
r
r
r 0
=
#
0 for r>0
for r =0
0
2rdr =
a. Show the form of a similarity solution =
r t
that is a possible
solution for this problem.
b. Solve for the vorticity.
5.11 Show that the form r t =At
f, where =r
t
, when inserted into the
vorticity equation
t
=
2
r
2
+
1
r
r
leads to an ordinary differential equation.

Chapter 6
The Boundary Layer Approximation
6.1 Introduction to Boundary Layers 170
6.2 The Boundary Layer Equations 171
6.3 Boundary Layer Thickness 174
6.4 Falkner-Skan Solutions for Flow Past
a Wedge 175
6.4.1 Boundary Layer on a
Flat Plate 176
6.4.2 Stagnation Point Boundary
Layer Flow 178
6.4.3 General Case 178
6.5 The Integral Form of the Boundary
Layer Equations 179
6.6 Axisymmetric Laminar Jet 182
6.7 Flow Separation 183
6.8 Transformations for Nonsimilar
Boundary Layer Solutions 184
6.8.1 Falkner Transformation 185
6.8.2 von Mises Transformation 186
6.8.3 Combined Mises-Falkner
Transformation 187
6.8.4 Crocco’s Transformation 187
6.8.5 Mangler’s Transformation for
Bodies of Revolution 188
6.9 Boundary Layers in Rotating
Flows 188
Problems—Chapter 6 191
6.1 Introduction to Boundary Layers
As we saw in Chapter 5, solutions to the full Navier-Stokes equations are few in number
and difficult to obtain. In the exact solutions of the Navier-Stokes equations, it was
repeatedly seen that when a local Reynolds number was large, viscous effects are felt
mainly in the immediate vicinity of a solid boundary. In 1904 Prandtl introduced an
approximate form of the Navier-Stokes equations that holds in the thin boundary layer
near the wall, where viscous effects are comparable to the inertia effects. Here, the
relationship between the boundary layer equations and the full Navier-Stokes equations
is investigated by demonstrating how the boundary layer equations can be derived as a
limiting form of the Navier-Stokes equations.
To understand how inviscid flow theory and boundary layer theory fit with the
Navier-Stokes equations, consider the following example, first presented by Friedrichs
in 1942. His model equation is
d
2
f
dx
2
+
df
dx
=a f0 =0f1 = 1 (6.1.1)
170

6.2 The Boundary Layer Equations 171
The second-order derivative can be thought of as the viscous terms with a small viscosity
, the first derivative as a “momentum,” and the constant a as a pressure force. When the
highest-order derivative is neglected (“inviscid” approximation), the first-order equation
that remains has the solution f =ax +c c being a constant of integration. Clearly, only
one of the boundary conditions can be satisfied. Our version of relaxing the “no-slip”
condition would be to impose f1 =1, giving c =1−a. Thus, our “inviscid” solution is
f
1
x =1 +ax −1 (6.1.2)
To take care of the unsatisfied boundary condition, recall that in all of the similarity
solutions the coordinate was “stretched” by dividing by the small viscosity, giving a
thin layer at the wall. For this example, let =x/, so that our equation becomes
1
d
2
f
d
2
+
1
df
d
=a
The temptation now is to disregard the right-hand side and find
f =b +ce
−
To satisfy the boundary condition at x = 0, select c =−b,so
f
2
=b
1−e
−
(6.1.3)
To “match” the two solutions, require next that there is some region where the two
solutions overlap. In that region, write both solutions in one of the two variables (x and
), and then take a limit, the limit being either x →0 or →, depending on which
variable was chosen.
Frequently, it is simpler to choose as the variable of choice. In that case our first
solution is f
1
x =1 +a −1. To the lowest-order, b = 1−a and
f
2
=1 −a
1−e
−
×
1+ax −1 away from the wall
1−a
1−e
−
near the wall
(6.1.4)
Putting this in the context of fluid mechanics, to the lowest-order approximation
as the Reynolds number becomes large
=1/Re → 0
, a good approximation to the
solution of the Navier-Stokes equations is to solve the Euler equations for the slip
velocity on the boundary and use Prandtl’s boundary layer approximation for the flow
near the wall.
Other forms of boundary layers exist as well. As we will see in Chapter 8, slow
flows at low Reynolds numbers also require similar handling. Boundary layers can also
exist as shear layers in the interior of a region—for example, the Gulf Stream where
it departs the U.S. coast and crosses the Atlantic Ocean. Other areas of physics exhibit
similar phenomena, such as the behavior of the free sides of a thin elastic plate when
it is bent and current flowing in a solid wire at high frequencies. For more detailed
explanations of the matching process, see Lagerstrom and Cole (1955), Goldstein (1960),
and Van Dyke (1964).
6.2 The Boundary Layer Equations
In all of our exact solutions of the Navier-Stokes equations it was seen that the pressure
gradient along a wall was of greater magnitude than that of the pressure gradient

172 The Boundary Layer Approximation
perpendicular to the wall, and that the viscous terms involving second derivatives
along the wall were smaller than those involving derivatives taken perpendicular to
the wall. The continuity equation was always satisfied in full, whereas the momentum
equation in the direction perpendicular to the wall introduced only very low orders of
magnitude terms.
To help in deriving the boundary layer equations, scale the various terms in the
Navier-Stokes equations so that these orders of magnitude hold true, particularly when
the Reynolds number is large. As a bookkeeping scheme it is convenient to build these
notions as to the orders of magnitude of the various terms into our dimensionaliza-
tion so that these orderings appear automatically. To do this, introduce dimensionless
coordinates
x = x
D
/L y = y
D
√
Re/L t =t
D
/T (6.2.1)
with the Reynolds and Strouhal numbers given by
Re = U
D
L/ and St =L/U
D
T (6.2.2)
Here, x
D
y
D
are the dimensional lengths along and perpendicular to a wall, U
D
is a
representative constant body or stream velocity, T is a time scale for unsteady effects,
and L is a body length along the wall. The existence of constant L and U
D
, and hence
of a constant Re, is vital to what follows, as will be discussed later.
Since changes along the body occur on a length scale L, whereas those perpendicular
to the body occur over a distance of the order of the (thin) thickness of the boundary
layer L/
√
Re, the biased dimensionalization introduced in equation (6.2.1) “stretches”
the thin y
D
by multiplying it by
√
Re to make the partial derivatives reflect their true
orders of magnitude.
Introducing a dimensionless stream function (as done in the similarity solutions of
the full Navier-Stokes equations) by
=
D
/
√
Re (6.2.3)
gives dimensionless velocities
v
x
=
y
=
1
U
D
y
=
u
D
U
D
(6.2.4)
v
y
=−
x
=−
√
Re
U
D
x
=
√
Re
v
D
U
D
(6.2.5)
These dimensionless velocities bring out the physical fact that, at the outer edge of the
boundary layer u
D
will be of magnitude U
D
, and v
D
will be of the (small) magnitude
U
D
/
√
Re. Our stretching of the coordinates, then, along with the nondimensionalization
of , has stretched the velocity components appropriately as well.
Making pressure dimensionless by
p = p
D
/U
2
D
(6.2.6)
the dimensionless Navier-Stokes equations for incompressible flow with constant density
and viscosity in two-dimensional Cartesian coordinates become
St
v
x
t
+v
x
v
x
x
+v
y
v
x
y
=−
p
x
+
2
v
x
y
2
+
1
Re
2
v
x
x
2
(6.2.7)
6.2 The Boundary Layer Equations 173
and
St
v
y
t
+v
x
v
y
x
+v
y
v
y
y
=−Re
p
x
+
2
v
y
y
2
+
1
Re
2
v
y
x
2
(6.2.8)
For large values of the Reynolds number, the limit of equations (6.2.7) and (6.2.8) is
St
v
x
t
+v
x
v
x
x
+v
y
v
x
y
=−
p
x
+
2
v
x
y
2
(6.2.9)
and
0 =−
p
y
(6.2.10)
Equation (6.2.10) states that the pressure gradient across the thin boundary layer is
negligible, and thus the pressure gradient at the outer edge of the boundary layer, as
obtained from the Euler equations, can be used. Since the inviscid velocity component
perpendicular to a wall vanishes, this means that the Euler equations at the wall are
−
p
x
=St
U
t
+U
U
x
(6.2.11)
where U is the velocity along the wall (made dimensionless by U
D
) as predicted from
inviscid theory. Thus, in equation (6.2.9) the pressure gradient is known, and, since v is
related to u through continuity, there is only one equation in one unknown to be solved.
The appropriate boundary conditions are the no-slip conditions at the wall,
v
x
=v
y
=0aty = 0u=v =0aty = 0 (6.2.12)
and a joining to the inviscid velocity—that is,
v
x
→U (6.2.13)
at the outer edge of the boundary layer.
Having developed the boundary layer equations in dimensionless form, usually it
is more convenient when doing problems to start with the dimensional form. This is, in
two dimensions with v = u v 0,
u
t
+u
u
x
+v
u
y
=
U
t
+U
U
x
+
2
u
y
2
(6.2.14)
p
y
=0 (6.2.15)
and
u
x
+
v
y
=0 (6.2.16)
with u =v =0 on the wall and u approaches U at the outer edge of the boundary layer.
Mathematically, the outer edge of the boundary layer can be defined as being at
an infinite value of dimensionless y, since in the stretching of the y coordinate the
Reynolds number has been made large. In practice, a value for dimensionless y of five
or so can often be regarded as infinity for practical purposes, since, as has been seen in
the previous similarity solutions, u will not vary much for values of y beyond that point.
Our boundary layer equations have been derived for a flat boundary. For curved
boundaries, providing x is regarded as being locally tangent to the boundary and y as
