Georgi, Howard. Physics 16 - Mechanics and Special Relativity (англ.)

reﬂect this axis in the symmetry plane, we must get another principal axis with the same principal

moment, as illustrated below:

symmetry

plane

.

putative principal axis

.

reﬂected principal axis

(18)

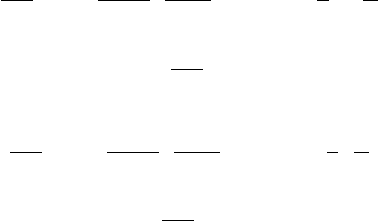
Now there are two possibilities. One possibility is that the reﬂected axis coincides with the original.

The only way this can happen is for the original axis to be perpendicular to the symmetry plane,

in which case the theorem is true. The other possibility is that the reﬂected axis is different.

But then there are two distinct axes with the same principal moment (because they are physically

equivalent due to the symmetry). But then any axis in the plane formed by these two is a perfectly

good principal axis — in particular the one perpendicular to the symmetry plane — so again the

theorem is true.

8

Finishing the impulse problem

Let’s review where we are with the light rigid rectangular frame with masses at the corners, as

shown:

....................................................

.

..

.

y

x

..

.

..

.

..

.

..

.

..

.

..

.

..

m

.

.......................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................

×

~

F

j = 1

(`/2, `

0

/2, 0)

j = 2

(`/2, −`

0

/2, 0)

j = 4

(−`/2, `

0

/2, 0)

j = 3

(−`/2, −`

0

/2, 0)

(19)

where the force acts for a very short time, and the impulse is into the paper, with the form

Z

dt

~

F = −ˆz P (20)

for positive P . Earlier, we found the center of mass to be at the origin and we found the moment

of inertia tensor for this object about its center of mass to be

~

I

xx

= m `

0

2

~

I

yy

= m `

2

~

I

zz

=

~

I

xx

+

~

I

yy

~

I

xy

=

~

I

xz

=

~

I

yz

= 0 (21)

To complete the story, we need to compute the velocity of the center of mass, and the angular

velocity about the center of mass. The velocity is easy. We know the impulse, and we know the

total mass, so we can compute the change in momentum and thus the ﬁnal velocity. We’ll do this

in a minute. First we will do the interesting part and compute the angular velocity.

To do this, we ﬁrst compute the impulsive torque about the center of mass — that is

Z

dt ~τ (22)

over the short period during which the force acts. This is

Z

dt (~r

2

−

~

R) ×

~

F = (`/2, −`

0

/2, 0) × (0, 0, −P ) = (P `

0

/2, P `/2, 0) (23)

where we have used the fact that the force acts for a very short time, so that ~r

2

and

~

R do not change

signiﬁcantly while the force acts, and they are therefore effectively constants and can be taken

9

outside the integral in (23). This is equal to the change in angular momentum about the center of

mass, which is the ﬁnal angular momentum about the center of mass.

~

L = (P `

0

/2, P `/2, 0) (24)

But the angular momentum is related to the angular velocity by

~

L =

~

I · ~ω (25)

Because

~

L is conserved after the force acts, (25) remains true for any time afterwards. But here

is the point. Immediately after the force acts, the body has still not had a chance to move from its

position in (19). Therefore, the moment of inertial tensor is given by (21), which implies (using

(21) and (24))

(P `

0

/2, P `/2, 0) = (m`

0

2

ω

x

, m`

2

ω

y

,

~

I

zz

ω

z

) (26)

which in turn implies

ω

x

=

P

2m`

0

ω

y

=

P

2m`

ω

z

= 0 (27)

This is the desired result for the angular velocity about the center of mass immediately after the

hammer blow:

~ω =

Ã

P

2m `

0

,

P

2m `

, 0

!

(28)

The directions of the vectors

~

L and ~ω are shown below (in an arbitrary relative normalization,

because they have different units).

.

..

.

..

.

~

L

~ω

.

..

.

..

.

..

.

..

.

..

.

..

.

m

.

.......................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................

×

~

F

j = 1

(`/2, `

0

/2, 0)

j = 2

(`/2, −`

0

/2, 0)

j = 4

(−`/2, `

0

/2, 0)

j = 3

(−`/2, −`

0

/2, 0)

(29)

It is amusing (though not particularly signﬁcant) that ~ω points at mass 1.

10

(28) is very easy to understand in the limit ` = `

0

. In this case, the frame is a square, the x

and y principal moments are equal, so we can take the principal axes to be the diagonals, and (28)

simply describes rotation about the obvious diagonal, shown as the dashed line below:

....................................................

.

..

.

y

x

..

.

..

.

..

.

..

.

..

.

..

.

..

m

.

...................................................................................................................................................................................................................................................................................................................................................................................................................................................................

.

×

~

F

(30)

The ﬁnal velocities

Now to get the velocities of the individual masses, we use the fact that the motion of the body is a

combination of the translational motion of the center of mass plus the rotational motion about the

center of mass. The ﬁnal velocity of the center of mass is the impulse divided by the total mass:

~

V =

Ã

0, 0, −

P

4m

!

(31)

The velocity of mass j is then given by

~

V + ~ω × (~r

j

−

~

R) (32)

where ~ω is given by (28). Here they are:

~v

1

=

Ã

0, 0, −

P

4m

!

+

Ã

P

2m `

0

,

P

2m `

, 0

!

×

Ã

`

2

,

`

0

2

, 0

!

=

Ã

0, 0, −

P

4m

!

(33)

~v

2

=

Ã

0, 0, −

P

4m

!

+

Ã

P

2m `

0

,

P

2m `

, 0

!

×

Ã

`

2

, −

`

0

2

, 0

!

=

Ã

0, 0, −

3P

4m

!

(34)

11

~v

3

=

Ã

0, 0, −

P

4m

!

+

Ã

P

2m `

0

,

P

2m `

, 0

!

×

Ã

−

`

2

, −

`

0

2

, 0

!

=

Ã

0, 0, −

P

4m

!

(35)

~v

4

=

Ã

0, 0, −

P

4m

!

+

Ã

P

2m `

0

,

P

2m `

, 0

!

×

Ã

−

`

2

,

`

0

2

, 0

!

=

Ã

0, 0,

P

4m

!

(36)

12

lecture 20

Topics:

Where are we now

The free symmetric top in the space frame

Precession of tops

Nutation

Vectors in the body frame

Where are we now

The next couple of lectures are about as hard as it gets in this course. Now we are ﬁnally going

to face the music and try to ﬁgure out what happens when the angular momentum and the angular

velocity are not in the same direction. However, I am not going to be so cruel as to try to completely

solve the problem in the case of a general rigid body. We will analyze completely only the case

of the free precession of a symmetric top, with two equal moments of inertia. It not just that I am

taking pity on you. I think that it is possible to understand this in a way that is probably impossible

for the general case. Since I am interested in getting you to really understand things, rather than

just cramming facts into your head, I will focus on the simpler problem.

The animation FREETOPE.EXE shows the complicated motion of a symmetric top rotating

in space when the angular momentum is not aligned with a principal axis. Today, we are going

to understand this. I could teach you in a few minutes the formulas that describe the motion of

the free symmetric top in the space frame. But my personal view is that physics is more than a

sterile collection of equations and I don’t ﬁnd complicated collections of equations very satisfying.

Instead, I am going to spend most of this lecture on it. Our derivation will be slick, and I hope that

you will ﬁnd it so simple that you can easily reproduce it yourself, though you may ﬁnd yourself

wondering what happened. Beginning at the end of today’s lecture and ﬁnishing next time, we will

approach the problem again in a more mathematical way. I am hoping that seeing both of them

and some animations will give you some real intuition into what is going on.

The free symmetric top in the space frame

Suppose that a rigid body with two equal principal moments (this makes it a symmetric top) is

ﬂoating out in space, and is rotating freely about its center of mass. Because there are no external

torques on the system, the angular momentum

~

L is conserved. If

~

L points along a principal axis

of the body, then the angular velocity vector ~ω is parallel to

~

L and it also remains constant. The

interesting mathematical question is what happens to ~ω when it is not parallel to

~

L. The physical

question is then what the resulting motion looks like!

You should not be surprised that the key is the connection between the angular momentum and

the angular velocity through the moment of inertia tensor —

~

L =

~

I · ~ω (1)

1

The important point is that in the space frame, the principal axes of the top, ˆe

1

, ˆe

2

and ˆe

3

, are all

moving around as the body rotates, but at any given time, they form a complete orthonormal set of

vectors in terms of which we can expand any vector. For example

~ω = ˆe

1

(ˆe

1

· ~ω) + ˆe

2

(ˆe

2

· ~ω) + ˆe

3

(ˆe

3

· ~ω) = ω

1

ˆe

1

+ ω

2

ˆe

2

+ ω

3

ˆe

3

(2)

which just says that ~ω can be expanded in the basis vectors, ˆe

j

. Using (2) in this basis, (1) becomes

~

L = I

1

ω

1

ˆe

1

+ I

2

ω

2

ˆe

2

+ I

3

ω

3

ˆe

3

(3)

The relation (3) is entirely general, valid for an arbitrary moment of inertia, but here, we are dealing

with a symmetric top, for which two of the principle moments are equal. Let’s assume that the two

equal moments are I

1

and I

2

, with corresponding principle axes ˆe

1

and ˆe

2

. As you know from

the equal-moment theorem, this means that any axis in the plane of ˆe

1

and ˆe

2

(which is the plane

perpendicular to ˆe

3

) is a principle axis with the same principle moment. To remind you of this, I

will deﬁne I

⊥

as

I

⊥

= I

1

= I

2

(4)

Then we can write (3) as

~

L = I

⊥

ω

1

ˆe

1

+ I

⊥

ω

2

ˆe

2

+ I

3

ω

3

ˆe

3

(5)

Now because of (4), we can eliminate all reference to ˆe

1

and ˆe

2

which enormously simpliﬁes the

problem. If we now multiply both sides of (2) by I

⊥

and subtract both sides of the result from (5),

we get

~

L = I

⊥

ω

1

ˆe

1

+ I

⊥

ω

2

ˆe

2

+ I

3

ω

3

ˆe

3

− [ I

⊥

~ω = I

⊥

ω

1

ˆe

1

+ I

⊥

ω

2

ˆe

2

+ I

⊥

ω

3

ˆe

3

]

~

L − I

⊥

~ω = (I

3

− I

⊥

) ω

3

ˆe

3

(6)

or

~

L = I

⊥

~ω + (I

3

− I

⊥

) ω

3

ˆe

3

= I

⊥

~ω + I

⊥

Ω ˆe

3

(7)

where

Ω =

(I

3

− I

⊥

) ω

3

I

⊥

(8)

There is an ambiguity in the sign of ˆe

3

because the symmetry axis is not directional, and we resolve

this by always taking ω

3

> 0. The constantΩ has units of angular velocity, and it always satisﬁes

the inequality

−ω

3

≤ Ω ≤ ω

3

(9)

because

−I

⊥

≤ I

3

− I

⊥

≤ I

⊥

(10)

We will talk more about this in a general way soon, and you will explore this inequality quantita-

tively on the problem set .

2

It will sometimes be convenient to rewrite (7) as an equation for ω,

~ω =

~

L/I

⊥

− Ωˆe

3

(11)

The relations (7) and (11) have nothing in them beyond the connection between angular mo-

mentum and angular velocity and the fact that I

1

= I

2

= I

⊥

. They are true at any time, but do not

tell us directly about time dependence. However, it is the simplicity of these relations that allows

us to solve this problem much more easily than we could for a general rigid body. Mathematically,

it is the equality of I

1

and I

2

that allows us to eliminate both ˆe

1

and ˆe

2

from (7) and (11). Physi-

cally, this is particularly important because the one remaining principal axis in (7) and (11) is the

one we see in the typical symmetric top. The symmetry axis is special, and that makes (7) and (11)

particularly useful.

We can now get the time dependence by judiciously using the connection between rotation and

the cross product.

The ﬁrst step is to notice that ˆe

3

is a principal axis ﬁxed in the body, and it is therefore rotating

with the body. Like any other vector ﬁxed in the body, ˆe

3

has a time dependence given the the

cross product with the angular velocity vector ~ω.

d

dt

ˆe

3

= ~ω × ˆe

3

(12)

The relation (12) is a differential equation for the time dependence of ˆe

3

, but it is not particular

useful, because ~ω is also changing with time in a way that we do not yet know. Instead of trying to

bull our way through this, we can use (11) to write

d

dt

ˆe

3

=

³

~

L/I

⊥

− Ωˆe

3

´

× ˆe

3

=

~

L

I

⊥

× ˆe

3

(13)

This is a much more useful differential equation, because

~

L is constant. In fact, we don’t even have

to solve it, because we already know exactly what it means geometrically. The time dependence of

ˆe

3

given in (13) is what we expect if ˆe

3

is rotating about an axis in the direction of

~

L with angular

velocity

~

L/I

⊥

. Since

~

L is constant in the space frame, this must be what is actually going on for

all times — ˆe

3

is undergoing uniform circular motion with angular velocity

~

L/I

⊥

. Now we are

essentially done. From this, we can calculate everything.

First notice that the component of the angular momentum in the ˆe

3

direction (along the symme-

try axis of the top),

~

L · ˆe

3

is constant. Geometrically, this is because ˆe

3

is rotating around the ﬁxed

vector

~

L, so while its component perpendicular to

~

L is constantly changing, its parallel component

is not. Analytically it follows from (13) as follows:

d

dt

³

~

L · ˆe

3

´

=

~

L ·

d

dt

ˆe

3

=

~

L ·

Ã

~

L

I

⊥

× ˆe

3

!

= 0 (14)

But

~

L · ˆe

3

is related to ω

3

by (5),

~

L · ˆe

3

= I

3

ω

3

(15)

3

Thus (14) means that ω

3

is constant. This in turn means that Ω is constant also, so the coefﬁcients

in (7) and (11) are constant in time. Note also that using (15), Ω can be written as

Ω =

|

~

L |

I

⊥

I

3

− I

⊥

I

3

cos θ (16)

where θ is the angle between

~

L and the symmetry axis (between 0 and π/2).

Because of (11), we know that ~ω is a ﬁxed linear combination of

~

L and ˆe

3

, and therefore since

ˆe

3

is undergoing uniform circular motion with angular velocity

~

L/I

⊥

, then ~ω is also. We can see

this geometrically because (7) or (11) and (14) imply that

~

L, ˆe

3

and ~ω are ﬁxed in a plane and then

from (13) we can conclude that the plane formed by

~

L, ˆe

3

and ~ω rotates about the ﬁxed angular

momentum with angular velocity

~

L/I

⊥

. We can also see this analytically

d

dt

~ω (17)

=

d

dt

³

~

L/I

⊥

− Ωˆe

3

´

(18)

=

d

dt

³

−Ωˆe

3

´

(19)

=

~

L

I

⊥

×

³

−Ωˆe

3

´

(20)

=

~

L

I

⊥

×

³

~

L/I

⊥

− Ωˆe

3

´

(21)

=

~

L

I

⊥

× ~ω (22)

Notice that we have still not ﬁgured out explicitly what the perpendicular axes ˆe

1

and ˆe

2

are

doing. But this information is implicit in the relation (11). In words, this relation together with

(13) says that the angular velocity of the top can be taken apart into two components:

1. the rotation of the top around its symmetry axis ˆe

3

with angular velocity −Ω ˆe

3

; and

2. the rotation of ˆe

3

around the ﬁxed angular momentum with angular velocity

~

L/I

⊥

.

Let’s review the few key steps in this derivation. First we used the form of the moment of

inertia tensor to eliminate

Let us now look at the result in animated form in the program FREETOPE. This is an animation

of a rotating rectangular solid, with two opposite sides square, so that it has two equal principal

moments. This is an allowed free rotation of the body in space. It is best to start with I

3

/I

⊥

less

than one, corresponding to a prolate object — long and thin along the symmetry axis. Start (after

getting the aspect ratio and the speed to your liking) with I

3

/I

⊥

= 0.5 and an initial angle of 1.57

radians — that is about π/2. Then (15) implies ω

3

= 0. This makes physical sense, because if the

symmetry axis is perpendicular to the angular momentum, then the angular momentum is in the

direction of one the other principal axes, and the angular velocity and angular momentum are in

4

the same direction. So this describes the rotation of the body about one of the principal axes with

principal moment I

⊥

.

Then work your way towards smaller initial angles. What you see once you get away from

angle 0 or π/2 is three lines representing vectors in addition to to rotating solid. The green line is

the angular velocity vector, ~ω. The vertical purple line and the red line are the components of the

~ω vector along the directions of the angular momentum

~

L and the symmetry axis ˆe

3

. Thus these

vectors are a visual representation of the fundamental relation between

~

L, ~ω and ˆe

3

, expressed in

(7) and (11).

I hope that these animations will help you to understand more deeply the connection between

(11) and (13). The rate of rotation of the plane is always |

~

L|/I

⊥

, which is ﬁxed in the animations.

One way of describing the crucial relation (11) is that we have taken the angular velocity vector

apart into a component along ˆe

3

that describes the rotation of the object around its symmetry

axis and the vertical component

~

L/I

⊥

that describes the rotation of the plane of the symmetry

axis. Writing ~ω this way is great, because you should be able to see both components physically.

Notice that for the prolate object, for an initial angle less than π/2, ~ω is longer than

~

L/I. This

is prolateness in action - the moment of inertial about the long axis is smaller than I

⊥

, so (13)

implies that the magnitude of the angular momentum must be larger than |

~

L|/I

⊥

. The spin around

the axis and the spin around the axis of the angular momentum add positively to the total angular

velocity. For an oblate object, the opposite is true. The angular velocity vector is shorter than

|

~

L|/I

⊥

, which means that the spin around the axis is in the opposite direction. But in this case

as well, the plane of ˆe

3

and ω rotates about the ﬁxed angular momentum, which has the physical

effect that the symmetry axis precesses. It is worth staring at this animation for a long while.

Precession of tops

The precession of the symmetry axis about the direction of the angular momentum is the basic

physics behind the phenomenon of nutation in precessing tops. As you probably know, a spinning

top subject to a gravitational torque will precess. Let’s see how this works. The system is illustrated

5

Georgi, Howard. Physics 16 - Mechanics and Special Relativity (англ.)

Подождите немного. Документ загружается.