Galaktionov V.A., Svirshchevskii S.R. Exact Solutions and Invariant Subspaces of Nonlinear Partial Differential Equations in Mechanics and Physics

Подождите немного. Документ загружается.

7 Partially Invariant Subspaces and GSV 353

Assume that



≡ constant (7.81)

(exponential f (x) = e

µx

will be discussed last). Then, (7.80) is valid iff

[(α +2β)σ − c

](β + 2α) = 0,

εσ − c

[(α +2β)σ − c

] = 0,

[(α +2β)σ − c

]

γ −A

= σ A.

(7.82)

We may suppose that (α + 2β)σ − c

= 0. (If not, this yields c

= c

= δ =

0andg(x) ≡ 0, and hence, u(x , t) = f (x)a(t) is a simple solution in separate

variables.) Hence, from the ﬁrst condition in (7.82), we obtain (7.64), while the rest

of conditions are equivalent to the last two hypotheses in (7.64). Here it is assumed

that 2ασ + c

= 0; see comment below.

Finally, we need to determine the functions f (x) and g(x). Equation (7.70) with

β =−2α is equivalent to (cf. (7.79))















−



A−γ

that can easily be integrated. This yields the functions f (x) in (7.66), (7.68), and

(7.69), and g(x) follows from (7.77). This completes the proof.

Concerning exponential functions, if (7.81) is not valid and

f (x) = e

µx

, with a µ = 0,

it follows from (7.75) and (7.77) that there exists the solution

u(x , t) =

+ D

µx

a(t),

[(α +2β)σ − c

]µ

+ (εσ − c

)µ − σ A = 0,

(α +β)µ

+ εµ + γ − A = 0.

Here α, β,andA are arbitrary constants.

If 2ασ + c

= 0 (c

= 0), it follows from (7.82) and (7.62) that explicit solutions

exist in the case where

β =−2α = 0,γ= c

= δ = 0



σ =



Here A = 0 is arbitrary. It follows from (7.70) that Y =

solves a linear second-

order ODE, αY



+ εY



+ AY = 0, and (7.77) yields

g =



εc

β A

2. Case A = 0. Solutions u(x , t) ∈ M

with A = 0 have another form.

Theorem 7.16

Assume that

(7.53)

with

A = B = 0

holds. Then, there exists the

nontrivial solution

(7.65)

in the following cases:

(i)α+ 2β = 0,β= 0, c

= 0,δ=

(α+β)c

(α+2β)

, c

εc

α+2β

, (7.83)

provided that µ satisﬁes the following two equations (cf. (7.80) and (7.70)):

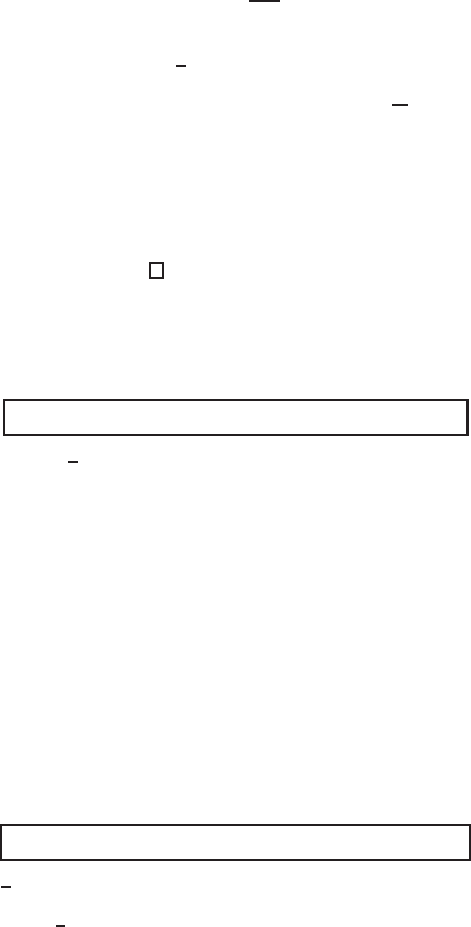
354 Exact Solutions and Invariant Subspaces

with functions

Y = ln| f |

and

given by

αY



+ (α + β)(Y



)

+ εY



+ γ = 0,

g(x) =−σ f (x)



f (x)

+ Df(x);

(7.84)

(ii)α=−2β, β = 0, c

= εσ, c

= 0,δ= 0,

Y = ln| f |

solves the ODE

αY





)

+εY



+γ = 0,

and

is deﬁned by

(7.84)

;

(iii)β= 0,α= 0, c

= αδ = 0, c

εδ

and

f (x)

satisﬁes the linear ODE

α f



+ ε f



+ γ f = 0, g

being given by

(7.84)

Proof. Setting A = 0 in (7.77), and assuming that (7.81) holds, we ﬁnd that a

nontrivial solution exists iff c

= (α + 2β)σ and c

= εσ, where σ is given by

(7.62). A simple analysis of this algebraic system for the parametersand (7.62)leads

to the above conclusions. ODEs for f (x ) follow from (7.70) with A = 0, while

(7.84) is equivalent to (7.75).

Let us now present a more detailed analysis of such solutions of particular PDEs.

Example 7.17 (Quadratic parabolic PDE) We begin with a parabolic model and

ﬁrst use Theorem 7.15. In view of (7.64), without loss of generality, set α = 1and

β =−2. The equation (7.50), (7.61) with P(D

) = 0 reads

= uu

− 2(u

)

+ γ u

+ εuu

+ c

u + c

+ δ. (7.85)

Transformation v =

gives a semilinear heat equation from combustion theory,

= v

+ (ε + c

v)v

− (γ v + c

+ δv

). (7.86)

It follows from (7.53) that a(t) ≡ 0 satisﬁes



= Aa ⇒ a(t) = e

(A = 0).

Then T [a, 1] ≡ a



= Aa, and, therefore, (7.63) is valid. It follows from Theorem

7.15 that, under the last two hypothesesin (7.64),equation(7.85) possesses solutions

u(x , t) = g(x ) + f (x )e

, (7.87)

where the coefﬁcients f and g are given in (7.66), (7.68), or (7.69). These solutions

coincide with soliton-type solutions of a similar PDE constructed by Kawahara and

Tanaka [329] and Carriello and Tabor [100] by Baker–Hirota-type techniques and

related Penlev´e-type analysis.

Example 7.18 (Hyperbolic equation) Consider now a quadratic hyperbolic PDE,

= uu

− 2(u

)

+ γ u

+ εuu

+ c

u + c

+ δ. (7.88)

Setting v =

yields

−

)

= v

+ (ε + c

v)v

− (γ v + c

+ δv

It follows from (7.53) that a(t) ≡ constant satisﬁes



= Aa. (7.89)

7 Partially Invariant Subspaces and GSV 355

Since, in this case, T [a, 1] = a



, we have by (7.89) that (7.63) holds. By Theorem

7.15, the type of the explicit solution (7.65) depends on the sign of the parameter A

given in (7.64):

A =

γ(3σ +c

)

2σ +c

, so that

u(x , t) = g(x ) + f (x )



√

+ D

−

√



for A > 0,

u(x , t) = g(x ) + f (x )



sin



|A|



+ D

cos



|A|



for A < 0.

If A = 0, by Theorem 7.16, we study the general PDE

= αuu

+ β(u

)

+ γ u

+ εuu

+ c

u + c

+ δ.

It follows from (7.89) with A = 0thata(t) = t, and hence,

u(x , t) = g(x ) + f (x) t ∈ M

Then, in the case of (7.83), the ODE for f in (7.84) can easily be integrated. For

instance, in the simplest case α + β = 0(whereδ = 0), we ﬁnd

f (x) = exp



−εx /α

−

x + D



For arbitrary D

and D

, the integral in (7.84) cannot be calculated explicitly.

Example 7.19 Consider the operator

T [u] = u(u

+ 2ωu



+ 2ωa



= Aa,

and, in particular,

a(t) = t e

−ωt

, if A =−ω

potheses (7.64) with A =−ω

, there exists a solution of the problem (7.59),

u(x , t) = g(x ) + f (x ) t e

−ωt

where g(x ) and f (x ) are given by (7.66), (7.68), or (7.69).

7.4.3 On quasilinear hyperbolic PDEs for A = B

In what follows, unlike Theorems 7.15 and 7.16, assume that

A = B. (7.90)

This condition is valid for the following hyperbolic PDE with the left-hand side of

the KFG equation (6.27):

= αuu

+ β(u

)

+ γ u

+ εuu

+ c

u + c

+ δ, (7.91)

which will be used in future illustrations of the main results. Then B = 0. Suppose

that α = 0.

As in Theorem 7.15, consider a general equation. It follows from (7.54), (7.60),

and (7.90) that functions g and f satisfy (7.70), (7.71) and

α(gf



+ fg



) + 2βg



+ ε(gf)



+ c

f +c



− (B − 2γ)gf = 0. (7.92)

where ω = 0. Then, the ODE for a(t) (see (7.53)) has the form a

One can see that (7.63) is valid. It then follows from Theorem 7.15 that, under hy-

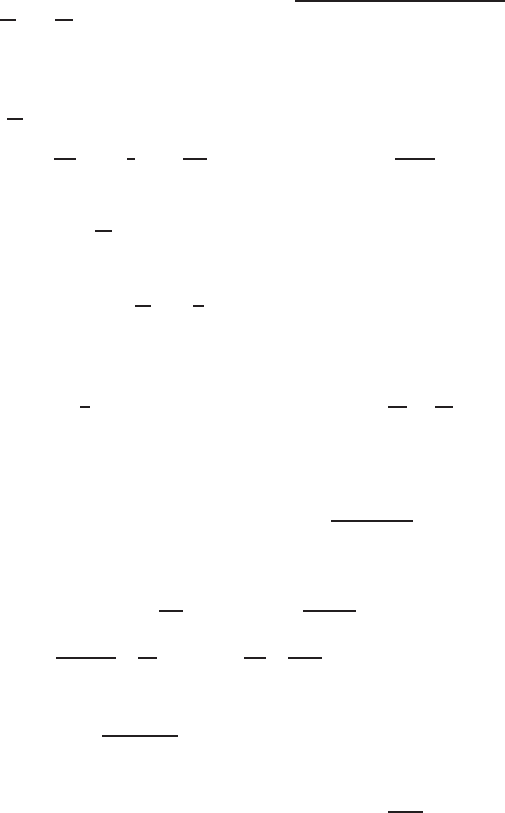
356 Exact Solutions and Invariant Subspaces

The only difference with (7.72) is the last term, where B = A. In this case, using the

β(gf



− fg



)

= c

f (gf



− fg



) − [δ − ( A − B)g

] f

. (7.93)

Hence, instead of (7.75), for β = 0,



g −

2β

R), where

R =±



− 4βδ + 4β(A − B)g

. (7.94)

Let us next derive a condition of solvability of the equation on M

. Substituting



and g



from (7.94) into (7.71) and using (7.70) yields



≡ z =



(A − B)αc

g + [A(α + 2β) − B(α + β)]g



β −

εc



R −



αc

R + 2(A − B)αβg

α+2β



−1

(7.95)

where the expression in the last square bracket is assumed to be non-zero. Let







(x ) ≡ constant and g(x ) ≡ constant. (7.96)

It follows from (7.70) that



≡ (



)



[A − γ − (α + β)z

− εz]. (7.97)

By substituting into (7.94) g



= g



with g

and z from (7.95), we arrive at the

following solvability criterion:

zg −

[A − γ − (α + β)z

− εz](z



)

−1

≡

2β

R, (7.98)

which, together with (7.95), must be the identity for all suitable F ∈ IR .

1. Existence for the rational case. Let us begin with a simple case where the irra-

tional function in (7.94) does not appear, i.e.,

= 4βδ and

R = σ g,σ=±2



β(A − B), β = 0. (7.99)

Then (7.95) is equivalent to

g =



αc

2β

z + n



−

σ(α+β)



−1

, (7.100)

where n

= A +

(α+β)

4β

−

εσ

2β

and n

εc

2β

−

ασc

4β

−c

. Plugging(7.100)into (7.98)

must yield the identity. One can verify that it is true iff a certain cubic polynomial is

trivial,



αc

σ(α+β)

2β



+ ...≡ 0forallz ∈ IR .

Finally, we obtain from (7.95) that the identity holds, if

α + β = c

= c

= δ = 0andε

β A

A−B

. (7.101)

These are the existence conditions of a nontrivial solution u(x, t) on M

((7.101)

corresponds to the case for which both square brackets in (7.100) vanish).

Proposition 7.20

Under hypotheses

(7.90)

and

(7.101)

,system

(7.70)

(7.71)

,and

(7.92)

admits a nontrivial solution

{g, f }

same technique as in the proof of Theorem 7.15, we derive that (cf. (7.74))

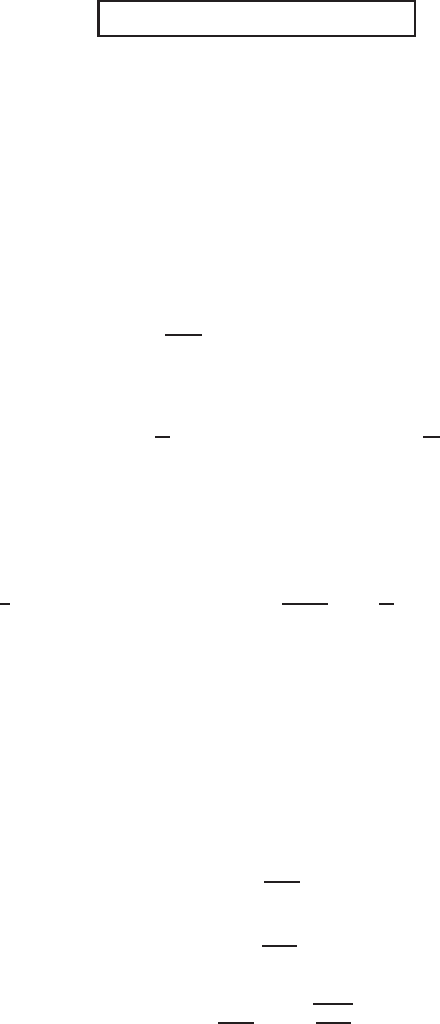
7 Partially Invariant Subspaces and GSV 357

Example 7.21 If (7.101) holds, equation (7.91) with α =−β = 1hastheform

= uu

− (u

)

+ γ u

+ εuu

. (7.102)

Transformation v = ln|u| yields



+ (v

)



= v

+ γ + εv

By Proposition 7.20, there exist solutions (7.65), where a(t) satisﬁes

T [a] ≡ a





= Aa

, with A =−ε

= 0. (7.103)

Recall that B = 0. In particular, the simplest function is a(t) = e

−ε

2/3

.Itthen

follows from (7.70) that Y = ln | f |,andg(x ) are given by



+ εY



+ (γ + ε

) = 0andg



= Y



± ε; (7.104)

u(x , t) = D exp



−

+γ

x + D

−εx



εx

+ a(t)



. (7.105)

2. Linear Case: β = 0. From (7.94), we get two subcases:

2.1. Nonexistence with c

= 0. Then, instead of (7.94),



= zg +

[(A − B)g

− δ], with z =



z =−



2(A − B)

αg

+ ε(A − B)c

+ (2A − B)c

−2(A − B)αδg + c

− εδc





3(A − B)αg

− δα + c



−1

(7.106)

zg −



A − γ − αz

− εz





)

−1

≡−

(A−B)

for g ∈ IR . (7.107)

Substituting z(g) and z



(g) from (7.106), we infer that (7.107) is the identity if a

certain eighth-order polynomial satisﬁes

(A − B)

+ ... ≡ 0forallg ∈ IR .

Since α = 0 by the assumption, a nontrivial solution exists in the case of A = B

only, which has been studied before.

2.2. Existence. Suppose now that

β = c

= 0. (7.108)

Then (7.93) implies

≡ g

A−B

> 0. (7.109)

In this case, functions {g

, f } solve the following system:

α f



+ ε f



+ (γ − A) f = 0, g

A−B

,γg

+ c

+ δ = 0. (7.110)

It is easily seen that such a constant solution g

of (7.110) exists if

≥ 4γδ and

γδ

A−B

± c



A−B

+ δ = 0. (7.111)

see (7.94) and (7.99). This yields the following solution:

Therefore, we derive similarly that (cf. (7.95))

Finally, we obtain the following solvability criterion (cf. (7.98)):

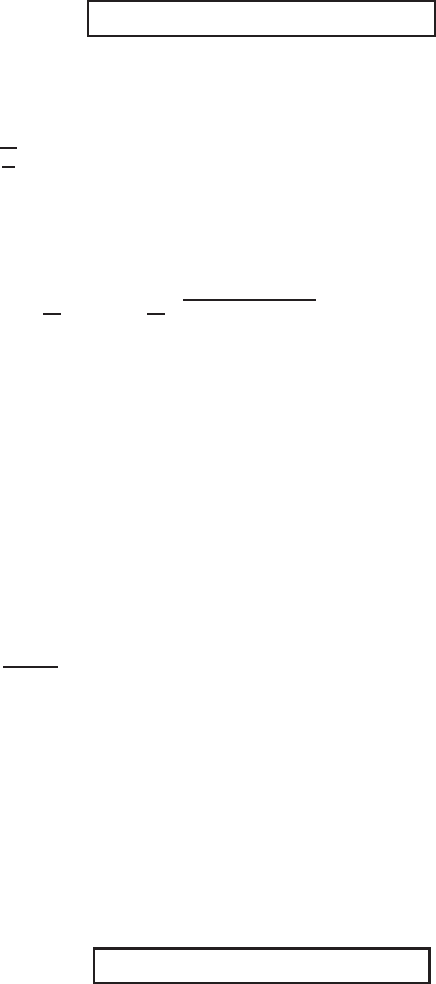
358 Exact Solutions and Invariant Subspaces

Proposition 7.22

Under hypotheses

(7.90)

(7.108)

(7.109)

,and

(7.111)

,system

(7.70)

(7.71)

,and

(7.92)

has a nontrivial solution

, f }

Example 7.23 For parameters (7.108), the equation (7.91) takes the form

= αuu

+ γ u

+ εuu

+ c

u + δ.

In view of Proposition 7.22, it possesses the solution

u(x , t) = g

+ f (x )a(t),

where a(t) solves (7.103), the constant A is given by (7.111) (here B = 0), and



. From (7.110),it follows that if G ≡ ε

− 4α(γ − A)>0, then f (x) =

, where µ

and µ

are different roots of αµ

+εµ+(γ − A) = 0.

Hence,

f (x) = (D

x + D

−εx /2α

, if G = 0,

f (x) = D

cos[ν

(x + D

)], if G < 0,

where ν

=−

2α

and ν

2α



4α(γ − A) − ε

3. General case. Let

β = 0andc

= 4βδ, (7.112)

i.e., (7.94) contains an irrational function. Substituting (7.95) into (7.98) and making

simpliﬁcations yields a linear combination of functions

1, g, g

, ... , g

R, g

R, ... , g

R, (7.113)

which must be identically zero. This gives fourteen algebraic equations for the pa-

rameters. In particular, from this system the following result is obtained.

3.1. Existence. Two existence cases are derived.

Proposition 7.24

Let

(7.90)

and

(7.112)

hold. Then, system

(7.70)

(7.71)

,and

(7.92)

possesses a nontrivial solution

{g, f }

with constant

f (x) ≡ f

β =

α(γ −B)

B−2γ

(B = 2γ), c

= c

= ε = 0,δ= 0, A = γ = 0, (7.114)

where

γδ > 0

β<0

Note that (7.114) is the sufﬁcient condition for identity (7.98) to be valid with

f = f

. Using (7.95) gives z = 0, and plugging this into (7.97) yields A = γ .

Other conditionsin (7.114) follow from (7.95) with z = 0, if we look fora nontrivial

function g(x) ≡ constant, such that all rational and irrational terms on the right-

hand side of (7.95) are linearly independent. This yields c

= c

= ε = 0and

A(α +2β)− B(α +β) = 0. A more general solution {g, f

} is given in the example

below.

Example 7.25 Under hypotheses (7.114) with B = 0(whereα =−2β), (7.91) is

=−2βuu

+ β(u

)

+ γ u

+ δ. (7.115)

7 Partially Invariant Subspaces and GSV 359

Then, system (7.70), (7.71), (7.92) reduces to







−2 ff



+ ( f

)



= 0,

−2βgg



+ β(g

)



+ γ g

+ δ = 0,

−2β(gf



+ g



f ) + 2βg



+ 2γ gf = 0.

(7.116)

As has been shown above, this system is compatible iff f (x) ≡ f

. In this case,

(7.115) admits the solution u(x, t) = g(x) + f

a(t),wherea





= γ a

.Itthen

follows from (7.116) with f = f

that g(x) has the form (D = 0 is arbitrary):

g(x) =



sin





−

x + D



,γδ>0,γβ<0,

g(x) = D exp







4γ D

exp



−





,γβ>0.

Proposition 7.26

Let

(7.90)

and

(7.112)

hold. Then, system

(7.70)

(7.71)

(7.92)

admits nontrivial solution

, e

µx

}

with

= 0,µ= 0,

provided that

(α +β)µ

+ εµ − (A − γ) = 0,γg

+ c

+ δ = 0,

[αµ

+ εµ − (B − 2γ)]g

+ c

µ = 0.

Assume that (7.96) is not valid and

g(x) = e

, f (x) = e

, where m = n.

It follows from (7.70), (7.71), and (7.93) that this solution exists if c

= c

= δ = 0,

and

(α +β)n

+ εn + γ − A = 0,

(α +β)m

+ εm + γ = 0,

β(m − n)

= A − B.

For equation (7.102), from (7.53), we have B = 0, and the abovesolution is included

into (7.105).

3.2. Nonexistence. We consider the last case where (7.96) holds. Then, as above,

(7.98) is equivalent to the identity on the linear span of the functions (7.113). The

ﬁnal result (obtained via computer supported symbolic manipulations) can be stated

as follows: under assumptions (7.90), (7.96) and (7.112), (7.98) is not the identity.

This means nonexistence of solutions {g, f } satisfying (7.96).

7.4.4 On quadratic operators with linear properties

This linear case is connected with the system 2) in Section 7.3.2. Previously, we have

studied the case where the operator T in (7.45) satisﬁed

T [u] ∈ W

= L{1, a, a

} for all u ∈ W

= L{1, a}.

Let us now show that if a(t) is such that

T [u] ∈ W

for all u ∈ W

, (7.117)

then, in some particular cases, there exist explicit solutions that differ from those

given above. Under hypothesis (7.117), the equation (7.50) on an invariant set has a

360 Exact Solutions and Invariant Subspaces

different form than (7.60). In particular, it follows from (7.55) that (7.117) is valid,

T [a] =

Aa, T [a, 1] =

B, (7.118)

where

A = 0and

B are some constants. In this case, the ﬁrst expression in (7.55)

reads T [g + fa] =

a +

Bgf. Hence, instead of (7.56),

A[g + fa] =

Bgf − F[g] + (

− F[g, f ])a − F[ f ]a

Therefore, there exists the set M

, given by (7.54), and the equation (7.59) has the



F[g] −

Bgf =−Q[g] − p,

F[g, f ] −

=−Q[ f ].

(7.119)

Observe that (7.117) holds if (cf. (7.118))

T [a] = A

a + A

and T [a, 1] = B

a + B

. (7.120)

In fact, below the case A

= B

= 0 is considered.

Theorem 7.27

Let

(7.61)

be valid. Let

(7.118)

with

A =

B = 0

hold. Then the

problem

(7.59)

admits the following solutions:

(i)

for

2α +β = γ = 0,εσ− c

= 0,αc

= ε(βσ −c

)

, with

(7.62)

u(x , t) =

−x /n

1−n

−x /n



−

σ n

x/n

+ σ n

x + a(t)



, (7.121)

where

σ(α+2β)−c

εσ−c

and

εσ−c

;

(ii)

for

2α +β = ε = γ = c

= 0

u(x , t) =

nx+D



− σ



+ D



+ a(t)



, n =

σ(α+2β)−c

. (7.122)

Proof. By using the same technique as in the proof of Theorem 7.15, we obtain

(7.75) and, instead of (7.77),

[(α +2β)σ − c

]



+ (εσ − c

) +

Af = 0. (7.123)

The abovesolution followsfrom the solvabilityof (7.123),together with the equation

α ff



+ β( f



)

+ ε ff



+ γ f

= 0. (7.124)

Having a solution f (x) of (7.123), (7.124), the function g(x ) is calculated from

(7.75).

Example 7.28 (Parabolic PDE) Consider equation (7.85). Under the hypotheses

of Theorem 7.27, there exist explicit solutions (7.121) or (7.122) with a(t) =

At.

These solutions are different from those given in Example 7.17.

Example 7.29 (Hyperbolic PDE) Under the same hypotheses, equation (7.88) has

explicit solutions (7.121) or (7.122) with a(t) =

+ Dt,whereD is arbitrary.

In addition to the case of (7.117), consider a(t) such that

T [u] ∈ W

= L{1} on W

of the set M , F[ f ] = 0 (see (7.54)), yielding

provided that (cf. (7.53))

form (cf. (7.60))

7 Partially Invariant Subspaces and GSV 361

It follows from (7.55) that this is true if

T [a] =

A, T [a, 1] =

where

A and

B are constants.Forinstance,for the operator T [u] = u

, there exists

a(t) =

, provided that

A > 0and

B = 0. The corresponding equation on



F[g] −

−

Bgf =−Q[g] − p,

F[g, f ] =−Q[ f ].

β(gf



− fg



)

= c

f (gf



− fg



) − f

(δ −

Bgf −

). (7.125)

In general, both existence and nonexistence are

OPEN PROBLEMS.Forβ = c

= 0,

where (7.125) takes the most simple form, a nontrivial solution does not exist.

Example 7.30 (On higher-order PDEs: dispersive Boussinesq equation) Con-

sider the fourth-order hyperbolic B(1,

) equation [496]

− v

−



√



− v

xxxx

= 0

that is formulated for nonnegative solutions. In general, such equations may admit

solutions of changing sign; see Example 5.12. Setting v = u

yields

T [u] ≡ uu

+ (u

)

=−uu

xxxx

− 4u

xxx

−3(u

)

+ uu

+ (u

)

+ u

(7.126)

According to the GSV in Section 7.3.1, looking for solutions u = g + fa(t) yields a

standard system (I) for a(t) that is easily solved, giving t or e

as usual. In contrast,

system (II) for f , g becomes essentially more involved than for the above second-

order cases. A complete consistency analysis is not straightforward. Moreover, for

such higher-order PDEs (7.126), looking for solutions on a trigonometric subspace,

u(x , t) = C

(t) + C

(t) cos(λx) + C

(t) sin(λx )(λ∈ IR )

yields an overdeterminedDS that allows only traveling wave compactons (similar to

those in [581]), with no exact dynamics around, as was shown in several examples

in Section 7.1. In other words, for higher-order operators F[u] and multi-term

∂

∂t

dependent operator T [u], the GSV becomes complicated and can provide us with

new exact solutions for some exceptional PDEs only.

7.4.5 On some other extensions

1. Partially invariant modules W

. In general, the operator (7.47) admits invariant

sets on the 3D subspace W

= L{1, a(t), b(t)}, where the functions 1, a(t),andb(t)

are linearly independent. If b

(t) = a(t), for instance,

a(t) = e

λt

and b(t) = e

λt

for some λ = 0 (7.127)

(this is reminiscent of soliton-type solutions), we ﬁnd a family of sets M on W

such

that, for any u = g(x ) + f (x)a(t) + h(x)b(t) ∈ M, A[g + fa+ hb] ∈ W

.Inthis

has the form (cf. (7.119))

Then, as in the proof of Theorem 7.15, we derive the equation (cf. (7.74) and (7.93))

362 Exact Solutions and Invariant Subspaces

case,

T [F + fa+ hb] = f

T [a] + h

T [b] + gfT[a, 1]

+ghT[b, 1] + fhT[a, b], F[g + fa+ hb] = F[g] + F[g, f ]a + F[g, h]b

+ F[ f ]a

+ F[h]b

+ F[ f, h]ab.

Using (7.127) yields the following result.

Lemma 7.31

Under hypothesis

(7.127)

, there exists the set on



u = g + fa+ hb : F[ f ] = A

, F[ f, h] = A



where



i, j

i+j

and



i, j

−i

+ 2

−j

)λ

i+j

Equation (7.50) can easily be stated on M

⊂ W

with A = (A

, A

). The gen-

eral problem of the existence and nonexistence of solutions on M

is OPEN.Some

examples given above can be treated as the existence result on the set M

⊂ W

.For

instance, Example 7.29 shows that the PDE under consideration admits the explicit

solution which formally satisﬁes u(x, t) ∈ M

⊂ W

= L{1, t, t

}. By translation

in time t → t + 1 in Example 7.19, we obtain a solution u(x , t) ∈ M

⊂

L{1, e

−ωt

, t e

−ωt

2. N-dimensional operators. Such sets M can be constructed for elliptic operators

in the N-dimensional space, e.g., for

F[u] = αuu + β|∇u|

+ γ u

+ εu(∇u · d)(d ∈ IR

as well as for others from Chapter 6. The problem of the existence and nonexistence

of solutions on M becomes more difﬁcult.

3. Operators with cubic nonlinearities. In general, a similar invariant analysis can

be done for operators of higher algebraic homogenuity. For instance, as in Sec-

tion 1.5, we can consider cubic operators

A[u] = T [u] − F[u] ≡



i, j,k

u −



i, j,k

(or use a combination of quadratic and cubic operators). Then, e.g., for exponential

functions a(t) = e

λt

with a λ = 0, A[u] ∈ L{1, a, a

, a

} for u = g + fa ∈ W

.In-

variant sets M ⊂ W

are deﬁned so that A[M] ⊆ L{1, a}. This yields two equations

for coefﬁcients {g(x ), f (x)}, which determine M with a harder consistency analysis.

7.5 Evolutionary invariant sets for higher-order equations

In this section, we use the notion of evolutionary invariant sets which are associ-

ated with a prescribed nonlinear PDE. Using a particular class of such equations, the

actual relation between evolutionary invariance and the standard concept of partial

invariance (invariant sets on linear modules) is shown. More general and sophisti-

cated classes of such problems will be introduced in Chapter 8.

Consider a 1D ((1+1)-dimensional)mth-order evolution PDE

= F[u] ≡ F(x, u, u

, ..., u

) in Q = IR × [0, 1], (7.128)

where u

= D

u. Suppose that F ∈ C

∞

(IR

m+2

), and solutions of (7.128) are as-

sumed to be smooth, u ∈ C

∞

(Q).