Fox J.A. Transient Flow In Pipes, Open Channels And Sewers

156

and

Unsteady

flow in open channels

lch.7

Sec.

7.71

The

positive

characteristic

Zco*

Zc(t),

Boundary

conditions

I57

slope

through

G

is

l/[u(r)

+ c(r)l

but,

as u(r)

:

,o

-

uu-Zcu:uE

Zcu

.

Assuming

that

DE is a straight

line then

along DE,

u * c is constant

(as

the slope

of the

line is ll(u+

c)) and u*2c

is

constant;

therefore

u and c must

be

constant.

Therefore

DD:DEand

Co:CE

.

so

uo-Zco:

Un-ZCn

but

A.B is a C*

characteristic;

so

un*2c6=

ua* hB

.

This

can only

be

true if ua:

uB

and c^

:

ca

irnd this implies

that

AB is

a straight

line

because 1.1(u

+ c) is

constant

along

AB.

Thus,

if i:0

and

j

=

0

and

if any one

of the C* or C-

families

is straight,

all

the

other members

of

that same

family

must

be straight also.

This argument

can be

applied to the

C-

family

in an exactly

similar

manner.

Next consider

a wave

propagating into and

over a uniform

flow.

E for a

uniform

flow is zero

as 1:

j;

so

the above

arguments

will

apply if the

wave is small.

The wave

will

propagate at a

velocity of

us

* co where

uo and co are the

velocity

and

celerity in

uniform flow

respectively.

Then,

in

Fig. 7

.6thecharacteristic OFcan

be

drawnwith

a

slope of 1/(us

* co).

Below

OFis

the

zone of

quiet,

i.e. the

zone

of

undisturbed

flow.

OFis a

positive characteristic

and,

as uo and

cs must be constant

in uniform

flow,

OF

must be a straight

line

.

If OF

is straight,

all

other

positive

characteristics

must

also

be

straight. The C-

characteristics

are not straight

except inside

the

zone of

quiet.

Consider a

wave starting

out from

r: 0

at time r, i.e.

from

point

G.

The

value of

u(l)

and

c(t)

at this

point

must

be known

but in

fact

only

one of

them

is likely

to be

available. Draw

a C-

characteristic

back from G

to line OF;it

will be seen

that

u(4

-

2c(t):

us-2cs

'

so u(r) and c(r)

are interdependent.

Then if c(t)

is specified,

u(t):

us-Zcs+Zc(t)

'

In fact

either

can

be specified

and

then

the other

can be deduced

from

this equation.

:3c(r)

*

us-

2c,

or,

expressing

c(t)

in

terms

of

u(t),

9:

t.tr1r)

-

o.5uo

* co

dr

From

the above,

the

values of

u and

c can

be

obtained

anywhere

in

the

plane. This

approach

can

be

used

to solve

many

situations

in

which

E:0.

The

technique

is

eiementary

but

the

results

can be extremely

valuable.

Further

techniques

using

this

assumption

will be

illustrated

later

in this

chapter.

7.7

BOI.]NDARY

CONDITIONS

7.7.L

The

upstream

reservoir

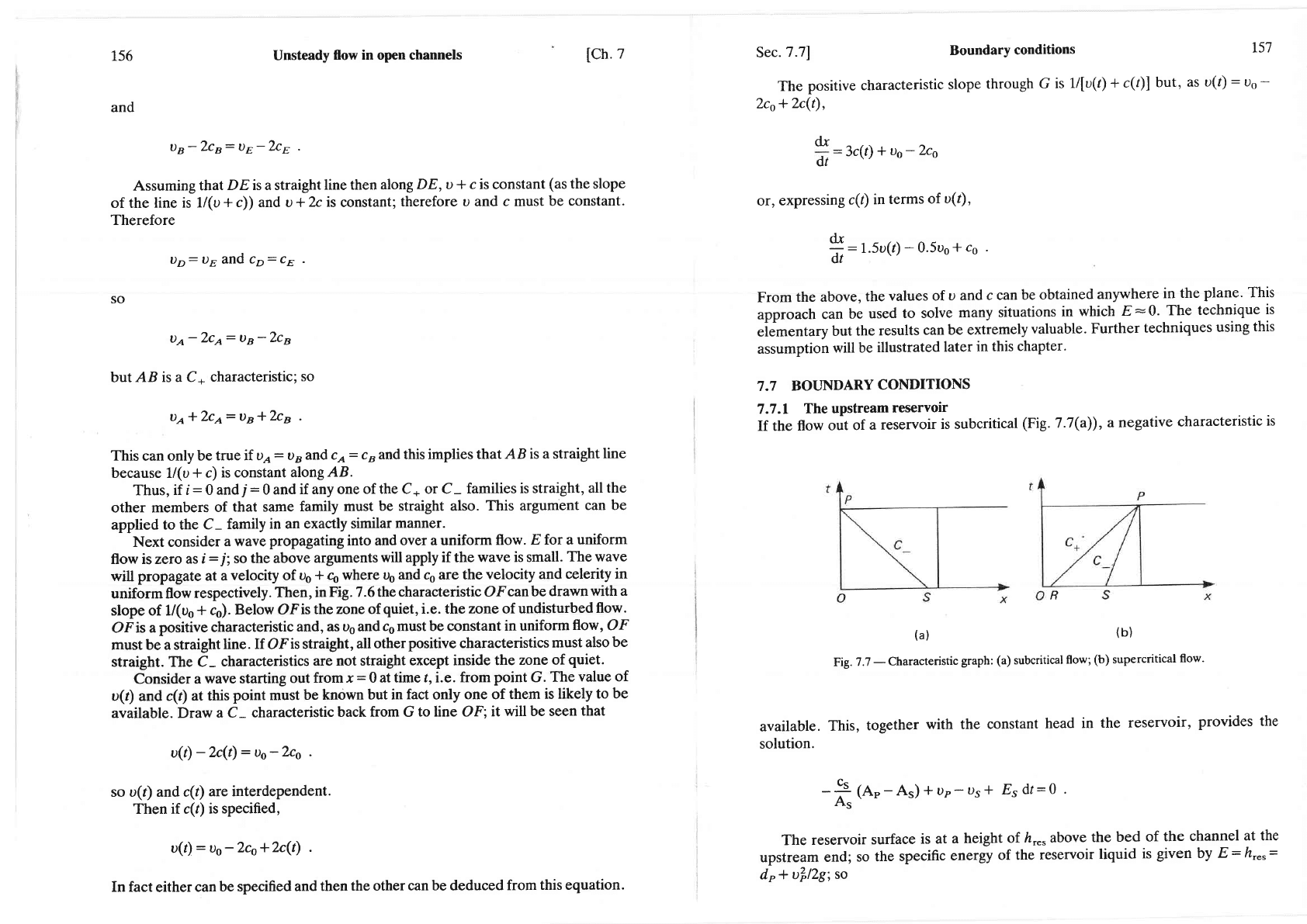
If the

flow

out

of

a

reservoir

is subcritical

(Fig.7.7(a)),

a negative

characteristic

is

(a)

(b)

Fig.7

.7

-

Characteristic

graph:

(a)

subcritical

flow;

(b)

supercritical

flow.

available.

This,

together

with

the constant

head

in

the

reservoir,

provides the

solution.

-F

(a"-Ar) *

up-

ur* E5

dr:o

.

Ast

The

reservoir

surface

is at

a

height

of

ft.." above

the

bed

of

the

channel

at the

upstream

end;

so

the

specific

energy

of the

reservoir

liquid

is

given by

E:

h,""=

dr+

u/lZg;

so

dr

dt

R

158

Unsteady

flow in

open channels

lch.

7

ur:tlIffi]"3;

The geometry

of

the required channel cross-section

specifies

the relationship

between Arand dr; so d" in the above equation

can be specified

as/(A")

where/is

the

required function.

The celerity cis

given

by

c:

y'gb

and 6 is equal

to AlBswhere

B,

is the

breadth of

the water

surface.

85 is a function of.

A; so 6

is a function of.

A.

Then

-?

(er-Ar)

+\,WM;l+

Es dt-o .

Ar'

A"

is

obtained

by interpolation

between

the first and second

Ax nodes as was

done in

the waterhammer section.

This is an equation

in which every

variable

is known

except A";

so

a

solution

is

possible. If the

channel

is rectangular, the solution

is

quadratic

but, if it is of a

more complex shape,

iterative

processes will

be

required to

obtain

Ap.

Tlhe Newton-Raphson

solution

technique should be

remembered

in this

connection. Let the function

to be solved

be/and let the differential

of it be

d/with

respect to the independent

variable. If the first

estimate

for the solution

isx,

then the

second

estimate

is x,

where

Iteration must be repeated

until the difference

betweenrl andx2is

acceptably small.

If the

flow in the channel

is supercritical

(Fig.

7.7(b)),

then the upstream

depth

must

be critical

and is

calculated using

the fact that the

Froude number

will be unity.

Then

D/16:1

and so

ult/SA/Ut--1.

The

value

of.

Albs is a

geometric

property

of

the area

A" and can be expressed

as a function of

Ar, i.e.

/(Ap);

so

u"tlffi: l and then

ua:\ffi

Now,

assuming that

from

just

inside the

reservoir to the entry of

the channel

there

will

be

no energy

loss, it

follows that E

:

dp *

uzrt2g and u"

:

!F

from the above

statement

that

the

Froude number equals

unity. Then

_

__

_f

*2_ *L

df

T{

I

E: hr"r:

ar*|,

.

Now

d" must be a

function of

A" also.

Let

this

function be

l,(A").

Both

the

function for 6

and the functionfor

d" must, of course,

be known.

Therefore

Sec.7.8l

I)ownstream

reservoirs

159

h,",:X(A")

+

f(Ar)

So

A" can be

calculated.

Once

^4" is determined, Dp

can be calculated

also from

the

equation up:

VB6.

The characteristic

equations

cannot be used to solve

the

boundary condition

as

they do not

intersect

at the upstream end

of the

channel.

Note that

the critical

slope

of

a

channel

is

defined as that

slope atwhich

the uniform flow

depth equalsthe

critical

depth.

The critical slope

equals

96/

Czm where C is the Chezy

C and

m is the

hydraulic

mean depth.

In a channel

of slope equal

to critical

or supercritical

slope

the

upstream

depth is

given

as

follows.

For a rectangular

cross-section

dP2

;-

-;

ftr.t

J

For a triangular

cross-section,

For a circular

cross-section,

p2

Ap:i

$-sinO)

,

where 0 is the

angle subtended

at the centre

by the

water surface.

If.il<R,0:2

arctan[1@tI|(R_.d)].Ifd.>R,0:2t_2arctan|l@l(d_R)].If

abs(d-R)<0.0001,

Q:r.

h,.,: o

*|:

^(t

-

cos

l)

.

f

(o

-

sin ty"'

(:)

Solve

for 0 using

the Newton-Raphson

technique

and

then solve

for d

which, of

course,

is

4nr.

h,""ld can

then

be

easily

evaluated.

Other

cross-sections

can

be solved

similarly

but circular

cross-sections

are,

probably,

the

most awkward.

It is straight

forward

to solve

the trapezoidal

section

and

it results

in a

quadratic

solution.

7.8

DOWNSTREAMRESERVOIRS

Again,

there are

two

possibilities:

subcritical

and supercritical

flow conditions.

As

with upstream

reservoirs,

if the flow

in the channel

is

subcritical,

then

a

positive

d,

_!

hr"t 5

ar:znfr

-*'l)

160

Unsteady

flow

in open channels

lch.

7

characteristic

can

be used

together

with the

reservoir condition

to

give

a solution.

However,

with supercritical

flows, the situation

is different

from

upstream

reservoirs

in

that a

solution

is

obtained

by

the use

of both characteristics

originating

in

the

last

Ar in

the reach

to

give

a solution.

7.8.1

The downstream

reservoir

with a

subcritical

approach

flow

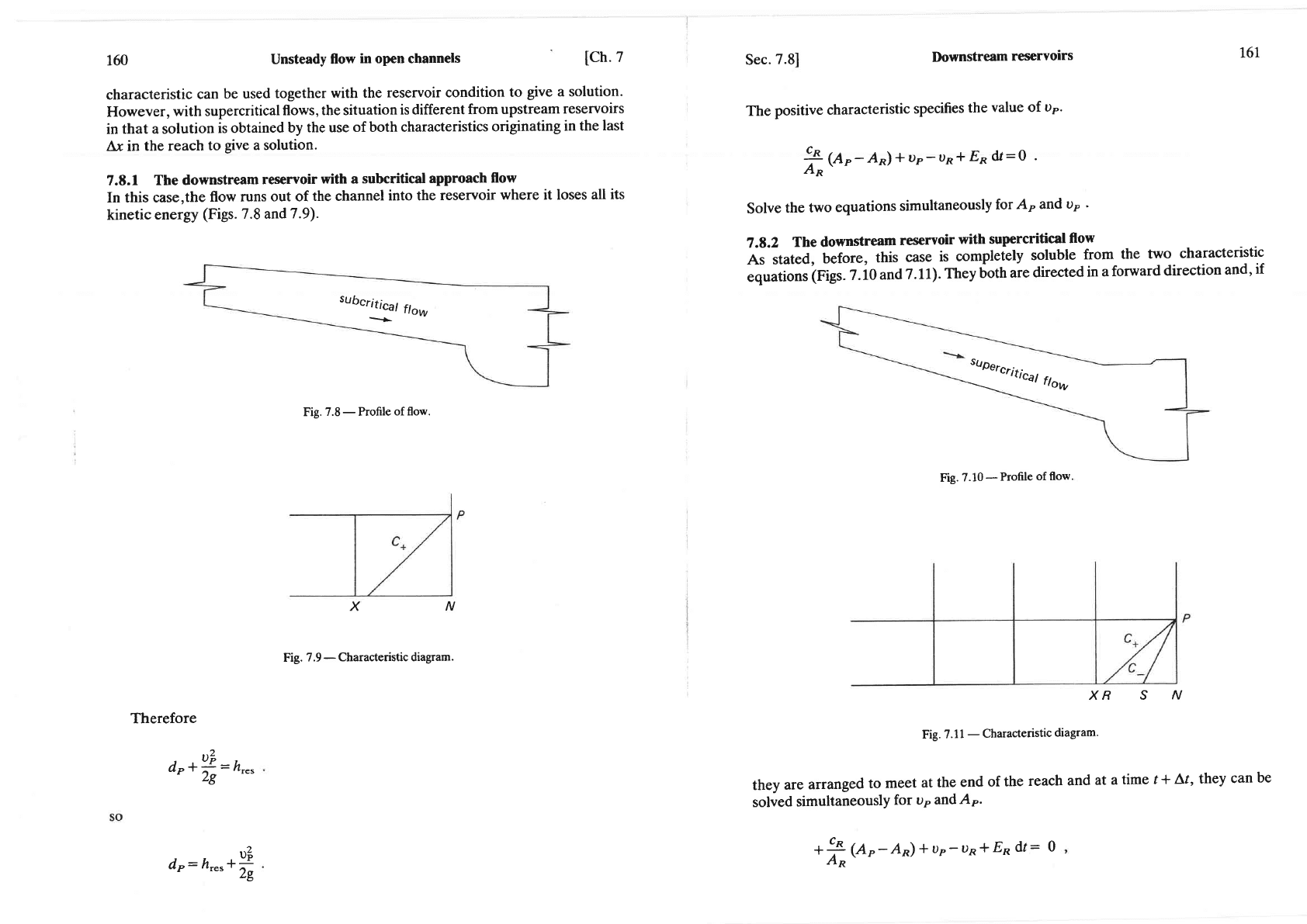
In this case,the

flow runs

out of

the channel

into the

reservoir

where it loses

all

its

kinetic

energy

(Figs.

7.8 and7.9).

Fig.

7.8

-

Profile

of flow.

Fig.

7.9

-

Characteristic

diagram.

Therefore

)

d"

+?

=

h,",

'zg

de:

hr"t+fi

D,ownstream

reservoirs

161

Sec.7.8l

N

x

The

positive

characteristic

specifies

the

value of

u"'

? @r-A^)

* ue-

u^*

E^

dt:0

.

Ap

Solve

the

two

equations

simultaneously

fot

Ap

and

u"

'

7.E.2

The downstream

reservoir

with

supercritical

flow

As stated,

before,

this

case

is

completely

soluble

from

the

two

characteristic

equations

(figr.

7 .L0

andT.LL).They

both

are

directed

in

a

forward

direction

and,

if

Fig.

7.11

-

Characteristic

diagram'

they

are

arranged

to

meet

at

the

end

of

the

reach

and

at

a

time

t +

Lt,

they

can

be

solved

simultaneously

for up

and

Ap.

subcritica/

f/ow

T.,"*

tflow

Fig.

7.10

-

Profile

of flow.

XR S

N

.

fr(Ar-A^)

+ ur

-

uR

*

En

dt:

o

162

Unsteady

flow

in

open

channels

cs

r^ ,r \ r

-

^"(Ap-ls)

+ Dp

-

us+ Es

dt-

o

-

Sec.7.9l

The

sluice

gate

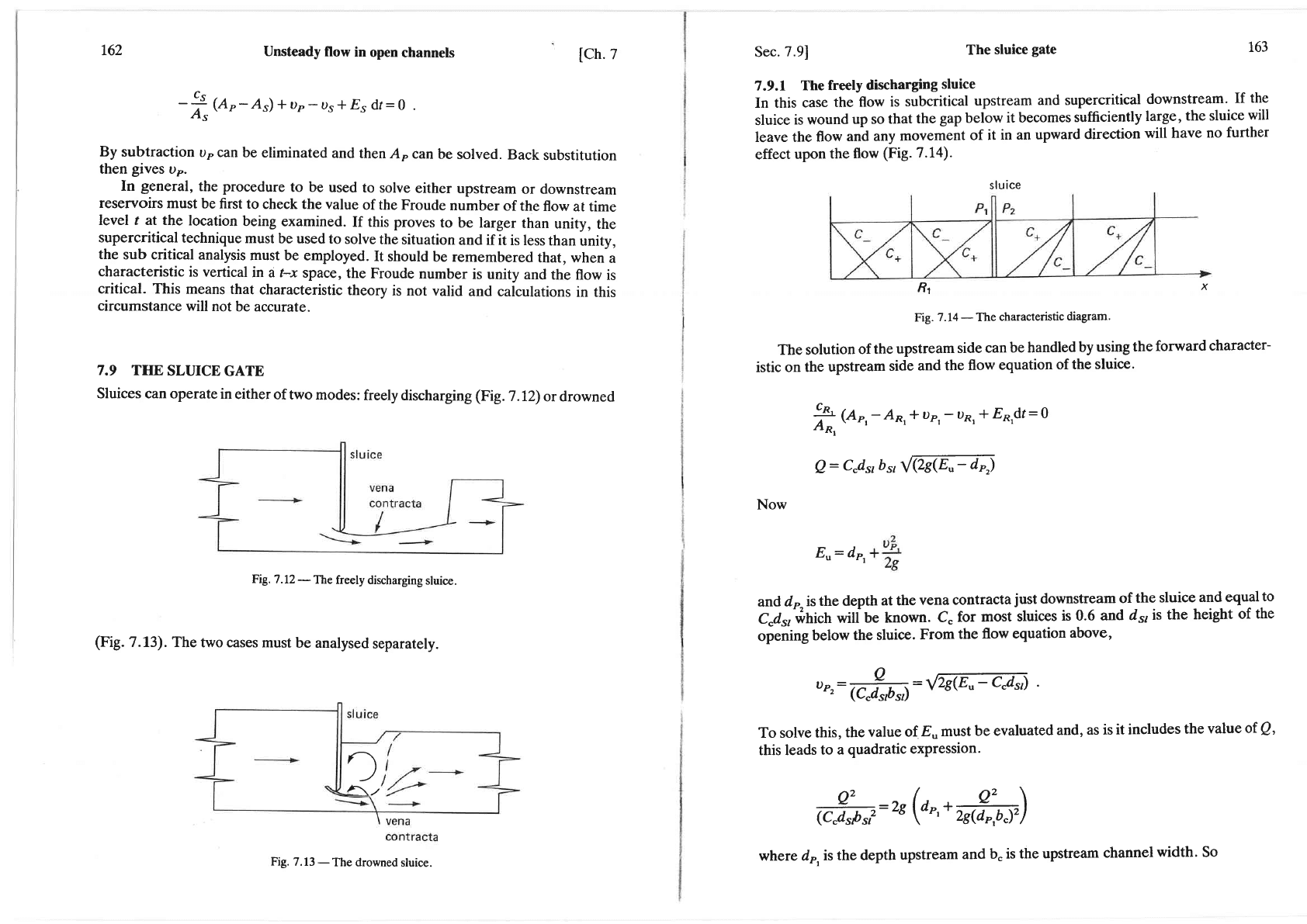
7.9.1 The

freely discharging

sluice

In this

case

the

flow

is

subcritical

upstream

and supercritical

downstream.

If

the

sluice

is wound

up so that

the

gap below

it becomes

sufficiently

large,

the

sluice

will

leave the

flow

and

any

movement

of

it

in an

upward

direction

will have

no further

effect

upon

the

flow

(Fig. 7.1a).

R1

x

Fig-

7.I4

-

The

characteristic

diagtam'

The solution

of

the

upstream

side

can

be handled

by

using

the

forward

character-

istic on

the

upstream

side

and

the

flow

equation

of the

sluice.

.c"r-

A*,

(A"r- ARr+

Dr,

DR,

* E^,dr:0

Q:C"d*b*l@4r)

Now

Eu:

dP,

and

d,

is

the depth

at the

vena contracta

just

downstream

of

the

sluice

and equal

to

Cgr,'&nich

wif

be known.

C"

for

most

sluices

is 0.6

and

ds

is

the

height

of

the

opening

below

the sluice.

From

the

flow equation

above,

n

'

r,:

efu--

!Zs(E"

-

c"dt)'

To solve

this,

the

value of

E,,

must

be evaluated

and,

as is

it includes

the

value

of

Q,

this leads

to a

quadratic

expression.

163

lch.7

By

subtraction

up

can be

eliminated

and

then

A" can

be

solved.

Back

substitution

then

gives

u".

In

general,

the procedure

to be

used

to

solve

either

upstream

or downstream

reservoirs

must

be first

to

check the value

of the Froude

number

of the

flow

at time

level r at the

location

being

examined.

If this

proves

to be larger

than

unity,

the

supercritical

technique

must

be used

to

solve

the

situation

and if

it is less

than

unity,

the

sub critical

analysis

must be

employed.

It should

be remembered

that, when

a

characteristic

is vertical

in a f-x

space,

the

Froude

number

is unity

and

the

flow is

critical. This

means

that

characteristic

theory

is not valid

and

calculations

in this

circumstance

will

not

be accurate.

7.9 TIIE

SLUICE

GATE

Sluices can operate

in

either

of two

modes:

freely

discharging (Fig.

7

.12)or

drowned

Fig.7

.I2

-

The

freely

discharging

sluice.

(Fig.

7.13). The

two cases

must

be analysed

separately.

Q,

I

q_\

(c"drhr?:Zs

\d"'.ffi)

where dp,isthe

depth

upstream

and

b.

is the

upstream

channel

width. So

sluice

)

\.u

Fig.

7.13

-

The drowned

sluice.

7l

(c

"d

srb

s)2

-

ll

(d

P,b.)2

Unsteady

flow

in

open

channels

lch.

7

Q:

Next,substitutethevalueof

u.,,whichequals

Qlb.dp),intotheupstreamcharacter-

istic

equation.

,^+_

1+

"

dRr

b4o,

-un*

E^dt-0

.

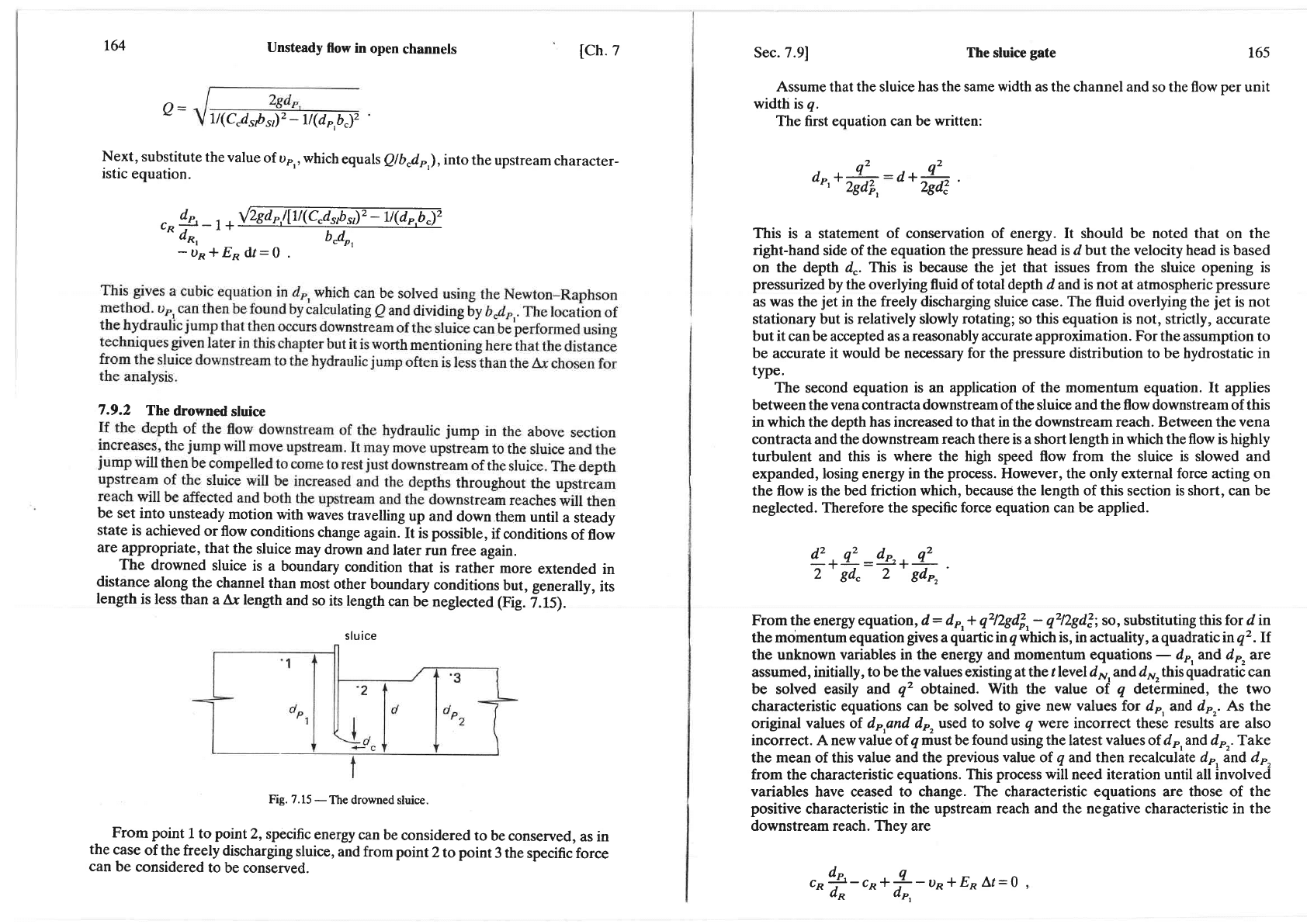
7.9.2

The

drowned

sluice

be

set into

unsteady

motion

with

waves

travelling

up and

down

them

until

a

steady

state

is

achieved

or flow

conditions

change

again.

It is

possible,

if conditions

of flow

are appropriate,

that

the

sluice

may

drown

and

later

run

free

again.

The

drowned

sluice

is

a

boundary

condition

that

is rathei

more

extended

in

distance

along

the

channel

than

most

other

boundary

conditions

but, generally,

its

length

is less

than

a Ar length

and so

its length

can

be neglected

Fig.

its)

Fig. 7.15

-The

drowned

sluice.

From point

1 to

point

2,

specific

energy

can be

considered

to

be conserved,

as in

the

case

of the freely

discharging

sluice,

and

from

point

2

to

point

3 the

specific force

can

be

considered

to

be conserved.

r64

sluice

Sec.7.9l

The

sluice

gate

165

Assume

that

the

sluice

has the same

width

as the

channel and so the flow

per

unit

width is

q.

The first equation can

be written:

-q2-o2

uP"T

-:--

-

-"'

2gd?,

* '

Zgt

'

This is a

statement

of conservation of energy. It should be noted that

on

the

right-hand

side of

the

equation

the

pressure

head is d

but

the

velocity

head is based

on the depth d.. This is because the

jet

that issues from the

sluice

opening is

pressurized

by the overlying fluid of total depth d and is not at atmospheric

pressure

as was the

jet

in the

freely discharging

sluice

case. The fluid overlying

the

jet

is not

stationary but is relatively slowly rotating; so this equation is not,

strictly, accurate

but it

can be accepted

as a reasonably accurate approximation. For

the assumption to

be

accurate it

would be necessary for the

pressure

distribution

to be hydrostatic in

type.

fire

second

equation is an

application

of the

momentum

equation.

It

applies

between the

vena

contracta

downstream of the

sluice

and the flow

downstream of this

in which

the depth

has increased to that in the downstream reach. Between the vena

contracta and the downstream

reach

there is a short length in which the

flow

is highly

turbulent and this

is where the high

speed

flow from the

sluice

is slowed and

expanded,

losing energy in the

process.

However, the only external force acting on

the flow is the

bed friaion which, becausd the length of this section is short, can be

neglected. Therefore the specific force equation can be applied.

d'-Q'

:b*

Qt

2'Bd. 2

'Bde"'

From the

energy

equation, O- drr+

q2lLgd|,- qzl2gd!;

so, substituting

this

for d

in

the momentum equation

grves

a

qriartic

in

q

which is, in actuality, a

quadratic

in

q'

.lf

the unknown variables

in the energy and momentum equations

-

dprand

drrare

assumed, initially,

to be the

values

existing

at the t level dN, and dr, this

quadratic

can

be

solved easily

and

qz

obtained. With the

value

of

q

determined, the two

characteristic

equations

can be solved to

give

new

values

for dp, and dpr. As the

original

values

of

derand dp, used to solve

q

were incorrect these results are also

incorrect. Anewvalueof

qmustbefoundusingthelatestvalues

of drranddpr. Take

the mean of this value

and

the

of

q

and then recalculate dprand dp,

from the characteristic equations.

This

process

will need iteration until all involved

variables

have ceased to

change. The

characteristic equations

are those of the

positive

characteristic

in the upstream reach and the negative characteristic in the

downstream reach. They are

t-h-

cn

*

t,-

u^*

E^At

=

0

,

166 Unsteady flow in

open

channels

doq

trt*c5*t,-u5*E5Ar:0

lch.

7

These

equations

are both quadratic

and

easily solved

(Fig.

7.16).

:l:T,il'."'?,1'"::"J'il:s

^

necessary

additional two

equatrons

Fig. 7.16

-

The

characteristic diagram for

a

drowned

sluice.

The iterative

process

is best

done

on a computer but, as the

analysis of

unsteady

flow

is also only

possible

by computing

methods, this is no

problem.

It

may happen

that the number of iterations required

becomes excessively large;

so it is necessary to

build into the subroutine

by which

the sluice is

solved a

rflamping

process

which

can

be

invoked when

this

happens. It is suggested

that thevalue

of

q

should be controlled so

that no large

alterations

in

its value

can occur

from one iteration to the next. The

taking

of

a mean

value

between two successive calculations

described

above

is such a

damping

process

but even

this may not damp

sufficiently

powerfully.

A

suitable

damping

value

of

q

may be obtained by

adding one

tenth of

the

most recent

value

to

nine

tenths

of the

previously

calculated

value.

Most of the dfficulty of

writing computer

programs

to

deal

with

unsteady flow in

open channels lies

in establishing stable ways

of solving high order

polynomial

or

transcendental equations. Transcendental equations

are involved

in channels of

circular

cross-section such

as

sewers.

7.IO

BROAD.CRESTEDWEIRS

The

analysis of a

broad-crested

weir

(Fig.

7.17)

is the

same as

that

for a spillway and is

very

similar to that

for freely discharging venturi

flumes and bed-hump flumes.

For a freely discharging broad-crested weir

the flow is

given

by

in this Ax

the

quasi-steady

approach described

above

is

used

Q

:

L.705C

db

*(E

u

-

h*)t''

(Arr-An)+

upr-

u^*E^

At=0

Sec.7.10l

Broad-crested

weirs

167

Fig.1

.17

-

The

broad-crested

werr.

where Co

is

the coefficient

of discharge

of the

weit, b*

is the

breadth

of

the

weir,

E,,

is

the specific

energy

of

the

upstream

flow,

and

ft* is the

height

of

the

weir

with

reference

to

the

bed

of

the

upstream

channel.

This result

can

be

found

in

any standard

text

on fluid

rnechanics.

only

the

upstream

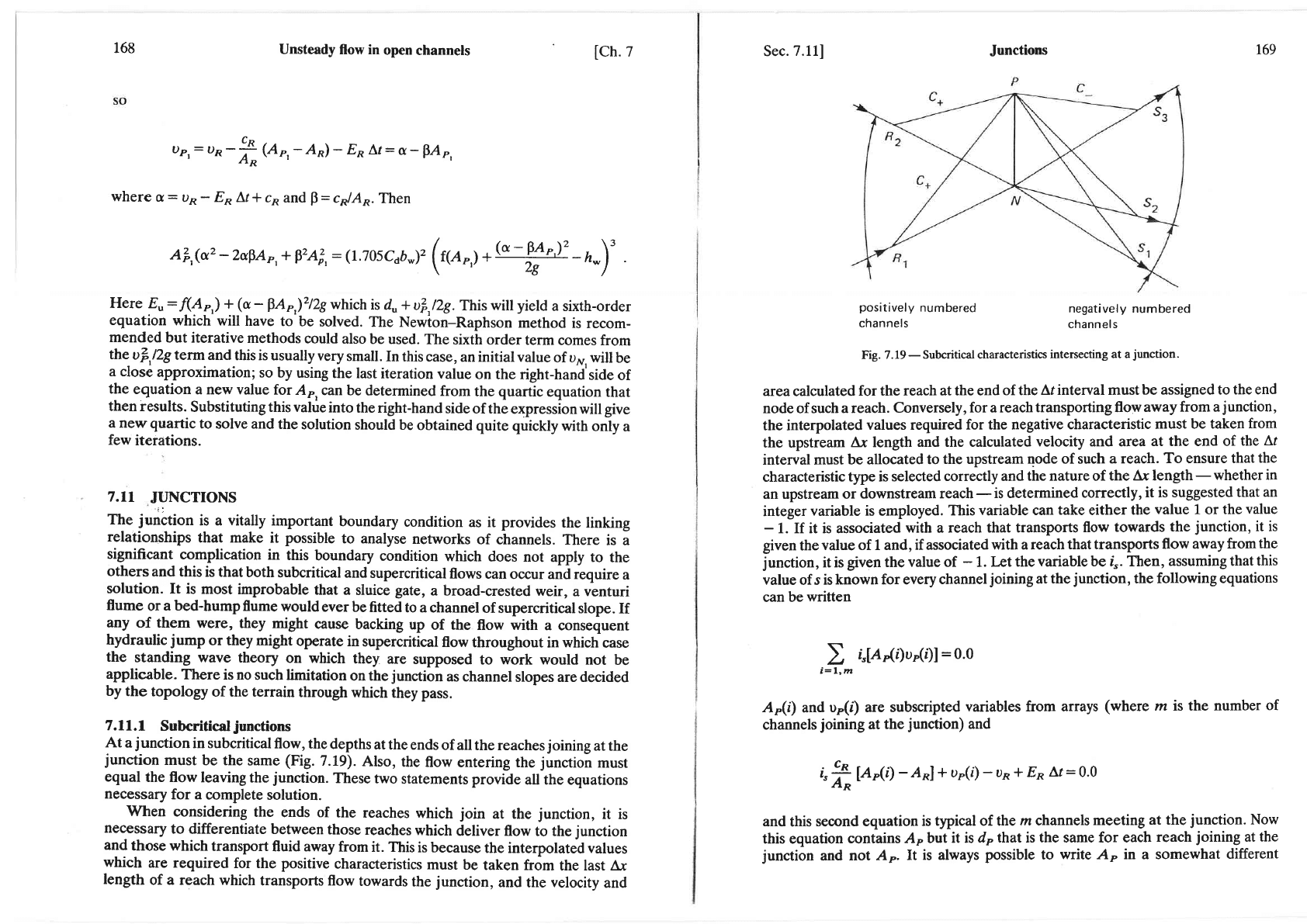
characteristic

(Fig.

7.18) is

needed,

together

with

the

weir

x

R

Nl

upstream

solution

bY

use

of C*

equation

and

weir

equation

Fig. 7.18

-

The

upstream

characteristic.

equation,

to solve

the

boundary

condition.

Q=l.705cdb*(Eu-

h*)to

and

the upstream

characteristic

equation

is

CR

AR

N2

sv

downstream

solution

bY

use

of

flow

from upstream

equation

and

C_

equation

broad-crested

weir

and

disturbed

downstream

flow

occupies

this

space

168

Unsteady

flow in

open

channels

fch.7

u,',

%-fr

(A",-A^)-

E^Lt:s-gAp,

where

d:

uR

-

ER

Lt+

c^and

p

=

cnlAn.

Then

A?.,(o'-ZupAr,+PtA1,:(1.705Cd

b),

("r

\ r

(u-P,A'tz

\3

\f(A",)*v-h*)

Here

E,:

f@r,)

+

(o

-

pAr,)212g

which

is

d" +

u2r,/zg.

This will

yield

a sixth-order

equation

which

will

have

to

be

solved.

The Newton-Raphson

method

is

recom-

mended

but iterative

rnethods

could

also be used.

The

sixth

order

term comes

from

the.ozp,lZg

term.and

this is

usually very

small.

In

this case,

an initial value

of ur, will

be

a close

approximation;

so by

using

the

last iteration

value

on the right-hand'side

of

the

equation

a new value

for

Ar,can

be determined

from

the

quartic

equation

that

then results.

Substituting this value

into

the right-hand

side

of the

expression will

give

a newquartic

to solve and

the

solution

should be

obtained quite

quickly

with

only

a

few

iterations.

7.II

JT]NCTIONS

The

junition

is a

vitally

important

boundary

condition

as it

provides

the

linking

relationships

that make

it

possible

to

analyse

networks

of channels.

There

is i

significant

complication

in

this

boundary

condition

which

does

not

apply to

the

others

and this is

that

both

subcritical

and

supercritical

flows

can

occur and require

a

solution.

It

is most

improbable

that a

sluice

gate,

a broad-crested

weir,

a

v-enturi

flume

or

a bed-hump

flume

would

ever be

fitted

to a

channel

of

supercritical

slope. If

any

of them

were,

they might

cause

backing

up

of the

flow with

a

consequent

hydraulic

jump

or they

might

operate

in

supercritical

flow

throughout

in which-case

the

standing wave

theory

on which

they

are

supposed

to

work

would not

be

applicable.

There

is no

such limitation

on the

junction

as channel

slopes are decided

by

the

topology

of the terrain

through

which

they pass.

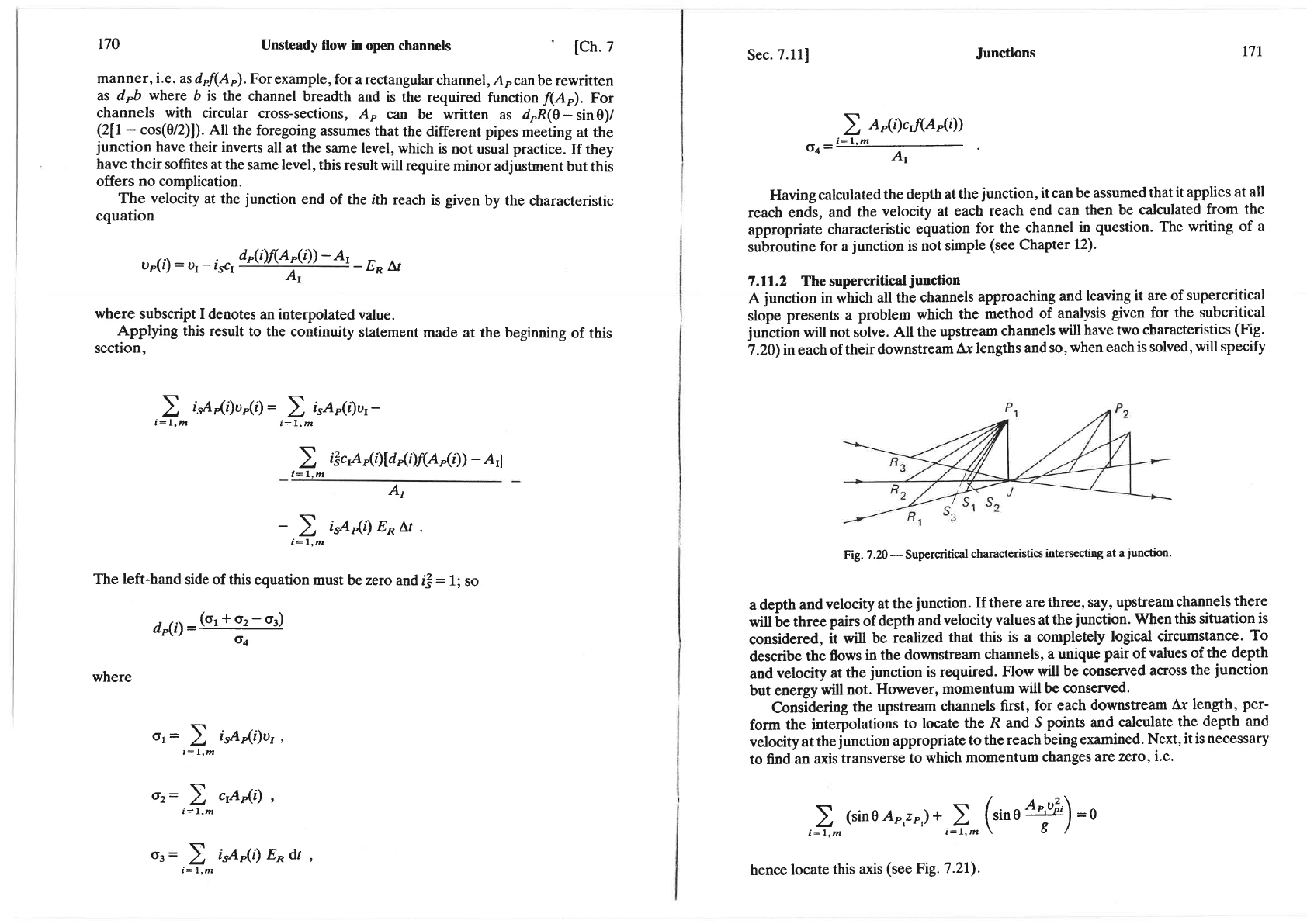
7.ll.l

Subcritical

junctions

At

a

j

unction

in subcritical

flow,

the

depths

at

the ends

of all

the reaches

joining

at the

junction

must

be the

same

(Fig.

7.19).

Also,

the

flow

entering the

junction

must

equal

the flow

leaving

the

junction.

These

two

statements

provide

all the

equations

necessary

for a

complete

solution.

When

considering

the

ends of

the

reaches

which

join

at the

junction,

it

is

necessary

to differentiate

between

those

reaches

which

deliver flow

to the

junction

and

those which

transport

fluid away

from

it. This

is because

the interpolated values

which

are required

for the

positive

characteristics

must

be

taken from

the last Ar

length

of a reach which

transports flow

towards

the

junction,

and

the

velocity

and

Sec.7.11l

Junctions

positively

numbered

channels

negatively numbered

channel s

Fig. 7.19

-

Subcritical characteristics

intersecting at a

junction.

area calculated

for the reach

at the end of

the

Arinterval must

be assigned to the

end

node of such

a reach

. Conversely,

for a reach

transporting flow

away

from a

j

unction

,

the interpolated

values required

for the negative

characteristic

must

be taken

from

the upstream

Ax length

and the calculated

velocity

and

area

at the end of

the

Ar

interval must

be allocated

to

the upstrearn

node of such

a reach.

To

ensure

that

the

characteristic

type is selected

correctly and

the nature of

the Ax length

-

whether

in

an upstream or

downstream

reach-is

determined

correctly,

it is suggested

that

an

integer

variable is

employed.

This

variable can

take

either

the

value

1- or

the

value

-

1. If it is associated

with a reach that

transports

flow

towards

the

junction,

it is

given

the

value of L and,

if associated

with a reach

that transports

flow away

from

the

junction,

it is

given

the

value of

-

1. I-et

the

variable be i,. Then,

assuming that

this

value

of s

is

known for every

channel

joining

at

the

junction,

the

following equations

can

be

written

i:l,m

Ar(i) and

up(i) are

subscripted

variables from arrays

(where

m is the number

of

channels

joining

at

the

junction)

and

i,

+ IAr(i)

-

A*l+ up(r)

-

un * E*

Lt:

0.0

"

An'

and this

seoond equation

is

typical of the

rz channels

rneeting

at the

junction.

Now

this

equation

contains.-4p

but

it is d"

that is the same for each

reach

joining

at

the

junction

and

not Ap.

lt

is always

possible

to

write A, in a somewhat

different

1,69

170

Unsteady flow

in

open

channels

lch.

7

manner,

i.e.

as d"f(Ar).

For

example,

for

a rectangular

channel,

Arcanbe

rewritten

as dfr

where

b is the

channel

breadth

and

is the

required

function

/(A").

For

channels

with

circular

cross-sections,

Ap

can be written

as dpR(Q

-

sinO)/

(2[1

-

cos(0/2)]). All

the

foregoing

assumes

that

the different

pipes

meeting

at the

junction

have

their inverts

all at the

same level,

which

is not usual

practice.

If they

have their

soffites

at the

same level,

this result

will

require

minor

adjustrnent

but

this

offers

no

complication.

The

velocity

at the

junction

end of the

ith reach

is

given

by the

characteristic

equation

up(i):

u,

-

irc,

dPQ)f(AP(i))

-

A'

E* Lt

where

subscript I denotes

an

interpolated

value.

Applying

this result

to the

continuity

statement

made

at the

beginning

of this

section,

i:I,m

i-l,m

i:l,m

AI

i:l,m

The

left-hand

side of this

equation must

be zero and

152

:

1;

so

/or+oz-os)

d{i):=-ff

where

O1

:

i:l,m

02-

i=L,m

O3-

i:l,m

Ar

Sec.

7.111

Junctions

\

e,1i1r,f(A,(i))

i:L-m

oo:T

Having

calculated

the depth

at

the

junction,

it can be

assumed

that

it applies

at all

reach ends,

and

the

velocity

at each

reach

end

can then

be calculated

from

the

appropriate

characteristic

equation

for

the channel

in

question. The

writing of

a

subroutine

for

a

junction

is

not

simple

(see

Chapter

l2).

7.11.2

The

supercritical

junction

A

junction

in

which

all the

channels

approaching

and

leaving

it are of

supercritical

slope

presents a

problem which

the

method

of

analysis

given

for

the

subcritical

;unction

will

not sblue.

All the

upstream

channels

will have

two

characteristics

(Fig.

7 .20)in

each

of

their

downstream

A.r

lengths

and

so,

when each

is solved,

will specify

Fig.7.N

-

Supercritical

characleristics

intersecting

at a

junction.

a depth

and

velocity

at

the

junction.

If there

are

three, say,

upstream

channels

there

will be

three

pairs of

depth

and

velocity

values

at the

junction.

When

this

situation is

considered,

it

will

be

realized

that

this

is a completely

logical

circumstance.

To

describe

the

flows

in the

downstream

channels,

a

unique

pair

of

values of

the depth

and

velocity

at

the

junction

is

required.

Flow

will be conserved

across

the

junction

but energy

will not.

However,

momentum

will be conserved.

C.onsidering

the

upstream

channels

first,

for each

downstream

Ax length,

per-

form

the interpolations

to

locate

the

R and

S

points

and

calculate

the

depth and

velocity

at the

iunction

appropriate

to the

reach

being examined.

Next,

it is

necessary

to find

an

axis

transverse

to

which

momentum

changes

are

zero,

i.e.

t7l

i:l,m

i:L,m

\

o

/

hence

locate

this

axis

(see

Fig.

7.2I).

172

Unsteady

flow in

open

channels

lch.

7

Then

sum the

components

of the

specific

force

arong

this

axis.

This

summed

value

can be

equated

to

that

of

the

specific

force

of the

flow

immediately

downstream

where

it

can

be assumed

that

the

upstream

flows

have

mixed. This

mixing

must

be assumed

to

have

occurred

beciuse

otherwise

the

problem

will

cease

to

be one

dimensional.

The analysis

of the

entire

network

is

based

on

this

assumption

that

the

flow is

one dimensional.

From

continuity

and

this value

of

the specific

force,

the

mean

depth

d, and velocity

u,

at

the

junction

can

be

determined.

The velocities

at

the upstream

end

of

the downstream

reachs

can

all be

taken as Dr.

This

is

because

in

supercritical

flow

no

effect

of the

downstream

flow is

transmitted

upstream;

so the

velocity

is

determined

entirely

by the

conditions

in

the

reaches upstream

of

the

junction.

These

values

are

available

for

use in

determining

the R and

S

values

in all

the

downstream

reaches

for

time

t +

Lt. The

current

values

(at

time

t, obtained

in the

can

be

used

to

obtain

un, dnand

u,

and d5, by interpolation,

in

the upstream

Ar

of

all

the downstream

reachei.

From

these, the values

of

velocity

and

depth

at the

second node

in the

downstream

reaches

can then

be obtained.

The

junction

is

thus

solved.

The

equations

to be

solved

are

?

@r-

A*)* up-

u^*

E*

At:0,

raR

CS

,,

A:

(o"-

A.) * up-

u5* E"

At:0

-

,

There

must

be a

pair

of such

equations

for

every

reach.

i:r,m U*r

I

/

r

I

where

the subscript

i

denotes

the fth

reach

and

z is

the

depth

of the centroid

below

the

surface.

Using continuity,

sl

Z

Ao,ur,:

Ai)t

i:l,m

gives

Ar*u"

for

all

downstream

reaches

at the

second

node.

The

function

that

relates

zr to the

cross-sectional

area

Arfor

every

reach

must

also

be known

of course.

7.11.3

The

case

of a

junction

at which

both supercritical

and subcritical

channels

join

This type

of

junction

is

very

difficult

to

deal with.

If supercritical

and

subcritical

flows

are

present

at the

same

time

travelling

surges

may

occur;

at

the

junction,

supercriti-

cal flows

from

narrow

channels may

run

across

deeper

subcritical

flows

from

other

Sec.7.12l

The

unsteady travelling

surge

173

line

of

symmetry

of approach

flow

Fig.7.2I

-

The

choice of the line of symmetry.

upstream

channels

cutting

across the surface

and creating

troughs

across them;

they

may cause surges

to travel

upstream

or downstream.

The flow

may

not be one

dimen-

sional and

there

may

be multiple

travelling surges

in different

reaches or

in one

reach.

Obviously,

it

would be desirable

to model

accurately every

phenomenon present,

but the

program to

do this

would be

prohibitively

lengthy and

very

difficult to

write.

The

only suggestion

that

the writer can

make is that the

junction

must be

greatly

simplified so

that

the

junction

can be crossed

and the rest of

the

network analysed.

The

presence

of

travelling

surges

can be neglected

and a one

dimensional

approach

can be used,

neglecting

all multi-dimensional

effects.

To obtain

depths

and

velocities

at

the

junction,

it is suggested

that

a simplified

method based only

on continuity

be

used. This

will ignore

wave action

and

will

preserve

only mass

flow.

It will not

even

do this accurately

because a storage

effect

in the Ar lengths

adjacent

to the

junction

is

used.

The flow

into the

upstream ends

of downstream

Ax

values

in the

upstream

reaches

minus the

flow out of

the downstream

ends of the

upstream

A.r

values in

the

downstream

reaches

is the rate at

which fluid

is being stored in

the

A.r values adjacent

to the

junction.

This rate of storage

gives

the rate at

which the surfaces

rise

in all

these

Ax

values. This is

not an accurate

technique

but the complication

of

the situation

is

such

that no other

method

can be

proposed.

It is

likely to delay

the

passage

of a

wave

through the

junction

and to flatten

it. However,

it has the ability

to receive

flow from

a

pipe

located above

the

water surface

and can cope

with supercritical

and subcritical

flow and any surges

that

may be

present.

Any travelling surges

which

may occur

in

the sections

of channel

not

adjacent

to the

junction

can

be

dealt

with using

the

technique

described

in the next

section.

Supercritical

flows entering

a

Ax length

can

be calculated

from

the two characteristic

equations

available

but

subcritical

flows

having only one

characteristic equation

need to

use the depth of

the

A.r length

which

it is approaching

to obtain solutions

of

depth and

velocity.

7.I2 THE UNSTEADY

TRAVELLING SURGE

Before

the analysis

can be

presented, it is necessary to consider

the

problem

of

surge

initiation. Using

complete

characteristic

theory

including

the

E term, it

is difficult

to

decide upon

a test for

the initiation

of a surge. Characteristic

theory

happily

predicts

the formation

of

a

wave

at

a

point

in

the channel

but because

of

the interpolations

performed in the calculation,

it cannot detect

whether the

wave is a shock,

i.e.

a

discontinuity,

or

a normal continuous

wave.

This can be seen

from

Fig.7

.22 in

which

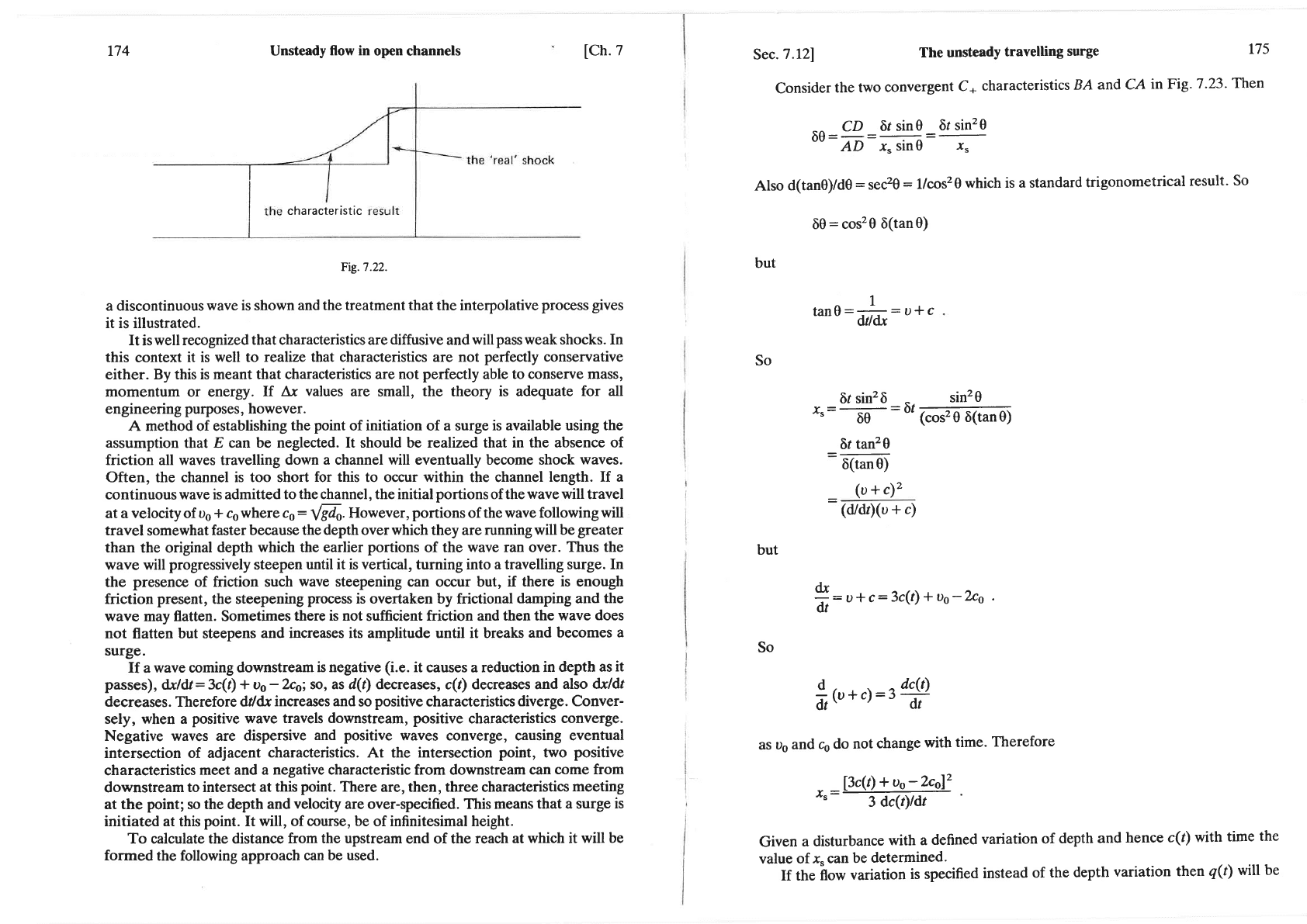
r74

Unsteady

flow in

open

channels

lch.

7

Fig.7.22.

a discontinuous

wave is shown

and the treatment that

the interpolative

process gives

it is illustrated.

It

is

well recognized

that characteristics are

diffusive

and

will

pass

weak shocks. In

this

context

it is

well

to

realize that characteristics are not

perfectly

conservative

either. By this is

meant that characteristics are

not

perfectly

able

to conserve mass,

momentum or energy.

If Al

values

are

small,

the

theory

is adequate for

all

engineering

purposes,

however.

A method of establishing

the

point

of initiation of a surge

is available using the

assumption

that E can

be neglected. It should be realized that in

the absence of

friction all waves travelling

down a channel will eventually become shock

waves.

Often, the channel is

too short

for this

to occur

within

the channel

length. If a

continuous wave is admitted

to the channel, the initial

portions

of the

wave will

travel

at a

velocity

of

ue * c6

where co:1ffi. However,

portions

of

the wave following

will

travel

somewhat

faster

because the

depth

over

which

they are running

will be

greater

than the original

depth

which the earlier

portions

of

the wave ran over.

Thus the

wave will

progressively

steepen

until it

is

vertical,

turning into

a travelling surge. In

the

presence

of

friction such

wave

steepening

can occur but,

if there

is

enough

friction

present,

the steepening

process

is overtaken by frictional

damping and the

wave may flatten. Sometimes

there is not

sufficient

friction

and

then the

wave

does

not flatten

but steepens

and increases its amplitude until it breaks

and

becomes a

surge.

ff

a

wave coming

downstream

is negative

(i.e.

it

causes a reduction

in

depth as

it

passes),

dxldt:3c(t)

+ vo-koi

so, as d(r) decreases,

c(r) decreases

and

also d.r/dr

decreases. Therefore

dtldx

increases and

so

positive

characteristics

diverge. Conver-

sely, when a

positive

wave

travels downstream,

positive

characteristics

converge.

Negative waves are dispersive

and

positive

waves converge, causing eventual

intersection of adjacent

characteristics. At

the

intersection

point,

two

positive

characteristics

meet and a negative characteristic from downstream

can

come from

downstream

to intersect

at

this

point.

There are, then, three characteristics

meeting

at the

point;

so the depth

and

velocity

are over-specified. This means

that a surge

is

initiated at

this

point.

It will, of

course, be

of infinitesimal height.

To calculate the distance

from the upstream

end of the

reach at

which it will be

formed

the following approach

can

be used.

characteristic

Sec.7.12l

The unsteady

travelling

surge

t75

Consider

the

two

convergent

C*

characteristics

BA

and CA

inFig.T

-23- Then

CD

6t

sin0

6t sin20

raH:-:-:-

AD

r,

sinO

-rs

Also

d(tan0yde:

sec20:

1./cos20

which

is a standard

trigonometrical

result.

So

60=cos20

6(tan0)

but

So

tano:

#":

D*

c

6t sin26

^

sin20

t'

=

-5b-

-

ot

Go62e-EGno)

6r

tan20

-5G"0)

(u

+ c)2

-

(d/dr)(u + c)

but

dr

i:'

* c:3c(t)

+

us-Zcs

'

So

d,

. \ -dc(t)

6r(u+c)-Ji

as

uo

and

cs do

not

change

with

time.

Therefore

l3c(t)*uo-Zcolz

x":@

Given

a disturbance

with

a

defined

variation

of

depth

and

hence

c(r)

with

time

the

value of

x, can

be

determined.

If the

flow

variation

is specified

instead

of

the

depth

variation

then

q(r)

will

be

Fox J.A. Transient Flow In Pipes, Open Channels And Sewers

Подождите немного. Документ загружается.