Fattorini H.O., Kerber A. The Cauchy Problem

Подождите немного. Документ загружается.

6.3. Fractional Powers of Certain Unbounded Operators

357

Let now T'> T and consider the domain 52 defined by the inequalities

<T, *0,

ICI <T'

minus the segment [T, T']. In view of (6.2.10),

<C'eaT

IIu(0)II

when

moves over the outer boundary of Q. On the other hand, if

T'J,

C'ealt-TIIIu(T )II

< C'e"(T -T) ll u(T )II

Applying the n constants theorem,

C'e"T'-aTWtr)Ilu(0)11'-wt''IIu(T)II"(l' (

E Q),

where w is the harmonic measure of the segment T < < T' with respect to

2. Taking into account that w

0 and assuming (as we may) that C >_ 1

in (6.2.3),

IIu(t)II <CC'e"Tllu(T)ilm(`) (0<t'<t<T)

if IIu(T)lI<1, where m(t')=inf{w(t); t'<t<T}>0 in view of the maxi-

mum principle for harmonic functions. Consequently, (6.2.5) will be satis-

fied if we take S = (ee-"T/CC')'/m("). This ends the proof.

6.2.3 Remark. Theorem 6.2.1 can be proved under weaker hypotheses. In

fact, letA be an operator in C'+ such that S(t) = exp(tA) admits an analytic

extension

to a sector Z+(4p) satisfying estimates of the type of (4.9.24).

It suffices to excise from 2 all those

with ICI < t" for some t" < t'. This

implies that the results in this section can be applied to the operators A I (p),

A,,, (/3) in Chapter 4 as well as to their one-dimensional versions in Section

4.3.

6.3. FRACTIONAL POWERS OF CERTAIN

UNBOUNDED OPERATORS

Throughout this and following sections, A will be a densely defined linear

operator such that it E p(A) if A > 0 and

IIR(X)II<C/X (A>o).

(6.3.1)

If A E C,(C,O), then (6.3.1) is satisfied. The converse is false (see Example

2.1.5).

Our objective is to define fractional powers A" of the operator A (or,

rather, of - A) with a view towards the study of bounded solutions of the

358

Some Improperly Posed Cauchy Problems

second order equation (6.1.19). To this end we shall employ (variants of) the

formula

(-A)"u= smalr j°°X"-1R(X)(-A)udX.

(6.3.2)

The motivation of (6.3.2) is evident: if A "is a number" a not in X 3 0 (that

is, if A is the operator of multiplication by a in one-dimensional Banach

space) and 0 < Re a < 1, then (6.3.2) becomes an identity, at least if the

branches of X"-' and (- a)" are adequately specified, to wit: ?"-' > 0 for

X > 0, with a similar choice of (- a)'. Two difficulties are obvious: on the

one hand, the formula (6.3.2) will define (- A)"u only for u E D(A) while

we may expect D((- A)") to contain strictly D(A) if, say, 0 < a < 1. On the

other hand, (6.3.2) only makes sense for 0 < Re a < 1. This last objection is

not serious, since this range of a (precisely, the interval 0 < a < 1) is by far

the most significant in applications; however, for the sake of completeness

we shall construct (- A)" for any a by appropriate modifications of (6.3.2).

The formal definition follows.

Let 0 < Re a < 1. The operator K. (with domain D(K") = D(A)) is

defined by (6.3.2), that is,

K"u=

j00X"_'R(X)(-A)udX

(6.3.3)

with the choice of branch of X"-' precised above. Convergence of (6.3.3) at

infinity is obvious from (6.3.1); near zero we use the identity

R(X)(-A)u=u-XR(X)u (6.3.4)

and (6.3.1). The operators K. are extended to other values of a as follows. If

u E D(A2), we can write, making again use of (6.3.4) (this time backwards):

K"u=

sinalr

= sinalr

I'R(x)(-A)dx

slna?T r°°X

_2R(X)A2udA-

sina1r

Au. (6.3.5)

a)IT

But the integrals on the right-hand side actually converge in 0 < Re a < 2,

thus (6.3.5) is an analytic extension of Ku to 0 < Re a < 2. Moreover, if

1 < Re a < 2 and u E D(A2), another application of (6.3.4) yields

K" (-A)u=

sin(a-1)Ir

a-2R(x)A2ud

=sina"r

fI(A"_1R(X)(-A)u+X"-2Au)da

sinalr j0X-2R(X)A2ud=K"u.

(6.3.6)

I I

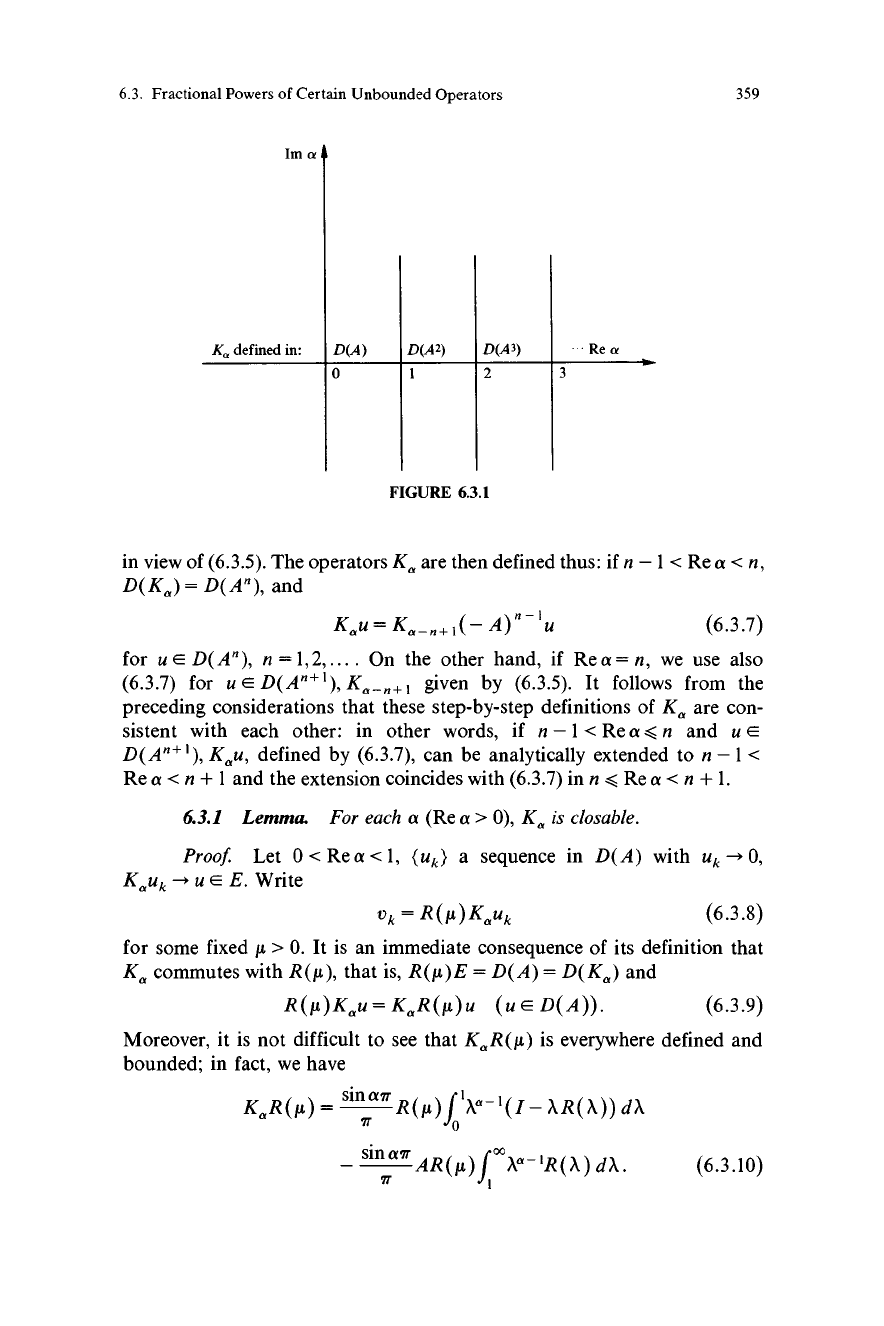
6.3. Fractional Powers of Certain Unbounded Operators 359

Im a

K. defined in: D(A)

D(A2)

FIGURE 6.3.1

D(A3)

- Rea

in view of (6.3.5). The operators Ka are then defined thus: if n - 1 < Re a < n,

D(Ka) = D(A"), and

Kau=Ka_"+I(-A)"-'u

(6.3.7)

for u E D(A"), n =1, 2,.... On the other hand, if Re a = n, we use also

(6.3.7) for u E D(A"+'), Ka_"+, given by (6.3.5). It follows from the

preceding considerations that these step-by-step definitions of Ka are con-

sistent with each other: in other words, if n -1 < Re a < n and u E

D(A"+'), Kau, defined by (6.3.7), can be analytically extended to n -1 <

Re a < n + 1 and the extension coincides with (6.3.7) inn < Re a < n + 1.

6.3.1 Lemma. For each a (Re a > 0), K. is closable.

Proof. Let 0 < Re a < 1, (uk) a sequence in D(A) with Uk - 0,

Kauk -+ u e E. Write

vk=R(t)Kauk

(6.3.8)

for some fixed It > 0. It is an immediate consequence of its definition that

Ka commutes with R(µ), that is, R(t)E = D(A) = D(Ka) and

R(tt)Kau=KaR(u)u (uED(A)). (6.3.9)

Moreover, it is not difficult to see that KaR(t) is everywhere defined and

bounded; in fact, we have

KaR( t) =

sinrazTR(µ)J'V-'(I

- xR(x)) A

sina7rAR(tt)1

Xa-R(X) A.

(6.3.10)

¶ i

360 Some Improperly Posed Cauchy Problems

Accordingly, we obtain taking limits in (6.3.8) that v - 0, hence R(µ)u = 0.

It follows that u = 0, which ends the proof in the range 0 < Re a < 1. The

result for n - 1 5 Re a < n follows recursively from (6.3.7) and from the

facts that Ka and A commute and that p(A) =ft 0. Details are left to the

reader.

Making use of Lemma 6.3.1 we define

(-A)a=Ka (Rea>O).

(6.3.11)

(Note that condition (6.3.1) does not imply that A -' exists, or even that A is

one-to-one; thus we cannot reasonably expect to be able to define (- A)a

for Re a < 0.) We prove next some results that show that (- A)' satisfies (at

least to some extent) properties that should be expected of a fractional

power.

6.3.2 Lemma.

(-A)'=-A.

Proof. Clearly we only have to prove that K,u = -A u for u E

D(A2). We prove instead the stronger statement

lim Kau = u (u c= D(A)). (6.3.12)

as a - 1 in any sector I arg(a -1)1 >, 9) > fr/2. To see this we note that

Kau-(-A)u=

sinra?r

i(R(x)

I)(-A)udA

= 1I + 12 (6.3.13)

dividing the interval of integration at X = a. Plainly,

Isin air 1

sup II((X+1)RO - I)Aull.

sin(Rea)7r X>

Since X R(X) v - v as A - oo (see Example 2.1.6), 111211 can be made small

independently of a if only we take a large enough. As for I it is easy to see

using (6.3.4) that the integrand is bounded by CARea - ', thus in view of the

factor sin air, 111, 11 -+ 0 as a -* 1. This ends the proof.

The corresponding equality for a= 0 ((- A)° = I) cannot hold in

general, since Kau = 0 whenever Au = 0. However, the equality becomes

true when A` exists. Precisely, we have the following more general state-

ment.

Then

6 .3.3 Lemma. Let u E D(A) such that AR(A)u - 0 as A - 0+.

limKau=u

as a -* 0 in any sector I arg a I < q < 77/2.

6.3. Fractional Powers of Certain Unbounded Operators 361

Proof. We have

K"u -

u=-sinalr xa_I(R(x)Au+Xi

)dA

sin

/'0O A"

((A+ l)R(A)u

- u) dA

A+i

=J1+J2,

where J, and J2 arise once again from division of the interval of integration

at A = a. To estimate the second integral we observe that (A+ 1)R(A)u - u

= R(X)(Au + u) and use (6.3.1): it follows that IIJ211 - 0 as a - 0 due to the

factor I sin as I. In the matter of J we observe that

II(A+1)R(A)u-ull<C+IIR(A)ull.

The portion of the estimate for J, corresponding to the constant C obviously

tends to zero when a - 0 in the sector. On the other hand,

Isina7rl f" ARe"

IIR(A)uIIdA=

Isinal

aARe"

IIAR(A)ulldA

A+1 1r

A + I

IsinaIrl

max IIAR(A)ull,

sin(Rea)7r 0-, X_< u

thus we can make IIJ1II arbitrarily small by taking a small enough. This

completes the proof.

An essential property in any definition of fractional power is the

additivity relation (- A)"+'a = (- A)(- A) 'a. In the present level of gener-

ality this identity may not necessarily hold; however, we have the following

result, which is nearly as strong.

6 .3.4

Lemma. Let Re a, Re/3 > 0. Then

(_ A)"+a = (- A)"(-

A)'8.

(6.3.14)

Proof We start by proving that

K"K,qu = K"+su (u E D(A2),Re(a+/3) < 1). (6.3.15)

Noting that Ku E D(A) = D(K"), we can write

K"Kau=

sin air sinfir r

(6.3.16)

the integral being absolutely convergent in the sector A, p. > 0 (to estimate

the integrand near the origin we use the the resolvent equation as we did in

(6.3.3). We make now a few modifications in (6.3.16). The first one is

described as follows. Divide the domain of integration into the two infinite

362 Some Improperly Posed Cauchy Problems

triangles tt 5 A and A S µ and perform the change of variables µ = Aa in the

first integral, A = ag in the second. Interchanging A and µ in the second

integral, we obtain

KaKu=

sinaor sin #77 rl(a"_1

+a#-1)A«+/t-'R(Aa)R(A)A2udada.

0 0

(6.3.17)

We use next the second resolvent equation(3.6) and (6.3.4), obtaining

R(Aa)R(A)A2u= 1 I a(R(A)-aR(Aa))(-A)u

(6.3.18)

and replace in the integrand. Due to the factor (1- a) the two resulting

integrals cannot be separated; however, we can write (6.3.17) as the limit

when p - 1- of

sinalr sin/i?r

paa-1

+ap-1

A«+# ,R(A)(- A)udadA

IT 0

sinair sin#iT / (JPaa+apxa+a-1R(Aa)(-A)udadA.

JO JO

1-0

(6.3.19)

Finally, we integrate first in A in the second integral (6.3.19) making the

change of variable Aa = it and again changing tt by A. We obtain

KaKsu=C(a,/3)J

°°Aa+p-'R(A)(-A)ud

A, (6.3.20)

where

sinalr sin/3r

1(ls-'+as-'-a-a-0-0

17 17

1-a

do.

It remains to evaluate C(a, /3). This can be done directly (using the

geometric series for (1- a) -1) or better yet by means of the following trick:

if we take E = C, A = - 1, then Ka =1 for all a; thus KaKp =1 and it

follows from (6.3.20) that

C(a,/3)=

sin(a+/3)77

whence (6.3.15) follows. Since Kau is an analytic function of a in 0 < Re a < n

for u E D(A"), it is clear that (6.3.15) can be extended to 0 < Re a < 1,

0 < Re/3 < 1 (with no conditions on a + /3) for u E D(A2). More generally,

if 0 < Re a, Re /3 < n (n >- 1), then

KaKsu = Ka+pu (u E D(A2n)). (6.3.21)

Given a (n -1 < Re a < n) and m >, n fixed, denote by K« the

restriction of Ka from its rightful domain D(A") to D(Am). Clearly Ka is

6.3. Fractional Powers of Certain Unbounded Operators

363

closable and K c Ka. We actually have

K« = K. = (- A) a.

(6.3.22)

To see this, let u E D(K"), (Uk) a sequence in D(A") with

Uk - U, K-"uk -

K"u, and (µk) a sequence of real numbers with µk - oo. Making use of the

fact that (µkR(µk)) is uniformly bounded and converges strongly to the

identity as k - oo, it is not difficult to see that

(

m-n

uk = uk' uk,

Kauk = K'(µkR(µk))

nuk

(µkR(AAm- nKauk'

Kau

as k - oo; since Uk E D(Am) = D(Ka), u e D(Ka), and Kau = Kau, show-

ing that (6.3.22) holds.

We have already noted in (6.3.10) that the operator KaR(i) (µ > 0)

is everywhere defined and bounded when Re a < 1. A look at the definition

of K" for general a in (6.3.7) shows that KaR(t)" is everywhere defined and

bounded when Re a < n. It follows easily from (6.3.9), (6.3.7) and the

definition of (- A)" that if u E D((- A)") = D(Ka), then

K"R(µ)"=(-A)"R(µ)"u=R(µ)"(-A)"u,

(6.3.23)

again for Re a < n.

We can now complete the proof of (6.3.14). Let Re a, Re,l3 < n (so

that (6.3.21) holds) and let u E D((- A)'(- A)fl), (µk) a sequence of

positive numbers with µk - 00

Since (AkR(tk))2n -> I strongly as k - 00, it

follows that (tkR(tk))2nu - u, whereas, in view of (6.3.21) and (6.3.23),

Ka+/3(;'kR(Ak))2nU = K"Kf(µkR(µk))Znu

= K.(AkRGtk))n(K',6UkRGtk))nu

(AkR(JUAnKa(µkR(AA"(-

A)%

(11kR(µk))2n(-A)a(-A)'u- (-A)a(-A)ou

ask -goo.

It follows that u E D((- A)"+#) and (- A)"+au = (- A)"(- A)au so that

(- A)"(- A)" (z (- A)"+,8; taking closures, it results that (- A)"(- A)sc

(- A)"+fl. The reverse inclusion follows if we note that (- A)"(- A)RD

KaKI = Ka+/3 and take the closure of the right-hand side.

We note an obvious consequence of Lemma 6.3.4; if we set a =,13 =1

and make use of Lemma 6.3.2 and of the fact that (since p(A)*0) A2 is

closed, we obtain K2 = A2 = (- A)2. Similarly,

Kn=(-A)n (6.3.24)

as we have the right to require from any definition of fractional power.

364 Some Improperly Posed Cauchy Problems

Some improvements on several of the preceding results are possible

when 0 E p(A) (which is not implied by (6.3.1)). Also (as we shall see in

next section), fractional powers can be defined in this case in a less ad hoc

way by means of the functional calculus for unbounded operators (see

Example 3.12).

The first obvious consequence of the existence of A -' is that we may

construct fractional powers of - A for exponents in the left half plane: in

fact, if n - 1 < Re a < n, n = 0,1,..., we define

(- A)- a= (-A )n-a(_

A)-".

(6.3.25)

It follows easily from (6.3.10) that (- A)flA-' is bounded if 0 < Rep < 1,

thus (- A)-" is bounded if Re a < 0. As a consequence of (6.3.24), (- A)-

assumes the correct values for a =1, 2,... .

In view of (6.3.25) the domain of the operator (- A)"(- A)-"

contains D(A) (note that D((- A)IO) D((- A)k) for Re/3 < k). Applying

Lemma 6.3.4, we obtain (- A)"(- A)-" = (- A)"(- A)"-"(- A)" c

(- A)"(- A) " = I. Since (- A)-' is bounded and (- A)" is closed,

(- A)-"E C D((- A)") and

(-A)a(-A)-a=l. (6.3.26)

We use now the fact (already pointed out in the proof of Lemma 6.3.1) that

K" and A -' (thus any power of A -') commute; then, if u E D(An) c

D((- A)"), (- A)-"Kau = (- A)-"(- A)"u = u; since (- A)" = K", this

equality must actually hold for u E D((- A)"), that is

(-A)-"(-A)au=u (uED((-A)a)).

Taking (6.3.26) into account,

(-A)-a=((-A)a)-'

(Rea>0O).

(6.3.27)

We can improve (6.3.14) significantly in the present case as follows.

Let BI, B2 be two operators possessing everywhere defined inverses Bj ' and

and let BI C B2. Then we show easily that BI = B2. If Re a > 0,

Rep > 0, we can apply this argument to Bi = (- A)"(- A)p (B-'=

(- A)-#(- A)-") and B2 = (- A)"+' (B2 ' = (- A)- ("+T)) observing that

(6.3.14) implies thatB, c B2. We obtain

(-A)"+s=(-A)a(-A)'s

(6.3.28)

for Re a, Rep > 0. We note that (6.3.28) cannot be extended to all values of

a and /3: while (- A)-"+" = (- A)° = I, (- A)-"(- A)' is only defined in

D((- A)"). The equality holds, of course, if Re a, Rep < 0.

For the proof of the following results we only assume (6.3.1) (plus

additional hypotheses as stated).

6.3.5 Example.

Let A be self-adjoint in the Hilbert space H, P(dX) the

resolution of the identity associated with A (note that (6.3.1) simply means

6.4. Fractional Powers of Certain Unbounded Operators

365

in this case that a (A) c (- oo, 0]). Then

(-A)"u=f0+(-x)aP(dx)u (uEE,Rea>0), (6.3.29)

where (- A)" is defined as in the beginning of this section and (6.3.29) is

interpreted by means of the functional calculus for unbounded functions of

X (Dunford-Schwartz [1963: 1, Ch. X]). Formula (6.3.29) defines (- A)' as

well when Re a < 0, although (- A)" may be unbounded too. ((- A)" is

bounded for Re a < 0 if and only if A is invertible with A -' bounded.)

Equality (6.3.28) holds if a, $ belong to the right (left) half plane.

6.3.6

Example. Moment inequalities.

Let 0 < a < /3 < y. Then there exists

a constant C = C(a, a, y) such that

II( - A)'ull < CII(-

A)7ull(P-a)/(Y-a)II(-

A) aull (y-a)/(Y-a)

(6.3.30)

for u E D((- A)Y). In particular, if we place ourselves under the hypotheses

of Example 6.3.5, inequality (6.3.30) holds with C = 1.

6.3.7

Example. Let A be bounded (resp. compact). Then (- A)" is

bounded (resp. compact) for Re a > 0.

6.4.

FRACTIONAL POWERS OF CERTAIN UNBOUNDED

OPERATORS (CONTINUATION)

We place ourselves again under the sole hypothesis (6.3.1), that is, we do not

assume existence of A -'.

6.4.1

Lemma. Assume that A satisfies (6.3. 1) for some C > 0. Then

R(X) exists in the sector

2+(T -)

= (X; largXl < T, X * 0),

where q) = aresin(1/C), and for every q)', 0 < q7' < q) there exists a constant C'

such that

IIR(X)II < C'/IXI

(X E +(v )).

(6.4.1)

The proof is rather similar to that of Lemma 4.2.3. The power series

of R(X) about A0 > 0,

R(A)= Y_ (X0

A)JR(A0)'+t

j=0

converges in IX - A 0I < A 0 /C and the union of all these circles is the sector

2+((p). Now, let T' < q),

A0 = IAI/cosarg A. Then we have

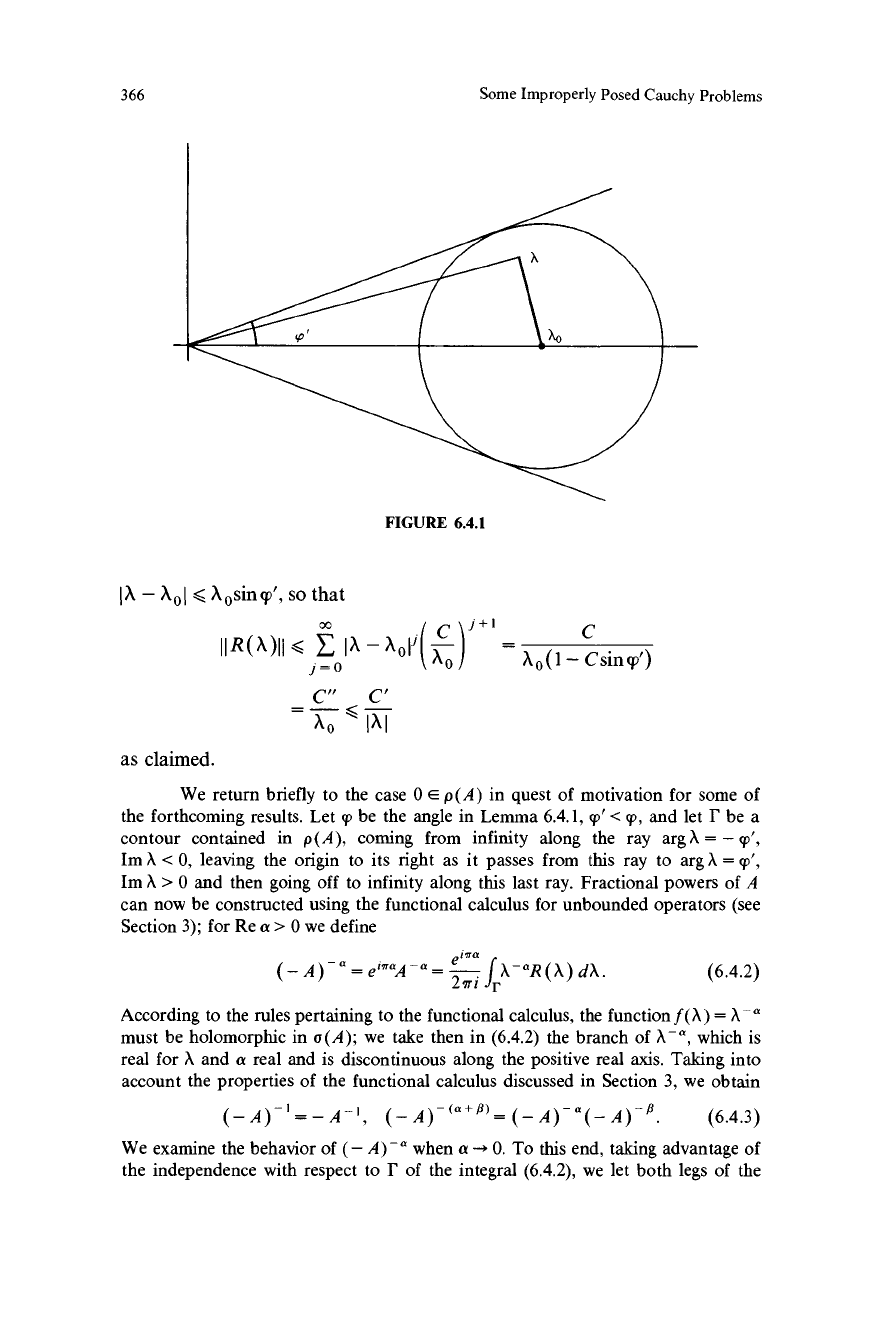
366

Some Improperly Posed Cauchy Problems

FIGURE 6.4.1

IX - Xoi < X0sinT', so that

1+'

1IR(X)i1 < E ix - X01i

_ x0(1- CsinT')

j=0

A0 < Ixi

as claimed.

We return briefly to the case 0 (=- p (A) in quest of motivation for some of

the forthcoming results. Let q) be the angle in Lemma 6.4.1, rp' < (P, and let 1 be a

contour contained in p(A), coming from infinity along the ray arg A = - qp',

Im A < 0, leaving the origin to its right as it passes from this ray to arg A = q)',

Im A > 0 and then going off to infinity along this last ray. Fractional powers of A

can now be constructed using the functional calculus for unbounded operators (see

Section 3); for Re a > 0 we define

tma

(-A) °

=e'vaA-a= e

. f X-"R(A) dX. (6.4.2)

27rt r

According to the rules pertaining to the functional calculus, the function f (A) = A

must be holomorphic in a(A); we take then in (6.4.2) the branch of A-a, which is

real for A and a real and is discontinuous along the positive real axis. Taking into

account the properties of the functional calculus discussed in Section 3, we obtain

(-A)-'=-A-', (-A)-(a+R)=(-A)-a(-A)-s.

(6.4.3)

We examine the behavior of (-

A)-a when

a - 0. To this end, taking advantage of

the independence with respect to I of the integral (6.4.2), we let both legs of the