Fattorini H.O., Kerber A. The Cauchy Problem

Подождите немного. Документ загружается.

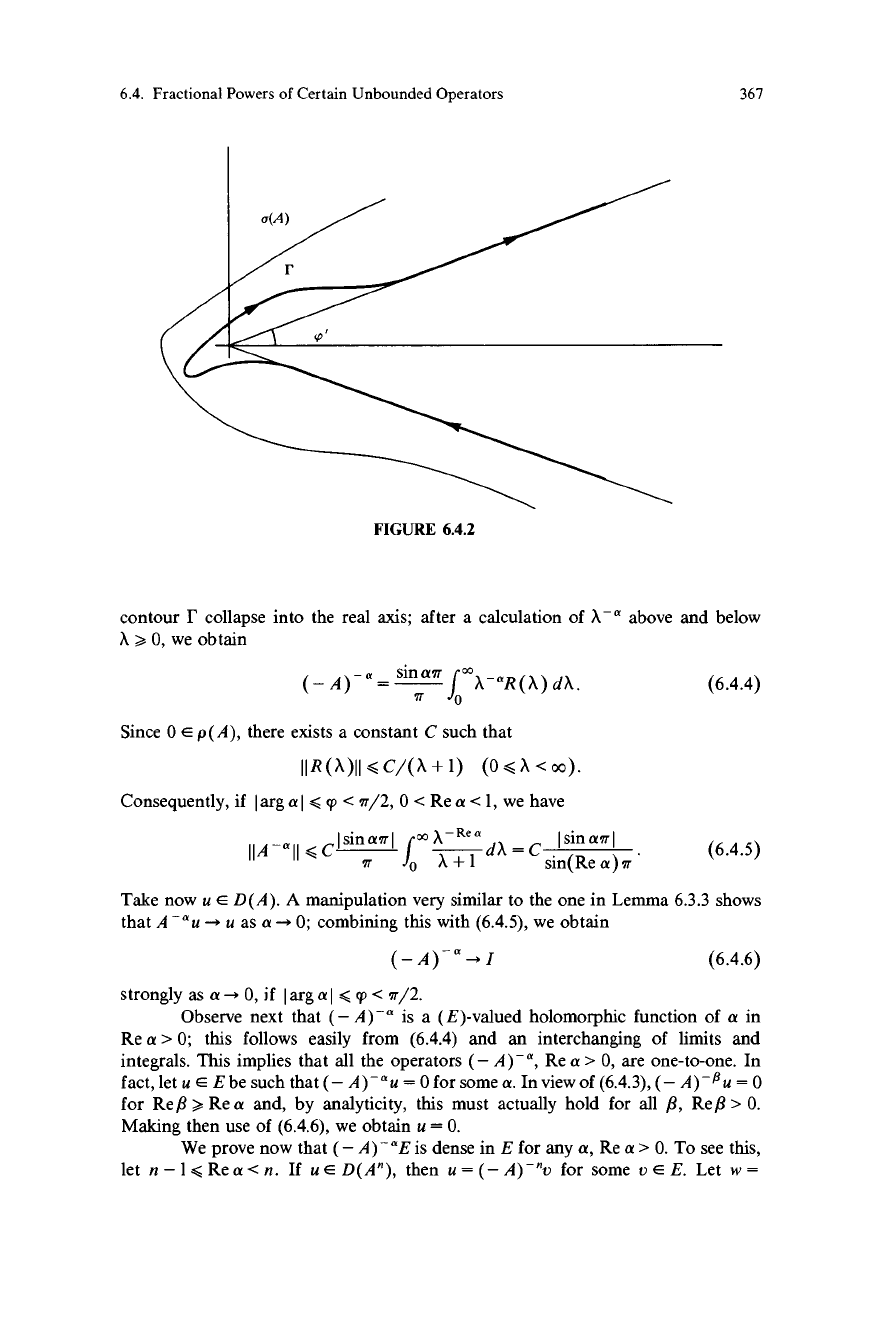
6.4. Fractional Powers of Certain Unbounded Operators

367

FIGURE 6.4.2

contour 1' collapse into the real axis; after a calculation of A-" above and below

A > 0, we obtain

"_ sina7r

A "R

- 4

6 4 4

( )

)

(

( . . )

Since 0 E p (A), there exists a constant C such that

IIR(X)II<C/(A+l) (0<A<oo).

Consequently, if

I arg a I < q < 9T/2, 0 < Re a < 1, we have

Isina7rI

00 A-Re°

_ IsinaITI

IIA II<C

(6 5)

X+I

sin(Rea)IT

Take now u E D(A). A manipulation very similar to the one in Lemma 6.3.3 shows

that A -"u - u as a - 0; combining this with (6.4.5), we obtain

(-A)-"->I (6.4.6)

strongly as a- 0, if I arg a < q< IT/2.

Observe next that (- A)-' is a (E)-valued holomorphic function of a in

Re a > 0; this follows easily from (6.4.4) and an interchanging of limits and

integrals. This implies that all the operators (- A)-', Re a > 0, are one-to-one. In

fact, let u E Ebe such that (- A)-"u = 0 for some a. In view of (6.4.3), (- A)-Pu = 0

for Re$ > Re a and, by analyticity, this must actually hold for all fl, Re$ > 0.

Making then use of (6.4.6), we obtain u = 0.

We prove now that (- A)-"E is dense in E for any a, Re a > 0. To see this,

let n-1<Rea<n. If uED(A"), then u=(-A)-"v for some vEE. Let w =

368

Some Improperly Posed Cauchy Problems

(- A)'-"v. Then

(-A)-aw=(-A)-a(-A)a-"v=(-A) "v=u.

Since D(A") is dense in E our assertion follows.

We complete finally our definition of fractional powers by setting

(-A)a=((-A)-a)-'

(6.4.7)

for Re a > 0; in view of the preceding observations, (- A)' is closed and densely

defined and all the properties proved in the previous section (e.g., (6.3.28)) follow

immediately.

For a near Re a = 0, we define (- A)" _ (- A)(- A)"- 1; it is easy to see

that this definition is consistent with the previous one wherever their ranges of

applicability overlap.

We note, finally, that this construction of fractional powers coincides with

the more general one developed in the previous section whenever both can be

applied; this can be immediately seen comparing (6.4.4) with (6.3.3).

It turns out that the assumption that 0 e p(A) leaves aside some important

applications and is better omitted. However, the functional calculus just developed

in this particular instance is an important heuristic guide even in the general case.

For instance, assume that we want to show that - (- A)' e C2+ by defining

S(t; - (- A)') directly in terms of R (X). Clearly, the right definition would be

S(t; -(- A)a) =S(t; - e-ImaAa)

f exp(-te-"'aV)R(X)dX

27ri r

(6.4.8)

which makes sense, say, if 0 < a < i (and the angle q)' is small enough). It is not

difficult to see that we can collapse the path of integration into the real axis in the

same way used to obtain (6.4.4); the result is formula (6.4.9) below, which has a

meaning as well when 0 E a(A).

Let a be real. Define an operator-valued function by

Sam= f

sinira)R(X)dX,

(6.4.9)

for

complex. In view of (6.4.1) the integrand is O(I X I"

') near the origin,

and dies down exponentially at infinity if

(cosira)Re > (sin

(6.4.10)

If is to be defined at least for real, we must have

0 <a< z.

(6.4.11)

On the other hand, if (6.4.11) is verified, inequality (6.4.10) will hold in the

sector

I arg

I < ,/i, where

= arctg(coslra/sin7ra) = ir('-z - a).

(6.4.12)

The most important case in applications, however, is a = z . Here (6.4.9)

becomes

1 c

S112(fl f sin2t'/2R(X) dA,

(6.4.13)

6.4. Fractional Powers of Certain Unbounded Operators

369

but the integral is divergent at infinity even if = t is real. To overcome this

difficulty we modify (6.4.13) by an integration by parts as follows:

Sl/2(t)= f'h(t,X)R(A)2dX (t>0), (6.4.14)

where

h(t, X) = 1 f'sinta'/2do

= 22 (sin V/2t

- t; /2cos X'/2t),

Irt

and it is easy to see that, if S > 0 there exists a constant C = Cs such that

Ih(t,X)I

0), Ih(t,X)I < CtXI/2 (0<

<1)

(6.4.15)

for t , S. This obviously implies that

IISI/2(t)II,c+c1t (t,s,0),

(6.4.16)

where C, C' may in principle depend on S. In case 0 E p (A), we may use the

first estimate (6.4.15) only, thus C' = 0 in (6.4.6).

Taking advantage of the estimates (6.4.15), continuity of h with

respect to t and the dominated convergence theorem in (6.4.14), we can

prove continuity of SI/2(t) in t > 0 in the norm of (E). On the other hand,

if u E D(A), we have

Sl/2(t)u-u=limo ! fNsintA'/2(R(X)u-Xu)dX

f'sin s;'/2(R(x)u- -1u) dX

+ - fi k sintX'/2R(X)AudX->0

ast-*0. (6.4.17)

Although is actually strongly continuous at the origin, we will not

prove this directly, as a much stronger result follows from estimates on the

resolvent of - (- A)'/2 and the theory in Chapter 4.

We proceed to compute the resolvent. Let µ be a nonzero complex

number in the sector (77 - 9))/2 < arg t 5 7r/2 so that - µ2 belongs to the

sector 2+(y -) in the statement of Lemma 6.4.1. Write

Q(µ)=-(µI-(-A)1/2)R(-µ2).

(6.4.18)

Since D((- A)'/2) 2 D(A), Q(µ) is everywhere defined and bounded. It

was observed in the proof of Lemma 6.3.1 that R(X) and K. commute for

any a (0 < a < 1) and this implies that R(X) and (- A)' = K, commute as

well; hence, if u E D((- A)t/2),

Q(µ)u=-R(-µ2)(µI-(-A)1/2)u.

(6.4.19)

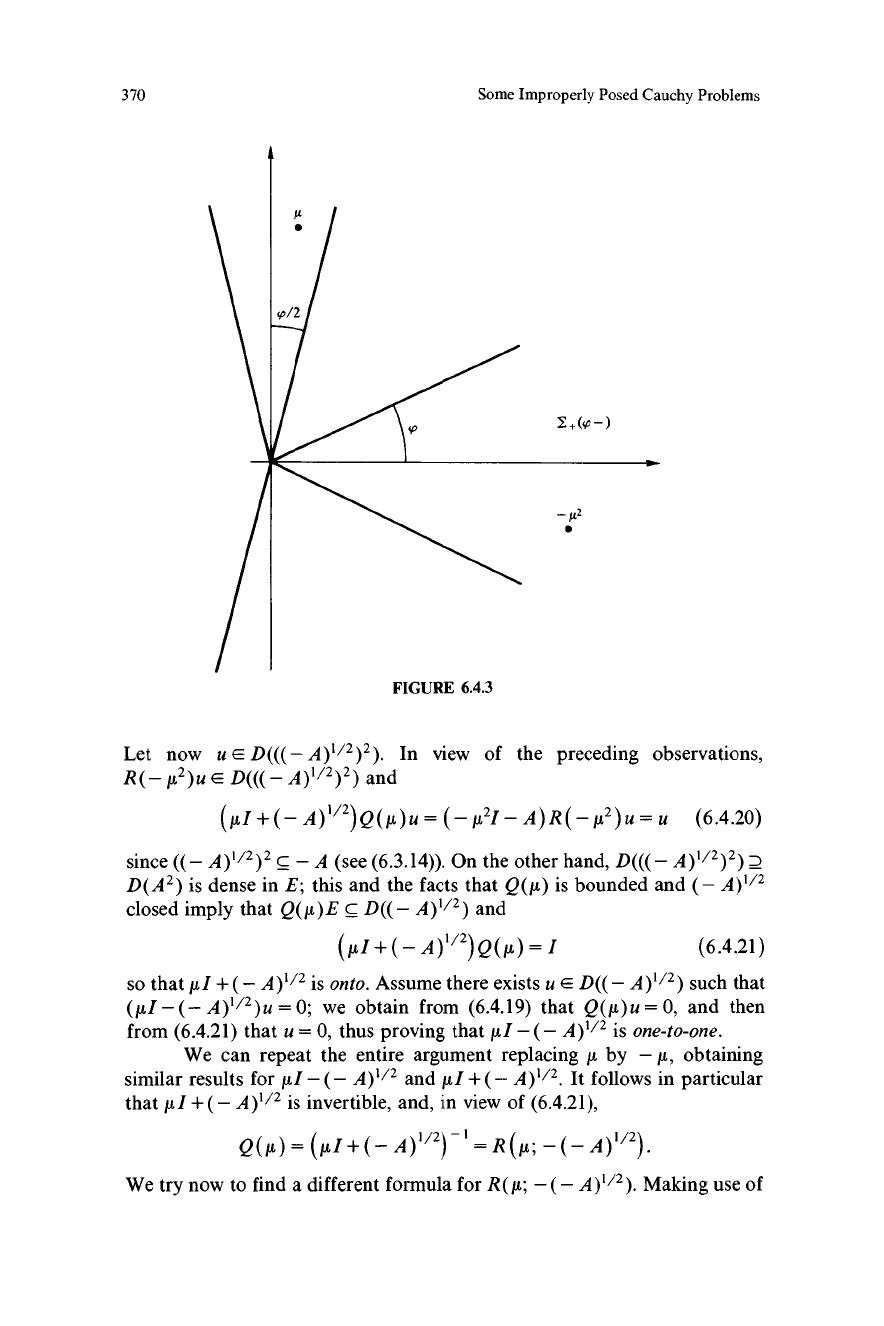
370

Some Improperly Posed Cauchy Problems

FIGURE 6.4.3

Let now u E D(((- A)'/2)2). In view of the preceding observations,

R(- µ2)u E D(((- A)'/2)2) and

(µI+(-A)112)Q(µ)u= (-µ2I-A)R(-µ2)u=u

(6.4.20)

since ((- A)'/2)2 c - A (see (6.3.14)). On the other hand, D(((- A)'/2)2) Q

D(A2) is dense in E; this and the facts that Q(µ) is bounded and (- A)'/2

closed imply that Q(µ)E C D((- A)'/2) and

(µI +(- A)'12)Q(µ) = I

(6.4.21)

so that µI + (- A)'/2 is onto. Assume there exists u E D((- A)'/2) such that

(µI -(- A)'/2)u = 0; we obtain from (6.4.19) that Q(µ)u = 0, and then

from (6.4.21) that u = 0, thus proving that µI -(- A)'/2 is one-to-one.

We can repeat the entire argument replacing µ by - µ, obtaining

similar results for µI - ( - A)'/2 and µI + (- A)'/2. It follows in particular

that µI + (- A)'/2 is invertible, and, in view of (6.4.21),

Q(µ) _ (µI+(-

A)'/2)-'

= R(µ; -(- A)' 2).

We try now to find a different formula for R (µ; - ( - A )1/2). Making use of

6.4. Fractional Powers of Certain Unbounded Operators

371

(6.4.18) and (6.3.3), we obtain

R(µ;-(-A)"2)=-µR(-µ2)- 1

X-1/2R(X)AR(-µ2)dX.

Using the second resolvent equation in the form

R(X)AR(-µ2) = -(x+µ2)-'(XR(X)+µ2R(-µ2))

and an elementary integration by residues, the formula

1/2

R(µ; -(-A)'/2)= _ f

+µ2R(X)dA (6.4.22)

results. Since the right-hand side of (6.4.22) can be extended analytically to

Re a > 0 from its original domain of definition, we obtain by an application

of Lemma 3.6 that every µ in the right half plane belongs to p ( - ( - A )1/2)

and that (6.4.22) holds in this larger domain. The region of existence of

R(µ; -(- A)'/2) can be further enlarged as follows. Let T be the angle in

Lemma 6.4.1, 0 < T' < q,; in view of (6.4.1) the domain of integration in

(6.4.22) can be deformed into the ray arg A = - T', Re A > 0, thus extending

analytically the right-hand side to the slanted half plane Re(µe','/2) > 0;

since q)'< q2 is arbitrary, an extension to Re(µe" /2) > 0 is obtained. A

similar argument involving rays arg A = (p', Re A >, 0 takes care of the region

Re(µe-'q'/2) < 0. Finally, an estimation of the integrals yields

JR(µ'-(-A)'/2)JJ'<C/lµl

(µE2+((IT+92')/2))

(6.4.23)

for every q2', 0 < q2'< q2, where C may depend on q2'. Details are very similar

to those in the proof of Theorem 4.2.1 and are thus omitted.

We apply now Theorem 4.2.1 itself: according to it, - (-A )1/2 E

l (((p/2) - ). Let S(t; - (- A)1/2 ) be, in the usual notation, the semigroup

generated by - (- A )1/2. We wish to prove that

S(t; -(-A)1/2)=S,/2(t) (t>1 0). (6.4.24)

In order to do this, we take u E D(A) and make use of (6.4.16) and (6.4.17)

(which implies that S,/2(t)u is continuous in t > 0.) An easily justifiable

interchange of order of integration shows that for any µ > 0,

f oe-'"S,/2(t)udt=lu+

r f

e-AtsintA'/2dt II R(X)u- 1 u) dX

o o /`

R(A)udX

o A+µ2

= R

(µ; _ (_ A )

1/2)

(6.4.25)

Since the same equality must hold for S(t; -(- A)'/2)u, we obtain that

372 Some Improperly Posed Cauchy Problems

S(t; -(- A)'/2)u and S,/2(t)u coincide for u E D(A) for t > 0. As both

operators are continuous (S,12(t)u because of the comments following

(6.4.17)), we obtain (6.4.24) if we define S,/2(0) = I, using uniqueness of

Laplace transforms and denseness of D(A).

We collect our results.

6.4.2 Theorem.

Let A

satisfy (6.3.1).

Then -(-A )1/2 E

(((V/2)-), where (p = aresin(l/C). If 0 E p(A), we have

(t>0).

(6.4.26)

Equality (6.3.14) can be improved for a = z to:

((- A)'/2)2

= (-

A)I/2(- A)'/2 = - A.

(6.4.27)

In fact, if µ is a complex number such that - µ2 E p(A), we have seen that

both µ and -µ belong to p(-(- A)'/2); thus

D(((- A)l/2)2)

R(µ; -(- A)1/2)R(-µ; -(- A)'/2)E

=-R(- L2;A)E=D(A).

This equality, combined with (6.3.14), implies (6.4.29).

6.4.3

Example.

Let A be dissipative (so that A is m-dissipative by virtue

of Assumption 6.3.1). Then -(- A)'/2 is m-dissipative.

6.4.4 Example.

Let E be a Banach lattice (see Section 3.7) and assume

that A is dispersive with respect to a (any) proper duality map 0. Then the

same is true of - (- A)'/2.

6.5. AN APPLICATION: THE INCOMPLETE

CAUCHY PROBLEM

It was pointed out in Section 6.1 that if A is a self-adjoint operator in a

Hilbert space such that - A is positive definite (see Example 6.1.5), then for

every u E D((- A

)1/2) there exists a unique solution of

u"(t)+Au(t)=0, (6.5.1)

u(0)=u, supllu(t)II<oo, (6.5.2)

t>0

which is given by u(t) = exp(- t(- A)'/2)u. We prove here a partial

generalization of that result with the help of the theory of fractional powers

developed in Sections 6.3 and 6.4.

Let A be an invertible operator satisfying the assumptions in Section

6.3; precisely, let [0, oo) c p(A) and

IIR(X)II <C/A (A>0). (6.5.3)

6.5. An Application: The Incomplete Cauchy Problem

373

Take u E D(((- A)'/2)2) = D(A) (see (6.4.27)) and let

u(t) = S,/2(t)u, (6.5.4)

where

is the semigroup defined in Section 6.4. In view of (6.4.16),

is a solution of (6.5.1), (6.5.2). Moreover, the solution is unique. To

prove this, let v(-) be an arbitrary bounded solution of (6.5.1) such that

v(0) = 0, and define w(t) = R(tt)v(t) for some p > 0 fixed. Then w(t) is

bounded in

t >, 0 together with w"(t) = - Aw(t) = - AR(IL)v(t) and

(-A )'/2w(t) = (- A)'/2R(t)v(t); clearly, IIw'(t)II = 0(t) as t - oo. De-

fine z(t) = w'(t)+(- A)'/2W(t). Then it is easy to see that z(t) is a genuine

solution of

z'(t) _ (- A)'/2Z(t) (t > 0), (6.5.5)

where z(0) = w, = w'(0) and 11z(t)II = O(t), Ilz'(t)II = O(t) as t -* oo in view

of the preceding estimates on w(.). Define

i(A) = f*extz(t) dt

(ReA <0).

(6.5.6)

The function i is obviously analytic in the left half plane. Integrating by

parts and making use of (6.5.5) and of the bounds on z, z', it is easy to see

that i(A) E D((- A)'/2) and

(XI+(-A)'/2)2(A)=-w, (ReA<0).

(6.5.7)

Hence the function z(A) is an analytic continuation of - R (X; - (- A )' /2 ) w

(which is defined in 2 +(z (7r + (p)-)) to the whole complex plane minus the

origin. In view of inequality (6.4.23), this continuation may have at most a

pole of order 1 at A = 0, so that we have

R(A;-(-A)1/2)w,=f(A)+u/A (A.0),

where f is an entire E-valued function and u E E. By virtue of (6.4.23) and

of the inequality

Ilz(A)II <C/IReAI

(ReA <0),

which follows immediately from (6.5.6), we see that f dies down at infinity

(hence vanishes identically), thus R(A; -(- A)'/2)w, = u/A. Making use of

the definition of resolvent, we deduce that

(XI+(-Al/2)u/A=u+(-A)'/2U/A=w1

obtaining that (- A)'/2u = 0 and u = w1, hence (- A)'/2w1 = 0. It follows

that

SI/2(t)w1= w,

(t.0).

(6.5.8)

We consider now the function y(t)= w'(t)-(- A)'/2w(t). Since (as we

easily verify) y is a genuine solution of y'(t) _ -(- A)'/2y(t) in t >, 0,

374

Some Improperly Posed Cauchy Problems

y(O) = w,, we obtain

y(t)=w'(t)-(-A)1/2w(t)=S,/2(t)wl=w,

(t>0).

(6.5.9)

Applying Sl/2(t) to both sides,

(Sl/2(t)w(t))'= wl (t >- 0),

hence

S112(t)w(t) = twl,

(6.5.10)

keeping in mind that w(0) = 0. Since the left-hand side of (6.5.10) must be

bounded, w1 = 0, thus S,12(t)W(t) = 0 in t >, 0. By virtue of Remark 4.2.2,

each S112(t) is one-to-one and we finally obtain that w(t) = 0, hence

v(t) = 0 in t >, 0. This proves uniqueness of solutions of (6.5.1), (6.5.2).

We collect the results obtained.

6.5.1

Theorem.

Let A be a densely defined, invertible operator

satisfying (6.5.3), and let u E D(A). Then there exists a unique solution u(.) of

(6.5.1), (6.5.2). This solution is given by the formula

u(t) =S112(t)u,

(6.5.11)

where S1/2 is a strongly continuous, uniformly bounded semigroup analytic in

the sector 2+((p/2)-), V = aresin(1/C) (C the constant in (6.5.3). The

generator of S1/2 is the operator - ( - A)1 /2.

We note that Theorem 6.5.1 obviously implies that the incomplete

Cauchy problem (6.5.1), (6.5.2) is well posed as defined in Section 6.1: the

solution of this problem satisfies

Ilu(t)II CIIuIi

(t,0),

(6.5.12)

where C does not depend on u.

6.6.

MISCELLANEOUS COMMENTS

Properly and improperly posed problems have been commented on in

Sections 1.7 and 6.1. Although the importance of problems that are improp-

erly posed in one sense or other began to be realized in the forties (see, for

instance, Tikhonov [1944: 1]), substantial progress in their treatment had to

wait until the late fifties. Thus we read in Courant-Hilbert [1962: 1, p. 230]:

"Nonlinear phenomena, quantum theory, and the advent of powerful

numerical methods have shown that "properly posed" problems are by far

not the only ones which appropriately reflect real phenomena. So far,

unfortunately, little mathematical progress has been made in the important

task of solving or even identifying and formulating such problems that are

not "properly posed" but still are important and motivated by realistic

6.6. Miscellaneous Comments

375

situations." Since then, a true explosion of research activity has occurred, as

attested by the size of the bibliography in Payne's monograph [1975: 1],

which is almost exclusively devoted to several types of improperly posed

Cauchy problems.

With the possible exception of the reversed Cauchy problem in

Section 6.1, none of the problems and examples in this chapter would

probably be recognized by specialists as improperly posed, but rather as

properly posed problems whose correct formulation is somewhat nonstan-

dard. We refer the reader to Payne [1975: 1] for examples, results and

general information on ill-posed Cauchy problems, and we limit ourselves to

a few comments on the material in this chapter.

(a) Section 6.2. Theorem 6.2.1, as well as the definition of problems

that are properly posed for bounded solutions, are due to Krein and

Prozorovskaya [1960: 1]. The fact that an improperly posed problem may be

"stabilized" by a priori restrictions on its solutions was already realized by

Tikhonov [ 1944: 1]. The reversed Cauchy problem for the heat equation

with a priori bounds on the solutions was considered for the first time by

John [1955: 11 (where the bound takes the form of a nonnegativity condi-

tion). The Cauchy problem for elliptic equations was studied in the same

vein by John [1955: 2], Pucci [1955: 1], [1958: 11, and Lavrentiev [1956: 11,

[1957: 1].

The inequalities leading to Theorem 6.2.1 can be considerably shar-

pened under more precise assumptions on the operator A. For instance, we

have

6.6.1 Example.

Let A be a symmetric operator in a Hilbert space H, u(-)

a solution of

u'(t)=Au(t) (0<t<T).

Then log I I u(t) II is convex in 0 < t < T; in particular,

Ilu(t)II < I1

u(0)II'-11TIIu(T)II`1T

(0 < t <T).

(6.6.1)

To see this assume first that u (t) * 0 in 0 < t < T. Then

(log IIu(t)112)"= 2{II

u(t)11-2(Au(t), u(t)))'

= -411 u(t)II -4(Au(t),

u(t))2+411 u11-2IIAu(t)II2,

which is nonnegative in view of the Schwarz inequality. To show (6.6.1) in

general it suffices to prove that u(t) vanishes identically if u(T) = 0. If this

is not true, we may obtain by translation an interval 0 < t < h, where

u(h) = 0, u(t) * 0 for 0 < t < h, a contradiction in view of (6.6.1) applied in

[0, h'], h'< h.

Similar logarithmic convexity results have been obtained for various

functions V(u(t)) of solutions of various classes of differential equations.

376

Some Improperly Posed Cauchy Problems

For some references on the subject see Knops-Payne [1971: 1], Levine

[1970: 1], [1970: 2], [1972: 1], [1973: 1], [1973: 2], Agmon [1966: 1], and

Agmon-Nirenberg [1963:

11, [1967:

11. An overview of the subject and

numerous other references can be found in Payne [1975: 1].

As general references in the field of ill-posed problems (some of them

not connected with the Cauchy problem), see Lavrentiev [1967:

1],

Lavrentiev-Romanov-Vasiliev [1970:

1], Tikhonov-Arsenin [1977: 1], and

Morozov [1973: 11.

(b) Sections 6.3, 6.4, 6.5.

The definition of fractional powers pre-

sented here is due to Balakrishnan [1959: 1], [1960: 1]. It generalizes earlier

definitions of Bochner [1949: 1] and Phillips [1952: 11, where A E e+(C, 0)

for some C (so that p(A) D (0, oo) and IIR(X)"II < CA-" for A > 0). Here the

semigroups generated by - ( - A)" can be explicitly written in terms of the

semigroup generated by A by means of the formula

exp(-t(-A)")u= f °°f"(t,s)exp(sA)uds, (6.6.2)

where ,,,(t, s) is the function that makes (6.6.2) an identity in the scalar

case:

f"(t, s) =

Iri

fo 0o

e\se-t"dX.

(6.6.3)

Here to > 0 and the branch of X" is chosen in such a way that A" is analytic

in Re X > 0 and Re X" > 0 there. See Yosida [ 1978:

1 ] for justification of

(6.6.2) and additional details.

The particular case of Balakrishnan's definition where A` E (E)

was discovered independently by Krasnosel'skii and Sobolevskii [1959: 1].

A vast literature on diverse properties of fractional powers and on

their applications to the abstract Cauchy problem exists. See Komatsu

[ 1966: 1], [1967: 1], [1969: 1] [1969: 2], [1970: 1], [1972: 1], Kozlov [1958: 1],

Guzman [1976:

11, Hovel-Westphal [1972:

11, Kato [1960: 11, [1961: 2],

[1961: 3], [1962: 11, Sobolevskii [1960:

1], [1961: 2], [1964: 5], [1966: 2],

[ 1967: 11, [1967: 3], [1972: 11, [1977: 11, Kalugina [ 1977: 1 ], Staffney [ 1976:

11, Volkov [1973:

1], Watanabe [1977: 11, Westphal [1968:

1], [1970:

1],

[1970: 2], [1974: 1], [1974: 2], Yoshikawa [1971: 1], Yoshinaga [1971: 11,

Emami-Rad [1975: 1], Balabane [1976: 1], Sloan [1975: 1], Krasnosel'skii-

Sobolevskii [1962:

1], [1964: 1), Krasnosel'skii-Krein [1957:

11, [1964:

1],

Langer [1962:

1], Nollau [1975:

1], and Lions [1962: 1]. For general

information on fractional powers and additional references see the treatises

of Krein [ 1967: 1 ], Tanabe [ 1979: 1 ], and Yosida [ 1978: 11. We note that the

theory of fractional powers is a particular instance of an operational

calculus, where one tries to define f(A) for a class of functions wide enough

while conserving (inasmuch as possible) desirable properties like (fg)(A) =