Fattorini H.O., Kerber A. The Cauchy Problem

Подождите немного. Документ загружается.

5.7. Approximation of Abstract Differential Equations

317

makes CY a Banach space. The operator Q : E - G' defined by

Qu = (Qiu, Q2u,...)

(5.7.5)

in linear and everywhere defined, and it is also easily seen to be closed. It

follows then from Theorem 3.1 that Q is bounded, that is,

I I Qu l l C' < M II U II E

for all u E E. This is equivalent to (5.7.4).

We say that the sequence (En, Pn), where Pn E (E; En), approximates

E if and only if

lim IlPnulln=llull

(uEE).

(5.7.6)

n-oo

It follows from Theorem 5.7.1 that

IlPnlln<M

(n>, 1). (5.7.7)

A sequence (un), un E En is said to converge to u E E (in symbols, un - u) if

and only if

Ilun-Pnulln-0as n-oo.

(5.7.8)

We note that the convergence relation (5.7.8) defines the element u uniquely:

in fact, if un - u, un - v, we have, in view of (5.7.6),

llu - vll= lint IIP (u-v)lln

n - oo

< lim Ilun - Pnulln+ lim Ilun-Pnvlln=0.

n-oo n- oo

A similar argument shows that

(lull = lim Ilunlln.

(5.7.9)

n- oo

5.7.2 Example. The sequence (En, Pn ), where En = E and Pn = I obvi-

ously approximates E. Convergence according to (5.7.8) is nothing but

convergence in E.

5.7.3

Example.

Let 2 be a bounded domain in I '. For each n =1, 2, .. .

divide 0 into a finite number of disjoint sets

s2 ='Q'n1 U 2n2 U ... U In r(n) (5.7.10)

in such a way that

lim (max diam Stnj) = 0 (5.7.11)

n ->oo1<j.r(n)

and select xnj E U'n j in an entirely arbitrary way. Let E = C(S2)R, En = IIBr(n)

= r(n)-dimensional Euclidean space endowed with the supremum norm

and let Pn: C(F2) -> Rr(n) be defined by

PnU= (u(xn1), u(xn2).... ,U(xn r(n))).

Then {En, Pn} approximates E. A similar result holds for C(S2)c.

5.7.4 Example. Let 2, S2n j be as in Example 5.7.3; assume, moreover, that

each SZn j is measurable. Let E = LP(12) (1 < p < oo) and let En be the

318 Perturbation and Approximation of Abstract Differential Equations

subspace of LP(S2) consisting of all functions that are constant in each S2n

with the norm inherited from LP(2). Define an operator Pn: LP(2) -> En

(Pnu)(x) = mnj(u)

(x E 9nj)'

where mn j is the average of u in Stn j,

m(u) = 1 f u(x) dx,

(5.7.12)

µ'nj a j

µn j the hypervolume of Stn j. By Holder's inequality,

Imnj(u)I <

jIPp(f lu(x)IPdx

I/P

1-nj

so that each P,, is a bounded operator; in fact, Il Pnull

n =

(lull, hence we have

IIPnII<I.

(5.7.13)

and the fact that (En; Pn) approximates E is obvious.

The main problem considered in this section is the following. Let

(En, P,,) approximate E, and let A E (2+(E ), An E C+(En ). Under which

conditions can we assure that

SS(t)u-S(t)u? (5.7.14)

where Sn(t) = exp(tAn), S(t) = exp(tA), and convergence is understood in

the sense of (5.7.8), uniformly with respect to t in one way or other. A

solution is given in the following result; from now on we assume that

(En, Pn) approximates E.

5 .7.5

Theorem.

Let A E (2,(C, w; E), An E C+(C, w; En) (where

C, w do not depend on n). (a) Assume that for each u E E

llSn(t)Pnu-PnS(t)ulln- 0 (5.7.15)

uniformly on compact subsets of t >, 0, where Sn (t) = exp(tAn ), S(t) = exp(tA).

Then, for each u E E,

IIR(A; An)Pnu - PnR(X; A)ull

n '

0 (5.7.16)

uniformly in Re X >, w' for each w' > w. (b) Conversely, assume that (5.7.16)

holds for some A with Re X > w. Then (5.7.15) holds uniformly on compact

subsets of t >, 0.

Proof

Assume that (5.7.15) holds uniformly on compact subsets of

t > 0. Let Re X >, w' > w. Using formula (2.1.10), we obtain

IIR(X;An)Pnu-PnR(X;A)ulln<

re-wtllSn(t)Pnu-PnS(t)ullndt.

(5.7.17)

5.7. Approximation of Abstract Differential Equations

319

Divide the interval of integration at some sufficiently large c > 0; in t > c we

use the fact that II SS(t)Pnu - PPS(t)uII

n <

Ce"t independently of n, while

we take profit of the uniform convergence assumption in

t < c. This

completes the proof of (a).

To prove (b), let A be such that (5.7.16) holds and consider the

(E; En )-valued function

H(s) = Sn(t - s)R(A; An)PnS(s)R(A; A)

in 0 < s < t.

It is easily seen that H is continuously differentiable with

derivative

H'(s) = - Sn(t - s)A,,R(A; A,,)P,,S(s)R(A; A)

+ Sn(t - s)R(A; An)PnS(s)AR(X ; A)

= Sn(t - s)(PnR(A; A)- R(A; An)Pn)S(s)

We apply H' to an element u E E and integrate in 0 < s < t; after norms are

taken, the inequality

IIR(A; An)(PnS(t)-Sn(t)P,,)R(A; A) ulln

<Ce"tJ te-"SII(PnR(A; A)-R(X; A,,)Pn)S(s)uII nds

(5.7.18)

results. Noting that

IIS(s)II < Ce"t, using (5.7.16), and applying the

dominated convergence theorem to the integral, we see that if v E D(A) (so

that v = R(A; A)u),

IIR(A;An)(PnS(t)-Sn(t)PP)vlln-*0

(5.7.19)

as n - oo, uniformly on compacts of t >, 0. But the operators acting on v in

(5.7.19) are uniformly bounded on compact subsets of t >, 0 (by a constant

times (A - w)-'), hence the limit relation must actually hold for all u E E,

uniformly on compact subsets of t >, 0.

On the other hand, we have

R(A; A.)Sn(t)Pn - Sn(t)PnR(A; A) = S.(t)(R(A; An) Pn - PnR(A; A))

(5.7.20)

and

R(A; An)PnS(t)-PnS(t)R(A; A) _ (R(A; An) Pn - PnR(A; A))S(t).

(5.7.21)

We obtain then from (5.7.20) that, for u E E,

II(R(A; A.)Sn(t)Pn - Sn(t)P.R(A; A)) ulln - 0

(5.7.22)

uniformly on compacts of t >, 0. Likewise, it follows from (5.7.21) that,

again for arbitrary u,

II(R(A; An)PnS(t)- PPS(t)R(A; A))ulln -* 0 (5.7.23)

320

Perturbation and Approximation of Abstract Differential Equations

in t >, 0. To see that convergence is uniform on compact subsets of t >, 0, we

observe that S( - ) u, being continuous in t >, 0, must be uniformly continuous

on compact intervals 0 < t < c < oo ; accordingly, given e > 0, we can find a

partition 0 = to < tj < ... < t = c of [0, cl such that II S(t)u - S(t')uII < e

for any two points in the same interval; writing then S(t) = S(ty) u +

(S(ty) u - S(t) u) in (5.7.21) and using the uniform boundedness of

II(R(X; An)Pn - A))S(t)II, the required uniformity property follows.

We combine now (5.7.19), (5.7.22), and (5.7.23) and obtain that

A)ulI - 0 (5.7.24)

uniformly on compacts of t >, 0 so that (5.7.15) follows for any v E

R(X; A)E = D(A) and thus, by denseness of D(A) and uniform

boundedness of IIPnS(t)- it holds for any u E E uniformly on

compact subsets of t >, 0.

The following result shows that the operator A need not even be

introduced in the statement of Theorem 5.7.5 if we impose additional

assumptions on the A.

5.7.6 Theorem. Let An E 3+(C, w; En), where C, w do not depend

on n. Assume that there exists some X with Re X > w such that for each u E E

there exists v E E with

0. (5.7.25)

Assume, moveover that

lim IIXR(X;A,,)Pnu-Pnulln=0

(5.7.26)

for each u E E, uniformly with respect to n. Then there exists A E e+(C, w; E)

such that (5.7.16) holds; a fortiori, (5.7.15) is satisfied uniformly on compacts

Of t>0.

Proof. Taking into account the observations after (5.7.8) and the

equality (5.7.9), we deduce that the element v in (5.7.25) is unique and that

IIvii < liminfliR(X; An)jinllull <C(X - w)-'hull

(5.7.27)

so that v = Q(X) u, Q (X) a linear bounded operator in E; so far we are only

certain of the existence of Q(X) for the value of X postulated in Theorem

5.7.6. That it actually exists for other values of A follows from

5.7.7 Lemma. Let the assumptions of Theorem 5.7.6 be satisfied.

Then (5.7.25) holds for every A in Re X > to (with v depending on A).

Proof. Let A be such that (5.7.25) is verified. Then

II R(A; An)2Pnu - R(A; An)Pnviin < C ( X -

w)-1IIR(A; An)Pnu

- Pnv1I

n -

Since IIR(A; An)Pnv - Pnwlln - 0 with w = Q(X)v = Q(A)2u, we have

5.7. Approximation of Abstract Differential Equations

321

IIR(A; An)2Pnu - P Q(A)2ull

n -

0 as n -* oo. Arguing further in the same

way, we obtain

IIR(A; An)kPnu - PPQ(X )kull

_ 0

(5.7.28)

as n - oo for each fixed u e E, k =1,2,.... We use now formula (3.4): if

lµ-Al<ReX-w,

R(µ; An)

= E

(A-µ)kR(A;

An)k+t

k=0

Making use of all the relations (5.7.28) and of the uniform bound on the

I I R (,N; An) k l 1, we see that (5.7.25) actually holds in

I A - III < Re X - w.

Carrying out this "extension to circles" repeatedly in an obvious way,

Lemma 5.7.7 follows. A consequence of the argument is that (5.7.25) holds

uniformly on compact subsets of Re A > w.

Proof of Theorem 5.7.6.

It follows from the second resolvent equa-

tion (3.6) for each R(A; An) that

Q(A)- Q(µ) = (µ - A)Q(A)Q(t) (5.7.29)

for Re A, Rep > w; incidentally, this equality shows that Q(A) and Q(it)

commute. Let 6A(A) = Q(A)E and let GJt(A) be the nullspace of Q(A). It

follows easily from (5.7.29) that both and % are constant, that is,

lt(X)=6A,

Ot(A)=6J(, (ReA>w).

(5.7.30)

We combine now (5.7.25) and (5.7.26), obtaining

lim

IIXQ(A)u-ull=

lim

a oo

- oo

(lim

IPnAQ)u-uln)

n oo

lim ( lim (I(AR(A; An)Pnu - Pnu)Il n)

X oo

n-oo

+ lim (lim IIAR(A;An)Pnu-APnQ(A)ulln)

Xoo n->oo

= 0 (5.7.31)

for all u e E. Accordingly, if u c= 9L, u = lim AQ(A)u = 0; in other words,

GJt = (0)

(5.7.32)

so that each Q(A) is one-to-one. The limit relation (5.7.31) also implies that

is dense in E since u can be approximated by AQ(A)u E t. The operators

Ax = Q(X)- t are then well defined and have as a common domain the dense

subspace Ji,. We show finally that

XI-AX=ttI-Aµ=A (ReA,Reµ>w); (5.7.33)

to see this we apply A,\Aµ =A

Ax to both sides of (5.6.29). It follows

immediately from the definition of A that every A with Re A > w belongs to

322

Perturbation and Approximation of Abstract Differential Equations

p(A) and

R(X;A)=Q(X).

Using (5.6.28) for any such A,

IIR(X;A)kuII= lim IIPnQ(A)kulln

n-oo

lim IIR(A; An)kPnulln

n- oo

<C(ReX-w)-kllull (ReX>w,k=1,2.... ),

(5.7.34)

where C does not depend on k. Hence A E e, (C, co; E) and the assumptions

of Theorem 5.7.5(b) are satisfied. This ends the proof.

We note a curiosity pertaining to the case where En = E, Pn = I.

5.7.8

Example.

Let Sn

be a sequence of strongly continuous semi-

groups such that for each u E E the sequence Sn(t)u converges uniformly in

0 < t < 8 > 0. Then there exists C, to independent of n such that I I Sn (t )I I

CeWt.

It is of great interest to have conditions insuring the convergence of

the propagators Sn that bear on the operators An themselves rather than on

their resolvents R(A; An). Such conditions are given below (Theorem 5.7.11).

Given a sequence (En; Pn) approximating E and an operator An in each En

with domain D(An), we define @, the extended limit of the An (in symbols,

ex-limn

An or simply ex-lim An) as follows: an element u E E belongs to

D(C?) if and only if there exists a sequence (un), un E D(An) and a

v = & u E E such that un - u and Anun - v, that is,

Ilun - Pnulln - 0,

ILAnttn - Pnvlln - 0

(5.7.35)

as n - oo. In general, we cannot expect 6 to be single valued, even in very

special situations.

5.7.9

Example. If En = E, Pn = I, An = A, then ex-lim An is single valued

if and only if A is closable; in that case, ex-lim An = A.

The following result establishes conditions under which d is single

valued.

5.7.10 Lemma. Assume that there exists co > 0 such that, for each

un E D(An),

II('I-An)unlln%c(X -w)Ilunlln

(5.7.36)

for A > w and n =1, 2,..., the constant c > 0 independent of n. Assume,

moreover, that D(C) is dense in E. Then CT is single valued and

II(XI-(l)ull>c(X-w)Ilull

(X>w).

(5.7.37)

5.7. Approximation of Abstract Differential Equations

323

Proof

If d is not single valued, there exists v E E, v - 0 and a

sequence (un ), un E D(An) such that

llunlln -* 0,

II Anun -

PnvIIn -* 0

as n - oo. On the other hand, if u is an arbitrary element of D(G ), there

exists some sequence (un) and some v (not necessarily unique) such that

(5.7.35) holds. Since

for A>W

and

II(AI-An)(un+Aun)-PP(Xu-v-Xi )IIn-*O

n-'00,

we have

I1au-v-Av11

=limllPP(Xu-v-1\0I1n

=limll(AI-An)(un+Xun)Iln>- hmC(X-W)Ilun+AUnlln

= c(A - W) limllunlln = C(A - W) limllPnulln = c(A - W )IIuII

(5.7.38)

Dividing by A and letting A -* oo, we obtain

IIu -'U11 % cIlull

(5.7.39)

Since D(d) is dense in E, we can choose u with Ilu - vll < cllull, which

contradicts (5.7.39) and shows that (9', is single valued. To obtain inequality

(5.7.37), it suffices to read (5.7.38) for v = 0.

5.7.11

Theorem. Let An E (+(C, to; E,,), where C, W do not depend

on n. Assume, moreover, that E = ex-lim An is densely defined in E and that

(XI - &)D(d) is dense in E for some A > W. Then Q E (?+(C, w; E) (in

particular, it is single valued) and for each u E E

SS(t)u

S(t) u

(5.7.40)

uniformly on compact subsets of t >, 0, where S(t) = exp(t& ). Conversely, if

for each u e E the sequence (Sn(t)u) converges uniformly on compact subsets

of t > 0, d = ex-lim An is single valued, belongs to (2+(C, W; E) and (5.7.40)

holds, with S(t) = exp(td).

Proof

Since each An belongs to C'+(C, w; En) inequalities (5.7.36)

hold with c =1/C; taking into account the fact that d is densely defined, it

follows from Lemma 5.7.10 that d is single valued and (5.7.37) holds. We

show next that d is closed. Let (un,) be a sequence in D(ll) with un, -* u E E,

d un, -' v E E. Making use of the definition of (?, we can find for each m a

sequence (un,n; n > 1), umn E D(An), such that

lim llUmn-Pnumlln=0,

lim llAnunm-Pndunrlln=o-

n -oo n- oo

324

Perturbation and Approximation of Abstract Differential Equations

It is easy to see that this implies the existence of a sequence

u E E

such that (5.7.35) holds, showing that u E D(LL) and du = v. The closedness

of d and inequality (5.6.37) obviously imply that

(AI - 61)D(Q)

is a closed subspace of E for each A > w. Since (XI - CL) D(L) was assumed

dense in E for some A > w, (Al - L)D(L) = E for that value of A and

R(A; L) = (Al - LL)-' exists. Let v be an arbitrary element of E; write

v = (Al - LL)u, u E D(L), and select a sequence

u E

such that

Ilu,, - P,,uII-

(5.7.41)

hence the assumptions of Theorem 5.7.6 are satisfied with v = Q(A)u =

R(A; L)u,15 thus d E L?+(C, w; E) and (5.7.40) holds uniformly on compact

subsets of t >, 0. Conversely, assume that for each u e E and each t >, 0 there

exists v = v(t) such

(5.7.42)

uniformly on compact subsets of t >, 0. Arguing much in the same fashion as

in the construction of Q(A) in Theorem 5.7.6, we can show that v(t) _

S(t) u, S(t) a semigroup with I I S(t )I I < Ce' (t >, 0); also, since

IIS(t')u-S(t)uII

with

PPS(t)ull

and the convergence is uniform on compact subsets of t >- 0,

is strongly

continuous. If A is its infinitesimal generator, it only remains to show that

A = L = ex-lim A,,,

which we do now. Let u E D(A); write v = (Al - A)u for some A > w so

that

u=R(A;A)v= f e-xtS(t)vdt

and define

u = R(A; An)P,,v

=f e-J"S,,(t)P,,vdt.

'5Assumption (5.7.26) is only used to show that v =Q(A)u = R(A; A)u, where A is

densely defined operator with A e p(A); this is unnecessary here since Q(A)u = R(A; (?).

5.7. Approximation of Abstract Differential Equations 325

Then we have

Ilu" - P"uII" <f o"

e-XhIIS,,

(t)Pnv - P S(t)vII"dt

(5.7.43)

and we show that 11u,, - P"ull" - 0 as in the proof of Theorem 5.7.5(a). On

the other hand, Au = Au - v, Au" = Xu" - Pv so that

IIA,,u,, -P"AuII"<XIIu"-P"ull",

(5.7.44)

and it follows that u E D(d) with du = Au, in other words, that A C CT (note

that CT, being densely defined must be single valued by Lemma 5.7.9). We

can then apply (a) to show that d E C'+ and an argument similar to that

ending Lemma 2.3.9 shows that A = 6 as claimed.

5.7.12 Example. The method of lines for a parabolic equation. Let a be a

bounded domain of class C(00) in R',

A = 0 + c(x),

(5.7.45)

where c E C(°°)(S2). As a very particular case of the results in Chapter 4

(Theorem 4.8.11), we know that the operator A with domain consisting of

all u E Cr(S2) such that u E W2' °(E2) for all p < oo and Au E Cr(S2) (F _

boundary of 0) belongs to (2+(1, w) in E = Cr(S2) if

w = maxc(x).

X E 0

(We consider only real spaces and the Dirichlet boundary condition.) The

choice of approximating spaces is essentially that of Example 5.7.3, but the

subdivision of S2 is precisely specified as follows. Assuming 52 is contained in

the hypercube H defined by I x j I < a (1 < j < m ), for each n =1, 2,... we

divide the hypercube into (2n)' hypercubes determined by division of each

side of H into 2n equal segments. These hypercubes will be denoted as

follows: if a = (a1.... ,am) is a multi-index of integers with - n < aj < n - 1,

H" a denotes the hypercube defined by the inequalities

aja/n <x. < (aj + 1)a/n.

(5.7.46)

Also, x",,, denotes the point (a,a/n,...,ama/n) and for each n, J" is the set

of all multi-indices a with x,,,,, E S2, X" the set of all x",,, in 2, r(n) the

number of elements in J". The approximating spaces will be E" = R '(")

whose elements are denoted by ,

a; a E J") or simply each of

these spaces is endowed with the supremum norm

II" = Max

n a

(5.7.47)

The operators P" are defined by

P"u = (u(x",a); a E J"). (5.7.48)

The operators A" will be finite difference approximations to the partial

differential operator A. Obviously there is a great latitude in the choice of

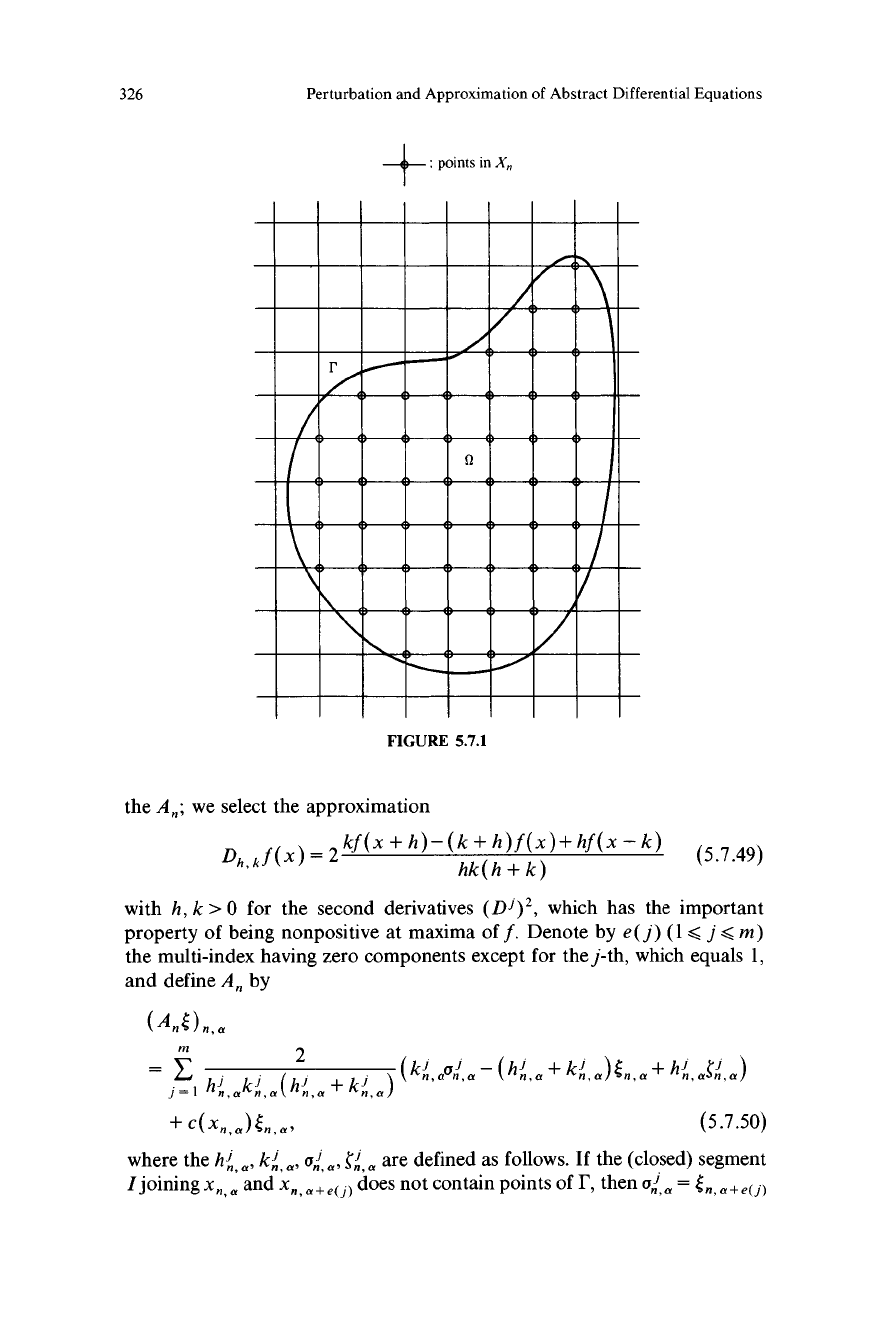
326

Perturbation and Approximation of Abstract Differential Equations

: points in X

FIGURE 5.7.1

the An; we select the approximation

D,,

kf(x)-2kf(x+h)-(k+h)f(x)+hf(x-k)

hk(h+k

7 49)

. .

)

with h, k > 0 for the second derivatives (Di)2, which has the important

property of being nonpositive at maxima of f. Denote by e (j) (1 < j < m)

the multi-index having zero components except for the j-th, which equals 1,

and define An by

(An)n,a

m 2

= r J

(Ti

-(hJ

J J 'J

J (hl

+ kJ

)

n,a

J=1 hnakna nana

+ C(Xn,a)En,a,

(5.7.50)

where the h,;,

k,;,

a, a

fin,

are defined as follows. If the (closed) segment

I joining xn

and xn,

a+e(j)

does not contain points of r, then an,a =

sn, a+e(j)