Fattorini H.O., Kerber A. The Cauchy Problem

Подождите немного. Документ загружается.

4.3. Applications to Ordinary Differential Operators

197

We take now u E LP(0,1) and A sufficiently large. We have

SP(t)u=R(X;

Ap(Q0,,01))(AI-Ap(/3o,$,))2SP(t)R(X; AP(/3o,$1))u

= R(A; A2(Q0, a1))(AI - A2(Qo, #,))2Sz(t)R(A; AP(Q0, $,)) u,

(4.3.41)

the last equality justified by (4.3.40) and preceding considerations. In view

of Theorem 4.3.1 forp = 2, S2(t) can be analytically extended to Y. +(ir/2-)

and satisfies (4.3.4) there for any (p

with 0 < 4p < it/2. Noting that

A2($0, $,)2S2G) = (A2(130,

and using (4.1.18), we can show

that for every 99 with 0 < p < it/2 and for each e > 0 there exist C, w

depending on 99 and a such that

II(AI-A2(/30,$,))zSzq)II<Cewli'I

( Egy(m), ICI%e) (4.3.42)

with C and w depending on T. Consider now D(Ap(,130, $1)), which consists

of all u E W" P(0,1) that satisfy the boundary conditions at 0 and 1. Since

D(AP(ao, /3,)) is a Banach space both under the norm of W,P and under

(the norm equivalent to) the graph norm IIuII'= II(XI - Ap(f30,,0,))uII and

the first norm dominates the second (times a constant), it follows from the

closed graph theorem that both are equivalent, so that, taking norms in

(4.3.41) (or, rather, in its analytic extension to Re t > 0), we obtain from

(4.3.40) that

can be extended to a C[0, l]-valued analytic function in

2+(ir/2-) and

Ap(Qo,al))u112

<Cew13"Illullp

under the conditions claimed in Theorem 4.3.5. This ends the proof.

As it may be expected, greater x-smoothness may be obtained if the

coefficients of A0 are assumed smoother. An auxiliary estimate will be

needed.

4.3.6 Lemma.

Let a (resp. b, c) be k + 2 times (resp. k + 1, k times)

continuously differentiable in 0 < x < 1;

let 1 < p < oo and A large enough.

Then the only solution of

(JAI-Ap(Qo,ar))u=f (4.3.43)

in D(A(,13o, /3,)) belongs to

W1+2.P(0,1) if f E Wk"P(0, I). Moreover, there

exists a constant C = Ck independent off such that

IIuIIWF+2.p <CIIfllws,p.

(4.3.44)

Proof. For k = 0 the result is of course a consequence of the

identification of the domain of Ap(/3o,,13,) in Section 3.4. Consider now the

198

Abstract Parabolic Equations: Applications to Second Order Parabolic Equations

case k =1. If u is an arbitrary solution (no boundary conditions) of the

homogeneous equation au" + bu' + (c - A) u = 0, then v = u' satisfies av" +

bv' + (c - X) v = g = - a'u" - b'u'- c'u (where g is continuous in 0 < x < 1)

in the sense of distributions. It follows then essentially as in Lemma 3.2.5

that v is twice continuously differentiable in 0 < x < 1, so that u E C(3)[0,1].

Let now G(x, ) be the Green function of A(/3o,,8/)- Al, where A is large

enough. Then we can write the solution of (4.3.43) in the form

u(x) = - f IG(x, f) f d

- ur(x) f a(E)(W(E) f (

)

(t) dt

(see Section 3.4 for the description of all the functions involved). We then

have

u'(x) = - u, (x) fX

(4.3.45)

Since both a, W are nonzero and continuously differentiable and uo, ul E

C(3)[0,1], it is clear that u E W3'p(0,1) and that an inequality of the type of

(4.3.44) holds.' The proof for k = 2,3,... is similar.

To simplify the statement of the next result we assume infinite

differentiability of the coefficients of Ao.

4.3.7 Theorem.

Let a, b, c be infinitely differentiable in 0 < x < 1.

Then each generalized solution u(t, x) = u(t)(x) _ (SS(t)u)(x) of u'(t) _

Ap(/3o, /3I)u(t), 1 < p < oo (after eventual modification in an x-null set for

each t) can be extended to a function uQ, x) infinitely differentiable in x and

analytic in for Re > 0; moreover, for every e > 0, every positive qq with

q, < it/2 and every integer k = 0, 1.... there exist C, co depending on e, T and k

such that

Ceu'1111jull ( E Y_(p), ICI % e),

(4.3.46)

where the norm on the right-hand side is the LP norm. A similar statement

holds for solutions in C.

Proof.

We have already observed in the proof of Theorem 4.3.5

that the W2'p norm and the norm II uII'

= II(AI - Ap(Qo, #j))uII are equiva-

lent in D(Ap(/3o, /6t))

(X large enough).

If u E D(Ap($0, /31)2),

then

(Al - Ap(ao, /3,))u belongs to W2'

, and

it follows from Lemma 4.3.6 that

1 Of course, that U E W3'°(0, t) follows directly from Lemma 3.2.6. The present

argument is used to establish (4.3.44).

4.4. Second Order Partial Differential Operators. Dissipativity

199

U E

with

IlUll w4.P <CII(xI- Ap(/3o,/3,))ullW2.P

< C'II(AI - Ap ($O, f31))Z UII

LP .

An inductive argument then shows in the same fashion that

llullwzj

<Ckll(XI-Ap(/30,b31)YullLP.

(4.3.47)

Write Ap(/30,

(Ap(/30,

Using (4.1.18) for ICI % r/J

and (4.3.47), we deduce that satisfies an estimate of the

required form. The result then follows from the fact (consequence of (4.3.40)

applied to higher derivatives) that Wk+ I , p(0,1) c C(k)[0,1 ] with bounded

inclusion.

4.3.8 Remark. If the operator A is written in variational form (2.2.35) all

the results in this section (except for Lemma 4.3.6 and Theorem 4.3.7) can

be proved under the only assumption that a, b are continuously differentia-

ble; the assumption on c is the same. In Lemma 4.3.6 it is enough to assume

that a, b are k + 1 times continuously differentiable, c is k times continu-

ously differentiable.

4.3.9 Example. The results in Example 3.4.5 can be extended to the

operators in the present section (i.e., the dissipativity assumptions on the

boundary conditions can be lifted).

4.4.

SECOND ORDER PARTIAL

DIFFERENTIAL OPERATORS. DISSIPATIVITY

Throughout the rest of this chapter we shall study operators of the form

A0u= E E ajk(x)D'Dku+ Y_ bj(x)D'u+c(x)u

(4.4.1)

j=1 k=1

j=1

in a domain SZ in Euclidean space Rm with boundary F. The coefficients will

be assumed to be real and, for the time being, as differentiable as required

in the various computations. Note that if the a jk are continuously differen-

tiable in SI, we may write A0 in divergence or variational form,

m m

A0u= E E D'(ajk(x)Dku)+ E bj(x)D'u+c(x)u, (4.4.2)

j=1 k=1

j=1

where

bj = bj - E Dkajk. (4.4.3)

k=1

In this section and the next we shall use either of the two forms as

convenience dictates. We may and will assume that the matrix d(x)=

200

Abstract Parabolic Equations: Applications to Second Order Parabolic Equations

(ajk(x)) is symmetric for all x, since we can always replace ajk(x) by

z{ajk(x)+akj(x)}, which does not modify the action of Ao on functions.

Before discussing the assignation of boundary conditions, we ex-

amine in this section the behavior of A0 applied to functions that vanish for

large

I x I and near the boundary of Q. To this end, we define D(Ao) to be

the space V2)(0) of all twice continuously differentiable functions on SZ

with compact support contained in SZ (recall that supp u, the support of u, is

the closure of the set (x E Sl; u(x) * 0)). We shall consider Ao in the

complex spaces LP(SZ), 1 < p < oo and C0(Sl), the space of all functions

continuous in SZ that tend to zero as

I x I - oo if 9 is unbounded 2; if 9 is

bounded, E = C(S2 ).

4.4.1

Lemma.

The operator A0 is dissipative in C0(SZ) (dissipative

with respect to a duality map) if and only if

l(x)>_0, c(x)<0 (xESt), (4.4.4)

where d (x) >, 0 means the matrix 9 (x) is positive definite,

m m

E E ajk(x)SJSk > 0

( E 118").

(4.4.5)

i=1 k=1

The proof runs very much as in the one-dimensional case (Lemma

3.2.1)

thus we omit some details.

Let u E D(Ao), u 0, m(u) =

(x E St; I u(x)l = hull). Since u(x) = 0 for x E 1 and at infinity, m(u) is

bounded and m(u)tlT = 0. Any element t in 0(u) c E(S2) is supported by

m(u) and u(x) t(dx) is a positive measure in m(u). Since Iu12 attains its

maximum in m (u ), we must have

ZDjiu12 = u1Dju1 + u2Dju2 = 0

(x E m(u)) (4.4.6)

for j =1, 2,

... , m, (where u = u 1 +'U2), and the Hessian matrix 5C (x; 1u12)=

(DjDkl u(x)l2) is negative definite (- X is positive definite) for x E m(u).

We note now that

' DjDklu12

= u1DjDku1 + u2DiDku2 + Diu1Dku1 + DJu2Dku2

while, for x E m(u) we have

u_1D'Dku = II u11-2(u1DjDku1 + u2DjDku2)

+ i11 u11-2(u1DjDku2 - u2DjDku1)

and

u-'Dju = ill ull_2(uiDju2 - u2Dju1 ).

It follows immediately

that Re(u-'Dju) = 0 and Re(u-'DJDku) _

2Naturally D(A0)=6D (2) (U) is not dense in E=Co(Sl). This has no bearing on

dissipativity.

4.4. Second Order Partial Differential Operators. Dissipativity

201

II uII-2(zDjDklul2 - DjulDkul - Dju2Dku2); accordingly,

Re(µ, A0u)

(IIuII -2LLajk(' DjDkI ul2 - DjulDkul -

Dju2Dku2)+c)udtL < 0

m(u)

(4.4.7)

in view of the hypotheses and of the following elementary result on linear

algebra.

4.4.2 Lemma.

Let CT = (ajk), 9C = (hjk) be symmetric positive defi-

nite m X m matrices. Then

m m

L L

ajkhjk i 0 (4.4.8)

k=1 i=1

Proof.

Let J = (bjk) be an orthogonal matrix such that JC =

where ' indicates transpose and 6D is a diagonal matrix; since the elements

v 1, ... , v,, in the diagonal are eigenvalues of JC they must be nonnegative.

Clearly we have

Y.Y.ajkhjk =

vi bik vi

which is obviously nonnegative. This ends the proof.

To prove that both conditions (4.4.4) are necessary, let be an

arbitrary vector in R m, a a positive number, x0 a point in

Q. Since

E0 = (EJ k) is a positive definite matrix we can find a real valued twice

differentiable function with compact support contained in 0 (that is, a

function in D(A0)) having a single maximum at x0 and such that

u(x0)=a,

The duality set 0(u) than consists of the single element a80 (80 the Dirac

measure at x0) and

(80, Au) _ - (e E, ) + ac(x0),

(4.4.9)

which can be made positive by adequate choice of , a if any of the two

conditions (4.4.4) are violated.

Second order operators A0 satisfying (4.4.5) are called elliptic in 1.

As we shall see next, the ellipticity condition is also necessary and sufficient

(together with additional assumptions on the bj and c) for dissipativity of A0

in LP(S2).

4.4.3 Lemma.

The operator A0 is dissipative in LP (1 < p < oo) if

li(x)>,0 (xE2) (4.4.10)

and

c(x)+

(xE$2). (4.4.11)

202

Abstract Parabolic Equations: Applications to Second Order Parabolic Equations

The first inequality is necessary in all cases; the second is necessary when

p=1.

Proof. If p > 1 the only duality map 0: LP -> LP'( p' -' + p - ' = 1) is

B(u) = II ulI2-pI u(x)Ip-2u(x) (u - 0). Forp >, 2 we prove just as in the case

m =1 that if u e D(Ao), then 0(u) has continuous first partials and

IIuIVp-2D'O(u)(x)

= (p-2)I uIp-4Re(UDiu)U+ l

Ulp-2D'u

while, on the other hand,

DiluI" = plulp-2Re(iDJu).

To compute Re(B(u), Aou) we use the divergence form (4.4.2) of the

operator, obtaining

Ilullp-2Re(0(u), Aou)

= Re f,{EEDJ(ajkDku)+E(bj -EDkajk)DJu+cu)IuIp-2udx

_ -(p -2)fflUlp-4{ EEajkRe(uDYu)Re(i1Dku)) dx

f0llllp 2(LEaikD'uDku) dx

+P +pc)Iulpdx<0

(4.4.12)

by means of integration by parts in each variable (since u vanishes near the

boundary I no assumptions on applicability of the divergence theorem are

necessary). Note that in establishing (4.4.12) we have made use of the

inequality

(4.4.13)

valid for arbitrary complex

In the case I < p < 2 the computations are formally valid, but (as already

pointed out when m =1 in Section 3.2) it is not clear whether 9(u) is absolutely

continuous in any one variable. We bring again into play the class 6J'd (2) of piecewise

analytic, twice continuously differentiable functions of one variable with compact

support. If u E D(Ao), then u can be uniformly approximated together with its

derivatives of order < 2 by a linear combination of functions of the form

U1® ... ®Um,

where the u, are twice continuously differentiable functions of one variable and the

support of each term in the linear combination is contained in a (a theorem of this

type is proved in Schwartz [1966: 1, p. 108]. A similar statement can be easily seen

to hold with

U. E

A linear combination v of the type obtained has the

property that xj - v (x) (x,....,xj - i,xj + .,...,xm fixed) belongs to 90(2) so that

6(u) is absolutely continuous as a function of any one of its variables and therefore

the manipulations leading to (4.4.12) are fully justified also for I < p < 2.

4.4. Second Order Partial Differential Operators. Dissipativity

203

The case p =1 is again handled by a limiting argument as we did in

Lemma 3.2.2; also, the treatment for m =1 can be extended to show that

(4.4.11) is necessary when p = 1.

Finally, the necessity of (4.4.10) for I < p < oo follows from

4.4.4 Example. Let p > 1, and let (ask), g, be continuous in Q. Assume that

lull

uIP-ZEY_ajkDjuDku+gluIP) dx>- 0

for all u E 6D (S2) R. Then

(?(x)>-0 (xe2).

An important role in the rest of the chapter will be played by the

formal adjoint Ao of A0 defined by

Ao in the same way as D(Ao). Integrating by

parts, we obtain

f(Aou)vdx= fuuAovdx

(4.4.15)

for u, v e D(A0) = D(A'), which equality can be taken as a definition of

A'. We relate now the dissipativity conditions for A 0 and A'. Clearly (4.4.4)

is the same either for A. or Ao and it is easy to see that the inequality

(4.4.11) holds for an operator A 0 in LP if and only if it holds for Ao in Lv',

p'-' + p-' =1 (in Co if p = 1). The necessity of these conditions in L' and

Co then shows that A0 is dissipative in L'(S2) if and only if Ao is dissipative

in Co(S2).

From Section 4.6 onwards the operator A0 will generally be written

in divergence form (4.4.2). In this notation, the formal adjoint is given by

Aou=EEDJ(ajk(x)Dku)-Y_D'(bj(x)u)+c(x)u

(4.4.16)

and the dissipativity condition (4.4.11) for E = LP(l ), 1 < p < oo, takes the

simple form

c(x)- p Y, D'bj (x) <0.

(4.4.17)

Obviously, the analogous dissipativity conditions for C(S2) are the same

irrespective of the form we use in writing A0.

We note finally that the fact that (4.4.11) (or (4.4.17)) is not

necessary for dissipativity of A. when I < p < oo has already been pointed

out in Section 2.3 for the case m = I (Example 3.2.4). A counterexample for

the case m > 1 can be constructed in an obvious way using that result.

4.4.5

Example. Show that inequality (4.4.11) is necessary for dissipativity

of A0 in L'(2).

204

Abstract Parabolic Equations: Applications to Second Order Parabolic Equations

4.5. SECOND ORDER PARTIAL DIFFERENTIAL OPERATORS.

ASSIGNATION OF BOUNDARY CONDITIONS

We need now conditions on 0, F, in order to be able to apply the divergence

theorem in 2,

fr(U, v) do

= fudivUdx,

(4.5.1)

to smooth vector fields U with compact support, where v is the outer normal

vector at F and do denotes the hyperarea differential on F. We introduce a

sequence of classes of sets where the conditions for validity of (4.5.1) are

amply satisfied.

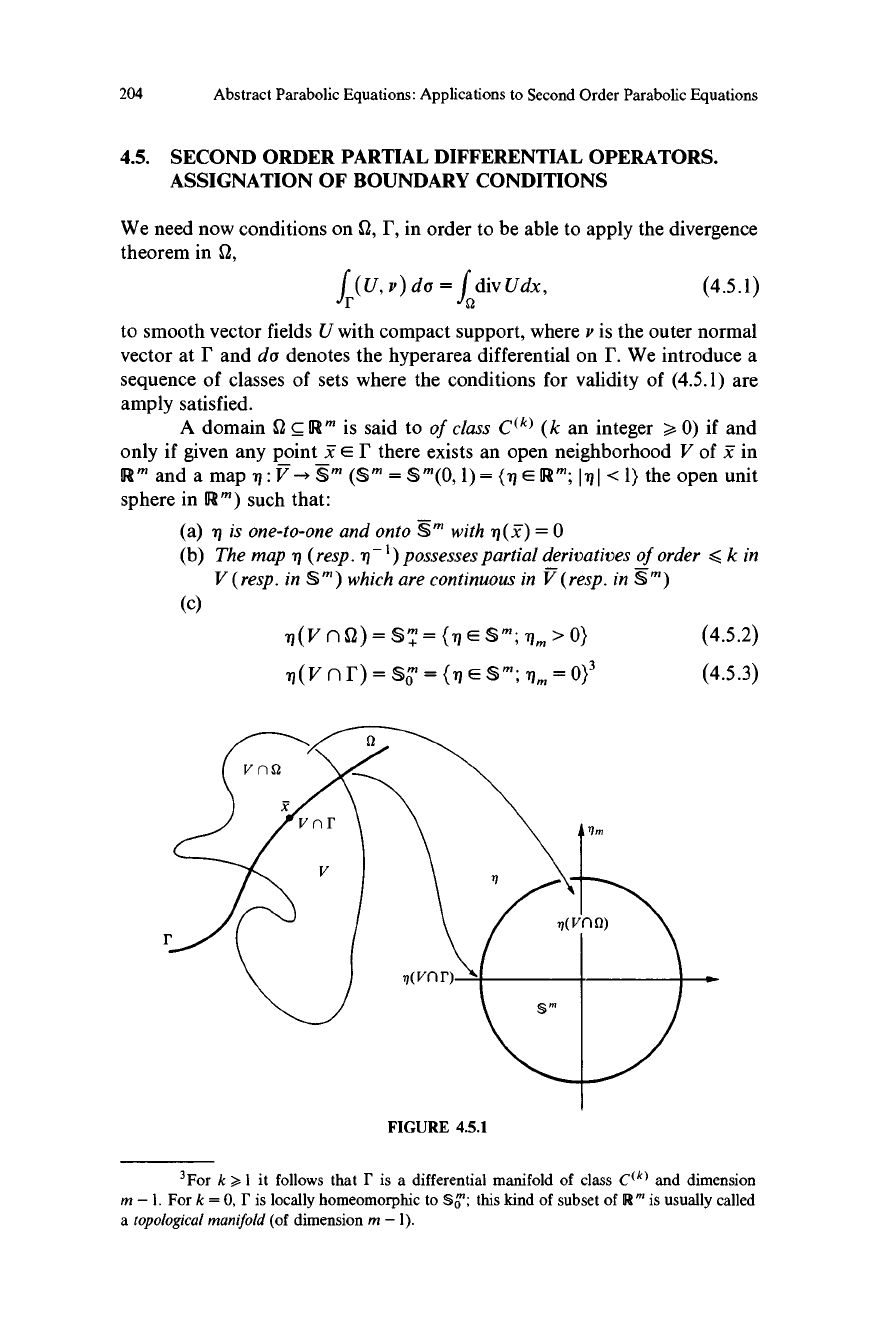
A domain St C Rm is said to of class C(k) (k an integer > 0) if and

only if given any point x E F there exists an open neighborhood V of V in

U8 m and a map rl : V --> 9' (S m = S m (O, 1) = ( rl E R m; 17 11 < 1) the open unit

sphere in R m) such that:

(a) q is one-to-one and onto 9m with n(x) = 0

(b) The map rl (resp. n -') possesses partial derivatives of order S k in

V (resp. in S m) which are continuous in V (resp. in S")

(c)

,1(((V n 0i) _ s+ = (,i e 5m; jm > 0)

V n r) _ .s 0 = (71 E §m; nm = 0)'

FIGURE 4.5.1

(4.5.2)

(4.5.3)

3For k > I

it follows that I is a differential manifold of class C(k) and dimension

m - 1. For k = 0, I' is locally homeomorphic to S(; this kind of subset of R "' is usually called

a topological manifold (of dimension m -1).

4.5. Second Order Partial Differential Operators. Assignation of Boundary Conditions

205

We shall call any of the neighborhoods V postulated above a boundary patch

with associated map q. An argument involving local compactness of R'

shows that we can find a sequence V1, V2, ... of boundary patches and a

sequence S 1, S2, ...

of spheres §n = S "' (x,,, pn) _ (x E R 'n; I x - x,, I < p,,)

(called interior patches) with the following properties:

(d) Let K be any compact subset of Rm. Then

KnVn=o, Kn§n=o

(4.5.4)

except for a finite number of indices.

(e) There exists p', 0 < p'< 1, and a sequence (p; ), 0 < pn < pn such

that S2

is contained in the union of the §m(xn, pn) and the

V = rln'(Sm(0, p')) (?ln is the associated map of Vn).

In intuitive terms, the (Vn) and the (S) cover 0 with "something to

spare near the boundaries."

We point out that in the case where 0 is bounded, the Heine-Borel

theorem shows that the cover (Vn)U(S,) can be chosen finite.

It is easy to see that, given p", PI, P2, with p'< p" < 1, Pp,? < Pn" <

pn we can find nonnegative functions , 2,... in 6D with (x) =1 in

§'(0,

=1 in Sm(xn, pn), (x) = 0 outside of §'(0, p"), n(x) = 0

outside of Sm(xn, p;; ). Since, in view of (d), all the terms of the sum

(4.5.5)

except for a finite number, vanish on any given compact set it is clear that

is well defined and k times continuously differentiable if 2 is of class

C(k) ; moreover (e) implies that

T(x) > 0

(x E S2)

so that if we define X;, (x) = n(x)/T(x), Xn(x) = '(rln(x))/T(x), we obtain

a partition of unity (X;,)U(Xn),

_ Xn(x)=1

(xES2)

YXn(x)+Y

(4.5.6)

subordinated to the cover (Sn)U(Vn) in the sense that the support of each X

is contained (with something to spare) in either a boundary or an interior

patch. This partition of unity (which has some deluxe features not always

needed) consists of k times continuously differentiable functions if a is of

class 00 and will allow us to piece together local results. In the sequel,

interior patches `§n will be denoted by Vn' and boundary patches by Vh.

We note, finally, that various additional conditions can be imposed

on the V and the Vh without modifying the previous statements; for

example, we may require these sets to be of diameter <8 (this will be

essential in the proof of Theorem 4.8.4). With reference to Green's theorem

(4.5.1), it is not difficult to see that it holds in a domain of class 00 for

206

Abstract Parabolic Equations: Applications to Second Order Parabolic Equations

vector fields U continuously differentiable on n and having compact sup-

port; in particular, v and do are well defined everywhere. It is clear that the

requirement that 0 be of class C(') leaves out such everyday domains as

cubes, where the divergence theorem holds; however, this is not very

important since we shall be forced to suppose in most of our treatment that

SZ is at least of class C(2). On the other hand, although tougher domains will

be admitted in Section 4.6, no use of the results in this section will be made

there.

We study now dissipativity of certain extensions of A0, beginning

with the LP case. We denote by Al the operator (4.4.1) with domain

D(A1) _ 6 (2)(SZ) consisting of all functions u twice continuously differentia-

ble in I with compact support. We assume that SZ is of class C(') and that

the coefficients ask, bj, c admit extensions to St having as many continuous

derivatives as needed in each statement.

We look for dissipative restrictions of A 1. Our first computation is an

offspring of (4.4.12), taking into account the boundary terms. As before, we

transform first A0 to divergence form and then apply the divergence

theorem, obtaining

IIuIIp-2Re(O(u), A,u) = Ref (EED1(ajkDku))Iulp-2udx

fuclulpdx

= - (p -2)

fuI uIp-4{ EEajkRe(iD'u)Re(uDku)) dx

- fuI

ulp-2( Y,E,aikDJuDkg) dX

+ I fu(EED'Dkajk-EDibi+pc)luIpdx

+p fr(E(bj-EDkajk)vj)Iulpdo, (4.5.7)

where v1,

... ,1 m are

the components of the outer normal vector P. If

hypotheses (4.4.10) and (4.4.11) in the previous section are satisfied it is

clear that the integrals over SZ contribute a nonpositive amount to (4.5.7). To

streamline the writing of the two boundary integrals we introduce the

following notations:

m m

D' =

L L ajkv,

DkU,

(4.5.8)

j=1 k=1