Fattorini H.O., Kerber A. The Cauchy Problem

Подождите немного. Документ загружается.

2.2. The Cauchy Problem in - oo < t < oo. The Adjoint Equation

(see Section 6 and references there.) In particular, if a(A) is contained in the

imaginary axis, then A E (2 (1, 0). (Recall that if the spectrum of a normal

operator is contained in the imaginary axis, then A= iB, where B is

self-adjoint.) The propagator S(t) can be computed by formula (2.2.20) and

we have

IIS(t)II=ew't,

IIS(-t)II =e"2t (t, 0).

Let A be an operator in C3+(C, w). It is of great interest to know

whether the adjoint A* belongs to E+ as well. The question has a very simple

answer when E is reflexive; in fact, in this case D(A*) is dense in E*,

p(A*) = p(A), and R(X; A*) = R(X; A)* (Section 4). It follows then that A*

satisfies as well (and with the same constants) inequalities (2.1.11) and thus

belongs to (2+ The same is true, of course, in relation to the class e. To

identify S*(-), the propagator of

u'(t) = A*u(t),

we observe that if u E E and u* E E*,

(2.2.22)

fooo e-'"(S*(t)u*, u) dt = (R(µ; A*)u*, u) = (u*, R(µ; A)u)

= f 00 e-"t(S(t)*u*, u) dt (µ > w).

It follows then from uniqueness of Laplace transforms that S*(t) = S(t)*

for t > 0. If A belongs to (2, a similar argument takes care of S*(t) for t

negative. We formalize these comments in the following

2.2.9 Theorem. Let A E (+(C, w), and assume E reflexive. Then

A* E e+(C, w) as well. The solution operator of (2.2.22) is where

the solution operator of 2.2.8. The same conclusion holds for the class (2 (C, W).

If the space E is not reflexive, the situation is considerably more

complicated. In fact, it may be no longer true that A* is densely defined;

worse yet, there might be elements u* of D(A*) for which no solution of

(2.2.22) with u* as initial datum exists.

2.2.10 Example. We use the result in Example 2.2.5 with p =1. In this case

E* = L°°(- oo, oo). To compute the adjoint of A, we observe that if u E D(A*), then

there exists v( = A*u) in E* such that

f u(x)w'(x) dx = f v(x)w(x) dx

(2.2.23)

for all w E D(A). Since this equality must hold in particular when w is a test

function, it follows that u' = - v (in the sense of distributions), so that u, if

necessary modified in a null set, is absolutely continuous. Conversely, if u is

Properly Posed Cauchy Problems: General Theory

u(t + h) - u(t)

t t+h t+1 t+l+h

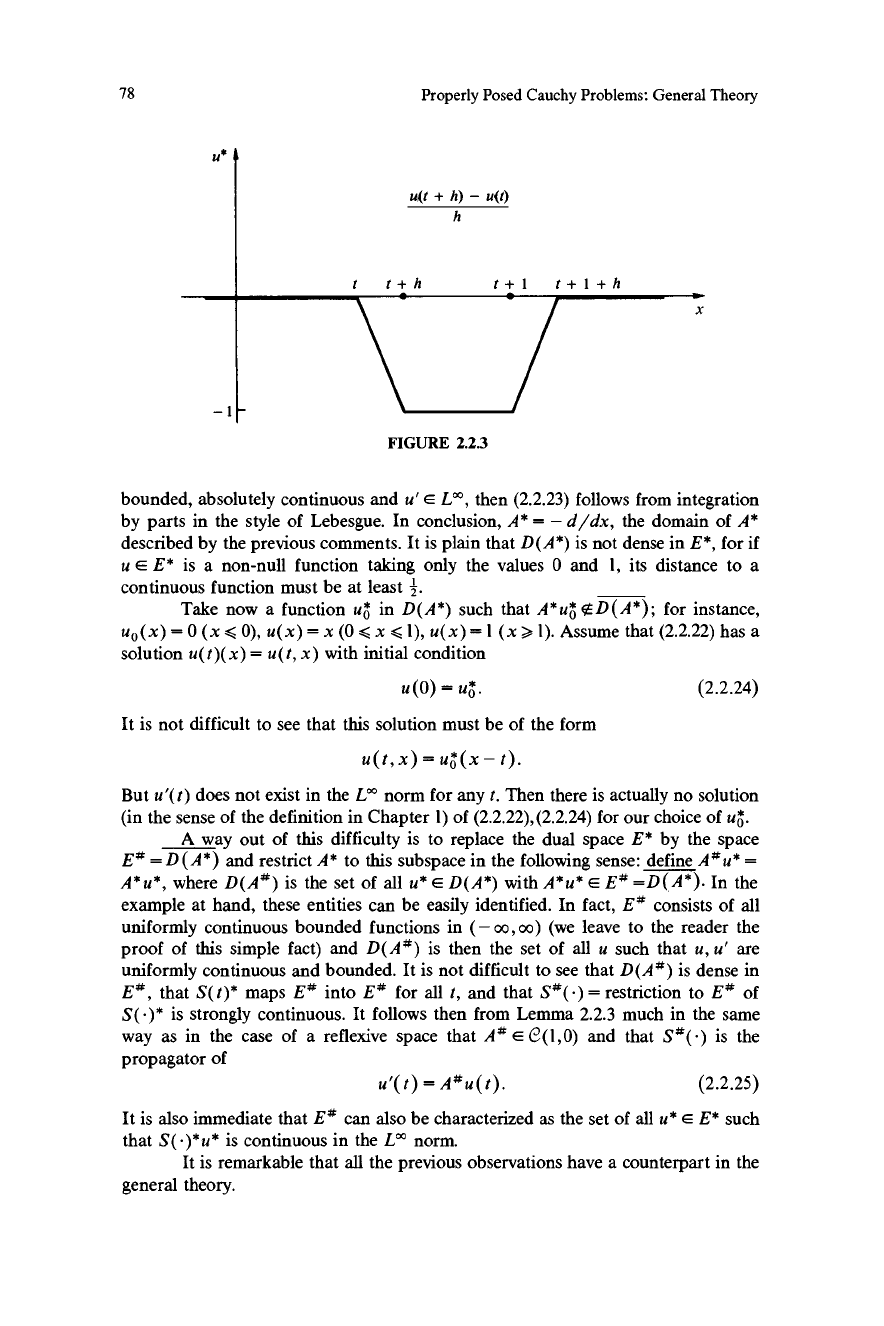
FIGURE 2.2.3

bounded, absolutely continuous and WE=- L°°, then (2.2.23) follows from integration

by parts in the style of Lebesgue. In conclusion, A* = - d/dx, the domain of A*

described by the previous comments. It is plain that D(A*) is not dense in E*, for if

u E E* is a non-null function taking only the values 0 and 1, its distance to a

continuous function must be at least -

Take now a function uo* in D(A*) such that A*uo* eD(A*); for instance,

uo(x) = 0 (x < 0), u(x) = x (0 < x < 1), u(x) =1 (x > 1). Assume that (2.2.22) has a

solution u(t)(x) = u(t, x) with initial condition

u(0) = uo. (2.2.24)

It is not difficult to see that this solution must be of the form

u(t,x)=uo*(x-t).

But u'(t) does not exist in the L°° norm for any t. Then there is actually no solution

(in the sense of the definition in Chapter 1) of (2.2.22),(2.2.24) for our choice of uo*.

__& way out of this difficulty is to replace the dual space E* by the space

E# =D(A*) and restrict A* to this subspace in the following sense: define A#u* =

A*u*, where D(A#) is the set of all u* E D(A*) with A*u* E E# =D(A*). In the

example at hand, these entities can be easily identified. In fact, E# consists of all

uniformly continuous bounded functions in (- oo, oo) (we leave to the reader the

proof of this simple fact) and D(A*) is then the set of all u such that u, u' are

uniformly continuous and bounded. It is not difficult to see that D(A#) is dense in

E*, that S(t)* maps E# into E# for all t, and that S#(.) = restriction to E# of

is strongly continuous. It follows then from Lemma 2.2.3 much in the same

way as in the case of a reflexive space that A# E (2(l,0) and that

is the

propagator of

u'(t)=A#u(t). (2.2.25)

It is also immediate that E# can also be characterized as the set of all u* E E* such

that

is continuous in the L°° norm.

It is remarkable that all the previous observations have a counterpart in the

general theory.

2.2. The Cauchy Problem in - oo < t < oo. The Adjoint Equation

2.2.11 Theorem. Let A E '+(C, w) with E* not necessarily re-

flexive; let E# be the closure of D(A*) in E*, A*u* = A*u*, the domain of

A# consisting of all u* E D(A*) such that A*u* E E*. Then D(A#) is dense

in E* and A# E t+(C, w) in E*; the propagator of (2.2.25) is S*(-), the

restriction of S(-)* to E*. The subspace E* can also be characterized as the

set of all u* E E* for which S(.)*u* is continuous in t >_ 0. The same

conclusions hold for the class 9.

Proof We have

A*R(A;A*)u*=XR(X;A*)u*-u* (u*EE*)

so that if u* E E#, then A*R(A; A*)u* belongs as well to E#. In other

words,

R(A; A*)E* C D(A#).

(2.2.26)

Since IIR(A: A*)II = IIR(X; A)II = O(1/A) as A - oo, we obtain from Exam-

ple 2.1.6 that

lim \R(X;A*)u*=u*

(2.2.27)

A- o

for all u* E E*. This, combined with (2.2.26), clearly shows that D(A*) is

dense in E#. We have obtained as a consequence the fact that R(X; A*)E*

c E*, so that equalities (4.11) and (4.13) imply

R(A; A#)

R(A; A*)I

E#,

(2.2.28)

where the right-hand side of (2.2.28) is the restriction of R(X; A*) to E#. It

is then obvious that IIR(A; A#)II < IIR(A; A*)II = IIR(A; A)II and that a simi-

lar inequality holds for the powers R(A; A#)", hence Theorem 2.1.1 implies

that A# E (2+(C, w). Let S#(.) be the propagator of u'(t) = A*u(t). Then if

u*EE*,uEE,

fooo e-s`(S#(t)u*, u) dt

= (R(A; A*)u*, u) = (R(X; A*)u*, u)

= (u*, R(X; A)u)

f OOe`(u*, S(t)u) dt (A > w).

By uniqueness of Laplace transforms and arbitrariness of u, we obtain that

S*(t)u* = S(t)*u*, hence

S#(t) = S(t)* I E'

is clear from this that is continuous in t > 0 if u* E E*.

Conversely, let u* be an arbitrary element of E* such that is

continuous at t = 0. If e> 0 and 8 is so small that IIS*(t)u*- u*II < e for

Properly Posed Cauchy Problems: General Theory

0 < t < 8, we obtain

IIXR(X;A*)u*-u*Il <X

j°°e-atIIS(t)u*_u*lldt

<E+0(1) asX-goo

dividing the interval of integration in (0, 8) and (8, oo). It follows that

XR(A; A*)u* - u* as A - oo. But R(A; A*)u* E D(A*), thus u* ED A* _

E*. The proof of Theorem 2.2.11 is thus complete.

The result just proved would be of course of little use unless we can show

E* is "large enough" in some sense. Since D(A*) is weakly dense in E* (Section 5),

the same is true of E# and of D(A#); in particular, if (u*, u) = 0 for all

u* E D(A*), it follows that u = 0. More than this can be proved; in fact, we have

the following result.

2.2.12 Example.

Define a new norm in E by the formula

Ilull'= sup(I (u*, u) I

; u* (=- E*, llu*ll < 1).

Then

(lull' < (lull < Cllull',

where C = lim inf

a - 0IIX

R (X; A)11 (Hille-Phillips [1957; 1, p. 422]).

The operator A* E e+ associated with an A E e+ will be called the

Phillips adjoint of A. Likewise, S*(.) is the Phillips adjoint of S(-).

2.3.

SEMIGROUP THEORY

Let t -* S(t) be a function defined in t, 0 whose values are n X n matrices.

Assume that

S(0)=I, S(s+t)=S(s)S(t) (s,t,0) (2.3.1)

and that S is continuous. Then (see Lemma 2.3.5 and Example 2.3.10)

S(t) = etA (2.3.2)

for some n X n matrix A or, equivalently, S is the unique solution of the

initial value problem

S'(t) = AS(t), S(0) = I.

(2.3.3)

This result is due to Cauchy [1821: 1] for the case n =1 and to Pblya [1928:

1] in the general case. Roughly speaking, it can be said that the core of

semigroup theory consists of generalizations of the Cauchy-Pblya theorem

to functions taking values in an infinite dimensional space E. What

makes the theory so rich is the fact that "continuity," which has only one

reasonable meaning in the finite-dimensional case, can be understood in

many ways when dim E = oo; for instance, we may assume S continuous in

the norm of (E), strongly continuous, or weakly continuous. Another

2.3. Semigroup Theory

fundamental difference is that, while the Cauchy-P51ya theorem guarantees

extension of S to t < 0 when dim E < oo, this extension may not exist at all

in the general case. However, it can be said that the close connection

between the initial-value problem (2.3.3), its solution (2.3.2), and the func-

tional equation (2.3.1) is maintained in infinite-dimensional spaces if the

correct definitions are adopted.

We call a semigroup any function S(.) with values in (E) defined in

t > 0 and satisfying both equations (2.3.1) there. Note that we have proved

in Section 2.1 that the propagator of an equation

u'(t) = Au(t) (2.3.4)

for which the Cauchy problem is well posed is a strongly continuous

semigroup. The converse is as well true. In fact, we have

2.3.1 Theorem. Let be a semigroup strongly continuous in

t > 0. Then there exists a (unique) closed, densely defined operator A E C2+

such that S is the evolution operator of (2.3.4).

Proof We define A, the infinitesimal generator of S, by means of

the expression

Au= lim

1 (S(h)-I)u,

(2.3.5)

ho+ h

the domain of A consisting precisely of those u for which the limit (2.3.5)

exists. We show first that D(A) is dense in E. To this end, take a > 0, u E E

and define

u"= 1

S(s)uds.

a o

Some manipulations with the second equation (2.3.1) show that

h(S(h)-I)u"a{

+hS(s)uds-hJhS(s)uds).

(2.3.6)

In view of the continuity of S, the limit as h - 0 of the right-hand side of

(2.3.6) exists and equals a-1(S(a)u - u). Accordingly, u" E D(A) and

Au" = a (S(a) u - u). (2.3.7)

But a similar argument shows that u" - u as a -* 0, which establishes the

denseness of D(A).

We prove next that A is closed. To this end, observe that, if u E E

and t, h > 0,

(S(h)-I)S(t)u=S(t)h (S(h)-I)u,

hence if we take u E D(A) and let h - 0+, S(t)u E D(A) and

AS(t) u = S(t)Au.

(2.3.8)

Properly Posed Cauchy Problems: General Theory

This clearly implies that

(Au)a=Aua= a(S(a)u-u) (uED(A)). (2.3.9)

Let be a sequence in D(A) with u - u, Au,, -* v for some u, v E E.

Then

h(S(h)-I)u=nlimo I (S(h)-I)u

= lim (Au,, )h=vh (h>0).

n-oo

Taking limits as h - 0+, we see that u E D(A) and that Au = v, which

shows the closedness of A.

To prove that A E C+, we begin by verifying the first half of the

definition of well-posed Cauchy problem (Section 1.2). Take u E D(A) and

integrate (2.3.8) in 0 < s < t: we obtain

f tS(s)Auds =

f tAS(s)uds = A

f tS(s)uds

=S(t)u-u,

where we have made use of (2.3.7) in the last equality. This clearly shows

that S(.) u is differentiable in t > 0 and

S'(t)u = S(s)Au = AS(s)u,

thus settling the existence question. To show continuous dependence on

initial data, we proceed in a way not unlike the closing lines of Theorem

2.1.1. Observe first that, since is strongly continuous, II

ul I must be

bounded on bounded subsets of t > 0. By the uniform boundedness theorem

(Section 1), there exist constants C(t) such that

IIS(s)II <C(t) (0<s<t)

(2.3.10)

for all t >, 0. Making use of this it is not difficult to show that if u(.) is an

arbitrary solution of (2.3.4), then v (s) = S(t - s) u(s) is continuously dif-

ferentiable in 0<s<t and v'(s)=S(t-s)Au(s)-AS(t-s)u(s)=O.

Hence

u(t) = S(t)u(0),

(2.3.11)

and the fact that the continuous dependence assumption is satisfied is an

immediate consequence of (2.3.10) and (2.3.11). This ends the proof of

Theorem 2.3.1.

Considering semigroups (rather than differential equations) as our

primary objects of interest, which seems to be traditional in the literature,

we can combine Theorems 2.1.1 and 2.3.1 in the single

2.3.2

Theorem.

The operator A is the infinitesimal generator of a

strongly continuous semigroup S satisfying

IIS(t)II <Ce"t

(t >, 0) (2.3.12)

2.3. Semigroup Theory

if and only if A is closed and densely defined, R(X) exists for Re X > co, and

IIR(X)nII'< C(ReX-w)-" (ReX>w,n>l).

(2.3.13)

The treatment of the case - oo < t < oo is entirely similar. A function

with values in (E), defined in - oo < t < oo and satisfying (2.3.1) there is

called a group. The analogues of Theorems 2.3.1 and 2.3.2 in this case are

2.3.3 Theorem. Let be a strongly continuous group. Then there

exists a (unique) closed, densely defined operator A E C2 such that S is the

evolution operator of (2.3.4).

2.3.4

Theorem.

The operator A is the infinitesimal generator of a

strongly continuous group S satisfying

IIS(1)II<Ce"1t

(-oo<t<oo) (2.3.14)

if and only if A is closed and densely defined, R(A) exists for I Re A I > w, and

IIR(X)nII<C(IRe

XI-(0)-n

(ReX>w,n>, l). (2.3.15)

These results, together with the representation for S outlined in

Remark 2.1.2, constitute an extension of the Cauchy-Pblya theorem to the

infinite-dimensional case. If we replace strong continuity by uniform con-

tinuity, the corresponding extension is much simpler (Lemma 2.3.5) al-

though of scarce interest for the study of the Cauchy problem (see Example

1.2.2).

It was already observed (Example 1.2.2) that every bounded operator A

belongs to (2 and the solution of (2.3.3) is continuous (in fact analytic) in the norm

of (E). Using Theorem 2.3.1, or directly, it is easy to show that S is the semigroup

generated by A; in conclusion, a bounded operator always generates an uniformly

continuous semigroup. We give a proof of the converse.

2.3.5 Lemma. Let the semigroup be continuous at t = 0 in the norm of

(E). Then its infinitesimal generator A is bounded.

Proof. Let C, to > 0 be such that (2.3.12) holds. Then, if A > w,

(R(A)--I)u= f _e-"(S(t)-I)udt (uEE)

so that

< f

Ooe'rl(t) dt,

(2.3.16)

where rl(t) = IIS(t)- III is continuous for t = 0, n(O) = 0, and is bounded by Cew` + 1

in t > 0. Let e > 0 and S > 0 such that s (t) < e if 0 < t < S. Splitting

the interval of

integration at t = S we obtain

f°°e-Xtn(t)dt< a +o -

Properly Posed Cauchy Problems: General Theory

as X - oo. This shows, in combination with (2.3.16), that IIR(X)- A-'III = o(A-') as

oo. Accordingly, if A is large enough,

I I A R (A) - III < I, which implies that

AR(A)-hence R(A)-must have a bounded inverse. But R(A)-'= Al - A, thus A

must be itself bounded. This ends the proof of Lemma 2.3.5.

We note in passing that the result in Lemma 2.3.5 can be obtained with

weaker hypotheses; in fact, we only need

liminf AJ Se-;`rl(t) dt < 1

(2.3.17)

for some (then for any) 8 > 0.

2.3.6 Example.

Prove that (2.3.17) is a consequence of

limsupllS(t) - III < 1

t - 0+

so that (2.3.18) implies boundedness of the infinitesimal generator.

(2.3.18)

2.3.7 Example.

The condition

liminfllS(t)-III=0

t-.0+

does not imply boundedness of the infinitesimal generator, even if

is a group.

(Hint:

Let (ej; j > 0) be a complete orthonormal sequence in a Hilbert space H,

and let (0(j)) be an increasing sequence of positive integers with 0(j + 1)- 0(j) -> 00

as j - oo. Define S(t)Ecjej = Ecjexp(i2e(j)t)e.. Then is a strongly continuous

group with IIS(t)II =1 for all t. If to =

2-e(n)+(v for

n large enough,

IIS(tn)-III <

suplexp(i2o(j)-e(n)+1r)-11

j>0

sup

Iexp(i2o(j)-9(n)+11T)_11

0<j<n-1

sup 2e(j)-9(n)+117

0<j<n-1

2-(9(n)-0(n- 1)) + 17r.

*2.3.8

Example. (Neuberger [1970: 1]).

If S is a group and

limsupllS(t)-III<2,

r-.0

then A must be bounded. For generalizations see Kato [ 1970: 2] and Pazy [ 1971: 2].

As an application of the results in this section, we prove

2.3.9 Lemma.

Let A be densely defined (but not necessarily closed)

and assume that A E C+ and that D = D(A) (that is, every element of D(A) is

the initial value of some solution of (2.3.4). Then A is closable and its closure A

belongs as well to @+, thus A is characterized by Theorem 2.1.1.

Proof.

Let

be the solution operator of (2.3.4) and B the

infinitesimal generator of (the strongly continuous semigroup) S. Clearly

2.3. Semigroup Theory

A c B, so that A is closable and 4-C B. By virtue of Theorem 2.3.1, B E (2,

and it is not difficult to see that the inclusion A c_ A c B and the fact that

A, B E-= (2+ imply that A E e+. But then, in view of Theorem 2.1.1 both

R(X; A) and R(X; B) exist for A large enough. If A $ B, let A E p(A)f p(B),

uED(B), u D(A), vED(A) such that (AI-A)v=(AI-B)u. Then if

w = u - v, w - 0 and (XI - B)w = 0, which is a contradiction.

2.3.10 Example. Lemma 2.3.5 can be proved in a fairly direct way, without

recourse to semigroup theory. In fact, let

be a semigroup continuous at t = 0 in

the norm of (E). Then S is (E)-continuous in t > 0 (this follows from (2.3.1)) and

t f'S(s)ds-III <l

for t sufficiently small so that j'S(s) u ds is nonsingular. Let now t = nh. Then

ftS(t)ds= lim h E S((j-1)h),

o n oo j=1

hence it follows that hES((j -1)h) is invertible for t, h small enough. Now,

h(S(h)-I)h Y_ S((j-1)h)=S(t)-I,

j=I

therefore h- '(S(h) - I) has a limit A e (E) in the norm of (E) and

A f'S(s)ds=S(t)-I

for t,<8 small enough, so that

is differentiable in the norm of (E) and

S'(t) = AS(t) in 0 < t < S. It follows from the remarks on uniqueness in Example

1.2.2 that S(t) = S(t) = exp(tA) for t < S, hence for all t since S(t) = S(t/n)n, and

a similar equation holds for S.

In the next four examples S(.) is a strongly continuous semigroup

and A its infinitesimal generator (or, equivalently, A is a closed densely

defined operator such that the Cauchy problem for (2.3.4) is properly posed

and S(.) is the propagator of (2.3.4).)

*2.3.11 Example (see Hille-Phillips [1957: 1, p. 3121).

Let h > 0, u E E.

Then

exp(th-1(S(h)-I))u-S(t)uash-0

(2.3.19)

uniformly on compacts of t > 0.

2.3.12 Example.

Using the previous example, it is possible to prove the

Weierstrass approximation theorem for continuous functions: if f is continu-

ous in a < x < b, there exists a sequence of polynomials pn such that pn - f

uniformly in a < t < b (place the interval conveniently, extend f to a

function in Co(- oo, oo) and apply (2.3.19) to the semigroup in Example

2.2.4).

86 Properly Posed Cauchy Problems: General Theory

2.3.13 Example (see Dunford-Schwartz [1958: 1, p. 624]).

For u E E,

lira exp(tXAR(X))u=S(t)u (2.3.20)

uniformly on compacts of t >, 0.

2.3.14 Example.

Let u, v E E such that h ' (S(h) - I) u -* v E *-weakly.

Then h-'(S(h)- I) u -* v in the norm of E (so that u E D(A) and Au = v).

*2.3.15 Example.

Let (S(t );

t > 0) be a (E)-valued function satisfying

both equations 2.3.1 and such that S(-) u is strongly measurable in E for

t >, 0 for all u E E. Then t - S(t)u is continuous in t > 0 for all u E E (see

Hille-Phillips [1957: 1, p. 305]). Continuity at t = 0 does not follow. By the

uniform boundedness principle, I I S(t )I I is bounded on compacts of t > 0 and

essentially as in the strongly continuous case it can be proved that IIS(t)II

grows exponentially at infinity. On the other hand, weak measurability of

(measurability of (u*, S(t)u) in t > 0 for all u E E, u* (-= E*) does not

imply any continuity properties of S(.) in t > 0 or even boundedness of

IIS(t)II in any subinterval of (0, oo) (loc. cit. p. 305 and references there).

2.3.16 Example.

Let (S(t); - oo < t < oo) be a strongly measurable group

in the sense defined in the previous example. Then S(.) is strongly continu-

ousin -oo<t<oo.

*2.3.17

Example.

Given two real numbers co, < w2, show that there exists

a strongly continuous semigroup with infinitesimal generator A such

that (a) a (A) is contained in the half plane Re X < w , and (b)

does not

satisfy

IIS(t)II <Ce"Z` (t,0)

for any constant C; compare with Example 1.2.3 and Example 2.2.8 (see

Hille-Phillips [1957: 1, p. 665], where a(A) is in fact empty; for another

example see Zabczyk [1975: 11).

2.4. THE INHOMOGENEOUS EQUATION

Let f be a continuous function defined in t >_ 0 with values in E, and let

A E (2+. Consider the equation

u'(t) = Au(t) + f(t).

(2.4.1)

Solutions of (2.4.1) are defined exactly as solutions of the homogeneous

equation (f = 0) were defined in Section 1.2. Since the difference of two

solutions of (2.4.1) is a solution of the homogeneous equation, it is clear that

there is (if any) only one solution of (2.4.1) satisfying the initial condition

u(0) = uo. (2.4.2)