Eschrig H. Topology and Geometry for Physics

Подождите немного. Документ загружается.

vðjcjÞ ¼

ð1Þ

ðcÞ¼

ð1Þ

ðjcjÞ: ð5:63Þ

The middle expression of this relation is called Euler’s characteristic v of the

polyhedron jcj: Leonard Euler observed that for any closed three-dimensional

ordinary polyhedron a

ðcÞa

ðcÞþa

ðcÞ¼2 holds (number of corners 

number of edges þ number of faces), even if arbitrary polygons are considered as

faces of the polyhedron (their number being a

ðcÞ). If a polygon with n corners is

divided into triangles, n 3 more edges (legs of the triangles) and n 3 more

faces (n  2 triangles instead of the single polygon) are introduced cancelling in

the ﬁrst sum (5.63). Figure 5.9 shows as an example the subdivision of a pentagon

into three triangles by introducing two more legs. The number of triangles equals

the number of pentagons (one) plus 2 ¼ 5 3:

The surface of an ordinary polyhedron is homeomorphic to a 2-sphere. The

latter has Betti numbers b

¼ 1; b

¼ 0; b

¼ 1 yielding 2 in the second sum of

(5.63). Thus, Euler found a topological invariant in the 18th century.

Proof of the second equality of (5.63) Observe the simple facts that for a

homomorphism of vector spaces f : X ! Y the relation dim X ¼ dim Im f þ

dim Ker f holds, and that for a chain complex

0 ! Z

¼ Ker d

! C

! B

r1

¼ Im d

! 0

is an exact sequence. Hence, dim C

¼ dim Z

þ dim B

r1

where for an abstract

complex dim C

ðc; RÞ¼a

since C

ðc; RÞ is spanned by the abstract r-simplices.

On the other hand (again for vector spaces), H

¼ Z

implies

dim H

¼ dim Z

 dim B

, and Poincaré’s original deﬁnition of Betti numbers was

¼ dim H

ðc; RÞ: Inserting all that into (5.63) proves equality there. Agreement

with (5.36) comes from the isomorphy (5.58) between singular simplicial chain

homology and abstract simplicial chain homology. h

Since the Betti numbers are topological invariants, Euler’s characteristic is also

a topological invariant, and the ﬁrst sum of (5.63) is independent of the ‘trian-

gulation’ of the polyhedron, its subdivision into simplices, and also is the same for

homeomorphic polyhedrons. The second sum is deﬁned for any space homotopy

equivalent to a polyhedron, and so is Euler’s characteristic. Moreover, combining

the last sum of (5.63) with Poincaré’s duality (5.61) and using of de Rham’s

theorem leads immediately to the result that Euler’s characteristic of a compact

orientable manifold of odd dimension is zero.

Fig. 5.9 A pentagon

subdivided into three

triangles

5.7 Euler’s Characteristic 147

Euler’s characteristic has many applications in topology and geometry. The

above proof ﬁts into a much more general scheme. Consider the category of

K-modules and a ﬁxed Abelian group A: Consider further an Euler–Poincaré

mapping / of K-modules into A, that is a mapping with the following property:

For every exact sequence 0 ! M

! M ! M

! 0 of K-modules, if /ðM

Þ and

/ðM

Þ exist, then /ðMÞ exists and /ðMÞ¼/ðM

Þþ/ðM

Þ: (For R-vector spaces

M; A ¼ Z and /ðMÞ¼dim M this is obviously the case of the proof above.) Now,

let 0 ! C!

D!

E ! 0 be an exact sequence of chain complexes of K-modules

with morphisms f and g of degree 0 like in (5.54) (there it was written for the

cochain case which just means a sign reversion of the degree of grading). Deﬁne

the characteristic v

ðCÞ¼

ð1Þ

/ðC

Þ: Then, if the characteristic v

deﬁned for two of the complexes C; D; E, then it is deﬁned for the third one and

ðDÞ¼v

ðCÞþv

ðEÞ: This results from the existence of the long exact

sequence H of (5.57) which can be viewed as a chain complex of K-modules with

trivial homology (all H

ðHÞ¼f0g) in which each H

ðCÞ or H

ðDÞ or H

ðEÞ is

placed between modules H

or H

r1

of the two other chains C; D; E: Since because

of the triviality 0 ¼ v

ðHÞ¼v

ðCÞv

ðDÞþv

ðEÞ (all /ðH

ðHÞÞ ¼ 0), the

above statement follows.

If one deﬁnes B

ðc; RÞ¼B

r1

ðc; RÞ and considers the exact sequence 0 !

Zðc; RÞ!

Cðc; RÞ!

ðc; RÞ!0 of chain complexes of real vector spaces with

the canonical injection i and the boundary operator o of the simplicial complex

which due to the deﬁnition of the grading of B

is of degree 0, then without

thinking one obtains (with /ðMÞ¼dim M) v

dim

ðCÞ¼v

dim

ðZÞþv

dim

ðB

Þ¼

dim

ðZÞv

dim

ðBÞ¼v

dim

ðZ=BÞ which is again (5.63).

Given a set A of modules over the same ring, deﬁned up to isomorphism and

such that for every exact sequence 0 ! M

! M ! M

! 0 the module M

belongs to A; if M

and M

belong to A; the set of Euler–Poincaré mappings

ð/; AÞ has a universal element ðc; KðAÞÞ; that is, an Abelian group KðAÞ and a

mapping c so that every A is a subgroup of KðAÞ with injection i and / ¼ i c.

KðAÞ is Grothendieck’s K-group of A. K-theory is another powerful tool to prove

theorems in algebra and topology.

5.8 Critical Points

As an application of (co)homology theory of great relevance in physics, the Morse

theory of critical points of smooth real functions on smooth manifolds is con-

sidered. Again the adjective smooth is dropped in the text.

Let M be an m-dimensional manifold and F 2CðMÞ be a real function on M:

Let x

2 M and let U

 M be a coordinate neighborhood of x

with coordinates

ðx

; ...; x

Þ: The subscript a is dropped where there is no risk of clarity.

148 5 Integration, Homology and Cohomology

The restriction Fj

is given by F

ðx

; ...; x

Þu

: A critical point x

of F is a

point where the differential dF vanishes, in local coordinates



¼ 0; i ¼ 1; ...; m: ð5:64Þ

This deﬁnition, like dF, is independent of the actual local coordinate system a, since

the Jacobian matrix ðw

1

of the second line of (3.7) is regular between two local

coordinate systems. The real value of F at the critical point is a critical value Fðx

Þ:

The critical point x

is non-degenerate, if the Hessian of F

at x



; ð5:65Þ

is a non-degenerate ði; jÞ-matrix. Again, this condition is independent of the used

local coordinate system. The index of the non-degenerate critical point is the

number k

of negative eigenvalues of the Hessian of F

at x

: Since the Hessian is

non-degenerate, it has all eigenvalues non-zero. A family of local coordinate

transformations with regular Jacobian matrices, smoothly depending on parame-

ters, cannot transform the determinant of the Hessian to zero, hence the eigen-

values cannot smoothly change sign depending on local coordinate systems. The

index again is uniquely deﬁned for a function F: Clearly, if the index is 0, F has a

minimum, if the index is m; F has a maximum, and if the index has another value,

F has a saddle point.

Consider a function F that has at most ﬁnitely many critical points on a compact

manifold M without boundary. By the Weierstrass theorem, F takes on its minimal

and maximal values on M: (Would that happen on a boundary, the corresponding

points would not necessarily be critical.) Denote by M

the subset F

1

ðð1; cÞÞ of

M, that is, the set of points x 2 M with values FðxÞ\c, and denote by S

its boundary

given by FðxÞ¼c: Clearly, M

 M

for c

c: One may think of M as an m-

dimensional generalization of a geographical surface of a porous ground and F as a

gravitational potential. If this geography is gradually ﬂooded up to sea level c (in

terms of a gravitational potential level), then M

is the part of the geography under

water. (In fact just this problem was analyzed for m ¼ 2 in a paper by J. C. Maxwell

in 1870 that can be regarded as the early root of Morse theory.)

In the following, local coordinates are used and the subscript a is dropped

throughout. In Chap. 9 it will be shown, that in every smooth manifold a

Riemannian metric g

; g

¼ d

; (p. 107) can be introduced. Consider the tangent

vector ﬁeld, in local coordinates given by

¼uðxÞg



1

ð5:66Þ

(Einstein summation understood). At non-critical points it is parallel to the tangent

vector g

oF=ox

and hence in the Riemannian metric orthogonal to S

FðxÞ

5.8 Critical Points 149

(The Riemannian metric is needed to ensure that this expression is regular at non-

critical points.) At critical points the right hand side of (5.66) is not deﬁned.

Therefore, the smooth non-negative prefactor uðxÞ is introduced which is deﬁned

to be unity outside 2d-balls centered at all critical points and zero inside the

corresponding d-balls. The right hand side of (5.66) is deﬁned to vanish inside

those d-balls. This vector ﬁeld can be integrated to a local 1-parameter group /

ðxÞ

with the obvious property

dFð/

ðxÞÞ

¼uðxÞ0 ð5:67Þ

for which purpose it was constructed. For t 0; /

maps every set M

into itself.

Let c

[ c such that for some small  the interval ðc ; c

þ Þ does not contain

critical values of F: Then, d can be chosen small enough so that uðxÞ¼1 on

n M

since for any real interval of F-values there are at most ﬁnitely many

critical points. Take t 2½0; c

 c and integrate (5.67)toFð/

c

ðxÞÞ  FðxÞ¼

c  c

for x 2 S

: Hence, /

c

maps S

into S

: Likewise it is seen that it maps

continuously (by the integral ﬂow of a smooth tangent vector ﬁeld) M

into M

Generally, from 0 u 1 in (5.67) it follows that jFð/

ðxÞÞ  FðxÞjjtj, hence

cc

¼ð/

c

1

maps continuously M

into M

: It follows that M

and M

are

homeomorphic.

A topological space M is called of category k ¼ catðMÞ, if it can be covered

with k contractible subsets of M but not with fewer number. A sphere S

; n [ 0 for

instance is of category 2, catðS

Þ¼2, since it is not itself contractible, but can be

covered with two contractible half-spheres. Category is a topological property,

homeomorphic spaces like for instance M

and M

above have the same category.

If c is a critical value corresponding to r critical points, then for small enough 

so that there are no more critical values in the interval ðc 2; c þ 2Þ, by the

same analysis a ﬂow /

from M

cþ

into itself is constructed. Choose u such that

the 2d-balls B

; i ¼ 1;...r, around the r critical points do not overlap M

c

and

each other and are inside M

cþ

: Then, /

provides a ﬂow of parts of M

cþ

into all of

c

 M

cþ

and of parts into the B

 M

cþ

: Take the contraction by this ﬂow to

see that catðM

c

Þþr contractible sets cover M

cþ

: Of course, several of them

may be covered by one contractible set, hence catðM

cþ

ÞcatðM

c

Þþr: Now,

start with c

\ min

FðxÞ: Then, M

¼ £ ; catðM

Þ¼0: By continuously

increasing c to a value c

[ max

FðxÞ, for which M

¼ M; catðM

Þ may jump at

most CðF : M ! RÞ times by one, where CðF : M ! RÞ is the number of critical

points of F: (Up to here they need not be non-degenerate.) The result is

CðF : M ! RÞcatðMÞ: ð5:68Þ

It is easily seen that for this result it would sufﬁce that M is any manifold, with

catðMÞ either ﬁnite or þ1, and that F would have a minimum and in every ﬁnite

real interval at most ﬁnitely many critical values each corresponding to ﬁnitely

many critical points.

150 5 Integration, Homology and Cohomology

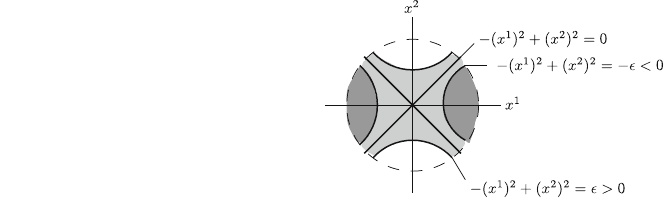
Let x

be a non-degenerate critical point of F of index k with critical value c:

A coordinate neighborhood U

 M centered at x

exists the coordinate image of

which may be chosen to be the open unit ball of R

with

¼ c ðx

ðx

þðx

kþ1

þþðx

þ higher terms:

(Morse observed that always coordinates can be found that all higher terms

vanish.) Figure 5.10 shows the case m ¼ 2 and k ¼ 1:

as an open subset of M is an m-dimensional manifold or empty. The change

of its homology at a critical value c can be studied by the change of homology of

the image of U

\ M

in R

. U

\ M

c

is empty for k ¼ 0, or homotopy

equivalent to a sphere S

k1

, that is two points, in the case of Fig. 5.10), while

\ M

cþ

is homotopy equivalent to a point for k ¼ 0, or to a ball B

, that is a

horizontal line segment, in the case of Fig. 5.10; think of a third axis x

in that

ﬁgure replacing x

while the new x

-axis should point into the drawing plane, and

put k ¼ 2, which makes the ﬁgure rotational symmetric around the x

-axis and

leads to a circle S

and a disk B

in the ðx

; x

Þ-plane instead of S

and B

along the

-axis).

To proceed, the concept of relative homology is helpful. Let N be a sub-

manifold of M and consider the singular chain complexes on both manifolds. For

short they will be called M-chains and N-chains. Clearly, every N-chain is also an

M-chain, hence C

ðN; RÞ is a subspace of C

ðM; RÞ; and the quotient spaces

ðM; RÞ=C

ðN; RÞ may be considered which form a chain complex in which M-

chains which differ only by an N-chain are identiﬁed. An M-chain whose boundary

is an N-chain represents a cycle in the quotient complex, and an M-chain which

combines with an N-chain to an M-boundary represents a boundary in the quotient

complex. It is readily seen that the boundary operator o for M-chains induces the

boundary operator

: C

ðM; RÞ=C

ðN; RÞ!C

r1

ðM; RÞ=C

r1

ðN; RÞð5:69Þ

and that

0 ! CðN; RÞ!CðM; RÞ!CðM; RÞ=CðN; RÞ!0 ð5:70Þ

is an exact sequence, which according to (5.57) induces the long exact sequence

Fig. 5.10 F

 c in the

image of a coordinate

neighborhood. The image of

\ M

c

is the dark

shadowed area, and that of

\ M

cþ

consists of both

the dark and the light

shadowed areas

5.8 Critical Points 151

!H

ðCðN; RÞÞ ! H

ðCðM; R ÞÞ ! H

ðCðM; R Þ=CðN; RÞÞ

! H

r1

ðCðN; RÞÞ! ð5:71Þ

HðCðM; RÞ=CðN; RÞÞ is called the relative homology of M mod N:

The exactness of the sequence A!

B!

C of vector spaces implies dim B ¼

dim Im g þdim Ker g ¼ dim Im g þ dim Im f (recall Im f ¼ Ker g). Since dim Im g 

dim C and dim Im f dim A, it follows dim B dim A þ dim C: Applied to (5.71)

this yields for the Betti numbers

ðMÞb

ðNÞþb

ðM mod NÞ; b

ðM mod NÞ¼dim H

ðCðM; RÞ=CðN; RÞÞ:

ð5:72Þ

Coming back to the case of a single non-degenerate critical point with index k on

an m-dimensional manifold, it was seen that ðU

\ M

cþ

ÞmodðU

\ M

c

Þ was

homotopy equivalent to B

mod S

k1

which is homotopy equivalent to S

(For instance a two-disc whose circumference is contracted into a point yields a

two-sphere: a piece of textile is tightened into a bag by going the left two steps of

Fig. 2.8 on p. 43 backwards.) The Betti numbers of an empty set are all zero, and

the Betti numbers of S

are b

¼ b

¼ 1 and all others zero (see (5.60)). Starting

with M

1

¼ £ and proceeding to M

¼ M, it is readily seen that

ðF : M ! RÞb

ðMÞ; ð5:73Þ

where C

is the total number of critical points of index k of a function Fona

compact manifold M provided F has only ﬁnitely many non-degenerate critical

points. This is the weak Morse inequality.

Next, following in (5.71) the equalities of dimensions in exact sequences given

before (5.72) from r þ 1 down to r ¼ 0, one ﬁnds (we drop the ﬁeld R for the sake

of shorter writing)

dim ImðH

rþ1

ðCðMÞ=CðNÞÞ ! H

ðCðNÞÞÞ

¼ dim H

ðCðNÞÞ  dim ImðH

ðCðNÞÞ ! H

ðCðMÞÞÞ

¼ b

ðNÞðdim H

ðCðMÞÞ dim ImðH

ðCðMÞÞ ! H

ðCðMÞ=CðNÞÞÞ

¼ b

ðNÞb

ðMÞþdim H

ðCðMÞ=CðNÞÞ

 dim ImðH

ðCðMÞ=CðNÞÞ ! H

r1

ðCðNÞÞÞ

¼ b

ðNÞb

ðMÞþb

ðM mod NÞ

ðb

r1

ðNÞb

r1

ðMÞþb

r1

ðM mod NÞÞþ

Realizing that the leftmost expression of this chain of equations is non-negative

and that all b

1

are zero one may cast this result into

ðMÞc

ðNÞþc

ðM mod NÞ; c

s¼0

ð1Þ

rs

; ð5:74Þ

152 5 Integration, Homology and Cohomology

which analogously to the above consideration yields the strong Morse inequality

s¼0

ð1Þ

ks

ðF : M ! RÞ

s¼0

ð1Þ

ks

ðMÞ: ð5:75Þ

Finally, (5.44) says that the long exact sequence (5.71) becomes trivial at the left

end for r [ m, which yields instead of the inequality (5.74) now an equality for the

Euler characteristics,

vðMÞ¼vðNÞþvðM mod NÞ: ð5:76Þ

This makes (5.75) also into an equality for that case, the so called algebraic

number of critical points of F,

s¼0

ð1Þ

ðF : M ! RÞ¼vðMÞ: ð5:77Þ

Recall that in all these results it was assumed that M is compact and F has only

ﬁnitely many non-degenerate singular points.

However, meanwhile Morse theory has been widely generalized to be exploited

in the theory of non-linear equations.

5.9 Examples from Physics

As a ﬁrst example, classical point mechanics is revisited again (cf. Sect. 3.7 and

the end of Sect. 4.4): It is easily checked, that the Liouville measure s

on the

phase space X can be expressed via the canonical (symplectic) 2-form x as

¼ dq

^^dq

^ dp

^^dp

ð1Þ

ðm1Þm=2

x ^^x

|ﬄﬄﬄﬄﬄﬄﬄﬄ{zﬄﬄﬄﬄﬄﬄﬄﬄ}

m factors

: ð5:78Þ

In the wedge product of m factors x all m! terms with all dq

; dp

distinct survive,

all with the same sign due to the pairing. The (not very important) reordering

according to Liouville’s deﬁnition then yields the sign factor.

Since x ¼dP and dx ¼ 0, the canonical 2-form is exact (coboundary) and

hence closed. The same is true for the 2m-form s

of the Liouville measure:

¼

ð1Þ

ðm1Þm=2

dðP ^x ^^x

|ﬄﬄﬄﬄﬄﬄﬄﬄ{zﬄﬄﬄﬄﬄﬄﬄﬄ}

m1 factors

Þ;

and ds

¼ 0 as a consequence, but also in general as a ð2m þ1Þ-form on the 2m-

dimensional phase space. Hence,

5.8 Critical Points 153

¼

ð1Þ

ðm1Þm=2

P ^x ^^x

|ﬄﬄﬄﬄﬄﬄﬄﬄ{zﬄﬄﬄﬄﬄﬄﬄﬄ}

m1 factors

for every subset U of the phase space X:

Most importantly, from the invariance (4.55)ofx under the time-evolution /

(Hamilton ﬂow), the same invariance of the Liouville measure follows

immediately:



¼ s

: ð5:79Þ

This is Liouville’s theorem which has well known important applications in

statistical physics, and which bears the well known danger of misinterpretation

too.

For more details on classical point mechanics see [3, 4].

Next, Maxwell’s electrodynamics on a four-dimensional manifold of space-

time is considered. The electromagnetic ﬁeld is a 2-form which in Minkowski

coordinates ðy

; y

Þ¼ðt; x

; x

Þ is given as

F ¼

^ dy

¼ E

dt ^ dx

þ E

dt ^ dx

þ E

dt ^ dx

 B

^ dx

 B

^ dx

 B

^ dx

; ð5:80Þ

where units with 

¼ l

¼ c ¼ 1 are used here and in the following (

is the

vacuum permittivity, l

the vacuum permeability and c the vacuum velocity of

light, all in ﬂat space-time).

Maxwell theory was brought into its most concise form half a century ago based

on E. Cartan’s exterior calculus and on Hodge’s duality (p. 121). This form holds

likewise in global Minkowski geometry of a ﬂat space-time as well as in general.

A pseudo-Riemannian geometry is introduced by the non-degenerate sym-

metric covariant metric tensor or fundamental tensor g, in local Minkowski

coordinates given as (in the following we use Einstein’s summation convention)

¼ðdtÞ

ðdx

Þðdx

ðdx

or ðg

Þ¼

0 1



: ð5:81Þ

It deﬁnes an indeﬁnite scalar product, sign carrying lengths, and angles in the

tangent space in the usual way,

ðXjYÞ¼g

; jXj¼ðXjXÞ

1=2

; \ðX; YÞ¼arccos

ðXjYÞ

jXjjYj

for jXj 6¼ 0 6¼jYj:

ð5:82Þ

It further provides a bijection between tangent and cotangent spaces,

¼ g

; g

1

¼ g

; g

¼ d

; ð5:83Þ

154 5 Integration, Homology and Cohomology

for which hx; Yi¼ðXjYÞ holds (cf. (4.24)). A more detailed treatment is given in

Chap. 9.

For the sake of generality, the next relations are given for m dimensions.

The coordinate independence of the scalar product in (5.82) implies g

¼ g

and hence g

¼ g

where the determinant of the

transformation (3.6) from the y

to the y

is the Jacobian J ¼ det w: Taking the

determinant of g yields det g ¼ det g

and hence J ¼jdet g=det g

1=2

: Therefore,

instead of (5.1),

s ¼jdet gj

1=2

^^dy

ð5:84Þ

yields a coordinate independent volume form in the present case. The corre-

sponding alternating covariant tensor is the Levi-Civita pseudo-tensor E

1m

jdet gj

1=2

: Its general components are

i

¼jdet gj

1=2

1m

i

; E

i

jdet gj

1=2

i

1m

; s

¼ sign det g; ð5:85Þ

where the contravariant form according to the bijection (5.83) follows from

1m

¼ g

g

i

¼ g

g

1m

i

jdet gj

1=2

¼ det g

1

jdet gj

1=2

In order to adjust Hodge’s star operator (5.14) to the present more general

case, the second line of (5.14) is written as

x ¼ i

E; ð5:86Þ

which according to (4.30) for an n-form x and any ðm nÞ-form r means

E; ri¼hE; x ^ ri¼E

i

nþ1

i

and hence (cf. (4.23))

ðxÞ

nþ1

i

ðm nÞ!

i

;

ðxÞ

nþ1

i

ðm nÞ!

i

ðm nÞ!

g

j

ð5:87Þ

A second star operation results in

ð  xÞ

k

nþ1

k

ðxÞ

nþ1

k

n!ðm nÞ!

nþ1

k

i

nþ1

k

i

¼ s

1m

nþ1

k

i

nþ1

k

1m

i

¼ s

ð1Þ

nðmnÞ

k

5.9 Examples from Physics 155

First, all items of the i

i

-sums are equal to d

k

1m

k

(no summation)

which cancels the factor 1=n! , and then the summation over the distinct indices

nþ1

k

cancels the other prefactor 1= ðm  nÞ!: The ﬁnal answer is

 ¼ s

ð1Þ

nðmnÞ

: ð5:88Þ

For consistency, the second relation (5.14) is also redeﬁned while the ﬁrst relation

remains in effect:

ð1Þ¼dy

^^dy

; ðdy

^^dy

Þ¼s

: ð5:89Þ

(In (5.14) g

¼ d

was assumed, then, for m ¼ 3; E

ijk

¼ E

ijk

¼ d

123

ijk

: For example,

for the three-dimensional 2-form F

build by the spatial part of (5.80) one has

from the ﬁrst equation (5.87) ðFÞ

¼E

ijk

¼ B

Besides the metric independent derivatives d and L

of exterior forms, the

codifferential operator d : DðMÞ!DðMÞ is introduced, which applied to an n-

form x is given by (sign factors are nasty but unavoidable in oriented manifolds)

dx ¼ð1Þ



1

d  x ¼ s

ð1Þ

mðnþ1Þþ1

 d  x; x 2D

ðMÞ;

dx ¼ð1Þ

mn

 d 

1

ð5:90Þ

The second equation of the ﬁrst line is obtained with (5.88) and ð1Þ

ðnþ1Þn

¼ 1 for

every n: One has x 2D

mn

ðMÞ; d  x 2D

mnþ1

ðMÞ and hence dx d  x 2

n1

ðMÞ: This implies that dF ¼ 0 for a 0-form (function) F: For a 1-form x ^¼X

(cf. (5.82)) and g

¼ d

one has dx ¼ div X; hence the codifferential operator

generalizes the divergence of a vector ﬁeld. The second line of (5.90) is obtained

by substituting 

1

x 2D

mn

ðMÞ for x 2D

ðMÞ and hence m n for n in the ﬁrst

equation of the ﬁrst line and operating with  from the left. It is readily seen that



1

d 

1

d ¼

1

¼0:

The Laplace–Beltrami operator is deﬁned as

D ¼ dd þ dd ð5:91Þ

which applied to a function in a ﬂat metric reduces to the ordinary Laplace

operator, DF ¼ div gradF: Simple rules are

dD ¼ Dd; dD ¼ Dd;  D ¼ D  : ð5:92Þ

The ﬁrst two follow readily from the deﬁnition (5.91) and d

¼ 0 ¼ d

: The last

one follows from corresponding commutation rules dd ¼ dd and dd ¼ dd

which demand just a bit more of straightforward calculations. One also ﬁnds for

x 2D

n1

ðMÞ; r 2D

ðMÞ

dðx ^rÞ¼dx ^r þð1Þ

n1

x ^ d  r ¼ dx ^r  x ^dr ð5:93Þ

where in the last equation the ﬁrst relation (5.90) was used.

156 5 Integration, Homology and Cohomology