Dunajski M. Solitons, Instantons, and Twistors

Подождите немного. Документ загружается.

296 A: Manifolds and topology

following prescription:

( f )(

n) = (cos θ,sin θ f (n)), for

n = (cos θ,sin θ n) ∈ S

so that ( f ) coincides with f on the equator of S

which is the intersection of

with the plane cos θ = 0. The suspension does not change the degree.

Now take any map f : S

−→ S

, and suspend it n − 1 times to get the required

map. For example, in spherical polar coordinates on S

the map (θ,φ) −→

(θ,kφ) has degree k.

Example.Let f : S

−→ S

be given by f

= f

) ∈ R

, | f | = 1, where x

are

local coordinates on S

. Then

deg( f )=

vol(S

)



abc

∧ df

8π



abc

∂

x, (A7)

as the area of the unit two-sphere is 4π and the relation between the area form

in spherical polars and Cartesian coordinates is

sin θdθ ∧ dφ = r

−3

(xdy ∧dz + ydz ∧ dy + zdx ∧ dy),

where 0 ≤ θ<π,0 ≤ φ<2π and

x = r sin θ cos φ, y = r sin θ sin φ, and z = r cos θ.

Example. Let f : X −→ SU(2) = S

, where X is a closed three-manifold. Then

deg( f )=

24π



Tr[( f

−1

df)

], (A8)

as the volume of S

is 2π

A.2.1 Homotopy

A smooth (continuous) homotopy of a map f : M −→ N is a smooth (continuous)

map

F : M × [0, 1] −→ N

such that F [x, 0] = f (x) for all x ∈ M. Each map f

(x):=F (x, t) is said to be

homotopic to f . The notion of homotopy is an equivalence relation and the maps

can be classiﬁed into homotopy classes.

Let π

(M, x

) be the set of homotopy classes of loops, that is, maps f from [0, 1]

to M which are based at x

in the sense that f (0) = f (1) = x

. This space and its

group structure which we are just about to describe do not depend on the choice of

the base point x

if M is path-connected. We will therefore often write π

(M). The

space π

(M) consists of oriented loops, that is, copies of S

in M. It has the strucure

of a group. A composition of two loops f

, and f

is a loop f

∗ f

obtainded by

following f

by f

( f

∗ f

)(t)=

⎧

⎨

⎩

(2t)0≤ t ≤

(2t −1)

≤ t ≤ 1.

A.2 Degree of a map and homotopy 297

The inversion of f is the loop

f (t)= f (1 − t). The group π

(M) is called the

fundamental group of M. By deﬁnition, M is simply connected if this group is

trivial.

Example. π

)=Z. Consider a continuous map f :[0, 2π] −→ S

. The base

condition is f (0) = 0, and the continuity implies f (2π)=2π k, where k ∈

Z.Two

maps f

and f

with the same k are homotopic by the relation

=(1− τ ) f

+ τ f

as f

(2π)=2πk.

The higher homotopy groups π

(M) generalize π

(M) replacing [0, 1] by a

k-dimensional closed disc D

=[0, 1]

. More precisely an element π

(M, x

)isa

homotopy class of maps D

−→ M sending the boundary S

k−1

of D

of the disc to

the point x

. The group operation is introduced as follows:

( f

∗ f

)(t

,...,t



,...,t

k−1

, 2t

)0≤ t

≤

,...,t

k−1

, 2t

− 1)

≤ t

≤ 1,

where we have regarded S

as a quotient space of a cube [0, 1]

obtained by

collapsing the boundary of the cube to a point. Using the last coordinate to deﬁne

the product is immaterial, and one gets the same group operation using any other

coordinate. The group π

(M) is abelian if k > 1. We list various results about

homotopy groups without proofs [21, 43]:

)=Z,π

n+1

)=Z

for n > 2,

)={0} for k < n,π

)=π

k+1

n+1

) for k < 2n − 1.

In particular the last two relations imply that S

is simply connected for n > 1.

The formulae (A5) and (A6) allow us to compute the degree of a smooth map.

The case of particular interest is M

= M

= S

. How about maps which are merely

continuous? Any continuous map from S

to itself is homotopic to some smooth

map [43], which allows us to deﬁne the degree of a continuous map f to be degree

of any smooth map homotopic to f . This means that two continuous maps from

to itself are homotopic iff they have the same degree, and so the degree is

an effective way of computing the homotopy class of a map. One consequence

important in soliton theory is that a map from S

to itself of degree 0 is homotopic

to a constant.

In Section 8.2.3 we will need the following result:

Proposition A.2.3 Let g

and g

be maps from S

to U(n) and let g

−→ U(n) be given by

(x):=g

(x)g

(x), x ∈ S

where the product on the RHS is the point-wise group multiplication. Then

]=[g

]+[g

], (A9)

298 A: Manifolds and topology

where

[g]=

24π



Tr[(g

−1

dg)

Proof This result holds because

Tr{[(g

)

−1

d(g

)]

} = Tr[(g

−1

)

+(g

−1

)

]+dβ,

where β is a two-form, and so dβ integrates to 0 by Stokes’ theorem. This was

explicitly demonstrated by Skyrme [147] in the case of SU(2).

Rather than exhibiting the exact form of β we shall use the following general

argument. The higher homotopy groups π

(G) of a Lie group G are abelian, and

the group multiplication in G induces the addition in the homotopy groups: if g

and g

are maps from S

to G then the homotopy class of the map g

: S

−→ G

deﬁned by the group multiplication is the sum of homotopy classes of g

and g

The proof of this is presented for example in [21] and essentially follows the proof

that the fundamental group of a topological group is abelian. Now π

(G)=Z for

any compact simple Lie group. If G = SU(2) this result just reproduces the calcu-

lation done by Skyrme as two continuous maps from S

to itself are homotopic iff

they have the same topological degree.

A.2.2 Hermitian projectors

We shall give a more detailed computation of the second homotopy group and

the associated topological charge for the complex projective space

n−1

.Weshall

need this in Section 8.2. It is convenient to choose a map and perform calculation

in a local framework. We can represent complex directions in

, which are the

elements of

n−1

, by vectors in C

with their ﬁrst component ﬁxed to 1. Then the

map f deﬁned by

n−1

 (1, f

,..., f

n−1

) −→ ( f

,..., f

n−1

) ∈ C

n−1

(A10)

belongs to the maximal holomorphic atlas of

n−1

. The results do not depend on

the choice of this map. Deﬁne the Hermitian projector to be the matrix R given by

R =

q ⊗ q

†

, where q =

⎛

⎜

⎝

n−1

⎞

⎟

⎠

. (A11)

It satisﬁes R

= R.

Now consider maps R :

→ CP

n−1

which extend from R

to the conformal

compactiﬁcation S

. The topological charge of R is deﬁned by

N = −

2π



Tr( R[R

, R

])dxdy = −

8π



∗

, (A12)

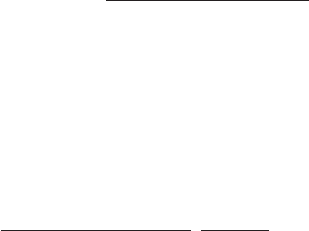
A.2 Degree of a map and homotopy 299

where

 = −4i ∂

∂ ln(1 +



l=1

| f

)=−4i



n−1



l=1

| f



− f



n−1



l=1

| f



∧ d

(A13)

is the Kähler form of the Fubini–Study metric on

n−1

and R

∗

 denotes its

pull-back. The ﬁrst expression for N given in (A12) is often more convenient for

calculations, while the second clariﬁes the topological character. The equality (A12)

is proved by establishing that in the chosen map both expressions give

−i



n−1



k, j =1

(1 +

N−1



l=1

| f

) −

(1 +

n−1



l=1

| f

)

∂( f

)

∂(x, y)

dxdy. (A14)

APPENDIX B

Complex analysis

This appendix introduces some elements of the theory of complex manifolds and

holomorphic vector bundles used in the twistor constructions as well as in other

parts of the book.

A function f : U →

C deﬁned on an open set U ⊂ C is complex differentiable at

λ ∈ U if

lim

h→0

f (λ + h) − f (λ)

exists. A function which is complex differentiable at any point of U is called holo-

morphic on U. Holomorphic functions satisfy the Cauchy–Riemann equations: if

λ = x + iy then

∂ f

∂λ

=0, where

∂

∂λ



∂

∂x

+ i

∂

∂y



If f is holomorphic inside and on an anticlockwise-oriented closed contour  ⊂

then the value of f at any point λ inside  is given by the Cauchy integral formula

f (λ)=

2πi





f (ξ)

ξ − λ

dξ.

It will become clear that the need for the rather sophisticated complex analysis

arises from the need to overcome two classical results: the Liouville theorem and

the maximum modulus theorem.

Theorem B.0.4 (Liouville theorem) Let the function f :

C → C be holomorphic

on the whole complex plane. If f is bounded then f is constant.

Proof The holomorphic function f admits the Taylor expansion around λ =0

convergent on

f (λ)=

∞



m=0

with the coefﬁcients a

are determined by the Cauchy integral formula

2πi



f (ξ)

m+1

dξ

B.1 Complex manifolds 301

where C

is a circle of radius r centred at 0. If there exist M > 0suchthat| f (λ)|≤

M for all λ then the estimate

|≤

2π



| f (ξ )|

|ξ

m+1

|dξ |≤

2π



2π

dθ ≤

holds. The statement now follows from taking a limit r →∞. Thus a

=0ifm > 0

and f = a

is a constant. 

The next closely related result asserts that if a modulus of a holomorphic function

attains a maximum on an open set, then the function is necessarily constant.

Theorem B.0.5 (Maximum modulus theorem) Let the function f be holomorphic

on an open disc with centre λ ∈

C and radius R, and such that | f (ξ )|≤|f (λ)| for

all ξ inside the disc. Then f is constant.

Proof Let C

(r,λ)

be a circle of radius r < R centreed at λ. Setting ξ = λ + re

iθ

the circle, the Cauchy integral formula gives

f (λ)=

2π



2π

f (λ + re

iθ

)dθ.

Taking the absolute values and using the assumptions of the theorem yields

| f (λ)|≤

2π



2π

| f (λ + re

iθ

)|dθ ≤|f (λ)|

and so



2π

[| f (λ)|−|f (r + λe

iθ

)|]dθ =0.

The integrand is continuous and non-negative so it must vanish for all r < R

and θ ∈ [0, 2π ]. Thus | f (λ)| = | f (ξ )| for all ξ inside the disc and f has constant

modulus. The Cauchy–Riemann equations now yield

(∂

f ) f =0

and hence f is a constant.



B.1 Complex manifolds

An n-dimensional complex manifold M is deﬁned as in Deﬁnition A.0.1 where

the transition maps between local coordinate systems

are required to be holo-

morphic. In a neighbourhood U of each point, there exist local holomorphic

coordinates z

(a =1, 2,...,n) such that in the intersection U ∩



U the transi-

tion maps

,...,z

) between two coordinate systems z

and

satisfy the

Cauchy–Riemann (CR) equations ∂

/∂z

= 0 and the non-degeneracy condition

det(∂

/∂z

) =0.

302 B: Complex analysis

Holomorphic functions, vector ﬁelds, and other tensors can be deﬁned as in

the real case by adding the requirement that the components should depend

holomorphically on the coordinates.

Example. Riemann sphere. The two-dimensional sphere is a one-dimensional

complex manifold with local coordinates deﬁned by stereographic projection.

Let (u

, u

) ∈ S

. Deﬁne two open subsets covering S

U = S

−{(0, 0, 1)} and

U = S

−{(0, 0, −1)},

and introduce complex coordinates λ and

λ on U and

U, respectively, by

λ =

+ iu

1 − u

and

λ =

− iu

1+u

The domain of λ is the whole sphere less the north pole; the domain of

λ is the

whole sphere less the south pole. On the overlap U

∩ U

we have

λ =1/λ which

is a holomorphic function.

Example. Projective spaces. The n-dimensional projective space CP

is the quo-

tient of

n+1

by the equivalence relation

, Z

,...,Z

) ∼ (cZ

, cZ

,...,cZ

) for c ∈ C

∗

The homogeneous coordinates Z

label the points uniquely, up to an overall

non-zero complex scaling factor. The complex manifold structure on

introduced by using the inhomogeneous coordinates. On the open set U

in which

= 0, we deﬁne z

(a =1,...,n)by

= Z

,..., z

α−1

= Z

α−1

, z

α+1

= Z

α+1

, ..., z

= Z

We shall use a special notation for the complex projective line

. The homoge-

neous coordinates are denoted by Z

=(Z

, Z

), the two set covering is U

= U

and U

U, and λ = Z

and

λ =1/λ are inhomogeneous coordinates in U

and

U, respectively.

Deﬁnition B.1.1 A holomorphic map f : M →



M is a continuous map such that

for each coordinate chart φ

: U

→ C

on M and

→ C



◦ f ◦

−1

is holomorphic.

Two complex manifolds M and



M are isomorphic if they are diffeomorphic by

a bi-holomorphic diffeomorphism, that is, if f and f

−1

are holomorphic in local

holomorphic coordinates. When n = 1, the projective space is isomorphic to the

Riemann sphere since we can take λ = Z

and

λ = Z

as the two local

coordinates (in the southern and northern hemispheres). An explicit isomorphism

between S

and CP

is given by

, u

) −→ [1 − u

, u

+ iu

]. (B1)

A holomorphic map f : M →

C is called a holomorphic function on M.

B.2 Holomorphic vector bundles and their sections 303

Theorem B.1.2 If M is connected and compact, the only holomorphic functions

on M are the constants.

Proof This follows from the maximum modulus theorem: | f | has to have a

maximum on the compact space M, but in a coordinate neighbourhood of this

point f ◦ φ

−1

is a holomorphic function whose modulus has a maximum in the

interior of an open set in

. Therefore f ◦ φ

−1

is constant by the maximum

modulus theorem (Theorem B.0.5), and so f is constant.



B.2 Holomorphic vector bundles and their sections

To obtain a useful theory of functions on compact complex manifold we have

to introduce holomorphic line (and vector) bundles and their sections. Roughly

speaking, a vector bundle over a manifold is a collection of vector spaces, one at

each point of the manifold

Deﬁnition B.2.1 A holomorphic vector bundle of rank k over a complex manifold

M is a complex manifold E, and a holomorphic projection π : E → M such that

For each z ∈ M, π

−1

(z) is a k-dimensional complex vector space.

Each point z ∈ M has a neighbourhood U

and a homeomorphism χ

such that

the diagram

−1

)

∼

× C

π !

is commutative.

αβ

:= χ

◦ χ

−1

: U

∩ U

→ GL(k, C)

is a holomorphic map to the space of invertible k × k matrices.

Remarks

Rank-one vector bundles are called line bundles.

We call F

αβ

a transition function, or a patching matrix. On the overlap U

∩ U

of two local trivializations we have

(z, u) ∼ (z, u



z, F

αβ

(z)u

, z ∈ U

∩ U

One way that we can specify a holomorphic vector bundle is by giving its transi-

tion maps F

αβ

between the open sets of some open cover U

(α in some indexing

set). These are holomorphic matrix-valued functions on the intersections with

the following properties:

αα

=1, F

αβ

βα

=1, F

αβ

βγ

γα

=1, no summation.

304 B: Complex analysis

The last relation is called the cocyle property, and holds on each triple intersec-

tion:

∩ U

It is not hard to see that every holomorphic vector bundle can be represented in

this way.

There is a certain non-uniqueness in the deﬁnition of transition functions.

Let E be given by (χ

, U

) and



E by ( ˜χ

, U

). Put H

= χ

◦ (˜χ

)

−1

∈

Map(U

, GL(k, C)). Then clearly



αβ

=(H

)

−1

αβ

. (B2)

We shall regard E and



E as equivalent if their transition functions satisfy (B2).

All the algebraic operations on vector spaces can be extended to holomorphic

bundles.

The product E = M ×

is called a trivial vector bundle.

Lemma B.2.2 The bundle is trivial, iff there exist holomorphic splitting matrices

: U

→ GL(k, C)

such that

αβ

= H

−1

. (B3)

Proof If (B3) holds, then F

αβ

is equivalent to Id by the relation (B2), so E is trivial.

If E given by (U

,χ

) is trivial, then there exists a bi-holomorphism χ : E →

M ×

. We deﬁne H

= χ

◦ (χ |

)

−1

, and note that

−1

αβ

=Id.



Deﬁnition B.2.3 A holomorphic section of a vector bundle E over M is a holo-

morphic map s : M → E such that π ◦ s =id

The local description is given by a collection of holomorphic maps s

: U

→ C

z −→ (z, s

(z)), for z ∈ U

with the transition rule s

(z)=F

αβ

(z)s

(z).

In the case of trivial bundles Lemma B.2.2 implies that we can specify global

sections by putting s

(z)=H

(z)ξ for some constant vector ξ .

We denote the space of holomorphic sections over U ⊂ M by (U, E)(or

(U, L)ifE = L is a line bundle). An important theorem (which we shall not

prove) is that (M, E) is ﬁnite-dimensional if M is compact.

Example. Tautological bundle. Consider M = CP

with the usual coordinate

patches U

and U

. First deﬁne a tautological line bundle

O(−1) = {(λ, (Z

, Z

)) ∈ CP

× C

|λ = Z

B.2 Holomorphic vector bundles and their sections 305

If we represent the Riemann sphere as the projective line, as above, then we

have the projection

→ CP

. The ﬁbre above the point with coordinate [Z]is

the one-dimensional line cZ through the origin in

containing the the point

, Z

). Deﬁne the trivializations

([Z], c(Z

, Z

))=([Z], cZ

) ∈ U

× C and

([Z], c(Z

, Z

))=([Z], cZ

) ∈ U

× C,

so that

= F

giving the transition function F

= λ.

Example. Other line bundles can be obtained by algebraic operations:

O(−n)=O(−1)

⊗n

, O(n)=O(−n)

∗

, and O = O(−1) ⊗ O(1), n ∈ N.

The transition function for O(n)isF = λ

−n

on U

∩ U

∼

∗

. A global holomor-

phic section of this line bundle is given by functions s and

s on

C related by

s(λ)=λ

λ)

on the overlap

∗

. Expanding these functions as power series in their respective

local coordinates and using the fact that

λ = λ

−1

,weget

∞



= λ

∞



−m

Equating coefﬁcients, we ﬁnd that

= a

= 0 for m > n and

= a

n−1

etc. Thus the global sections are given by polynomials



of degree less than or equal to n, and hence the space of holomorphic sections is

(n + 1)-dimensional, that is,

(CP

, O(n)) = 0 (n < 0) and dimH

(CP

, O(n)) = n +1 (n ≥ 0).

(B4)

The transition relation implies that

s(λ)(Z

)

λ)(Z

)

so we can deﬁne a function G :

→ C by

G(Z)=s(λ)(Z

)

for [Z] ∈ U

λ)(Z

)

for [Z] ∈ U

Then G is homogeneous of degree n. Conversely any holomorphic function on

homogeneous of degree n gives rise to a section of O(n). A global holomorphic