Dougherty С. Introduction to Econometrics, 3Ed

Подождите немного. Документ загружается.

AUTOCORRELATION

procedure is mainly of historical interest and most applications designed for time series analysis offer

alternative methods.

The output for the logarithmic demand function for housing is shown. You can see that the

regression results are identical to those for the explicit nonlinear specification. Note that the

coefficient of AR(1) is the estimate of

, and corresponds to C(2) in the previous regression.

The Cochrane–Orcutt Iterative Procedure for Eliminating AR(1) Autocorrelation

The starting point for the Cochrane–Orcutt iterative procedure is equation

(13.10), which may be rewritten

ttt

++=

where

−

−=

ttt

YYY

−

−=

ttt

XXX

, and

(1 –

). If you knew the value

, all you would have to do would be to calculate

and

from the data on

and

, and perform a simple regression of

. The coefficient of

would

be a direct estimate of

, and the intercept could be used to derive an estimate of

Of course, you do not know

and it has to be estimated, along with the other

parameters of the model. The Cochrane–Orcutt iterative procedure does this by

assuming that if the disturbance term follows an AR(1) process, the residuals will do

so as well (approximately), and hence a regression of

–1

will yield an estimate

. The procedure involves the following steps:

is regressed on

with the original, untransformed data.

2. The residuals are calculated.

is regressed on

–1

to obtain an estimate of

and

are calculated using this estimate of

and the equation at the top

of the box is fitted. The coefficient of

provides a revised estimate of

and the estimate of

yields a revised estimate of

5. The residuals are recalculated and the process returns to step 3.

The process alternates between revising the estimates of

and

, and revising the

estimate of

, until convergence is obtained, that is, until the estimates at the end o

the latest cycle are the same as the estimates at the end of the previous one, to a

prespecified number of decimal places.

AUTOCORRELATION

============================================================

Dependent Variable: LGHOUS

Method: Least Squares

Sample(adjusted): 1960 1994

Included observations: 35 after adjusting endpoints

Convergence achieved after 6 iterations

LGHOUS=C(1)*(1-C(2))+C(2)*LGHOUS(-1)+C(3)*LGDPI-C(2)*C(3)

*LGDPI(-1)+C(4)*LGPRHOUS-C(2)*C(4)*LGPRHOUS(-1)

============================================================

CoefficientStd. Errort-Statistic Prob.

============================================================

C(1) 6.131576 0.727244 8.431251 0.0000

C(2) 0.972488 0.004167 233.3567 0.0000

C(3) 0.275879 0.078318 3.522532 0.0013

C(4) -0.303387 0.085802 -3.535896 0.0013

============================================================

R-squared 0.999695 Mean dependent var 6.017555

Adjusted R-squared 0.999665 S.D. dependent var 0.362063

S.E. of regression 0.006622 Akaike info criter-7.089483

Sum squared resid 0.001360 Schwarz criterion -6.911729

Log likelihood 128.0660 F-statistic 33865.14

Durbin-Watson stat 1.423030 Prob(F-statistic) 0.000000

============================================================

Dependent Variable: LGHOUS

Method: Least Squares

Sample(adjusted): 1960 1994

Included observations: 35 after adjusting endpoints

Convergence achieved after 24 iterations

============================================================

Variable CoefficientStd. Errort-Statistic Prob.

============================================================

C 6.131573 0.727241 8.431276 0.0000

LGDPI 0.275879 0.078318 3.522534 0.0013

LGPRHOUS -0.303387 0.085802 -3.535896 0.0013

AR(1) 0.972488 0.004167 233.3540 0.0000

============================================================

R-squared 0.999695 Mean dependent var 6.017555

Adjusted R-squared 0.999665 S.D. dependent var 0.362063

S.E. of regression 0.006622 Akaike info criter-7.089483

Sum squared resid 0.001360 Schwarz criterion -6.911729

Log likelihood 128.0660 F-statistic 33865.14

Durbin-Watson stat 1.423031 Prob(F-statistic) 0.000000

============================================================

Inverted AR Roots .97

============================================================

Exercise

13.3

Perform a logarithmic regression of expenditure on your commodity on income and relative

price, first using OLS and then using the option for AR(1) regression. Compare the coefficients

and standard errors of the two regressions and comment.

13.4 Autocorrelation with a Lagged Dependent Variable

Suppose that you have a model in which the dependent variable, lagged one time period, is used as one

of the explanatory variables (for example, a partial adjustment model). When this is the case,

autocorrelation is likely to cause OLS to yield inconsistent estimates.

AUTOCORRELATION

For example, suppose the model is of the form

–1

+ u

(13.17)

If there were no autocorrelation, OLS would yield consistent estimates. Strictly speaking, the use of

the lagged dependent variable will make OLS estimates subject to some element of bias in finite

samples, but in practice this bias is not considered serious and is ignored. However, if the disturbance

term is subject to autocorrelation, the situation is entirely different. We will investigate the case where

is subject to AR(1) autocorrelation

–1

, (13.18)

Then the model may be rewritten

–1

, (13.19)

Lagging (13.17) one period, we see that

–1

–2

+ u

–1

(13.20)

Hence in (13.19) we have a violation of the fourth Gauss–Markov condition. One of the explanatory

variables, Y

–1

, is partly determined by u

–1

, which is also a component of the disturbance term. As a

consequence, OLS will yield inconsistent estimates. It is not hard to obtain an analytical expression

for the large-sample bias, but it is laborious and it will not be attempted here.

Detection of Autocorrelation with a Lagged Dependent Variable

As Durbin and Watson noted in their original article, the Durbin–Watson d statistic is invalid when the

regression equation includes a lagged dependent variable. It tends to be biased towards 2, increasing

the risk of a Type II error. In this case one may use the Durbin h statistic (Durbin, 1970), which is

also computed from the residuals. It is defined as

)1(

−

(13.21)

where

is an estimate of

in the AR(1) process,

)1(

−

s is an estimate of the variance of the

coefficient of the lagged dependent variable Y

–1

, and n is the number of observations in the regression.

Note that n will usually be one less than the number of observations in the sample because the first

observation is lost when the equation is fitted. There are various ways in which one might estimate

but, since this test is valid only for large samples, it does not matter which you use. The most

convenient is to take advantage of the large-sample relationship between d and

→

2 – 2

(13.22)

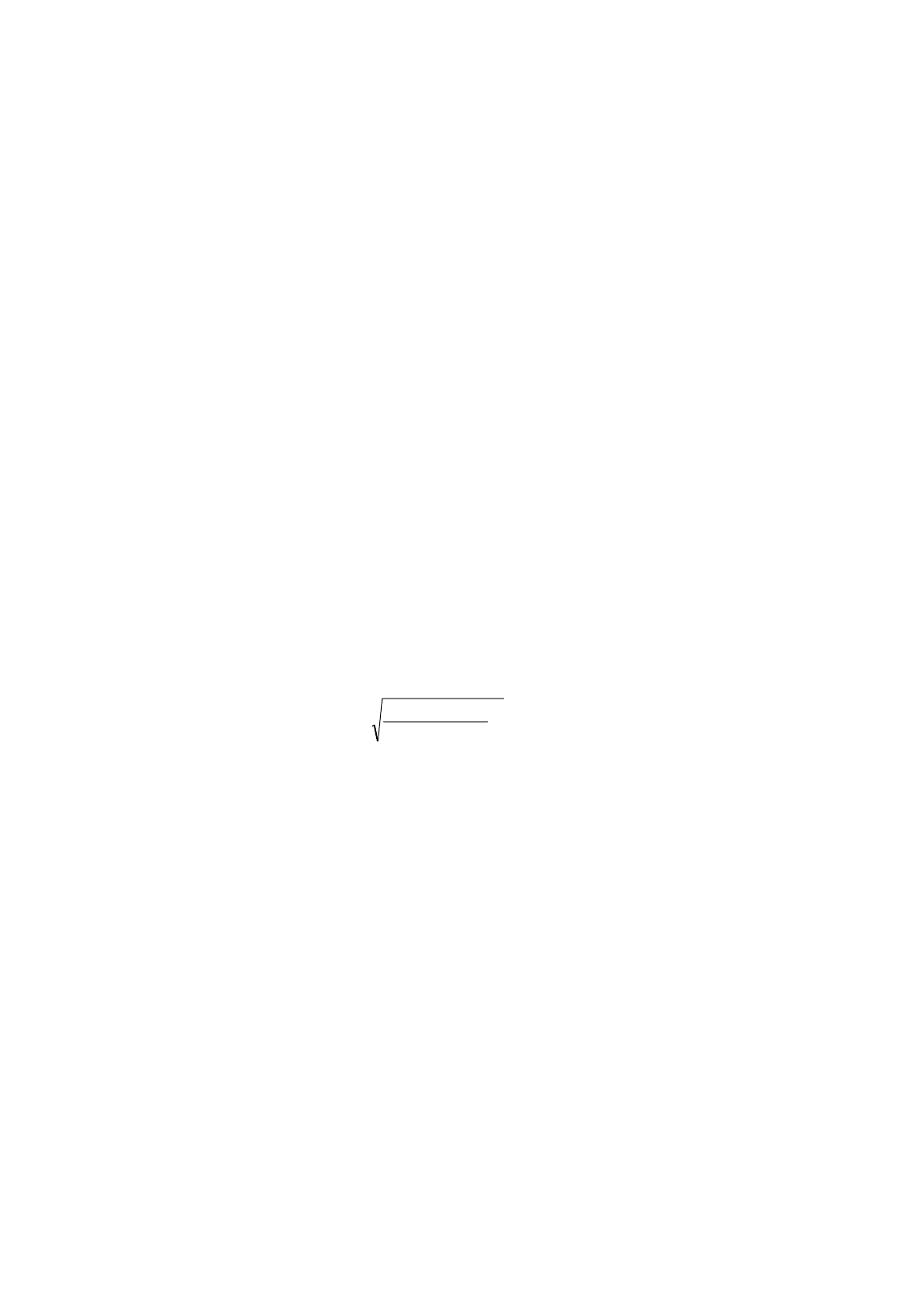
AUTOCORRELATION

From this one estimates

as (1 – ½d). The estimate of the variance of the lagged dependent

variable is obtained by squaring its standard error. Thus h can be calculated from the usual regression

results. In large samples, h is distributed as N(0,1), that is, as a normal variable with mean 0 and unit

variance, under the null hypothesis of no autocorrelation. The hypothesis of no autocorrelation can

therefore be rejected at the 5 percent significance level if the absolute value of h is greater than 1.96,

and at the 1 percent significance level if it is greater than 2.58, using two-tailed tests and a large

sample.

A common problem with this test is that the h statistic cannot be computed if n

s is greater than 1,

which can happen if the sample size is not very large. An even worse problem occurs when n

near to, but less than, 1. In such a situation the h statistic could be enormous, without there being any

problem of autocorrelation. For this reason it is a good idea to keep an eye on the d statistic as well,

despite the fact that it is biased.

Example

The partial adjustment model leads to a specification with a lagged dependent variable. That for the

logarithmic demand function for housing services was used as an exercise in the previous chapter.

The output is reproduced below. The Durbin–Watson statistic is 1.72. (1 – ½d) = 0.14 gives us an

estimate of

. The standard error of the lagged dependent variable is 0.0451. Thus our estimate of the

variance of its coefficient is 0.0020. There are 36 observations in the sample, but the first cannot be

used and n is 35. Hence the h statistic is

86.0

0020.0351

14.0

×−

×=

(13.23)

This is below 1.96 and so, at the 5 percent significance level, we do not reject the null hypothesis of no

autocorrelation (reminding ourselves of course, that this is a large-sample test and we have only 35

observations).

============================================================

Dependent Variable: LGHOUS

Method: Least Squares

Sample(adjusted): 1960 1994

Included observations: 35 after adjusting endpoints

============================================================

Variable CoefficientStd. Errort-Statistic Prob.

============================================================

C -0.390249 0.152989 -2.550839 0.0159

LGDPI 0.313919 0.052510 5.978243 0.0000

LGPRHOUS -0.067547 0.024689 -2.735882 0.0102

LGHOUS(-1) 0.701432 0.045082 15.55895 0.0000

============================================================

R-squared 0.999773 Mean dependent var 6.017555

Adjusted R-squared 0.999751 S.D. dependent var 0.362063

S.E. of regression 0.005718 Akaike info criter-7.383148

Sum squared resid 0.001014 Schwarz criterion -7.205394

Log likelihood 133.2051 F-statistic 45427.98

Durbin-Watson stat 1.718168 Prob(F-statistic) 0.000000

============================================================

AUTOCORRELATION

Autocorrelation in the Partial Adjustment and Adaptive Expectations Models

The partial adjustment model

ttt

uXY

++=

)(

−−

−=−

tttt

YYYY

≤

leads to the regression specification

tttt

uYXY

λλλ

+−++=

−

121

)1(

Hence the disturbance term in the fitted equation is a fixed multiple of that in the first

equation and combining the first two equations to eliminate the unobservable

will no

have introduced any new complication. In particular, it will not have caused the

disturbance term to be autocorrelated, if it is not autocorrelated in the first equation. By

contrast, in the case of the adaptive expectations model,

uXY

++=

121

)(

XXXX

−=−

the Koyck transformation would cause a problem. The fitted equation is then

1211

)1()1(

−−

−−++−+=

ttttt

uuXYY

λλ

and the disturbance term is subject to moving average autocorrelation. We noted that we

could not discriminate between the two models on the basis of the variable specification

because they employ exactly the same variables. Could we instead use the properties o

the disturbance term to discriminate between them? Could we regress

and

–1

test for autocorrelation, and conclude that the dynamics are attributable to a partial

adjustment process if we do not find autocorrelation, and to an adaptive expectations

process if we do?

Unfortunately, this does not work. If we do find autocorrelation, it could be that the

true model is a partial adjustment process, and that the original disturbance term was

autocorrelated. Similarly, the absence of autocorrelation does not rule out an adaptive

expectations process. Suppose that the disturbance term

is subject to AR(1)

autocorrelation:

AUTOCORRELATION

13.5 The Common Factor Test

We now return to the ordinary AR(1) model to investigate it a little further. The nonlinear equation

(1 –

) +

–1

–

–1

, (13.24)

fitted on the hypothesis that the disturbance term is subject to AR(1) autocorrelation, is a restricted

version of the more general ADL(1,1) model (autoregressive distributed lag, the first argument

referring to the maximum lag in the Y variable and the second to the maximum lag in the X

variable(s))

–1

, (13.25)

with the restriction

= –

. (13.26)

The presence of this implicit restriction provides us with an opportunity to test the validity of the

model specification. This will help us to discriminate between cases where the d statistic is low

because the disturbance term is genuinely subject to an AR(1) process and cases where it is low for

other reasons. The theory behind the test procedure will not be presented here (for a summary, see

Hendry and Mizon, 1978), but you should note that the usual F test of a restriction is not appropriate

because the restriction is nonlinear. Instead we calculate the statistic

Autocorrelation in the Partial Adjustment and Adaptive Expectations Models

(continued)

–1

Then

– (1 –

–1

– (1 –

) u

–1

– (1 –

–

) u

–1

Now it is reasonable to suppose that both

and

will lie between 0 and 1, and hence it is

possible that their sum might be close to 1. If this is the case, the disturbance term in the

fitted model will be approximately equal to

, and the AR(1) autocorrelation will have

been neutralized by the Koyck transformation.

AUTOCORRELATION

n log(RSS

/RSS

) (13.27)

where n is the number of observations in the regression, RSS

and RSS

are the residual sums of

squares from the restricted model (13.24) and the unrestricted model (13.25), and the logarithm is to

base e. Remember that n will usually be one less than the number of observations in the sample

because the first observation is lost when (13.24) and (13.25) are fitted. Strictly speaking, this is a

large sample test. Ii the original model has only one explanatory variable, as in this case, the test

statistic has a chi-squared distribution with one degree of freedom under the null hypothesis that the

restriction is valid. As we saw in the previous section, if we had started with the more general model

+ ... +

+ u

, (13.28)

the restricted model would have been

(1 –

) +

–1

–

–1

+ ... +

–

–1

. (13.29)

There are now k – 1 restrictions because the model imposes the restriction that the coefficient of the

lagged value of each explanatory variable is equal to minus the coefficient of its current value

multiplied by the coefficient of the lagged dependent variable Y

–1

. Under the null hypothesis that the

restrictions are valid, the test statistic has a chi-squared distribution with k – 1 degrees of freedom.

If the null hypothesis is not rejected, we conclude that the AR(1) model is an adequate

specification of the data. If it is rejected, we have to work with the unrestricted ADL(1,1) model

–1

+ ... +

–1

, (13.30)

including the lagged value of Y and the lagged values of all the explanatory variables as regressors.

The problem of multicollinearity will often be encountered when fitting the unrestricted model,

especially if there are several explanatory variables. Sometimes it can be alleviated by dropping those

variables that do not have significant coefficients, but precisely because multicollinearity causes t

statistics to be low, there is a risk that you will end up dropping variables that do genuinely belong in

the model.

Two further points. First, if the null hypothesis is not rejected, the coefficient of Y

–1

may be

interpreted as an estimate of

. If it is rejected, the whole of the AR(1) story is abandoned and the

coefficient of Y

–1

in the unrestricted version does not have any special interpretation. Second, when

fitting the restricted version using the specification appropriate for AR(1) autocorrelation, the

coefficients of the lagged explanatory variables are not reported. If for some reason you need them,

you could calculate them easily yourself, as minus the product of the coefficient of Y

–1

and the

coefficients of the corresponding current explanatory variables. The fact that the lagged variables,

other than Y

–1

, do not appear explicitly in the regression output does not mean that they have not been

included. They have.

Example

The output for the AR(1) regression for housing services has been shown above. The residual sum of

squares was 0.001360. The unrestricted version of the model yields the following result:

AUTOCORRELATION

============================================================

Dependent Variable: LGHOUS

Method: Least Squares

Sample(adjusted): 1960 1994

Included observations: 35 after adjusting endpoints

============================================================

Variable CoefficientStd. Errort-Statistic Prob.

============================================================

C -0.386286 0.177312 -2.178563 0.0376

LGDPI 0.301400 0.066582 4.526717 0.0001

LGPRHOUS -0.192404 0.078085 -2.464038 0.0199

LGHOUS(-1) 0.726714 0.064719 11.22884 0.0000

LGDPI(-1) -0.014868 0.092493 -0.160748 0.8734

LGPRHOUS(-1) 0.138894 0.084324 1.647143 0.1103

============================================================

R-squared 0.999797 Mean dependent var 6.017555

Adjusted R-squared 0.999762 S.D. dependent var 0.362063

S.E. of regression 0.005589 Akaike info criter-7.381273

Sum squared resid 0.000906 Schwarz criterion -7.114642

Log likelihood 135.1723 F-statistic 28532.58

Durbin-Watson stat 1.517562 Prob(F-statistic) 0.000000

============================================================

Before we perform the common factor test, we should check that the unrestricted model is free

from autocorrelation. Otherwise neither it nor the AR(1) model would be satisfactory specifications.

The h statistic is given by

54.1

0042.0351

24.0

×−

×=

(13.31)

This is below 1.96 and so we do not reject the null hypothesis of no autocorrelation.

Next we will check whether the coefficients appear to satisfy the restrictions implicit in the AR(1)

model. Minus the product of the lagged dependent variable and the income elasticity is –

0.7267

0.3014 = –0.22. The coefficient of lagged income is numerically much lower than this.

Minus the product of the lagged dependent variable and the price elasticity is –0.7267

–0.1924 =

0.14, which is identical to the coefficient of lagged price, to two decimal places. Hence the restriction

for the price side of the model appears to be satisfied, but that for the income side does not.

The common factor test confirms this preliminary observation. The residual sum of squares has

fallen to 0.000906. The test statistic is 35 log(0.001360/0.000906) = 14.22. The critical value of chi-

squared at the 0.1 percent level with two degrees of freedom is 13.82, so we reject the restrictions

implicit in the AR(1) model and conclude that we should use the unrestricted ADL(1,1) model instead.

We note that the lagged income and price variables in the unrestricted model do not have

significant coefficients, so we consider dropping them and arrive at the partial adjustment model

specification already considered above. As we saw, the h statistic is 0.86, and we conclude that this is

a satisfactory specification.

Exercises

13.4

A researcher has annual data on the rate of growth of aggregate consumer expenditure on

financial services, f

, the rate of growth of aggregate disposable personal income, x

, and the rate

of growth of the relative price index for consumer expenditure on financial services, p

, for the

AUTOCORRELATION

United States for the period 1959-1994 and fits the following regressions (standard errors in

parentheses; method of estimation as indicated):

1: OLS 2: AR(1) 3: OLS 4: OLS

1.20

(0.06)

1.31

(0.11)

1.37

(0.17)

0.32

(0.10)

–0.10

(0.03)

–0.21

(0.06)

–0.25

(0.08)

–0.11

(0.07)

(–1) - -

0.61

(0.17)

0.75

(0.15)

(–1) - -

–0.90

(0.35)

(–1) - -

–0.11

(0.09)

constant 0.01

(0.05)

–0.04

(0.09)

-0.05

(0.10)

0.03

(0.08)

-0.55- -

0.80 0.82 0.84 0.81

RSS

40.1 35.2 32.1 37.1

0.74 1.78 1.75 1.26

Explain the relationship between the second and third specifications, perform a common factor

test, and discuss the adequacy of each specification.

13.5

Perform a logarithmic regression of expenditure on your category of consumer expenditure on

income and price using an AR(1) estimation technique. Perform a second regression with the

same variables but adding the lagged variables as regressors and using OLS. With an

test,

check that the second specification is not subject to autocorrelation.

Explain why the first regression is a restricted version of the second, stating the restrictions,

and check whether the restrictions appear to be satisfied by the estimates of the coefficients of

the second regression. Perform a common factor test. If the AR(1) model is rejected, and there

are terms with insignificant coefficients in the second regression, investigate the consequences

of dropping them.

13.6*

Year Y K L Year Y K L

1899

100 100 100

1911

153 216 145

1900

101 107 105

1912

177 226 152

1901

112 114 110

1913

184 236 154

1902

122 122 118

1914

169 244 149

1903

124 131 123

1915

189 266 154

1904

122 138 116

1916

225 298 182

1905

143 149 125

1917

227 335 196

1906

152 163 133

1918

223 366 200

1907

151 176 138

1919

218 387 193

1908

126 185 121

1920

231 407 193

1909

155 198 140

1921

179 417 147

1910

159 208 144

1922

240 431 161

Source

: Cobb and Douglas (1928)

The table gives the data used by Cobb and Douglas (1928) to fit the original Cobb-Douglas

production function:

AUTOCORRELATION

tttt

vLKY

ββ

, and

, being index number series for real output, real capital input, and real labor input,

respectively, for the manufacturing sector of the United States for the period 1899–1922

(1967=100). The model was linearized by taking logarithms of both sides and the following

regressions were run (standard errors in parentheses; method of estimation as indicated):

1: OLS 2: AR(1) 3: OLS

log

0.23

(0.06)

0.22

(0.07)

0.18

(0.56)

log

0.81

(0.15)

0.86

(0.16)

1.03

(0.15)

log

(–1) - -

0.40

(0.21)

log

(–1) - -

0.17

(0.51)

log

(–1) - -

–1.01

(0.25)

constant –0.18

(0.43)

–0.35

(0.51)

1.04

(0.41)

0.19

(0.25)

0.96 0.96 0.98

RSS

0.0710 0.0697 0.0259

1.52 1.54 1.46

Evaluate the three regression specifications.

13.6 Apparent Autocorrelation

As has been seen above, a positive correlation among the residuals from a regression, and a

correspondingly low Durbin–Watson statistic, may be attributable to the omission of one or more

lagged variables from the model specification, rather than to an autocorrelated disturbance term. We

will describe this as

apparent

autocorrelation. Although the examples above relate to the omission of

lagged variables, it could arise from the omission of any important variable from the regression

specification.

Apparent autocorrelation can also arise from functional misspecification. For example, we saw in

Section 5.1 that, if the true model is of the form

++=

(13.32)

and we execute a linear regression, we obtain the fit illustrated in Figure 5.1 and summarized in Table

5.2: a negative residual in the first observation, positive residuals in the next six, and negative

residuals in the last three. In other words, there appears to be very strong positive autocorrelation.

However, when the regression is of the form