Doktorov E.V., Leble S.B. A Dressing Method in Mathematical Physics
Подождите немного. Документ загружается.

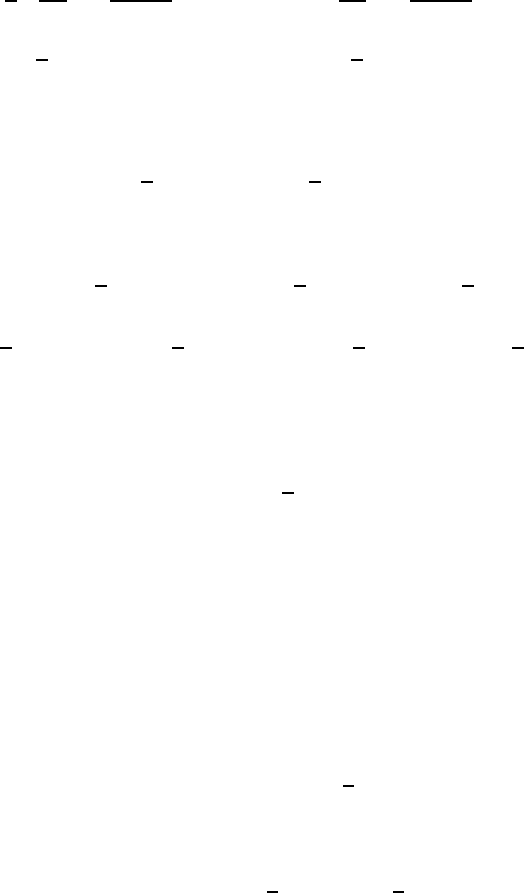
10.1 Nonlinear equations with singular dispersion relations: 1+1 dimensions 329
=
1
k
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
(ψ
−1
)
T
(ℓ)R
T
(ℓ) −
1
2πi
dℓ ∧ d
¯
ℓ
ℓ
(ψ
−1
)
T
(ℓ)R
T
(ℓ)
=
1
k
(ψ
−1
)
T
R
T
C
k
− (ψ
−1
)
T
R
T
C
0
=
1
k
(ψ
−1
)
T
(0) − (ψ
−1
)
T
(k)
,
because (ψ
−1
)
T
R
T
C
k
= 11 −(ψ
−1
)
T
. Therefore, (1/k)(ψ
−1
)
T
(11+R
T
C
k
)
−1
=
k
−1
(ψ
−1
)
T
(0), or
1
k
(11+R
T
C
k
)
−1
=
1
k
ψ
T
(0)(ψ
−1
)
T
(k). (10.38)
Continuing the calculation in (10.37), we get
g
t
= ψR
t
,
1
k
ψ
T
(0)(ψ
−1
)
T
(k) =
1
k
ψRΩψ
−1
, 11g −
1
k
ψΩR,(ψ
−1
)
T
g
=
1
k
(
¯
∂ψ)Ωψ
−1
, 11g+
1
k
ψΩ,
¯
∂(ψ
−1
)
T
g =
1
k
¯
∂(ψΩ
p
ψ
−1
)g−
1
k
ω
s
ψσ
3
ψ
−1
g.
Consider first the polynomial dispersion relation Ω
p
= α
n
k
n
σ
3
. Because
g(x, t) does not depend on k, we can insert it into the brackets ···.Then
g
−1
g
t
= α
n
(1/k)
¯
∂(k
n
ϕσ
3
ϕ
−1
).Asaresult,
S
t
= α
n
(
S,
1
k
¯
∂(k
n
ϕσ
3
ϕ
−1
)
)
. (10.39)
Denote ϕσ
3
ϕ
−1
= M,thenM
x
= −ik[S, M]. Let us introduce a moving
trihedral element ˜σ
±
= g
−1
σ
±
g and ˜σ
3
= g
−1
σ
3
g = S,whereσ
±
= σ
1
± iσ
2
are Pauli matrices, and a covariant derivative
∇
x
M = M
x
+[g
−1
g
x
, M].
The trihedral elements are covariantly constant, ∇
x
˜σ
α
= 0; therefore, we can
write ∇
−1
x
α
˜σ
α
Q
α
=
α
˜σ
α
x
dyQ
α
(y).Itcanbeeasilyshownthatg
−1
g
x
iswrittenintermsofS: g
−1
g
x
=(1/4)[S, S
x
]; hence,
∇
x
M = −ik[S, M]+
1
4
[S, [S, M]] . (10.40)
Nowwedecomposethe2× 2matrixM into “diagonal” and “off-diagonal”
parts,
M = M
d
+ M
a
=
1
2
S tr(MS)+
1
4
[S, [S, M]] ;
cf. (10.21). The quotation marks are relevant because diagonality and off-
diagonality are used here with respect to the moving trihedral elements. In
other words, S = S
d
,[S
d
, M
d
]=0,[S, M
a
]=2SM
a
, etc., but the upper
indices d and a do not mean the usual diagonality and off-diagonality.
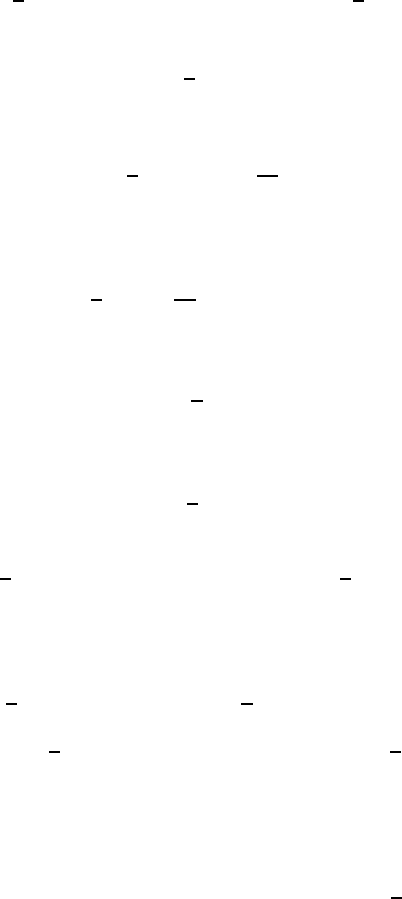
330 10 Generating solutions via
¯
∂ problem
Decomposing (10.40) yields
∇
x
M
d
=
1
4
[[S, S
x
], M
a
] , ∇
x
M
a
+2ikSM
a
=
1
4
[S, S
x
], M
d
. (10.41)
Hence,
M
d
= S +
1
4
x
dy [[S, S
y
], M
a
(y)] .
Inserting this M
d
into the second equation in (10.41), we get
∇
x
M
a
+2ikSM
a
=
1
4
[[S, S
x
],S]+
1
16
(
[S, S
x
],
x
dy [[S, S
y
], M
a
]
)
.
The first term on the right-hand side gives −S
x
. Introduce now the recursion
operator Λ
′
:
Λ
′
· =
i
2
∇
x
−
1
16
(
[S, S
x
],
x
dy [[S, S
y
], ·]
)
.
We have (Λ
′
− k)M
a
= −(i/4)[S, S
x
] a nd therefore
M
a
= −
i
4
(Λ
′
− k)
−1
[S, S
x
].
As a result, equation (10.39) yields (n ≥ 1)
S
t
=2α
n
S
¯
∂(k
n−1
M
a
) =
i
2
α
n
S
¯
∂
k
n−1
∞
m=1
k
−m
Λ
′m−1
[S, S
x
]
=
i
2
α
n
πSδ(k)
∞
m=1
δ
mn
Λ
′m−1
[S, S
x
]=−
i
2
α
n
SΛ
′n−1
[S, S
x
],
because
¯
∂(k
−n
)=πδ(k)δ
n1
. Hence, the Heisenberg spin chain hierarchy with
SDR is given by
S
t
+
i
2
α
n
SΛ
′n−1
[S, S
x
]=−2S
1
k
ω
s
(k)M
a
, (10.42)
∇
x
M
d
=
1
4
[[S, S
x
], M
a
] , ∇
x
M
a
+2ikSM
a
=
1
4
[S, S
x
], M
d
.
The Heisenber g spin chain equation with SDR corresponds to n =2and
α
2
= −2i.
Let us compare the recursion operators for the NLS and Heisenberg equa-
tions:
(Λ − k)M
a
= −iQ and (Λ
′
− k)M
a
= −
i
4
[S, S
x
].
It is trivial to show that the right-hand sides are interconnected by the gauge
transformation, (1/4)[S, S
x
]=g
−1
Qg. Therefore, the same connection should
exist for the recursion operators, Λ
′
= g
−1
Λg. We omit rather techni cal cal-
culation that proves this fact.
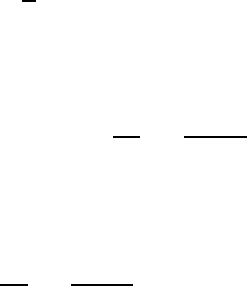
10.2 Nonlinear evolutions with singular dispersion relation 331
10.2 Nonlinear evolutions with singular dispersion
relation for quadratic bundle
In this section we extend the approach developed in Sect. 10.1 to the case of
the spectral transform matrix whose x-evolution is dictated by the quadratic-
in-k equation [126]. We saw in the previous section that the linear-in-k equa-
tion R
x
=ik[R, σ
3
] leads to the ZS spectral problem. Hence, we can expect
that the present case of k
2
will lead us to equations intimately related to
the modified NLS equation which was analyzed in Cha p. 8. We know from
this analysis that the mo dified NLS equation is noncanonical (in the sense of
the RH normalization condition) in the class of equations integrable by the
quadratic bundle. It will be seen th at the same noncanonicity occurs for the
¯
∂ problem.
10.2.1
¯
∂ Problem and recursion operator
As in Sect. 10.1, we b egin with the
¯
∂ problem
¯
∂ψ(k)=ψ(k)R(k),ψ(k)=
11+O(1/k),k→∞. (10.43)
Consider the spectral transform as an off-diagonal matrix even in k, R(−k)
= R(k). This condition resembles the parity property of the Jost solutions.
The spatial and temporal dependences of R(k)aregivenby
R
x
=
i
α
(k
2
+ β)[R, σ
3
],R
t
=[R, Ω], (10.44)
where α and β are real parameters, and the dispersion relation Ω = Ω
p
+ Ω
s
includes both the polynomial Ω
p
and singular Ω
s
parts,
Ω
p
= ω
p
σ
3
=
J
j=0
γ
2j
k
2j
σ
3
,Ω
s
=
1
2πi
dℓ ∧ d
¯
ℓ
ℓ
2
− k
2
ℓ
2
ω(ℓ
2
)σ
3
,
¯
∂Ω
s
= ω
s
(k
2
).
(10.45)
As before, the solution of the
¯
∂ problem (10.43) with the canonical normal-
ization is given by the Cauchy–Green integral formula
ψ(k)=
11+
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
ψ(ℓ)R(ℓ) ≡ 11+ψRC
k
, (10.46)
or
ψ(k)=11 · (11 − RC
k
)
−1
(10.47)
with the diagonal and off-diagonal parts of ψ obeying the parity properties
ψ
d
(−k)=ψ
d
(k),ψ
a
(−k)=−ψ
a
(k). (10.48)
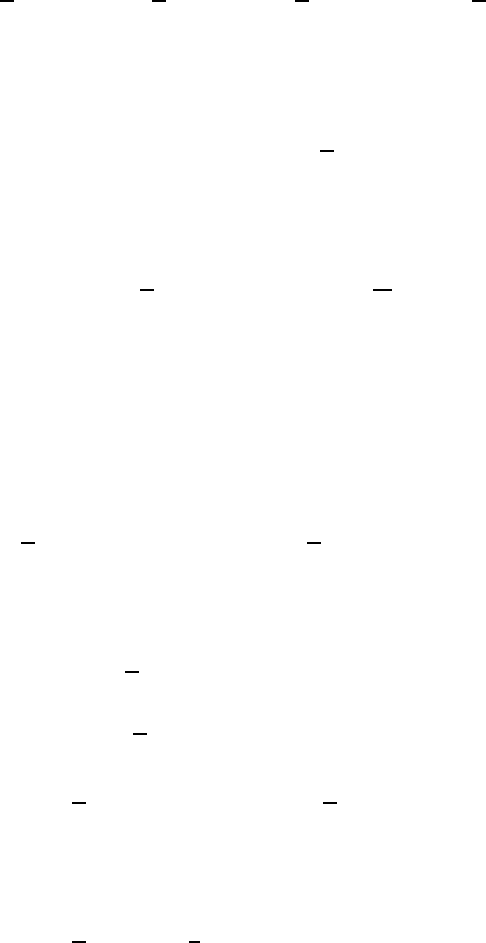
332 10 Generating solutions via
¯
∂ problem
In view of (10.44), differentiation of (10.47) in x gives
ψ
x
= −
i
α
(k
2
+β)[σ
3
,ψ]−
i
α
k[σ
3
, ψR]ψ−
i
α
[σ
3
, ψR]ψRψ−
i
α
[σ
3
, kψR]ψ.
(10.49)
In virtue of the parity property, ψR is an off-diagonal matrix, while kψR
is the diagonal one leading to [σ
3
, kψR] = 0. Define a p otential Q:
Q(x, t)=
0 q
−¯q 0
= −
i
α
[σ
3
, ψR]. (10.50)
Then (10.49) gives the linear spectral problem of the Wadati–Konno–Ichikawa
(WKI) type:
ψ
x
= −
i
α
(k
2
+ β)[σ
3
,ψ]+kQψ −
iα
2
Q
2
σ
3
ψ. (10.51)
Now we obtain a hierarchy of evolutions Q
t
. From (10.50) we get
Q
t
= −iα
−1
[σ
3
, ψR
t
]. The right-hand side is transformed as
(ψR)
t
=
¯
∂ψ
t
=
¯
∂
11 · (11 − RC
k
)
−1
t
=
¯
∂
ψR
t
C
k
(11 − RC
k
)
−1
=
¯
∂
ψR
t
(11 − C
k
R)
−1
C
k
= ψR
t
(11 − C
k
R)
−1
.
Continuing, we get
Q
t
= −
i
α
[σ
3
, ψR
t
(11 − C
k
R)
−1
, 11]=−
i
α
[σ
3
, ψR
t
, 11 · (11+R
T
C
k
)
−1
].
Here we take into account once again that 11 · (11+R
T
C
k
)
−1
=(ψ
−1
)
T
(k).
Consequently, we arrive at
Q
t
= −
i
α
σ
3
, ψRΩψ
−1
, 11−ψΩ, (ψ
−1
)
T
R
T
= −
i
α
σ
3
,
¯
∂(ψΩ
p
ψ
−1
)−(
¯
∂Ω
s
)ψ
−1
= −
i
α
⎡
⎣
σ
3
,
n
j=0
γ
2j
¯
∂(k
2j
M)
⎤
⎦
+
i
α
σ
3
, kω
s
(k
2
)M
,
where M(k)=ψ(k)σ
3
ψ
−1
(k). This function satisfies the associated spectral
equation
M
x
+
i
α
k
2
+ β +
1
2
α
2
Q
2
[σ
3
,M] − k[Q, M ] = 0 (10.52)
10.2 Nonlinear evolutions with singular dispersion relation 333
and can be expanded in the asymptotic ser ies
M = σ
3
+
∞
ℓ=1
M
(ℓ)
k
ℓ
,
with M
(2ℓ+1)
being off-diagonal and M
(2ℓ)
being diagonal matrices. They
satisfy the following equations:
M
(2ℓ)
x
=[Q, M
(2ℓ+1)
],
M
(2ℓ−1)
x
= −
i
α
[σ
3
,M
(2ℓ+1)
] −
i
α
β +
1
2
α
2
Q
2
[σ
3
,M
(2ℓ−1)
]+[Q, M
(2ℓ)
].
Hence,
M
(2ℓ)
= σ
3
δ
ℓ0
+
x
dx[Q, M
(2ℓ+1)
]
and
M
(2ℓ+1)
=(11+L)
i
2
ασ
3
∂
x
− β −
1
2
α
2
Q
2
M
(2ℓ−1)
,ℓ≥ 1,M
(1)
=iαQ.
Here
L· = −
iα
2
σ
3
(
Q,
x
dx[Q, ·]
)
.
Now we define the recursion operator
Λ =(11+L)
i
2
ασ
3
∂
x
− β −
1
2
α
2
Q
2
.
Then it follows that M
(2ℓ+1)
=iαΛ
ℓ
Q and M
a
= −iαk(Λ − k
2
)
−1
Q. Hence,
the hierarchy of nonlinear evolution equations with SDR associated with the
quadratic equation (10.44) is represented by
Q
t
= −2σ
3
n
j=0
γ
2j
Λ
j
Q +
2i
α
σ
3
kω(k
2
)M
a
(k)
together with the spectral equation (10.52).
Putting n =2,γ
0
= β
2
γ
4
, γ
2
=2βγ
4
,andγ
4
=2i/α
2
, we obtain the non-
linear equation corresponding to the WKI spectral problem with the canonical
normalization and with SDR:
iQ
t
+ σ
3
Q
xx
+iαQQ
x
Q +2βσ
3
Q
3
+
1
2
α
2
σ
3
Q
5
= −
2
α
kω(k
2
)M
a
. (10.53)
In order to find the soliton solution to equations (10.53) and (10.52), we
take the sp ectral transform R in the form
R(k)=2πiE
−1
0 c [δ(k − k
1
)+δ(k + k
1
)]
¯c
δ(k −
¯
k
1
)+δ(k +
¯
k
1
)
0
E,
(10.54)
334 10 Generating solutions via
¯
∂ problem
where E =exp
(i/α)(k
2
+ β)σ
3
x
and c = c(t). This choice of R is compatible
with the parity property. Substituting (10.54) into (10.46), we find explicitly
the matrix ψ:
ψ =
⎛
⎜
⎜
⎝
k
2
− k
2
1
k
2
−
¯
k
2
1
+
k
2
1
−
¯
k
2
1
k
2
−
¯
k
2
1
∆
−1
4ick
k
2
− k
2
1
∆
−1
exp
−
2i
α
(k
2
1
+ β)x
4i¯ck
k
2
− k
2
1
¯
∆
−1
exp
2i
α
(
¯
k
2
1
+ β)x
k
2
−
¯
k
2
1
k
2
− k
2
1
+
¯
k
2
1
− k
2
1
k
2
− k
2
1
¯
∆
−1
⎞
⎟
⎟
⎠
,
(10.55)
with
∆(x, t)=1+
|c|
2
¯
k
2
1
ξ
2
η
2
e
(8/α)ξηx
,k
1
= ξ +iη.
The time dependence c(t) is found from the second equation in (10.44) and
gives c(t)=c
0
exp
&
4[ν
′
p
+ ν
′
s
− i(ν
′′
p
+ ν
′′
s
)]t
'
, c
0
=const.Here
ν
′
p
=
4ξη
α
2
(ξ
2
− η
2
+ β),ν
′′
p
=
1
α
2
(ξ
2
− η
2
+ β)
2
− 4ξ
2
η
2
,
1
4π
dℓ ∧ d
¯
ℓ
ℓ
2
− k
2
1
ℓ
2
ω
s
(ℓ
2
)=ν
′
s
− iν
′′
s
.
Inserting ψ (10.55) and R (10.54) into (10.50), we immediately obtain the
soliton solution (k
1
= |k
1
|e
iμ
, |c
0
||k
1
| = ξη e
ρ
, ξη > 0)
q
s
(x, t)=−
4ξη
α|k
1
|
exp
(
−i
αν
′
p
2ξη
x +4(ν
′′
p
+ ν
′′
s
)t + μ
)
(10.56)
×sech
(
4ξη
α
x + α
ν
′
p
+ ν
′
s
ξη
t
+ ρ − iμ
)
.
Equation (10.53) b eing canonical within the class of equations integrable
via the quadratic b un d le has no physical applications. We will show next
that the physically important modified NLS equation follows from (10.53) by
means of a gauge transformation. In fact, this transformation is reduced to a
change of the normalization of the
¯
∂ problem.
10.2.2 Gauge transformation
To obtain the integrable modified NLS equation with a source, we per-
form a gauge transformation ψ = gψ
′
with a function g(x, t)=ψ(k =0);
hence, we arrive at the
¯
∂ problem with the normalization g
−1
(x, t),
ψ
′
= g
−1
(x, t)+O(1/k), and with the same matrix R. It is not difficult
10.3 Nonlinear equations with singular dispersion relation: 2+1 dimensions 335
toseethatatthesametimeΛ
′
= g
−1
Λg and the system of equations (10.52)
and (10.53) transforms to
iQ
′
t
+ σ
3
Q
′
xx
− iα(Q
′3
)
x
+2βσ
3
Q
′3
= −
7
ω
s
(k
2
)
k
α
[σ
3
,M
′a
(k)] + i[Q
′
,M
′d
(k)]
8
, (10.57)
M
′
x
+
(
i
α
(k
2
+ β)σ
3
− kQ
′
,M
′
)
=0.
Here Q
′
= g
−1
Qg and M
′
= g
−1
Mg.Letω
s
=iaπδ(Im k
1
)δ
(Re k
1
)
2
− γ
2
,
a, γ ∈ Re, and
Q
′
=
0 E
−
¯
E 0
,M
′
(γ)=
−np
¯pn
(γ).
Then we obtain the fo l lowing physically interesting system (the modified NLS
equation with SDR),
iE
t
+ E
xx
+iα(|E|
2
E)
x
− 2β|E|
2
E =
ia
α
p −
a
γ
En, (10.58)
p
x
+
2i
α
(γ
2
+ β)p =2γEn, n
x
= −γ(E¯p +
¯
Ep),
which describes a propagation of a subpicosecond optical pulse with the com-
plex envelope E in a no nlinear fiber containing resonant two-level impurities;
p and n are polarization and population difference,s respectively (a, α, β,and
γ are real parameters). The soliton solution to (10.58) follows immediately
from (10.56) as E
s
=(g
22
/g
11
)q
s
. Hence, we get
E
s
(x, t)=
∆(x, t)
¯
∆(x, t)
2
q
s
(x, t).
The N -soliton solution can be found in the same way with the use of an
evident generalization of the spectral transform matrix R. Equations (10.58)
were derived from physical arguments in [462].
10.3 Nonlinear equations with singular dispersion
relation: 2+1 dimensions
In the previous sections we elaborated a method to constru ct nonlinear SDR
equations in 1+1 dimensions. Now we demonstrate how to generalize this ap-
proach to 2+1 dimensions. As shown by Boiti et al. [61], the SDR equations
in 2+1 dimensions possess a number of peculiarities, the main one being the
absence of an explicit expression for the second Lax operat or T
2
= ∂
t
− V .
336 10 Generating solutions via
¯
∂ problem
These authors proposed a (2+1)-dimensional generalization of the Maxwell–
Bloch eq uations in the for m of a rather complicated system of four equations.
The approach of [61] was essentially based on the function V given implic-
itly. On the other hand, we know that the
¯
∂ formalism does not rely on the
Lax representation. Therefore, it is seems reasonabl e to use the
¯
∂ method
to derive the above class of equations, without making direct use of the
function V .
This program realized below relies on the bilocal approach initiated by
Konopelchenko and Dubrovsky [243] and elaborated to a full extent by Fokas
and Santini [161, 384]. It is precisely the bilocal formalism that allows us to
generate in a natural manner (2+1)-dimensional counterparts of many struc-
tures which successfully work in 1+1 dimensions.
10.3.1 Nonlocal
¯
∂ problem
Our starting point is the nonlocal
¯
∂ problem
¯
∂ψ(k)=
dℓ ∧d
¯
ℓψ(ℓ)R(k, ℓ),k,ℓ∈ C,ψ(k)=
11+O(1/k),k→∞,
(10.59)
where R(k, ℓ) is a distribution in C
2
. We denote the integral in (10.59) as
ψ(k)R
k
F ,whereF is an integral operator acting on the left in accordance
with (10.59); hence,
¯
∂ψ(k)=ψ(k)R
k
F. (10.60)
A solution of the
¯
∂ problem is given, as usual, by the Cauchy–Green integral:
ψ(k)=11+
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
dm ∧ d¯mψ(m)R(ℓ, m)
= 11+
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
(ψ(ℓ)R
ℓ
F )=11+ψ(k)R
k
FC
k
. (10.61)
Therefore, a solution of the
¯
∂ problem is compactly written as
ψ(k)=11 · (11 − R
k
FC
k
)
−1
. (10.62)
The pairing is defined as for 1+1 dimensions (10.4), except for the property
ψR
k
F, φ = ψ, φ
ˆ
R
k
F ,where
ˆ
R(k, ℓ)=R
T
(ℓ, k).
Assume a linear parametric dependence of R(k, ℓ) on spatial variables of
the form
∂
x
R(k, ℓ)=iℓσ
3
R(k, ℓ)−ikR(k,ℓ)σ
3
,∂
y
R(k, ℓ)=i(k−ℓ)R(k, ℓ). (10.63)
10.3 Nonlinear equations with singular dispersion relation: 2+1 dimensions 337
Now we show that this choice of the dependence of R(k, ℓ) on spatial variables
leads to the ZS spectral problem on the plane. Differentiating (10.62) in x,we
obtain ∂
x
ψ = ψ(∂
x
R
k
)FC
k
(11−R
k
FC
k
)
−1
. By means of the definitions of the
integral operators F and C
k
and (10.63) we perform the following calculation:
ψ(∂
x
R
k
)FC
k
=
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
dm ∧ d¯mψ(m)∂
x
R(ℓ, m)
=
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
dm ∧ d¯mψ(m)[σ
3
R(ℓ, m) − R(ℓ, m)σ
3
]
=
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
(iℓψσ
3
R
ℓ
F ) −
1
2πi
dℓ ∧ d
¯
ℓ i
1+
k
ℓ − k
(ψR
ℓ
F )σ
3
.
Since we have from (10.61) ψR
k
FC
k
= ψ − 11, then (10.63) and the evident
relation R
k
FC
k
(11 − R
k
FC
k
)
−1
=(11 − R
k
FC
k
)
−1
− 11 yield
∂
x
ψ = −ikψσ
3
− iψR
k
F σ
3
ψ +ikσ
3
(11 − R
k
FC
k
)
−1
. (10.64)
Similarly,
∂
y
ψ =ikψ +iψR
k
F ψ − ik(11 − R
k
FC
k
)
−1
. (10.65)
Adding (10.64) and (10.65) yields
∂
x
ψ + σ
3
∂
y
ψ − ik[σ
3
,ψ] − i[σ
3
, ψR
k
F ]ψ =0. (10.66)
Hence, if we identify
−i[σ
3
, ψR
k
F ]=Q(x, y) (10.67)
with the p otential, then (10.66) gives the ZS spectral problem on the plane:
(∂
x
+ σ
3
∂
y
+ Q)ψ − ik[σ
3
,ψ]=0. (10.68)
Note that our formalism works much easier in 2+1 dimension than in 1+1
dimensions. Indeed, we do not need to transform terms like k(
11 −R
k
FC
k
)
−1
in (10.64) and (10.65) because they cancel each other in the combination
(∂
x
+ σ
3
∂
y
)ψ.
ThetimedependenceofR(k,ℓ) is g iven, as usual, by a linear equation
∂
t
R(k, ℓ)=R(k, ℓ)Ω(k) − Ω(ℓ)R(k, ℓ). (10.69)
Here Ω(k) is a matrix-valued dispersion relation. It consists of a holomor-
phic (polynomial) part Ω
p
(k) a nd a nonanalytic (singular) part Ω
s
(k). It is
instructive to derive the evolution linear problem ∂
t
ψ = Vψ+ ψΩ. It follows
from (10.60) and (10.69) that
∂
t
ψ = ψ(∂
t
R)FC
k
(11−R
k
FC
k
)
−1
=(ψR
k
FΩC
k
−ψΩR
k
FC
k
)(11−R
k
FC
k
)
−1
=(ψR
k
FΩC
k
− ψΩ)(11 − R
k
FC
k
)
−1
+ ψΩ, (10.70)
338 10 Generating solutions via
¯
∂ problem
which gives
Vψ=(ψR
k
FΩC
k
− ψΩ)(11 − R
k
FC
k
)
−1
.
In order to reveal peculiarities of the Lax operator V , it is sufficient to restrict
ourselves to consideration of the singular part of the disp ersion relation. Let
Ω
s
(k)=
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
ω(ℓ)σ
3
,
¯
∂Ω(k)=ω(k)σ
3
.
The calculation yields
V (k)ψ(k)(11 −R
k
FC
k
)=
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
[ψ(ℓ)R
ℓ
F ]Ω(ℓ) − ψΩ
=
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
dm ∧ d¯mψ(m)R(ℓ, m)
1
2πi
ds ∧ d¯s
s − ℓ
ω(s)σ
3
− ψΩ.
The denominator is written as
1
(ℓ − k)(s − ℓ)
=
1
s − k
1
ℓ − k
−
1
ℓ − s
.
Then we have
V (k)ψ(k)(
11 − R
k
FC
k
)
=
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − k
dm ∧ d¯mψ(m)R(ℓ, m)
1
2πi
ds ∧ d¯s
s − k
ω(s)σ
3
−
1
2πi
ds ∧ d¯s
s − k
ω(s)
1
2πi
dℓ ∧ d
¯
ℓ
ℓ − s
dm ∧ d¯mψ(m)R(ℓ, m)σ
3
− ψΩ
= ψR
k
FC
k
Ω(k)−
1
2πi
ds ∧ d¯s
s − k
ω(s)(ψR
s
FC
s
)σ
3
−ψΩ = −ω(k)ψ(k)σ
3
C
k
,
wherewehaveusedψR
s
FC
s
= ψ(s) − 11. Hence,
V (k)ψ(k)=−ω(k)σ
3
C
k
(11 − R
k
FC
k
)
−1
.
Multiplying this relation by (
11 − R
k
FC
k
) and applying the
¯
∂ operator, we
obtain
(
¯
∂V )ψ + V (ψR
k
F ) − VψR
k
F = −ψ
¯
∂Ω
s
,
which gives the integral equation for the Lax function V [61]:
¯
∂V (k)=−ψω
s
(k)ψ
−1
+
dℓ∧d
¯
ℓ [V (ℓ) − V (k)] ψ(ℓ)R(k, ℓ)ψ
−1
(k). (10.71)
Hence, the function V is known only within a solu t ion of the integral equation.
Note that (10.71) includes the inverse function ψ
−1
. However, in 2+1
dimensions (in contrast to 1+1 dimensions), there does not exist a simple
equation [like (10.68)] for ψ
−1
. Hence, a problem arises of finding a natural
(2+1)-dimensional counterpart of the inverse function well defined in 1+1 di-
mensions. We will see next that such a function d oes exist and, moreover, that
our formalism unambiguously suggests a true choice of this function.
