Doktorov E.V., Leble S.B. A Dressing Method in Mathematical Physics
Подождите немного. Документ загружается.


9.3 Davey–Stewartson I equation 309
Now we introduce a spectral parameter k by means of the transformation
ψ(ξ, η)=M(ξ,η, k)E
k
(ξ,η),E
k
(ξ,η)=diag
e
ikη
, e
−ikξ
.
In terms of the matrix M, the spectral equation (9.139) takes the form
DM −
i
2
k[σ
3
,M]+
1
2
QM =0,D= diag(∂
ξ
,∂
η
). (9.146)
We will seek solutions M (ξ, η, k) of the spectral equation that are bounded in
the (ξ, η)-plane for any k and al l ow the asy mpt otic expansion
M(ξ, η, k)=11+k
−1
M
(1)
(ξ,η)+O(k
−2
).
Substituting this expansion into (9.146), we can reconstruct the potential:
Q(ξ,η)=i[σ
3
,M
(1)
(ξ,η)], or q(ξ, η)=2iM
(1)
12
(ξ,η). (9.147)
As before, we will work with solutions of the spectral problem which are
written in the integral form. It can be shown that there exist eigenfunctions
with definite analytic properties in the k-plane. Namely, the matrix functions
M
±
(ξ,η, k) determined by the Green functions G
±
,
M
±
(ξ,η, k)=11 −
G
±
(·,k)
1
2
Q(·)M
±
(·,k)
(ξ,η), (9.148)
where
G
+
(·,k)Φ(·,k)
(ξ,η) (9.149)
=
⎛
⎜
⎜
⎜
⎝
ξ
−∞
dξ
′
Φ
11
(ξ
′
,η,k)
ξ
−∞
dξ
′
Φ
12
(ξ
′
,η,k)e
ik(ξ−ξ
′
)
−
∞
η
dη
′
Φ
21
(ξ,η
′
,k)e
−ik(η−η
′
)
η
−∞
dη
′
Φ
22
(ξ,η
′
,k)
⎞
⎟
⎟
⎟
⎠
and
G
−
(·,k)Φ(·,k)
(ξ,η) (9.150)
=
⎛
⎜
⎜
⎜
⎝
ξ
−∞
dξ
′
Φ
11
(ξ
′
,η,k) −
∞
ξ
dξ
′
Φ
12
(ξ
′
,η,k)e
ik(ξ−ξ
′
)
η
−∞
dη
′
Φ
21
(ξ,η
′
,k)e
−ik(η−η
′
)
η
−∞
dη
′
Φ
22
(ξ,η
′
,k)
⎞
⎟
⎟
⎟
⎠
are analytic in the upper and lower half planes of the k-plane, respectively.

310 9 Dressing via nonlocal Riemann–Hilbert problem
9.3.2 Spectral data and RH problem
To determine sp ectral data for the spectral problem (9.146) with the p otential
Q, we should calculate a jump ∆ = M
+
−M
−
of eigenfunctions a cross the real
axis Imk = 0. After straightforward calculation making use of (9.148)–(9.150)
we obtain
∆(ξ,η, k)=Γ (ξ, η, k) −
G(·,k)
1
2
Q(·)∆(·,k)
(ξ,η), Imk =0, (9.151)
where
Γ =
1
2
0 −
∞
−∞
dξ
′
(qM
−
22
)(ξ
′
,η,k)e
ik(ξ−ξ
′
)
ǫ
∞
−∞
dη
′
(¯qM
+
11
)(ξ,η
′
,k)e
−ik(η−η
′
)
0
and the Green function
G is defined as
G(·,k)Φ(·,k)
(ξ,η)
=
⎛
⎜
⎜
⎝
ξ
−∞
dξ
′
Φ
11
(ξ
′
,η,k)
ξ
−∞
dξ
′
Φ
12
(ξ
′
,η,k)e
ik(ξ−ξ
′
)
η
−∞
dη
′
Φ
21
(ξ,η
′
,k)e
−ik(η−η
′
)
η
−∞
dη
′
Φ
22
(ξ,η
′
,k)
⎞
⎟
⎟
⎠
.
Hereafter we will not specify the integration limits in the case of integration
along the whole line.
Letusseekthejumpintheform
∆(ξ,η, k)=
dℓM
−
(ξ,η, ℓ)E
ℓ
(ξ,η)f(k, ℓ)E
−1
k
(ξ,η) (9.152)
with a 2 × 2 matrix function f (k, l) that determines the spectral data. Sub-
stituting (9.152) into (9.151), we have
∆(ξ,η, k)=Γ (ξ, η,ℓ)−
dℓ
G(·,ℓ)
1
2
Q(·)M
−
(·,ℓ)E
ℓ
(·)f(k, ℓ)E
−1
k
(·)
(ξ,η).
(9.153)
Comparing (9.153) and (9.151), we obtain after simple calculation
dℓE
ℓ
(ξ,η)f(k, ℓ)=−
1
2
dℓ
0
dξ
′
(qM
−
22
)(ξ
′
,η,k)e
−iℓξ
′
00
f(k, ℓ)
+
1
2
0 −
dξ
′
(qM
−
22
)(ξ
′
,η,k)
ǫ
dη
′
(¯qM
+
11
)(ξ,η
′
,k)e
ikη
′
0
. (9.154)
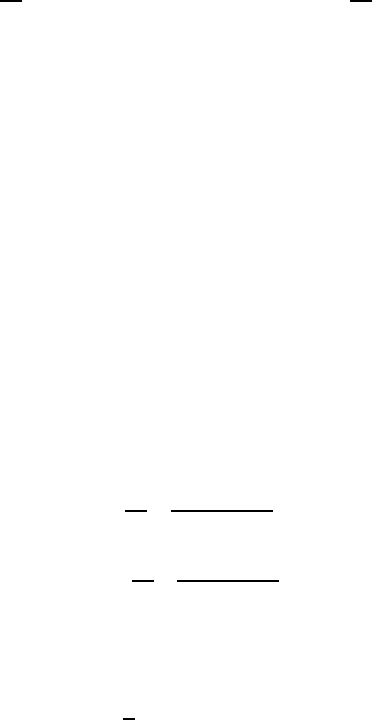
Let us act on (9.154) with the matrix op erator
1
2π
diag
dη
′
e
−ipη
′
,
dξ
′
e
ipξ
′
.
9.3 Davey–Stewartson I equation 311
This results in
f(k, ℓ)=
0 −S(k, ℓ)
T (k, ℓ)0
−
dp
0 S(p, ℓ)
00
f(k, ℓ), (9.155)
where the spectral functions are defined as
S(k, ℓ)=
1
4π
dξdηqM
−
22
e
−ikξ−iℓη
,T(k, ℓ)=
ǫ
4π
dξdη ¯qM
+
11
e
ikη+ilξ
(9.156)
and T (k, ℓ)=ǫ
¯
S(ℓ, k) [162]. It immediately follows from (9.155) that f
22
=0;
hence,
f(k, ℓ)=
−
dpT (k, p)S(p, ℓ) −S(k, ℓ)
T (k, ℓ)0
. (9.157)
Inserting (9.157) into (9.152), we find that the second column of the jump
satisfies the equation
M
+
12
M
+
22
(k) −
M
−
12
M
−
22
(k)=−
dℓS(k, ℓ)e
ikξ+iℓη
M
−
11
M
−
21
(ℓ). (9.158)
Similarly, for the first column we have
M
+
11
M
+
21
(k) −
M
−
11
M
−
21
(k)=−
dℓT (k, ℓ)e
−ikη−iℓξ
M
+
12
M
+
22
(ℓ). (9.159)
Equations (9.158) and (9.159) determine the nonlocal RH problem. In accor-
dance with the Cauchy–Green formula (1.98) the solution of the RH problem
is given by the integral equations of the form
M
±
11
M
±
21
(k)=
1
0
+
1
2π
dp
p − (k ± i0)
dℓT (p, ℓ)e
−ipη−iℓξ
M
+
12
M
+
22
(ℓ),
M
±
12
M
±
22
(k)=
0
1
−
1
2π
dp
p − (k ± i0)
dℓS(p, ℓ)e
ipξ+iℓη
M
−
11
M
−
21
(ℓ).
(9.160)
Hence, the reconstruction of the potential q(ξ,η) from the solution of the RH
problem is given with account for (9.147) by the formula
q(ξ, η)=
1
π
dkdℓS(k, ℓ)e
iℓη+ikξ
M
−
11
(ξ,η, ℓ). (9.161)
9.3.3 Time evolution of spectral data and boundaries
To find solutions of the DS I equation using the IST method, we should de-
termine the time evolution of the spectral function S(k, ℓ). As usual, the
second Lax equation is exploited for this sake. In the case of the initial
bo undary value problem we, however, cannot naively invoke (9.140) in its

312 9 Dressing via nonlocal Riemann–Hilbert problem
present fo rm because of the nontrivial boundary conditions. In order to rec-
oncile boundary conditions with the evolutionary Lax equation, note that
we can add a term
dℓψ(k, ℓ)γ(k − ℓ) with some matrix function γ to the
right-hand side of (9.140), without breaking the compatibility condition. Now
we invoke such a freedom to incorporate boundary values u
1
and u
2
into
the Lax pair. Assume that the right-hand side of (9.140) contains an ad-
ditional term W (ξ, η, k) and consider a matrix function ψ
−
related to M
−
by ψ
−
(ξ,η, k)=M
−
(ξ,η, k)E
k
(ξ,η). From the integral formulas (9.148) and
(9.150) for M
−
it immediately follows that matrix elements of ψ
−
obey simple
asymptotics in some directions ξ →±∞and/or η →±∞.Asanexample,
ψ
−
12
→ 0atξ → +∞ and ψ
−
22
→ e
−ikξ
at η →−∞; hence, we can write
ψ
−
12t
= −
∞
ξ
dξ
′
ψ
−
12t
ξ
′
and ψ
−
22t
=
η
−∞
dη
′
ψ
−
22t
η
′
.
Inserting into the integrands evolution equations for the entries of ψ
−
which
follow from
iψ
−
t
+ σ
3
ψ
−
yy
+ Qψ
−
y
+ Aψ
−
+ W =0,
yields, in particular,
W
12
W
22
= −k
2
ψ
−
12
ψ
−
22
(k)+
dℓ
ψ
−
12
ψ
−
22
(ℓ)γ
1
(k − ℓ).
Here γ
1
(k − ℓ) is related to the boundary value u
1
(ξ,t)bymeansof
u
1
(ξ,t)=
dℓγ
1
(k − ℓ)e
i(k−ℓ)ξ
. (9.162)
Similarly,
W
12
W
22
(k)=k
2
ψ
−
11
ψ
−
21
(k) −
dℓ
ψ
−
11
ψ
−
21
(ℓ)γ
2
(k − ℓ)
and
u
2
(η, t)=
dℓγ
2
(k − ℓ)e
−i(k−ℓ)η
.
As a result, the improved evolutionary Lax equation has the form
iψ
−
t
+ σ
3
ψ
−
yy
+ Qψ
−
y
+ Aψ
−
+ k
2
ψ
−
σ
3
−
dℓψ
−
(ℓ)γ(k − ℓ)σ
3
=0, (9.163)
γ(k − ℓ)=diag[γ
2
(k − ℓ),γ
1
(k − ℓ)] .
Subsequent actions are rather standard. In terms of ψ
−
, the spectral func-
tion S(k, ℓ) is written as
S(k, ℓ)=
1
4π
dξdηq(ξ, η)ψ
−
22
(ξ,η, k)e
−iℓη
.

9.3 Davey–Stewartson I equation 313
Hence, it is nat u ral to consider ψ
−
12
for ξ →−∞:
lim
ξ→−∞
ψ
−
12
(ξ,η, k) ≡ χ(k, η)=
1
2
dξdηq(ξ, η)ψ
−
22
(ξ,η, k).
Moreover, it is easy to show that χ(k,η)=
dℓe
iℓη
S(k, ℓ).
We consider (9.163) for ψ
−
12
at ξ →−∞. In this limit
iχ
t
+ χ
ηη
+ u
2
χ − k
2
χ +
dℓχ(ℓ, η)γ
1
(k − ℓ)=0. (9.164)
Let us analyze the term with the integral. Expressing γ
1
(k−ℓ) from (9.162) as
γ
1
(k − ℓ)=
1
2π
dξ
′
e
−i(k−ℓ)ξ
′
u
1
(ξ
′
,t)
and substituting it in (9.164) yields the purely exponential k-dependence of
the integrand. This means that it is reasonable to multiply this term by
(2π)
−1
dke
ikξ
and integrate in k to have the delta-function δ(ξ − ξ
′
). As
a result, we obtain
1
2π
dke
ikξ
dℓχ(ℓ, η)γ
2
(k − ℓ)=u
1
(ξ,t)
S(ξ,η).
Here
S(ξ,η)=(1/2π)
dℓe
iℓξ
χ(ℓ, η), i.e.,
S(ξ,η) represents in fact the Fourier
transform of the sp ectral function S(k, ℓ):
S(ξ,η)=
1
2π
dξdη e
ikξ+iℓη
S(k, ℓ).
Transforming the rest of the terms in (9.164) after multiplying by the above
integral operator, we obtain the linear evolution equation for
S(ξ,η) [162]:
i
S
t
+
S
ξξ
+
S
ηη
+(u
1
+ u
2
)
S =0. (9.165)
We should solve this equation with the initial value
S(ξ,η,0), which in turn is
determined by the initial value q(ξ,η,0), and the known boundary functions
u
1
and u
2
.
Equation (9.165) allows the separation of variables of the form
S(ξ,η,t)=
X(ξ,t)Y (η,t). This leads to the appearance of the nonstationary linear
Schr¨odinger equations with the boundary functions as potentials:
iX
t
+ X
ξξ
+ u
1
(ξ,t)X =0, iY
t
+ Y
ηη
+ u
2
(η, t)Y =0. (9.166)
In what follows we will be interested in the purely discrete spectra of (9.166)
(the so-called refle ctionless boundaries). It is easy to verify directly that the
orthonormal eigenfunctions X
n
(ξ,t)andY
n
(η, t) of the discrete spectrum of
(9.166) can be written in a closed form as solutions of the algebraic equations

314 9 Dressing via nonlocal Riemann–Hilbert problem
X
n
+
N
1
j=1
m
n
¯m
j
μ
n
+¯μ
j
exp
−(μ
n
+¯μ
j
)ξ +i(μ
2
n
− ¯μ
2
j
)t
X
j
= m
n
e
−μ
n
(ξ−iμ
n
t)
, (9.167)
Y
n
+
N
2
j=1
ℓ
n
¯
ℓ
j
λ
n
+
¯
λ
j
exp
−(λ
n
+
¯
λ
j
)η +i(λ
2
n
−
¯
λ
2
j
)t
Y
j
= ℓ
n
e
−λ
n
(η−iλ
n
t)
, (9.168)
while the potentials are expressed in terms of X
n
and Y
n
as
u
1
(ξ,t)=−2∂
ξ
N
1
i=1
¯m
i
exp [−¯μ
i
(ξ +i¯μ
i
t)] X
i
(ξ,t),
u
2
(η, t)=−2∂
η
N
2
j=1
¯
ℓ
j
exp
−
¯
λ
j
(η +i
¯
λ
j
t)
Y
j
(η, t). (9.169)
Evidently, the solutions of (9.167) and (9.168) take the form
X
n
(ξ,t)=
N
1
i=1
(11+C
ξ
)
−1
nj
m
j
e
−μ
j
(ξ−iμ
j
t)
,
Y
n
(η, t)=
N
2
j=1
(11+C
η
)
−1
nj
ℓ
j
e
−λ
j
(η−iλ
j
t)
, (9.170)
where the Hermitian matrices C
ξ
and C
η
are defined as follows:
(C
ξ
)
ni
=
m
n
¯m
i
μ
n
+¯μ
i
exp
−(μ
n
+¯μ
i
)ξ +i(μ
2
n
− μ
2
i
)t
,
(C
η
)
nj
=
ℓ
n
¯
ℓ
j
λ
n
+
¯
λ
j
exp
−(λ
n
+
¯
λ
j
)η +i(λ
2
n
−
¯
λ
2
j
)t
.
Therefore, the spectral function
S(ξ,η,t) is written as
S(ξ,η,t)=
N
1
i=1
N
2
j=1
ρ
ij
X
i
(ξ,t)Y
j
(η, t) (9.171)
and the complex parameters ρ
ij
are determined from the initial conditions:
ρ
ij
=
dξdη
S(ξ,η,0)
¯
X
i
(ξ,0)
¯
Y
j
(η, 0). (9.172)
Orthonormality of X
n
and Y
n
gives rise to
X
n
¯
X
i
= ∂
ξ
(11+C
ξ
)
−1
ni
,Y
n
¯
Y
j
= ∂
η
(11+C
η
)
−1
nj
. (9.173)
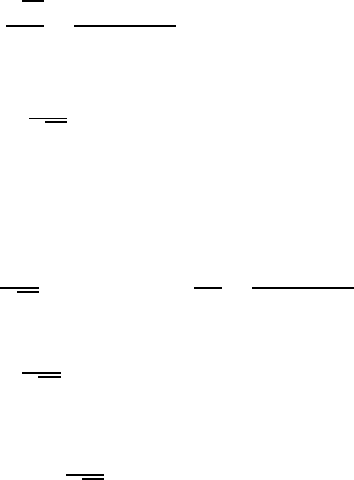
9.3 Davey–Stewartson I equation 315
9.3.4 Reconstruction of potential q(ξ, η, t)
The factorized representation (9.171) of
S(ξ,η,t) corresp onds to the degener-
acy o f the kernel S(k,ℓ) of the integral equations (9.160) that determines the
solution of the nonlocal RH problem. Namely,
S(k, ℓ)=
N
i=1
S
i
(k)
S
i
(ℓ). (9.174)
As a result, the second column
M
+
12
,M
+
22
T
(k) in (9.160) can b e written as
M
+
12
M
+
22
(k)=
0
1
−
√
2π
2π
dp
p − (k +i0)
e
ipξ
N
i=1
S
i
(p)F
i
(ξ,η), (9.175)
where
F
i
(ξ,η)=
1
√
2π
dℓe
iℓη
S
i
(ℓ)
M
−
11
M
−
21
(ℓ).
Let us multiply (9.175) by (2π)
−1/2
dke
−ikξ
¯
S
r
(k) and integrate in k.This
gives
G
r
(ξ,η)=
0
1
¯σ
r
(ξ) (9.176)
−
dk
¯
S
r
(k)e
−ikξ
i
1
√
2π
dℓσ
i
(ℓ)F
i
(ξ,η)
1
2πi
dp
p − (k +i0)
e
i(ξ−ℓ)p
.
Here
G
r
(ξ,η)=
1
√
2π
dke
−ikξ
¯
S
r
(k)
M
+
12
M
+
22
(k)
and σ
i
(ξ) determines the Fourier transform of S
i
(k):
σ
i
(ξ)=
1
√
2π
dke
ikξ
S
i
(k).
The last integral in (9.176) gives 2πiθ(ξ − ℓ)exp[ik(ξ − ℓ)]. Then after simple
manipulations with (9.176) we eventually obtain the algebraic equation
G
r
(ξ,η)+
N
i=1
α
ri
(ξ)F
i
(ξ,η)=
0
1
¯σ
i
(ξ), (9.177)
where
α
ri
=
ξ
−∞
dℓ¯σ
r
(ℓ)σ
i
(ℓ). (9.178)
Performing a similar calculation with the column (M
−
11
,M
−
21
)
T
,weobtain

316 9 Dressing via nonlocal Riemann–Hilbert problem
F
r
(ξ,η)+ǫ
N
j=1
β
rj
G
j
(ξ,η)=
1
0
σ
r
(η). (9.179)
Here
β
rj
(η)=
η
−∞
dℓσ
r
(ℓ)
¯
σ
j
(ℓ) (9.180)
and σ
j
(ℓ) is the Fourier transform of
S
j
:
σ
j
=
1
√
2π
dℓe
iℓη
S
j
(ℓ). (9.181)
Then from (9.177) and (9.179) we find a system of algebraic equations for the
first component f
r
of F
r
:
f
r
− ǫ
N
j=1
⎛
⎝
N
j=1
β
rj
α
ji
⎞
⎠
f
i
= σ
r
. (9.182)
Inserting into (9.161) the factorized form (9.174) of S(k, ℓ), we arrive at the
closed formula for the potential q:
q(ξ, η)=2
N
i=1
σ
i
(ξ)f
i
(ξ,η). (9.183)
Hence, we have two representation of the Fourier transform of S(k, ℓ, t).
One of them is written i n terms of σ
i
and σ
i
, S(ξ, η, t)=
i
σ
i
(ξ,t)σ
i
(η, t),
and the other one is given by (9.171). Therefore, we can take
σ
i
(ξ,t)=X
i
(ξ,t), σ
i
(η, t)=
N
2
j=1
ρ
ij
Y
j
(η, t). (9.184)
Recall that we should calculate f
i
(ξ,η) which enters (9.183). Taking advantage
of the representation (9.184) and the orthonormality property (9.173), we
can calculate integrals in (9.178) and (9.180). Then after rather lengthy but
straightforward calculation we obtain the formula for the po tential q [162]:
q(ξ, η,t)=
N
1
i=1
N
2
j=1
X
i
(ξ,t)Y
j
(η, t)Z
ij
(ξ,η, t), (9.185)
where Z
ij
obeys the matrix algebraic equation Z −ǫHZ = ρ.Herethematrix
H is given by H = ρ(11+C
η
)
−1
¯ρ
†
(11+C
ξ
)
−1T
and the matrix ρ is defined in
(9.172). Solutions of the DS I equation which correspond to the reflectionless
boundaries are called (N
1
,N
2
) dromions [162].
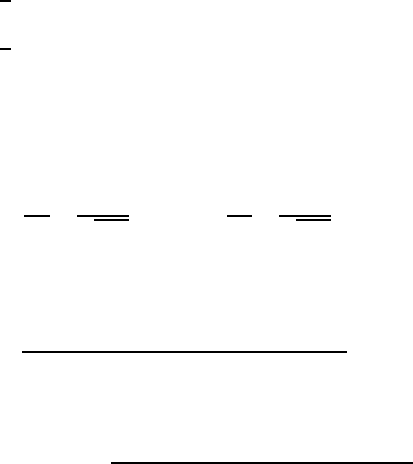
9.3 Davey–Stewartson I equation 317
9.3.5 (1, 1) Dromion solution
It is instructive to derive explicitly the simplest (1, 1) dromion solution. It
corresponds to ǫ = N
1
= N
2
= 1. In what follows we will omit the summation
subscript 1. In this case equations (9.167) and (9.170) give
X(ξ,t)=
1
2
me
−μ
R
ξ
0
e
iμ
I
ξ+i(μ
2
R
−μ
2
I
)t
sechμ
R
(ξ +2μ
i
t − ξ
0
),
Y (η, t)=
1
2
ℓe
−λ
R
η
0
e
iλ
I
η+i(λ
2
R
−λ
2
I
)t
sechλ
R
(η +2λ
I
t − η
0
),
u
1
(ξ,t)=2μ
2
R
sech
2
μ
R
(ξ +2μ
R
t − ξ
0
),
u
2
(η, t)=2λ
2
R
sech
2
λ
R
(η +2λ
I
t − η
0
),
where μ = μ
R
+iμ
I
, λ = λ
R
+iλ
I
, and real parameters ξ
0
and η
0
are defined as
ξ
0
=
1
μ
R
ln
|m|
√
2μ
R
,η
0
=
1
λ
R
ln
|ℓ|
√
2λ
R
.
It is convenient to introduce running coordinates z
ξ
= μ
R
(ξ +2μ
I
t − ξ
0
)and
z
η
= λ
R
(η +2λ
I
t − λ
0
). Then
Z = ρ
exp(−z
ξ
− z
η
)coshz
ξ
cosh z
η
exp(−z
ξ
− z
η
)coshz
ξ
cosh z
η
+ |ρ|
2
/4
and the (1, 1) dromion solution takes the form
q(ξ, η,t)=4ρ(μ
R
λ
R
)
1/2
exp(−iΦ)
4coshz
ξ
cosh z
η
+ |ρ|
2
exp(z
ξ
+ z
η
)
with the phase
Φ = μ
I
ξ + λ
I
η − (μ
2
R
+ λ
2
R
− μ
2
I
− λ
2
I
)t − arg(mℓ).
This solution describes a localized object in the (ξ, η)-plane which decays ex-
ponentially in all directions in the plane and moves with velocity (−2μ
I
, −2λ
I
).
Therefore, the dromion velocity is completely determined by the boun d aries,
while the initial value ρ influences the direction of motio n in the (ξ, η)-plane.
Explicit calculation of the (2, 2) dromion solution is too cumbersome to
be reproduced here. Fokas and Santini [162] performed the analysis of the
asymptotic behavior of the (2, 2) dromion. They showed that this solution
decays asymptotically into four single-hump constituents. Though the total
energy of the (2, 2) dromion is conserved, the constituents can exchange energy
among themselves. Besides, dromions do not in general preserve their form
upon interaction.
