Das Sh.P. Statistical Physics of Liquids at Freezing and Beyond

Подождите немного. Документ загружается.

10.2 First-order transitions 491

have been built on possible scenarios involving ﬁrst-order transitions in the special situation

of there being a large number of available metastable states.

10.2.1 Metastable aperiodic structures

In Chapter 2 we demonstrated the application of classical density-functional theory (DFT)

to studying the ﬁrst-order freezing transition of the liquid into an ordered crystalline state.

DFT methods have also been applied to study the supercooled liquid state below the freez-

ing point T

for systems with aperiodic structures (Singh et al., 1985; Dasgupta, 1992;

Kaur and Das, 2001a). The latter are modelled in terms of localized density proﬁles (over

suitable time scales) around a disordered set of lattice points. The metastable states are

identiﬁed as minima of the free-energy landscape intermediate between a crystal and a

homogeneous liquid state. Computation of the thermodynamic free energy of such sys-

tems requires an explicit functional form for the order parameter, i.e., the inhomogeneous

density function in terms of a suitable set of parameters. The free-energy functional is

minimized with respect to these parameters. As we saw in Chapter 2, a very success-

ful prescription of the density distribution for the crystalline case is obtained from the

superposition of Gaussian density proﬁles centered on a lattice with long-range order,

ρ(r ) =



φ(|r −



|), (10.2.1)

where {



} denotes the underlying lattice and the function φ is taken as the isotropic

Gaussian φ(r ) =(α/π )

3/2

−αr

. In the case of an aperiodic structure {R

} constitutes a

random structure. α is the variational parameter such that its inverse characterizes the

width of the peak and therefore signiﬁes the degree of mass localization in the system.

The homogeneous liquid state in this representation is characterized by Gaussian proﬁles

of very large width corresponding to the limit α →0. Each Gaussian proﬁle provides the

same contribution to the sum on the RHS of eqn. (10.2.1) corresponding to all spatial

positions.

The free energy of the liquid is obtained as a sum of two parts – the ideal-gas term and

the interaction term,

F[ρ]=F

[ρ]+F

[ρ]. (10.2.2)

The ideal-gas term of the free-energy functional (in units of β

−1

) is expressed as a func-

tional of the inhomogeneous density function ρ(r) in the form

[ρ]=



dr ρ(r )

(





ρ(r )

− 1

)

, (10.2.3)



being the thermal de Broglie wavelength. For the highly localized structures which have

been investigated, generally α was chosen to be large (greater than ≈ 50) and eqn. (10.2.3)

492 The thermodynamic transition scenario

was approximated by its asymptotic value for large α (see eqn. (2.2.21) discussed in

Chapter 2),

= f

[ρ]≈−

+ ln





(

)

3/2



. (10.2.4)

The above asymptotic formula for the case of large α is particularly applicable for a highly

localized mass distribution of the crystalline state. For the aperiodic structures, however,

the low-α range where overlapping Gaussian proﬁles from different sites contribute to

is also relevant. In this case F

is evaluated numerically from the integral given in

(10.2.3) as

[ρ]=



dr φ(r)





dR φ(r −R)

(

δ(R) + ρ

(R)

)



− 1

, (10.2.5)

where the random structure on which the density proﬁles are centered is obtained on the

average in terms of the site–site correlation function. A standard procedure generally fol-

lowed here to obtain the latter is to use the g

(R) corresponding to Bernal’s random

structure (Bernal, 1964), which is generated through Bennett’s algorithm (Bennett, 1972).

The site–site correlation reduces to the pair correlation of the Bernal structure at the close-

packed density. In this regard, it should be noted that for a hard-sphere system identiﬁcation

of the most closely packed random structure is somewhat anomalous (Torquato et al.,

2000). In the present context, however, the Bernal structure is simply applied as a tool

to evaluate the free energy for an inhomogeneous density proﬁle centered at the random

set of lattice points. Similar studies of the same free-energy functional when tested with

random structures obtained from computer-simulation studies (Kim and Munakata, 2003)

gave similar results.

The ideal free-energy value evaluated with the expression given in eqn. (10.2.5) is shown

in Fig. 10.1 (inset) for ρ

=1.12. The numerical result approaches the asymptotic (large-α)

result (dashed line) for α>20 within 5% as shown in the ﬁgure. The interaction part F

of the free energy is evaluated using the standard formalism with the expression for the

Ramakrishnan–Yussouff (RY) functional,

F

=−



dr



dr

c(|r

−r

|;ρ

)δρ(r

), (10.2.6)

which gives the difference between the free energies of the solid and liquid phases of

average density ρ

.Hereδρ (r ) is the density ﬂuctuation from the average value ρ

.The

expansion (10.2.6) for the free energy of the liquid works as a better approximation for the

free energy of the solid state in the low-α region of the density function than for the highly

localized region for large α.

The existence of metastable minima corresponding to low and high values of α has been

seen using the above free-energy functional. The results we discuss here are for hard-sphere

systems, and generally the solution of the Percus–Yevick equation with Verlet–Weiss cor-

rection for the direct correlation function, c(r), of the reference liquid state is used in

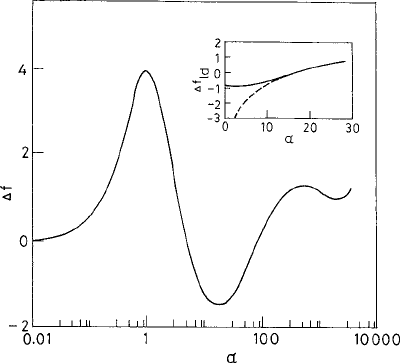
10.2 First-order transitions 493

Fig. 10.1 The difference in free energy per particle denoted as f /ρ between the solid and liq-

uid states vs. α

∗

(ασ

)atn

∗

) =1.12. The inset shows the ideal-gas free-energy term vs.

∗

for n

∗

=1.12. The solid line is obtained from the exact evaluation of formula (10.2.5) and the

dashed line is the approximate formula (10.2.4) for large α. Reproduced from Kaur and Das (2001a).

 American Institute of Physics.

obtaining them. For the hard-sphere system, Singh et al. (1985) obtained metastable min-

ima for the free energy corresponding to very large values of the width parameter α.This

represents a highly localized inhomogeneous density distribution. A qualitatively different

free-energy minimum with the optimum density function characterized by small α values

has been obtained subsequently (Kaur and Das, 2001a). The low-α minimum appears at

α ≈ 18 for ρ

=1.12, while the corresponding large-α minimum occurs for ασ

> 100. A

typical result is displayed in Fig. 10.1. The free energy corresponding to the low-α minima

is generally less than that for the highly localized (large-α) state throughout the density

range above n

=1.15. The low-α minimum becomes more stable than the homoge-

neous liquid state (α → 0) beyond density n

=1.10. The corresponding “hard-sphere

glass” state for high α values becomes more stable w.r.t. the homogeneous liquid state at an

average density n

=1.14. The occurrence of the highly localized structures for large α

is also sensitive to having special tails in the large-r behavior of the input c(r) (Singh et al.,

1985). The existence of the low-α minima, on the other hand, is found to be rather robust

against different input direct correlation functions, and they occur even with the purely

repulsive PY c(r) without any tail. Treatments of the above free-energy functional using

the MWDA described in Chapter 2 also obtain the metastable minima for the less local-

ized metastable structure corresponding to small α values. Evaluation of the free energy of

ﬁnite-size systems with computer models using ﬂuctuating values of the width parameter

α at different sites has also demonstrated (Chaudhary et al., 2005) the existence of similar

metastable states.

494 The thermodynamic transition scenario

The two minima with the random structure for low and high values of α correspond

to very different degrees of localization of the particles. The α corresponding to the min-

imum free-energy value is inversely proportional to the root-mean-square displacement

of the particles from their sites and hence deﬁnes the Lindemann ratio for the state. The

metastable supercooled state seen in computer simulations (Stillinger, 1995; LaViolette,

1985) shows better agreement with the low-α minimum. The Lindemann ratio of super-

cooled liquid seen in simulations is approximately three times that of the crystal at freezing

(Stillinger, 1995). The height of the free-energy barrier between the metastable glassy

structure and the uniform liquid state grows with increasing packing fraction. The extrap-

olated height of the barrier diverges with a power law at the packing fraction value ϕ

≈

0.62 with exponent 1.57 from the simple density-functional calculation (Kaur and Das,

2001a). In the next section our discussion of the mosaic theory implies a similar power-law

divergence of the barrier (to coming out of a frozen amorphous structure) at the thermo-

dynamic phase-transition point. In the case of the hard-sphere system this critical point

is described in terms of the density. In comparison with the replicated-liquid-state the-

ory for the glassy state (described in Section 10.5.1) we obtain the critical Kauzmann

packing fraction η

=0.628 (Parisi and Zamponi, 2010), while an effective potential cal-

culation (see Section 10.3.2) for the self-generated disorder gives η

=0.62 (Cardenas

et al., 1999).

10.2.2 Random ﬁrst-order transition theory

The above studies of the density-functional models demonstrate that metastable free-energy

minima exist in the supercooled region. Indeed, they correspond to a class of intermediate

minima for different amorphous structures distributed on a lattice without any long-range

order. Note that in the above analysis the width parameter α can also be varied (Odagaki

and Yoshimori, 2000; Odagaki et al., 2002; Chaudhary et al., 2005). The metastable mini-

mum occurs at a ﬁnite value of α, signifying a ﬁnite Lindemann ratio. Accordingly, the

system is expected to undergo a ﬁrst-order phase transition. However, such a scenario

would imply a ﬁnite latent heat associated with the glass transition similar to that for

the crystallization transition. For understanding the structural-glass transition without any

latent heat, here we invoke an analogy to certain types of spin-glass models. These systems

undergo a phase transition characterized by a jump in a locally deﬁned order parameter

without any absorption of latent heat. This has been termed a random ﬁrst-order transi-

tion (RFOT). Such systems include Potts spin glasses (Gross et al., 1985), the p-spin glass

(Mézard et al., 1987), and reﬁned p-spin versions (Gardner, 1985) of the random-energy

model (Derrida, 1981). Like the structural glasses, these spin-glass models also exhibit

an entropy crisis, with the corresponding conﬁgurational entropy vanishing at the phase-

transition point. In the case of the structural glass the RFOT is linked to the existence of

a large number of aperiodic structures, in contrast to the unique crystalline state for the

freezing transition. While drawing the parallel between structural and spin glasses it is

worth remembering the obvious differences. The spin-glass models mentioned above are

10.2 First-order transitions 495

characterized by long-range interactions, whereas the structural glasses have short-range

interactions (see the discussion in Section 8.4).

The idea of the RFOT in supercooled liquids is based on the formation of an entropic

droplet (Kirkpatrick et al., 1989) in the deeply supercooled liquid. A primary assumption

in this theory is that the supercooled liquid is close to the metastable equilibrium. Hence

it is applicable to more generic glass-forming systems that are unlike those formed by

rapid quenching from a melt and are in a state far from equilibrium. In the mean-ﬁeld

description the liquid is generally assumed to be globally in a metastable state. How-

ever, in the ﬁnite-dimensional system such a description is valid only over a time scale

beyond that on which the system comes out of the metastable state. The deeply super-

cooled liquid can be in any one of the extensive number of possible metastable states,

each corresponding to an aperiodic structure. The study of the density-functional models

described above has demonstrated the existence of such intermediate states. The possi-

bility of an extensive number of aperiodic states (of the same free energy f ) gives rise

to a ﬁnite conﬁgurational entropy S

( f ) for the supercooled liquid. The RFOT theory is

based on the fact that for systems with ﬁnite-range interactions this entropy will lead to an

instability for the global mean-ﬁeld state, and the system will break up into a mosaic of

structures for the supercooled liquid. As we have seen in the case of classical nucleation

theory (see Chapter 3), there is a free-energy drive towards formation of the condensed

phase, which is more stable than the parent phase. This is unlike the present case of a

mosaic of different aperiodic states for which the free energies are the same. However,

here the drive is entropic. There is a likelihood of formation of the new phase since

there are so many of them available for the system to escape into, hence giving rise to

the ﬁnite S

( f ). This entropy drive is −L

where s

is the conﬁgurational entropy

per unit volume in the supercooled domain of size L. However, there is a cost in form-

ing the new phase that is proportional to σ

, which is like the surface-tension term

in expression (3.1.9) for the free energy of formation of the critical nucleus in classical

nucleation theory. The exponent μ here is kept at a more general level than the typical

surface contribution and σ

denotes the “generalized” surface tension between the two

phases. We expect the value of the exponent μ to depend on the order parameters in terms

of which the phases are described. For a simple Ising-like description μ =d − 1 and the

energy cost is simply proportional to the surface area between the two phases. In gen-

eral, μ ≤d − 1. The total free-energy cost for formation of the bubble of the new phase is

obtained as

F =−Ts

+ σ

. (10.2.7)

The above free-energy cost is optimized to obtain the size of the critical domain or the

correlation length ξ



μσ



1/(d−μ)

(10.2.8)

496 The thermodynamic transition scenario

and the corresponding value of the optimum free energy is obtained as

≡ F

∗

= σ

(1 − κ)ξ

∼

1/(1−κ)

(Ts

)

κ/(1−κ)

, (10.2.9)

where κ = μ/d ≤ 1 − 1/d and is a constant less than 1 for d ≥ 2. E

represents the

energy barrier to the formation of the new phase. The crucial aspect of this result is that the

energy barrier to formation of this new phase grows with the correlation length ξ

whereas

in the Adam–Gibbs theory we have seen in eqn. (10.1.11) that the barrier E

grows as ξ

The deeply supercooled liquid consists of glassy clusters separated by domain walls. As

the temperature of the liquid is lowered their size (∼ξ

) grows. The correlation length ξ

inversely proportional to the conﬁgurational entropy S

. At the Kauzmann temperature T

the correlation length ξ

diverges as S

→ 0. The formation and growth of a nucleus in a

ﬁrst-order phase transition (described in Chapter 3) is qualitatively different from the above

picture. In the earlier situation the nucleus simply consists of one phase, which grows in the

parent phase. In the mosaic picture (Wolynes, 1989) even inside a glassy cluster another

cluster can grow due to the entropic drive making the role of ﬂuctuations very important.

Using the concept of entropic droplets and assuming that there is an interface cost to putting

different metastable states into contact in the mosaic picture, a glassy coherence length has

been obtained (Biroli and Bouchaud, 2004).

The dynamics of the liquid is controlled by activated processes in which there is constant

formation of the amorphous clusters. At T

the barrier to such a transformation is inﬁnite

and hence the relaxation time diverges. The frozen liquid is now caught in a single aperi-

odic structure with ﬁnite shear modulus. In the original proposal of this theory (Kirkpatrick

et al., 1989) scaling and renormalization-group ideas were used to claim that μ =d/2, i.e.,

κ =

. In this case the growth of the barrier E

∼ 1/S

and, assuming the relation (10.1.7),

we obtain that the relaxation time τ(T ) ∼ 1/(T −T

), which is the Vogel–Fulcher expres-

sion. However, using κ as a free parameter does not change the basic contents of the theory.

The entropic-droplet theory was subsequently constructed for a realistic system of a ran-

dom hetero-polymer model by Takada and Wolynes (1997). The density-functional model

for the free energy with respect to the RFOT theory has been studied subsequently by Xia

and Wolynes (2000, 2001) and by Biroli et al. (2008).

The scenario of a thermodynamic phase transition at T

discussed above is linked to the

existence of a static correlation length that diverges at the transition temperature. A quali-

tatively different kind of dynamic length scale related to the dynamics of the supercooled

liquid has been identiﬁed, and we have already discussed this in Section 4.4.2. Indeed, the

relaxation time in the supercooled liquid grows beyond any conceivable experimental time

scale already near the calorimetric glass transition T

, which is higher than T

. Evidence of

a growing static correlation length in the measurable temperature range has been difﬁcult

to ﬁnd, however. As we have already noted, the static structure factor of the liquid in terms

of density correlation functions does not display any such building correlation. In fact, the

characterization of a proper physical deﬁnition of the length scale is not clear in the present

situation.

10.2 First-order transitions 497

In recent times some new insight into this search for possible correlation lengths has

been gained by focusing on correlations of variables other than the simple density ﬂuc-

tuations. Yamamoto and Onuki (1998a,1998b) performed extensive MD simulations of a

binary soft-sphere mixture of N

= N

=5000 particles in two and three dimensions. The

details of this interaction potential between the two species of the mixture are as described

in Section 4.3.1. Bonds between two neighboring particles are deﬁned in this system by

using a length scale corresponding to the sharp ﬁrst peak in the static correlation function.

At a time t, two particles i and j of the liquid belonging to species α and β in a mixture are

at r

(t) and r

(t), respectively. These two particles are considered bonded if the following

constraint is satisﬁed:

(t) =|r

(t) − r

(t)|≤A

αβ

, (10.2.10)

with A

=1.1 (in two dimensions) and 1.5 (in three dimensions). This bond is considered

broken at a later time t +t



if the distance r

(t +t



) becomes larger than A

αβ

.Asasetof

suitable parameters the following choice is made: A

=1.6 (in two dimensions) and 1.5(in

three dimensions). From the simulation data, the total number of surviving bonds starting

from an initial conﬁguration t is counted. Yamamoto and Onuki (1998b) observed that the

breaking of the bonds in the supercooled liquid showed some intriguing correlations. In

two dimensions a substantial increase in the bond-breakage time τ

from 17 to 5 × 10

(expressed in the units indicated in Section 4.3.1) is seen for the liquid state on changing

the thermodynamic parameter for the binary system from 

eff

=1to

eff

=1.4 (see eqn.

(4.3.14) for the deﬁnition of 

eff

). In the supercooled state, the actual mapping of the

broken bonds displays strong clustering. Although it was identiﬁed in terms of a dynamic

quantity of bond breaking, a sort of static correlation function S

(q) of the broken bonds

is deﬁned with the following relation:

(q) =



i, j

exp(iq · R

)

, (10.2.11)

where R

=(r

(t) + r

(t))/2 is the center position of a broken pair at a time t in the

supercooled state and N

is the total number of broken bonds in the time interval [t, t +].

Yamamoto and Onuki (1998b) reported that the angle-averaged form of this correlation

function satisﬁes the Ornstein–Zernike form S

(0)/



1 + ξ



and identiﬁed a correlation

length ξ

that grows with the supercooling of the liquid. Hence, on considering a speciﬁc

type of properties of the supercooled state (distinct from the standard order parameters

such as density), signatures of a growing correlation are seen in the supercooled state.

Recently, Tanaka et al. (2010) associated a growing static length scale with various

supercooled liquids in simulations as well as from experiments. This correlation is again

identiﬁed not in terms of the usual density correlation functions but in terms of a new

structural order parameter related to bond-orientational order in the liquid. In some sys-

tems, such as poly-disperse colloids, one can identify static structural ordering via bond-

orientational order. In a two-dimensional binary soft-sphere mixture structural entropy has

498 The thermodynamic transition scenario

been used to identify the corresponding correlation function. However, such static order

has not been seen in a three-dimensional binary soft-sphere mixture. The viability of a

growing static correlation therefore remains somewhat questionable at this stage.

10.3 Self-generated disorder

Our discussion of the previous section is primarily based on thermodynamic considerations

of the supercooled liquid which is in a state of metastable equilibrium at a tempera-

ture T < T

. A full statistical-mechanical treatment of the problem (from a microscopic

approach) for the analysis of the dynamic behavior and the development of the barrier

to molecular rearrangement is still lacking. In the ﬁnal sections of this book we discuss

theories for the metastable state that have been formulated from a microscopic approach.

This also results in the extension of the ideas of statistical mechanics to the ideal glassy

state below T

. At moderate supercooling the dynamics is largely controlled by coupling

of dominant density ﬂuctuations, and is described in terms of the MCT discussed in the

previous chapters. Beyond a crossover temperature, T

(say), the dynamics is controlled

by thermally activated hopping of the system between different basins in the free-energy

landscape. These basins correspond to conﬁgurations with inhomogeneous density distri-

butions. As the temperature of the liquid is supercooled to the glass-transition temperature

, the liquid essentially freezes into a solid and attains a ﬁnite shear modulus. This is

interpreted as that the system is caught in a local minimum of the free energy with only

vibrational motion in the free-energy basin. In real space this implies that the particles

vibrate around their mean positions, which form a disordered lattice structure. Note that

this is a mean-ﬁeld picture in which the system is caught in a metastable minimum corre-

sponding to an amorphous structure. Using this picture, we now formulate a model for the

amorphous solid in terms of the interaction potential between the constituent particles of

this system.

10.3.1 Effective potential and overlap functions

In a mechanical description the classical liquid of N particles is represented by a point

in the 3N -dimensional conﬁguration space (Huang, 1987). Let us denote by “y” the con-

ﬁguration (i.e., the collection of 3N coordinates of all of the particles) reached when the

system is quenched to a temperature close to T

. For temperatures below T

the system

will remain “close” (in the phase space) to y. Small-scale vibrations are allowed in order

to equilibrate the system at the temperature of the heat bath, T (say). Thus we can view

the deeply supercooled liquid as one in which the slow degrees of freedom are quenched

and fast degrees of freedom thermalize at a temperature T . The parts of the phase space

comprising the points at a ﬁxed “distance” from y deﬁne a conditional statistical ensem-

ble. In order to study the behavior of the supercooled system, we focus here on the free

energy of the liquid corresponding to this ensemble. The ensemble is obtained in terms of

the partition function Z

, which involves summing over the constrained Gibbs–Boltzmann

10.3 Self-generated disorder 499

measure. The existence of the glassy state in the present context is given the following

interpretation: the free energy for the constrained state constitutes an “effective potential”

for the system and the minima of this potential represent the “equilibrium” state of the

glassy system.

Evaluation of the effective potential or the constrained free energy, i.e., the average of

ln Z

, requires using the replica trick developed for the spin-glass problem (Mézard et al.,

1987). The replicated system reduces to a multi-component mixture in which analytic con-

tinuation on the number of components has to be done. But it is important to note at this

point that the averaging in the spin glass is over the quenched impurities, which are absent

in the case of a structural glass. The frozen reference conﬁguration in fact plays the role of

quenched variables in the structural case.

We consider N identical point-like particles in a volume V and described by coordinates

} for i =1,...,N . We denote this conﬁguration simply as x. The interaction potential

between the particles is v(x

−x

). Let the conﬁguration of this system at T

be denoted y.

Below T

large-scale motions are frozen and only small-scale vibrations are allowed. We

devise a criterion to deﬁne the part of the conﬁguration space over which the distance from

the point y is small. This can be quantiﬁed by introduction of the following normalized

“distance” or “overlap” function:

q(x, y) =



i, j=1

o(|x

− y

|), (10.3.1)

where the function o(r) is deﬁned as

o(r) =

1, for r ≤ σ

0, for r >σ

(10.3.2)

with σ being the size of each particle and 

a small number less than 1. Note that, between

the two conﬁgurations, the ith particle in y can overlap with the jth particle in x. Thus the

centers in the respective cases can lie within a distance less than σ

. For conﬁgurations x

close to y the corresponding q(x, y) ≈ 1, whereas for widely separated conﬁgurations the

value of q is small. Using the above deﬁnition of the overlap function q(x, y), a restricted

Boltzmann–Gibbs distribution is deﬁned as one consisting of points at a ﬁxed “distance” q

from y,

P(x|y) =

−β H(x)

(β)

δ(q(x, y) − q), (10.3.3)

where Z

(β) is the integral over x of the numerator on the RHS of eqn. (10.3.3), and is

the partition function with respect to y as a frozen conﬁguration, β = 1/(k

T ), where

T is the temperature. In general, the above distribution will be strongly dependent on the

parent location y. This makes the outcome of a cooling experiment completely arbitrary

and impossible to associate with any measurement. Since by deﬁnition at the calorimetric

500 The thermodynamic transition scenario

glass-transition temperature T

the liquid freezes for all practical time scales, we expect

that the measure of y is obtained as

μ(y) ≈

−β

H(y)

Z(β

)

, (10.3.4)

where β

=1/(k

). The extensive quantities measured from the distribution function

(10.3.3) will be independent of y in the thermodynamic limit. A suitable interpretation of

this will be to take the frozen reference conﬁguration y as being like a quenched variable

that is effectively producing self-generated disorder in the structural glass.

Let us now consider the free energy associated with the distribution P(x|y) averaged

over the distribution of y,

(q,β) =−



−β H(y)

Z(β)





dx e

−β H(x)

δ(q(x, y) − q)



, (10.3.5)

where Z(β) is the integral of exp(−β H (y)) over y. Note that for simplicity we have

assumed that the distribution of the frozen conﬁguration y is controlled by the same tem-

perature as the vibrational degrees of freedom. The delta-function constraint implies that

for every y we restrict the measure of x to being only over the part of the phase space for

which the global constraint q(x, y) is equal to some ﬁxed value q. In order to devise a prac-

tical way to compute G

(q,β)satisfying the global constraint, we now introduce a “ﬁeld”

γ coupling to the overlap function q(x, y) in the Hamiltonian and deﬁne the corresponding

free energy as

F(γ, β) =−



−β H(y)

Z(β)





dx e

−β{H (x)−γ q(x,y)}



. (10.3.6)

On taking a derivative of F with respect to γ we obtain

∂F(γ )

∂γ

=−



−β H(y)

Z(β)



dx q(x, y)

−β{H (x)−γ q(x,y)}

dx e

−β{H (x)−γ q(x,y)}

. (10.3.7)

The global constraint of q(x, y) =q reduces the RHS of eqn. (10.3.7) to −q. Following the

standard method of taking the Legendre transform in thermodynamics, we make a change

of variable from γ to q by opting for the function G

(q,β)deﬁned as

(q,β) = min

{

F(γ ) + γ q

}

. (10.3.8)

This is the free energy of the glassy state as a function of the “distance” q which char-

acterizes the constrained Gibbs–Boltzmann measure. G

is also referred to as the effec-

tive potential for the glassy state, with its minima characterizing the equilibrium (in the

restricted sense) states of the supercooled liquid.