Coburn J.W., Herdlick J.D. College Algebra: Graphs and Models
Подождите немного. Документ загружается.

between them. Example 15 shows that when we multiply a binomial and its conjugate,
the “outers” and “inners” sum to zero and the result is a difference of two squares.
EXAMPLE 15
䊳
Multiplying Binomial Conjugates
Compute each product mentally:
a. b. c.
Solution
䊳
a. difference of squares
b. difference of squares:
c. difference of squares:
Now try Exercises 117 through 124
䊳
In summary, we have the following.
The Product of a Binomial and Its Conjugate
Given any expression that can be written in the form , the conjugate of the
expression is and their product is a difference of two squares:
Binomial Squares
Expressions like are called binomial squares and are useful for solving many
equations and sketching a number of basic graphs. Note
using the F-O-I-L process. The expression is called a
perfect square trinomial because it is the result of expanding a binomial square. If we
write a binomial square in the more general form and
compute the product, we notice a pattern that helps us write the expanded form
more quickly.
repeated multiplication
F-O-I-L
simplify (perfect square trinomial)
The first and last terms of the trinomial are squares of the terms A and B. Also, the
middle term of the trinomial is twice the product of these two terms:
The F-O-I-L process shows us why. Since the outer and inner products are identical,
we always end up with two. A similar result holds for and the process can be
summarized for both cases using the symbol.
1A B2
2
AB AB 2AB.
A
2
2AB B
2
A
2
AB AB B
2
1A B2
2
1A B21A B2
1A B2
2
1A B21A B2
x
2
14x 49x
2
14x 49
1x 72
2
1x 721x 72
1x 72
2
1A B21A B2 A
2
B
2
A B
A B
x
2
a
2
5
b
2
ax
2
5
b ax
2
5
b x
2
4
25
0
2
5
x
2
5
x
12x2
2
15y2
2
12x 5y212x 5y2 4x
2
25y
2
10xy (10xy) 0xy
1x2
2
172
2
1x 721x 72 x
2
49
7x 7x 0x
ax
2
5
b ax
2
5
b12x 5y212x 5y21x 721x 72
20 CHAPTER R A Review of Basic Concepts and Skills R–20
College Algebra Graphs & Models—
LOOKING AHEAD
Although a binomial square can
always be found using repeated
factors and F-O-I-L, learning to
expand them using the pattern is
a valuable skill. Binomial squares
occur often in a study of algebra
and it helps to find the expanded
form quickly.
cob19545_chR_011-024.qxd 11/22/10 10:46 AM Page 20
R–21 Section R.2 Exponents, Scientific Notation, and a Review of Polynomials 21
College Algebra Graphs & Models—
The Square of a Binomial
Given any expression that can be written in the form
1.
2.
CAUTION
䊳
Note the square of a binomial always results in a trinomial (three terms). In particular,
.
EXAMPLE 16
䊳
Find each binomial square without using F-O-I-L:
a. b. c.
Solution
䊳
a.
simplify
b.
simplify
c.
simplify
Now try Exercises 125 through 136
䊳
With practice, you will be able to go directly from the binomial square to the
resulting trinomial.
9 61x
x
1A B2
2
A
2
2AB B
2
13 1x2
2
9 213
#
1x2 11x2
2
9x
2
30x 25
1A B2
2
A
2
2AB B
2
13x 52
2
13x2
2
213x
#
52 5
2
a
2
18a 81
1A B2
2
A
2
2AB B
2
1a 92
2
a
2
21a
#
92 9
2
13 1x2
2
13x 52
2
1a 92
2
1A B2
2
A
2
B
2
1A B2
2
A
2
2AB B
2
1A B2
2
A
2
2AB B
2
1A B2
2
,
F. You’ve just seen how
we can compute special
products: binomial conjugates
and binomial squares
4. The expression can be classified as
a of degree , with a leading
coefficient of .
5. Discuss/Explain why one of the following
expressions can be simplified further, while the
other cannot: (a) (b)
6. Discuss/Explain why the degree of is greater
than the degree of Include additional
examples for contrast and comparison.
2x
2
y
3
.
2x
2
y
3
7n
4
#
3n
2
.7n
4
3n
2
;
2x
2
3x 10
䊳
CONCEPTS AND VOCABULARY
Fill in each blank with the appropriate word or phrase. Carefully reread the section, if necessary.
R.2 EXERCISES
1. The equation is an example of the
property of exponents.
2. The equation is an example of the
property of exponents.
3. The sum of the “outers” and “inners” for
is , while the sum of the outers and inners
for is .
12x 5212x 52
12x 52
2
1x
3
2
2
1
x
6
1x
2
2
3
x
6
䊳
DEVELOPING YOUR SKILLS
Determine each product using the product and/or power properties.
7. 8. 9.
10. 11. 12. d
2
#
d
4
#
1c
5
2
2
#
1c
3
2
2
1a
2
2
4
#
1a
3
2
2
#
b
2
#
b
5
11.5vy
2
218v
4
y2
16p
2
q212p
3
q
3
224g
5
#
3
8
g
9
2
3
n
2
#
21n
5
cob19545_chR_011-024.qxd 11/22/10 10:46 AM Page 21
22 CHAPTER R A Review of Basic Concepts and Skills R–22
College Algebra Graphs & Models—
Simplify using the product to a power property.
13. 14.
15. 16.
17. 18.
19. 20.
21. 22.
23. 24.
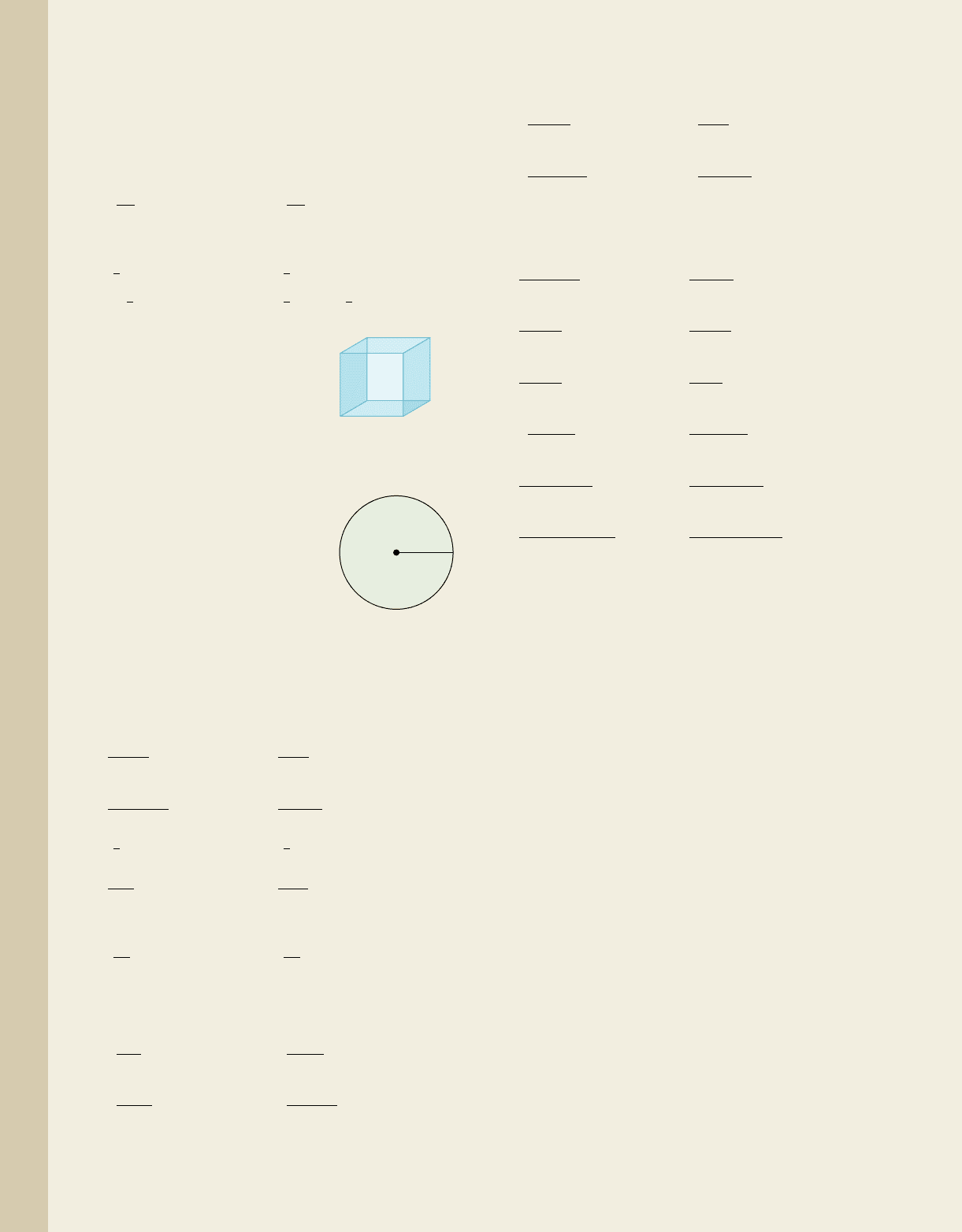
25. Volume of a cube: The
formula for the volume of a
cube is where S is the
length of one edge. If the
length of each edge is
a. Find a formula for volume
in terms of the variable x.
b. Find the volume of the cube if
26. Area of a circle: The formula
for the area of a circle is
where r is the length
of the radius. If the radius is
given as
a. Find a formula for area in
terms of the variable x.
b. Find the area of the circle if
Simplify using the quotient property or the property of
negative exponents. Write answers using positive
exponents only.
27. 28.
29. 30.
31. 32.
33. 34.
35. 36.
37. 38.
Simplify each expression using the quotient to a power
property.
39. 40.
41. 42. a
0.5a
3
0.4b
2
b
2
a
0.2x
2
0.3y
3
b
3
a
5v
4
7w
3
b
2
a
2p
4
q
3
b
2
1
2
3
2
2
1
1
2
2
3
142
2
122
3
3
m
2
2
h
3
1
5
6
2
1
1
2
3
2
3
5m
3
n
5
10mn
2
12a
3
b
5
4a
2
b
4
8z
7
16z
5
6w
5
2w
2
x 2.
5x
3
,
A r
2
,
x 2.
3x
2
,
V S
3
,
1
2
3
m
2
n2
2
#
1
1
2
mn
2
21
3
8
x2
2
116xy
2
2
1
4
5
x
3
2
2
1
3
4
x
3
y2
2
12.5a
3
2
2
13a
2
b
2
2
3
10.7c
4
2
2
110c
3
d
2
2
2
a
b
3a
b
3
a
p
2q
b
2
12.5h
5
k2
2
13.2hk
2
2
3
13p
2
q2
2
16pq
2
2
3
43. 44.
45. 46.
Use properties of exponents to simplify the following.
Write the answer using positive exponents only.
47. 48.
49. 50.
51. 52.
53. 54.
55. 56.
57. 58.
59. 60.
61. 62.
63. 64.
65. 66.
Convert the following numbers to scientific notation.
67. In mid-2009, the U.S. Census Bureau estimated the
world population at nearly 6,770,000,000 people.
68. The mass of a proton is generally given as
0.000 000 000 000 000 000 000 000 001 670 kg.
Convert the following numbers to decimal notation.
69. The smallest microprocessors in common use
measure across.
70. In 2009, the estimated net worth of Bill Gates, the
founder of Microsoft, was dollars.
Compute using scientific notation. Show all work.
71. The average distance between the Earth and the
planet Jupiter is 465,000,000 mi. How many hours
would it take a satellite to reach the planet if it
traveled an average speed of 17,500 mi per hour?
How many days? Round to the nearest whole.
72. In fiscal terms, a nation’s debt-per-capita is the
ratio of its total debt to its total population. In the
year 2009, the total U.S. debt was estimated at
$11,300,000,000,000, while the population was
estimated at 305,000,000. What was the U.S. debt-
per-capita ratio for 2009? Round to the nearest
whole dollar.
5.8 10
10
6.5 10
9
m
2n
0
12n2
0
5x
0
15x2
0
2
2
2
1
2
0
3
0
3
1
3
2
4
1
8
1
2
1
5
1
132
0
172
0
4
0
5
0
312x
3
y
4
z2
2
18x
2
yz
0
14a
3
bc
0
713a
2
b
2
c2
3
18n
3
813n
2
2
3
612x
3
2
2
10x
2
1p
4
q
8
2
2
p
5
q
2
a
a
3
#
b
c
2
b
4
15
3
2
4
5
9
1a
2
2
3
a
4
#
a
5
5k
3
20k
2
20h
2
12h
5
5m
5
n
2
10m
5
n
9p
6
q
4
12p
4
q
6
a
9p
3
q
2
r
3
12p
5
qr
2
b
3
a
5p
2
q
3
r
4
2pq
2
r
4
b
2
a
4p
3
3x
2
y
b
3
a
5m
2
n
3
2r
4
b
2
3x
2
3x
2
3x
2
5x
3
cob19545_chR_011-024.qxd 7/28/10 2:24 PM Page 22

R–23 Section R.2 Exponents, Scientific Notation, and a Review of Polynomials 23
College Algebra Graphs & Models—
96.
97.
98.
99.
100.
101. 102.
103. 104.
105. 106.
107. 108.
109. 110.
111. 112.
113. 114.
115. 116.
For each binomial, determine its conjugate and find the
product of the binomial with its conjugate.
117. 118.
119. 120.
121. 122.
123. 124.
Find each binomial square.
125. 126.
127. 128.
129. 130.
131. 132.
Compute each product.
133.
134.
135.
136. 1a 621a 121a 52
1k 521k 621k 22
1a 321b 52
1x 321y 22
11x 72
2
14 1x2
2
15c 6d2
2
14p 3q2
2
15x 32
2
14g 32
2
1a 32
2
1x 42
2
p 12x 16
11 3r6 5k
c 37x 10
6n 54m 3
13y
2
2212y
2
1212x
2
521x
2
32
15x 3y212x 3y214c d213c 5d2
16a b21a 3b213x 2y212x 5y2
1n
2
5
21n
2
5
21m
3
4
21m
3
4
2
1z
1
3
21z
5
6
21x
1
2
21x
1
4
2
1q 4.921q 1.221p 2.521p 3.62
15 n215 n213 m213 m2
16w 1212w 5217v 4213v 52
12h
2
3h 821h 12
1b
2
3b 2821b 22
1z 521z
2
5z 252
1x 321x
2
3x 92
1s 3215s 42
Identify each expression as a polynomial or
nonpolynomial (if a nonpolynomial, state why);
classify each as a monomial, binomial, trinomial, or
none of these; and state the degree of the polynomial.
73.
74.
75. 76.
77. 78.
Write each polynomial in standard form and name the
leading coefficient.
79.
80.
81.
82.
83.
84.
Find the indicated sum or difference.
85.
86.
87.
88.
89.
90.
91. Subtract from
using a vertical format.
92. Find decreased by
using a vertical format.
Compute each product.
93.
94.
95. 13r 521r 22
2v
2
1v
2
2v 152
3x1x
2
x 62
4x
2
3xx
4
3x
3
x
4
2x
3
x
2
2x
q
4
2q
3
q
6
2q
5
q
5
2q
4
q
2
2q
1
5
9
n
2
4n
1
2
2 1
2
3
n
2
2n
3
4
2
1
3
4
x
2
5x 22 1
1
2
x
2
3x 42
10.4n
2
5n 0.52 10.3n
2
2n 0.752
15.75b
2
2.6b 1.92 12.1b
2
3.2b2
15q
2
3q 42 13q
2
3q 42
13p
3
4p
2
2p 72 1p
2
2p 52
8 2n
2
7n
12
2
3
x
2
3v
3
14 2v
2
112v2
c
3
6 2c
2
3c
2k
2
12 k
7w 8.2 w
3
3w
2
q
3
2q
2
5qp
3
2
5
4
r
3
2.7r
2
r 15n
2
4n 117
2x
3
2
3
x
2
12x 1.2
35w
3
2w
2
112w2 14
䊳
WORKING WITH FORMULAS
137. Medication in the bloodstream:
If 400 mg of a pain medication are taken orally, the number of milligrams in the bloodstream is modeled by the
formula shown, where M is the number of milligrams and t is the time in hours, Construct a table of
values for through 5, then answer the following.
a. How many milligrams are in the bloodstream after 2 hr? After 3 hr?
b. Based on part a,would you expect the number of milligrams in the bloodstream after 4 hr to be less or more?Why?
c. Approximately how many hours until the medication wears off (the number of milligrams in the bloodstream is 0)?
t 1
0 t 6 5.
M ⴝ 0.5t
4
ⴙ 3t
3
ⴚ 97t
2
ⴙ 348t
cob19545_chR_011-024.qxd 11/22/10 10:48 AM Page 23

24 CHAPTER R A Review of Basic Concepts and Skills R–24
College Algebra Graphs & Models—
138. Amount of a mortgage payment:
The monthly mortgage payment required to pay off (or amortize) a loan is given by the formula shown, where M is
the monthly payment, A is the original amount of the loan, r is the annual interest rate, and n is the term of the loan
in months. Find the monthly payment (to the nearest cent) required to purchase a $198,000 home, if the interest rate
is 6.5% and the home is financed over 30 yr.
M ⴝ
Aa
r
12
b a1 ⴙ
r
12
b
n
a1 ⴙ
r
12
b
n
ⴚ 1
䊳
APPLICATIONS
139. Attraction between particles: In electrical theory,
the force of attraction between two particles P and
Q with opposite charges is modeled by ,
where d is the distance between them and k is a
constant that depends on certain conditions. This is
known as Coulomb’s law. Rewrite the formula
using a negative exponent.
140. Intensity of light: The intensity of illumination
from a light source depends on the distance from
the source according to , where I is the
intensity measured in footcandles, d is the distance
from the source in feet, and k is a constant that
depends on the conditions. Rewrite the formula
using a negative exponent.
141. Rewriting an expression: In advanced mathematics,
negative exponents are widely used because they are
easier to work with than rational expressions.
Rewrite the expression using
negative exponents.
142. Swimming pool hours: A swimming pool opens at
8
A
.
M
. and closes at 6
P
.
M
. In summertime, the
5
x
3
3
x
2
2
x
1
4
I
k
d
2
F
kPQ
d
2
number of people in the pool at any time can be
approximated by the formula ,
where S is the number of swimmers and t is the
number of hours the pool has been open (8
A
.
M
.:
9
A
.
M
.: 10
A
.
M
.: etc.).
a. How many swimmers are in the pool at 6
P
.
M
.?
Why?
b. Between what times would you expect the
largest number of swimmers?
c. Approximately how many swimmers are in the
pool at 3
P
.
M
.?
d. Create a table of values for
and check your answer to part b.
143. Maximizing revenue:A sporting goods store finds
that if they price their video games at $20, they
make 200 sales per day. For each decrease of $1,
20 additional video games are sold. This means the
store’s revenue can be modeled by the formula
where x is the number
of $1 decreases. Multiply out the binomials and use
a table of values to determine what price will give
the most revenue.
144. Maximizing revenue: Due to past experience, a
jeweler knows that if they price jade rings at $60,
they will sell 120 each day. For each decrease of
$2, five additional sales will be made. This means
the jeweler’s revenue can be modeled by the
formula where x is the
number of $2 decreases. Multiply out the
binomials and use a table of values to determine
what price will give the most revenue.
R 160 2x21120 5x2,
R 120 1x21200 20x2,
4, . . .t 1, 2, 3,
t 2,t 1,t 0,
S1t2t
2
10t
䊳
EXTENDING THE CONCEPT
145. If
what is the
value of k?
12x
2
4x k2x
2
3x 2,
13x
2
kx 12 1kx
2
5x 72
146. If then the expression
is equal to what number?
4x
2
1
4x
2
a2x
1
2x
b
2
5,
cob19545_chR_011-024.qxd 7/28/10 2:25 PM Page 24
College Algebra Graphs & Models—
LEARNING OBJECTIVES
In Section R.3 you will review how to:
A. Solve linear equations
using properties of
equality
B. Recognize equations that
are identities or
contradictions
C. Solve linear inequalities
D. Solve compound
inequalities
E. Solve basic applications
of linear equations and
inequalities
F. Solve applications of
basic geometry
In a study of algebra, you will encounter many families of equations, or groups of
equations that share common characteristics. Of interest to us here is the family of
linear equations in one variable, a study that lays the foundation for understanding
more advanced families. This section will also lay the foundation for solving a formula for
a specified variable, a practice widely used in science, business, industry, and research.
A. Solving Linear Equations Using Properties of Equality
An equation is a statement that two expressions are
equal. From the expressions and
we can form the equation
which is a linear equation in one variable (the
exponent on any variable is a 1). To solve an equa-
tion, we attempt to find a specific input or x-value
that will make the equation true, meaning the left-
hand expression will be equal to the right. Using
Table R.1, we find that is a
true equation when x is replaced by 2, and is a false
equation otherwise. Replacement values that make
the equation true are called solutions or roots of the equation.
31x ⫺ 12⫹ x ⫽⫺x ⫹ 7
31x ⫺ 12⫹ x ⫽⫺x ⫹ 7,
⫺x ⫹ 7,
31x ⫺ 12⫹ x
R.3 Solving Linear Equations and Inequalities
x
9
8
07
116
255
394
4133
⫺3
⫺7⫺1
⫺11⫺2
ⴚx ⴙ 731x ⴚ 12 ⴙ x
Table R.1
CAUTION
䊳
From Section R.1, an algebraic expression is a sum or difference of algebraic terms.
Algebraic expressions can be simplified, evaluated or written in an equivalent form, but
cannot be “solved,” since we’re not seeking a specific value of the unknown.
Solving equations using a table is too time consuming to be practical. Instead we
attempt to write a sequence of equivalent equations, each one simpler than the one be-
fore, until we reach a point where the solution is obvious. Equivalent equations are those
that have the same solution set, and can be obtained by using the distributive property to
simplify the expressions on each side of the equation. The additive and multiplicative
properties of equality are then used to obtain an equation of the form
The Additive Property of Equality The Multiplicative Property of Equality
If A, B, and C represent algebraic If A, B, and C represent algebraic
expressions and expressions and ,
then then and
In words, the additive property says that like quantities, numbers, or terms can be
added to both sides of an equation. A similar statement can be made for the multiplica-
tive property. These properties are combined into a general guide for solving linear
equations, which you’ve likely encountered in your previous studies. Note that not all
steps in the guide are required to solve every equation.
1C ⫽ 02
A
C
⫽
B
C
,AC ⫽ BCA ⫹ C ⫽ B ⫹ C
A ⫽ BA ⫽ B,
x ⫽ constant.
R–25 25
cob19545_chR_025-039.qxd 11/23/10 7:05 PM Page 25
Guide to Solving Linear Equations in One Variable
• Eliminate parentheses using the distributive property, then combine any like terms.
• Use the additive property of equality to write the equation with all variable terms
on one side, and all constants on the other. Simplify each side.
• Use the multiplicative property of equality to obtain an equation of the form
• For applications, answer in a complete sentence and include any units of measure
indicated.
For our first example, we’ll use the equation from our
initial discussion.
EXAMPLE 1
䊳
Solving a Linear Equation Using Properties of Equality
Solve for x:
Solution
䊳
original equation
distributive property
combine like terms
add
x
to both sides (additive property of equality)
add 3 to both sides (additive property of equality)
multiply both sides by or divide both sides by 5
(multiplicative property of equality)
As we noted in Table R.1, the solution is
Now try Exercises 7 through 12
䊳
To check a solution by substitution means we substitute the solution back into the
original equation (this is sometimes called back-substitution), and verify the left-
hand side is equal to the right. For Example 1 we have:
original equation
substitute 2 for
x
simplify
✓ solution checks
If any coefficients in an equation are fractional, multiply both sides by the least
common denominator (LCD) to clear the fractions. Since any decimal number can be
written in fraction form, the same idea can be applied to decimal coefficients.
EXAMPLE 2
䊳
Solving a Linear Equation with Fractional Coefficients
Solve for n:
Solution
䊳
original equation
distributive property
combine like terms
multiply both sides by LCD
distributive property
subtract 2
n
multiply by
Verify the solution is using back-substitution.
Now try Exercises 13 through 30
䊳
n 12
1 n 12
n 12
n 2n 12
4 4 1
1
4
n2 41
1
2
n 32
1
4
n
1
2
n 3
1
4
n 2 2
1
2
n 3
1
4
1n 82 2
1
2
1n 62
1
4
1n 82 2
1
2
1n 62.
5 5
3 112 2 5
3 12 12 2 2 7
3 1x 12 x x 7
x 2.
1
5
x 2
5 x 10
5 x 3 7
4 x 3 x 7
3 x 3 x x 7
3 1x 12 x x 7
31x 12 x x 7.
31x 12 x x 7
x constant.
26 CHAPTER R A Review of Basic Concepts and Skills R–26
College Algebra Graphs & Models—
A. You’ve just seen how
we can solve linear equations
using properties of equality
cob19545_chR_025-039.qxd 7/28/10 2:35 PM Page 26

B. Identities and Contradictions
Example 1 illustrates what is called a conditional equation, since the equation is true for
but false for all other values of x. The equation in Example 2 is also conditional. An
identity is an equation that is always true, no matter what value is substituted for the vari-
able. For instance, is an identity with a solution set of all real num-
bers, written as or in interval notation. Contradictions are
equations that are never true, no matter what real number is substituted for the variable.
The equations and are contradictions. To state the solution set
for a contradiction, we use the symbol “ (the null set) or “{ }” (the empty set). Recog-
nizing these special equations will prevent some surprise and indecision in later chapters.
EXAMPLE 3
䊳
Solving Equations (Special Cases)
Solve each equation and state the solution set.
a. b.
Solution
䊳
a. original equation
distributive property
combine like terms
subtract 12
x
; contradiction
Since is never equal to 12, the original equation is a contradiction.
The solution set is empty: { }
b.
original equation
distributive property
combine like terms
subtract 18
x
; identity
The result shows that the original equation is an identity, with an infinite
number of solutions: . You may recall this notation is read, “the set
of all numbers x, such that x is a real number.”
Now try Exercises 31 through 36
䊳
In Example 3(a), our attempt to solve for x ended with all variables being eliminated,
leaving an equation that is always false—a contradiction ( is never equal to 12).
There is nothing wrong with the solution process, the result is simply telling us the
original equation has no solution. In Example 3(b), all variables were again eliminated
but the end result was always true—an identity ( is always equal to ). Once
again we’ve done nothing wrong mathematically, the result is just telling us that the
original equation will be true no matter what value of x we use for an input.
C. Solving Linear Inequalities
A linear inequality resembles a linear equality in many respects:
Linear Inequality Related Linear Equation
(1)
(2)
A linear inequality in one variable is one that can be written in the form
where a, b, and and This definition and the following properties also apply
when other inequality symbols are used. Solutions to simple inequalities are easy to spot.
For instance, is a solution to since For more involved inequal-
ities we use the additive property of inequality and the multiplicative property of
2 6 3.x 6 3x 2
a 0.c 僆 ⺢
ax b 6 c,
3
8
p 2 12
3
8
p 2 12
x 3x 6 3
66
8
5x | x 僆 ⺢6
6 6
18x 6 18x 6
8 x 6 10x 24 18x 30
8 x 16 10x2 24 613x 52
8
8 12
12x 8 12x 12
2 x 8 10x 8 12x 4
2 1x 42 10x 8 413x 12
8x 16 10x2 24 613x 5221x 42 10x 8 413x 12
”
3 1x 3 x 1
x 僆 1q, q25x|x 僆 ⺢6,
21x 32 2x 6
x 2,
R–27 Section R.3 Solving Linear Equations and Inequalities 27
College Algebra Graphs & Models—
B. You’ve just seen how
we can recognize equations
that are identities or
contradictions
cob19545_chR_025-039.qxd 11/22/10 9:32 AM Page 27

inequality. Similar to solving equations, we solve inequalities by isolating the variable
on one side to obtain a solution form such as
The Additive Property of Inequality
If A, B, and C represent algebraic expressions and ,
then
Like quantities (numbers or terms) can be added to both sides of an inequality.
While there is little difference between the additive property of equality and the
additive property of inequality, there is an important difference between the multiplica-
tive property of equality and the multiplicative property of inequality. To illustrate, we
begin with Multiplying both sides by positive three yields a true
inequality. But notice what happens when we multiply both sides by negative three:
original inequality
multiply by negative three
false
The result is a false inequality, because 6 is to the right of on the number line.
Multiplying (or dividing) an inequality by a negative quantity reverses the order rela-
tionship between two quantities (we say it changes the sense of the inequality). We
must compensate for this by reversing the inequality symbol.
change direction of symbol to maintain a true statement
For this reason, the multiplicative property of inequality is stated in two parts.
The Multiplicative Property of Inequality
If A, B, and C represent algebraic If A, B, and C represent algebraic
expressions and expressions and
then then
if C is a positive quantity if C is a negative quantity
(inequality symbol remains the same). (inequality symbol must be reversed).
EXAMPLE 4
䊳
Solving an Inequality
Solve the inequality, then graph the solution set and write it in interval notation:
Solution
䊳
original inequality
clear fractions (multiply by LCD)
simplify
subtract 3
divide by ,
reverse inequality sign
result
• Graph:
• Interval notation:
Now try Exercises 37 through 46
䊳
x 僆 3
1
2
, q2
x
1
2
4
4x
4
ⱖ
2
4
4x 2
4x 3 5
6
a
2
3
x
1
2
b 162
5
6
2
3
x
1
2
5
6
2
3
x
1
2
5
6
.
AC 7 BCAC 6 BC
A 6 B,A 6 B,
6 7 15
15
6 6 15
21326 5132
2 6 5
6 6 15,2 6 5.
A C 6 B C
A 6 B
variable 6 number.
28 CHAPTER R A Review of Basic Concepts and Skills R–28
College Algebra Graphs & Models—
[
1123 2 340
1
2
WORTHY OF NOTE
As an alternative to multiplying or
dividing by a negative value, the
additive property of inequality can
be used to ensure the variable term
will be positive. From Example 4,
the inequality can be
written as by adding 4x to
both sides and subtracting 2 from
both sides. This gives the solution
which is equivalent to
x
1
2
.
1
2
x,
2 4x
4x 2
cob19545_chR_025-039.qxd 7/28/10 2:35 PM Page 28

To check a linear inequality, you often have an infinite number of choices—any
number from the solution set/interval. If a test value from the solution interval results
in a true inequality, all numbers in the interval are solutions. For Example 4, using
results in the true statement ✓.
Some inequalities have all real numbers as the solution set: while other
inequalities have no solutions, with the answer given as the empty set: { }.
EXAMPLE 5
䊳
Solving Inequalities
Solve the inequality and write the solution in set notation:
a. b.
Solution
䊳
a. original inequality
distributive property
combine like terms
add 3
x
Since the resulting statement is always true, the original inequality is true for
all real numbers. The solution is all real numbers .
b.
original inequality
distribute
combine like terms
subtract 3
x
Since the resulting statement is always false, the original inequality is false for
all real numbers. The solution is { }.
Now try Exercises 47 through 52
䊳
D. Solving Compound Inequalities
In some applications of inequalities, we must consider more than one solution interval.
These are called compound inequalities, and these require us to take a close look at
the operations of union “ ” and intersection “ ”. The intersection of two sets A and
B, written , is the set of all elements common to both sets. The union of two sets
A and B, written , is the set of all elements that are in either set. When stating the
union of two sets, repetitions are unnecessary.
EXAMPLE 6
䊳
Finding the Union and Intersection of Two Sets
For set and set ,
determine and .
Solution
䊳
is the set of all elements in both A and B:
is the set of all elements in either A or B:
Now try Exercises 53 through 58
䊳
Notice the intersection of two sets is described using the word “and,” while the
union of two sets is described using the word “or.” When compound inequalities are
formed using these words, the solution is modeled after the ideas from Example 6. If
“and” is used, the solutions must satisfy both inequalities. If “or” is used, the solutions
can satisfy either inequality.
A ´ B 52, 1, 0, 1, 2, 3, 4, 56.
A ´ B
A 傽 B 51, 2, 36.
A ¨ B
A ´ BA ¨ B
B 51, 2, 3, 4, 56A 52, 1, 0, 1, 2, 36
A ´ B
A ¨ B
¨´
7 6 6
3 x 7 6 3x 6
3x 12 5 6 2x 6 x
31x 42 5 6 21x 32 x
⺢
2 8
3x 2 3x 8
7 3x 5 2x 8 5x
7 13x 52 21x 42 5x
31x 42 5 6 21x 32 x7 13x 52 21x 42 5x
5x|
x 僆 ⺢6,
1
2
5
6
x 0
College Algebra Graphs & Models—
C. You’ve just seen how
we can solve linear inequalities
WORTHY OF NOTE
For the long term, it may help to
rephrase the distinction as follows.
The intersection is a selection of
elements that are common to two
sets, while the union is a collection
of the elements from two sets (with
no repetitions).
R–29 Section R.3 Solving Linear Equations and Inequalities 29
cob19545_chR_025-039.qxd 11/22/10 9:32 AM Page 29
