CIMA - C3 Fundamentals Of Business Mathematics

Подождите немного. Документ загружается.

170 6: Probability ⏐ Part C Probability

2.4 Complementary outcomes

You are desperate to pass more of the examinations than your sworn enemy but, unlike you, he is more likely to

pass the first examination if it is difficult. (He is very strange!) What is the probability of the first examination being

more suited to your enemy's requirements?

We know that the probability of certainty is one. The certainty in this scenario is that the examination will be easy

or difficult.

P(easy or difficult examination) = 1

From Paragraph 2.2, P(easy examination) = 1/2

P(not easy examination) = P(difficult examination)

= 1 – P(easy examination)

= 1 – 1/2

= 1/2

(A) = 1 – P(A), where A is 'not A'.

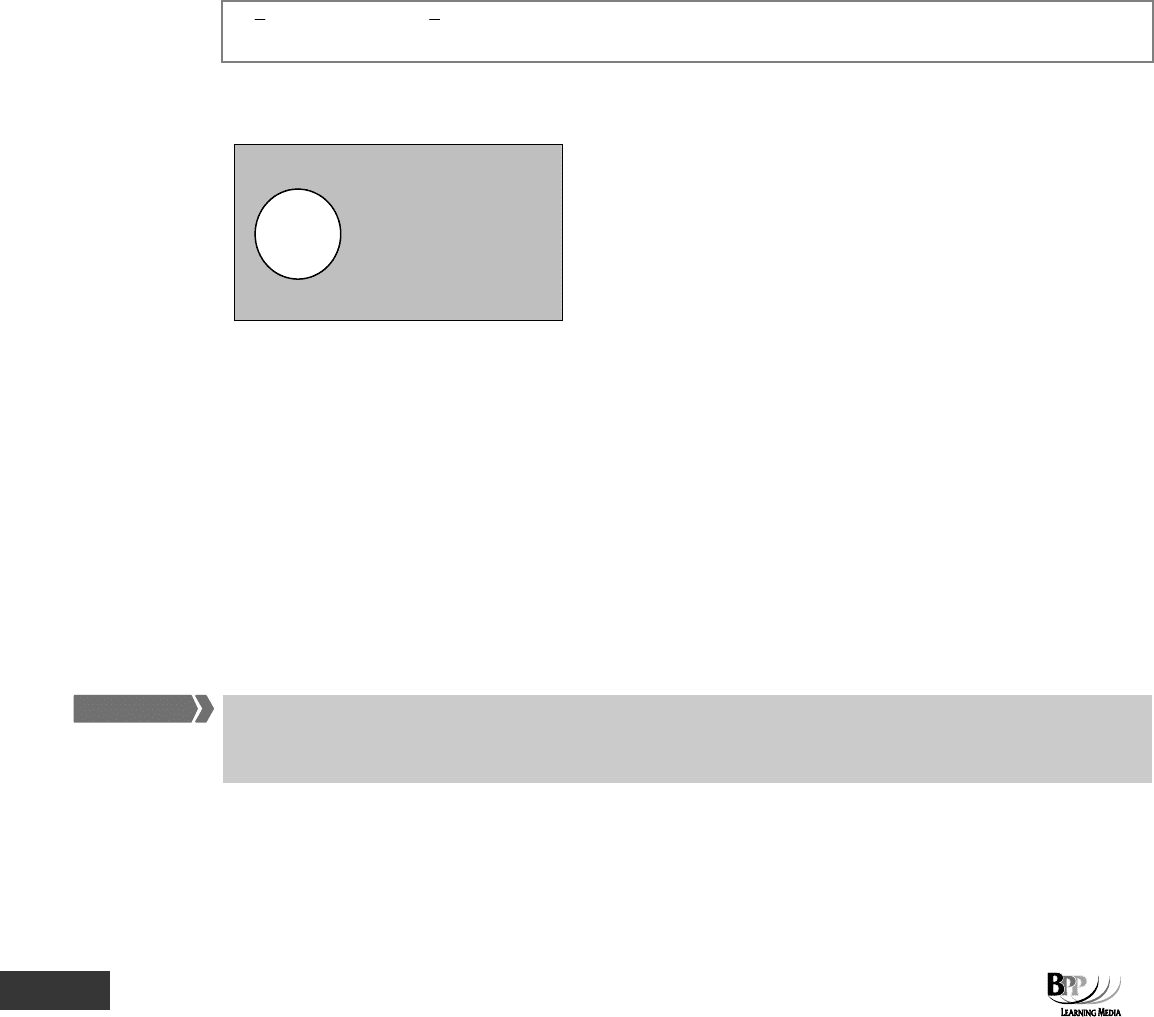
2.4.1 Venn diagram: Complementary outcomes

The probability of

not A is shown by the shaded region.

2.4.2 Example: Complementary outcomes

If there is a 25 per cent chance of the Rainbow Party winning the next general election, use the law of

complementary events to calculate the probability of the Rainbow Party

not winning the next election.

Solution

P(winning) = 25% = 1/4

P(not winning) = 1 – P(winning) = 1 –1/4 = 3/4

2.5 The simple addition or OR law

The simple addition law for two mutually exclusive events, A and B, is as follows.

P(A or B) = P (A ∪ B) = P(A) + P(B)

The time pressure in the second examination is enormous. The computer will produce a paper which will have

between five and nine questions. You know that, easy or difficult, the examination must have six questions at the

most for you to have any hope of passing it.

What is the probability of the computer producing an examination with six or fewer questions? In other words,

what is the probability of an examination with five

or six questions?

Formula to

learn

T F

RWAR

Part C Probability ⏐ 6: Probability 171

Don't panic. Let us start by using the basic principle.

P(5 questions) =

questions) 9 or 8 7, 6, 5,(= outcomes possible of number Total

nexaminatioquestionfiveaachievingofwaysofnumber Total

Likewise P(6 questions) =

Either five questions or six questions would be acceptable, so the probability of you passing the examination must

be greater than if just five questions or just six questions (but not both) were acceptable. We therefore add the two

probabilities together so that the probability of passing the examination has increased.

So P(5 or 6 questions) = P(5 questions) + P(6 questions)

Mutually exclusive outcomes are outcomes where the occurrence of one of the outcomes excludes the possibility

of any of the others happening.

In the example the outcomes are mutually exclusive because it is impossible to have five questions and six

questions in the same examination.

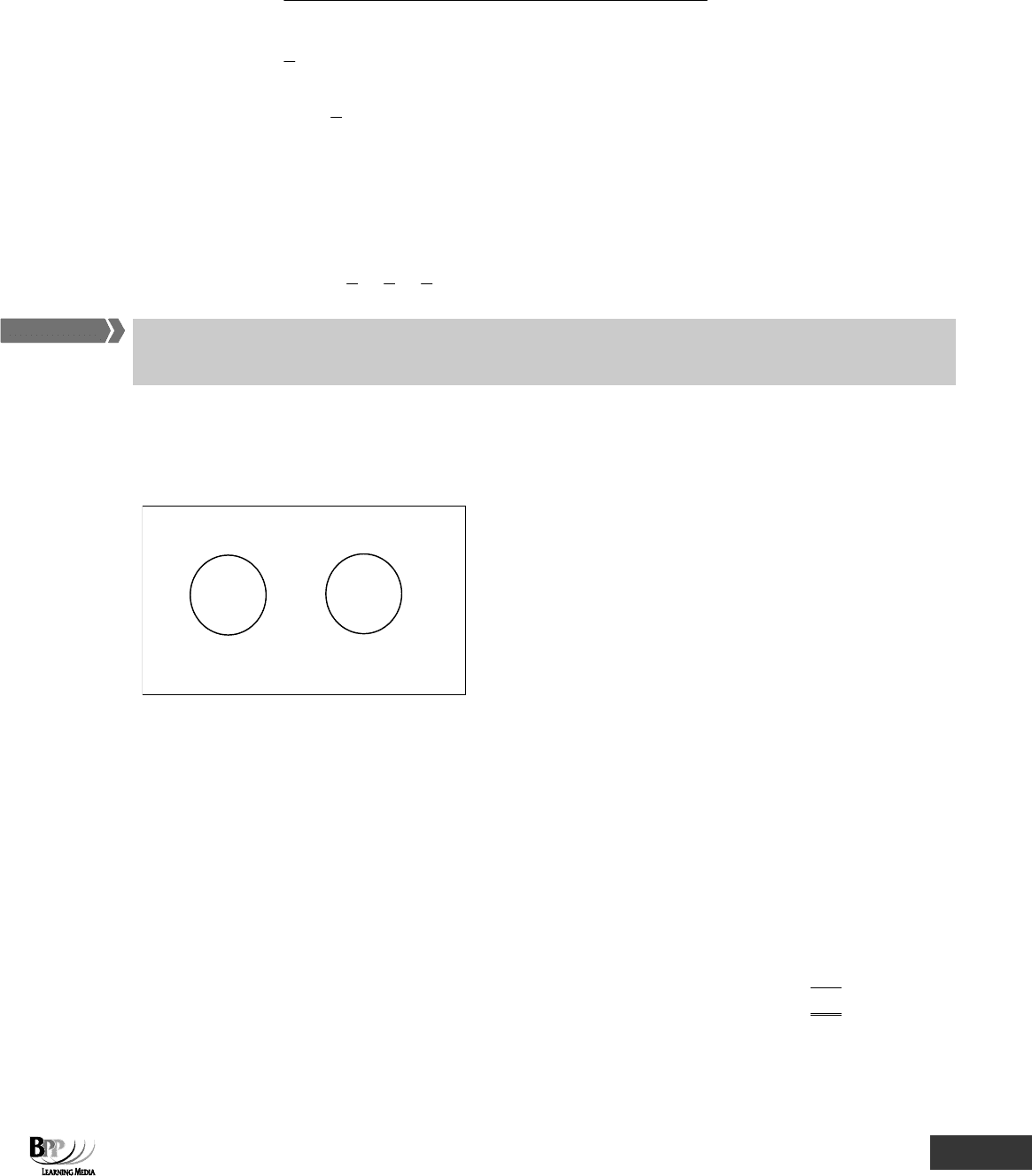
2.5.1 Venn diagram: Mutually exclusive outcomes

2.5.2 Example: Mutually exclusive outcomes

The delivery of an item of raw material from a supplier may take up to six weeks from the time the order is placed.

The probabilities of various delivery times are as follows.

Delivery time

Probability

≤ 1 week

0.10

> 1,

≤ 2 weeks

0.25

> 2,

≤ 3 weeks

0.20

> 3,

≤ 4 weeks

0.20

> 4,

≤ 5 weeks

0.15

> 5,

≤ 6 weeks

0.10

1.00

Required

Calculate the probability that a delivery will take the following times.

T F

RWAR

172 6: Probability ⏐ Part C Probability

(a) Two weeks or less

(b) More than three weeks

Solution

(a) P (≤ 1 or > 1, ≤ 2 weeks) = P (≤ 1 week) + P (>1, ≤ 2 weeks)

= 0.10 + 0.25

= 0.35

(b) P (> 3,

≤ 6 weeks) = P (> 3, ≤ 4 weeks) + P (> 4, ≤ 5 weeks) + P (> 5, ≤ 6 weeks)

= 0.20 + 0.15 + 0.10

= 0.45

2.6 The simple multiplication or AND law

The simple multiplication law for two independent events, A and B, is as follows.

P(A and B) = P (A ∩ B) = P(A)P(B)

P(A and B) = 0 when A and B have mutually exclusive outcomes.

You still have three examinations to sit: astrophysics, geography of the moon and computer art. Stupidly, you

forgot to revise for the astrophysics examination, which will have between 15 and 20 questions. You think that you

may scrape through this paper if it is easy

and if there are only 15 questions.

What is the probability that the paper the computer produces will exactly match your needs? Do not forget that

there is no link between the standard of the examination and the number of questions ie they are

independent

events.

The best way to approach this question is diagrammatically, showing all the possible outcomes.

Number of questions

Type of paper 15 16 17 18 19 20

Easy (E) E and 15* E and 16 E and 17 E and 18 E and 19 E and 20

Difficult (D) D and 15 D and 16 D and 17 D and 18 D and 19 D and 20

The diagram shows us that, of the twelve possible outcomes, there is only one 'desired result' (which is

asterisked). We can therefore calculate the probability as follows.

P(easy paper

and 15 questions) =1/12.

The answer can be found more easily as follows.

P(easy paper

and 15 questions) = P(easy paper) × P(15 questions) = 1/2 × 1/6 = 1/12.

The number of questions has no effect on, nor is it affected by whether it is an easy or difficult paper.

Independent events are events where the outcome of one event in no way affects the outcome of the other events.

2.6.1 Example: Independent events

A die is thrown and a coin is tossed simultaneously. What is the probability of throwing a 5 and getting heads on the

coin?

Important!

T F

RWAR

T F

RWAR

Part C Probability ⏐ 6: Probability 173

Solution

The probability of throwing a 5 on a die is 1/6

The probability of a tossed coin coming up heads is 1/2

The probability of throwing a 5 and getting heads on a coin is 1/2

× 1/6 = 1/12

2.7 The general rule of addition

The general rule of addition for two events, A and B, which are not mutually exclusive, is as follows.

P(A or B) = P (A ∪ B) = P(A) + P(B) – P(A and B)

The three examinations you still have to sit are placed face down in a line in front of you at the final examination

sitting. There is an easy astrophysics paper, a difficult geography of the moon paper and a difficult computer art

paper. Without turning over any of the papers you are told to choose one of them. What is the probability that the

first paper that you select is difficult

or is the geography of the moon paper?

Let us think about this carefully.

There are two difficult papers, so P(difficult) = 2/3

There is one geography of the moon paper, so P(geography of the moon) = 1/3

If we use the OR law and add the two probabilities then we will have double counted the difficult geography of the

moon paper. It is included in the set of difficult papers

and in the set of geography of the moon papers. In other

words, we are

not faced with mutually exclusive outcomes because the occurrence of a geography of the moon

paper does not exclude the possibility of the occurrence of a difficult paper. We therefore need to take account of

this

double counting.

P(difficult paper or geography of the moon paper) = P(difficult paper) + P(geography of the moon paper) –

P(difficult paper and geography of the moon paper).

Using the AND law, P(difficult paper or geography of the moon paper) = 2/3 + 1/3 – (1/3) = 2/3.

Since it is

not impossible to have an examination which is difficult and about the geography of the moon, these two

events are not mutually exclusive.

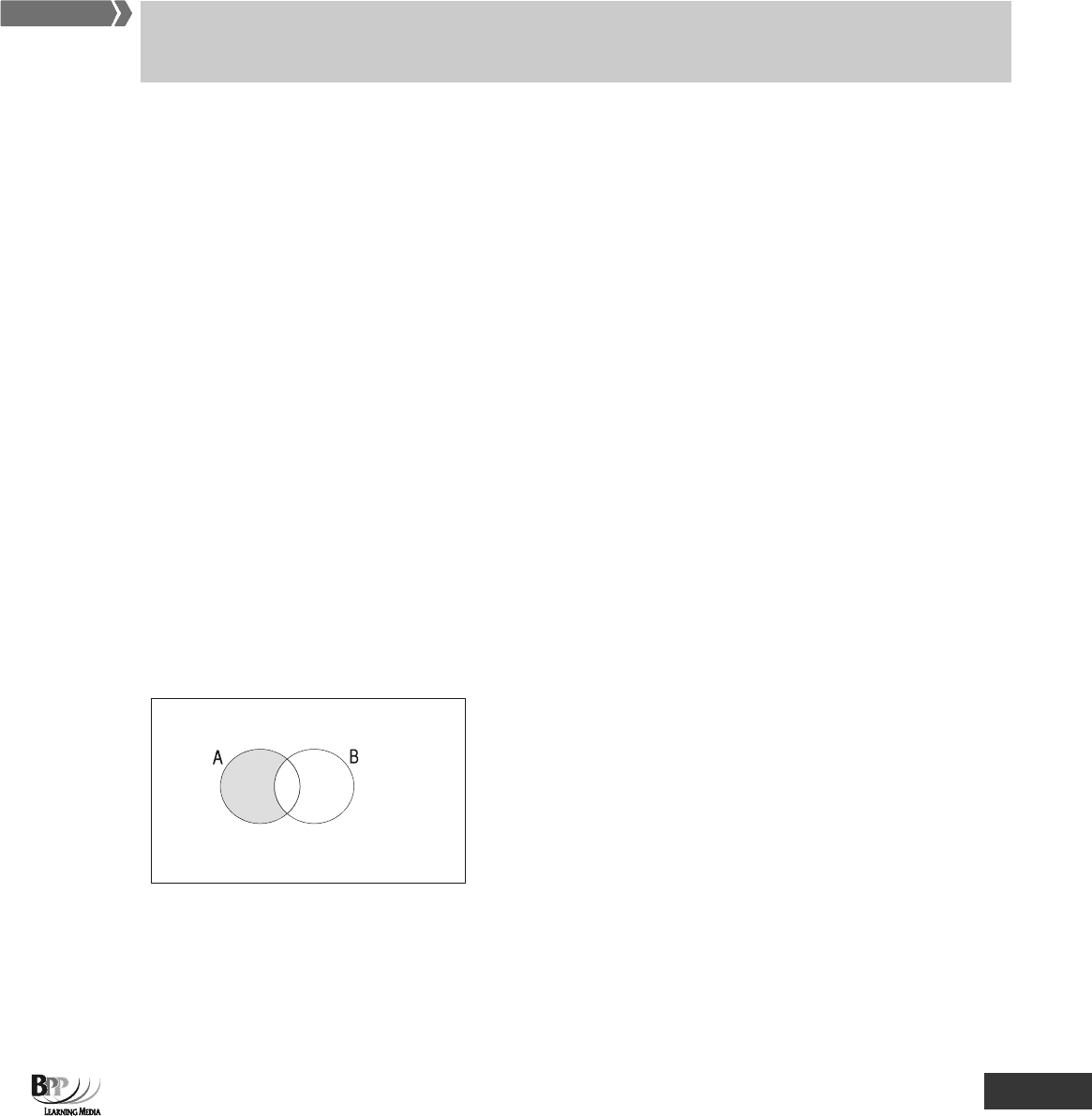
2.7.1 Venn diagram: General rule of addition

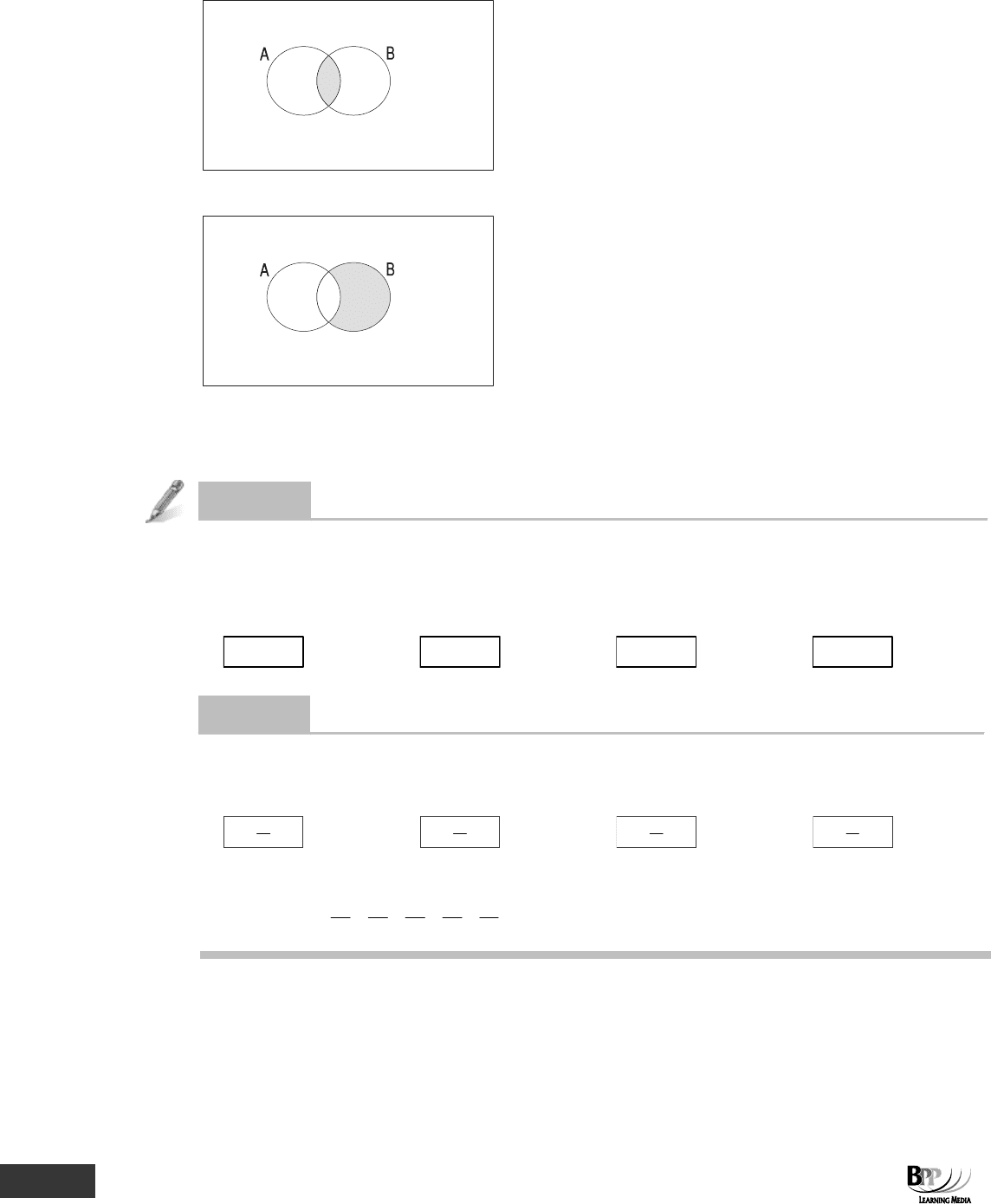
We can show how to calculate P(A ∪ B) from three diagrams.

The shaded area is the probability of A and

not B = P(A) – P(A ∩ B)

T F

RWAR

174 6: Probability ⏐ Part C Probability

The shaded area is the probability of A

and B = P (A ∩ B)

The shaded area is the probability of B and

not A = P (B) – (A ∩ B)

If we add these three sections together we get the formula for the probability of A

or B = P(A) + P(B) – P (A ∩ B)

Question

General rule of addition

If one card is drawn from a normal pack of 52 playing cards, what is the probability of getting an ace or a spade?

Probability

Ace Spade Ace of spades Ace or spade

Answe

Probability

Ace Spade Ace of spades Ace or spade

Working

P(ace or spade) =

==−+

Part C Probability ⏐ 6: Probability 175

2.8 The general rule of multiplication

The general rule of multiplication for two dependent events, A and B is as follows.

P(A and B) = P (A ∩ B) P(A) × P(B/A)

= P(B) × P(A/B)

Computer art is your last examination. Understandably you are very tired and you are uncertain whether you will be

able to stay awake. You believe that there is a 70% chance of your falling asleep if it becomes too hot and stuffy in

the examination hall. It is well known that the air conditioning system serving the examination hall was installed in

the last millennium and is therefore extremely unreliable. There is a 1 in 4 chance of it breaking down during the

examination, thereby causing the temperature in the hall to rise. What is the likelihood that you will drop off?

The scenario above has led us to face what is known as

conditional probability. We can rephrase the information

provided as 'the probability that you will fall asleep, given that it is too hot and stuffy, is equal to 70%' and we can

write this as follows.

P(fall asleep/too hot and stuffy) = 70%.

Dependent or conditional events are events where the outcome of one event depends on the outcome of the

others.

Whether you fall asleep is conditional upon whether the hall becomes too hot and stuffy. The events are not,

therefore, independent and so we cannot use the simple multiplication law. So:

P(it becomes too hot and stuffy and you fall asleep)

= P(too hot and stuffy)

× P(fall asleep/too hot and stuffy)

= 25%

× 70% = 0.25 × 0.7 = 0.175 = 17

When A and B are independent events, then P(B/A) = P(B) since, by definition, the occurrence of B (and therefore

P(B)) does not depend upon the occurrence of A. Similarly P(A/B) = P(A).

2.8.1 Example: Conditional probability

The board of directors of Shuttem Co has warned that there is a 60% probability that a factory will be closed down

unless its workforce improves its productivity. The factory's manager has estimated that the probability of success

in agreeing a productivity deal with the workforce is only 30%.

Required

Determine the likelihood that the factory will be closed.

Solution

If outcome A is the shutdown of the factory and outcome B is the failure to improve productivity:

P(A and B) = P(B)

× P(A/B)

= 0.7

× 0.6

= 0.42

Contingency tables can be useful for dealing with conditional probability.

Important!

T F

RWAR

T F

RWAR

T F

RWAR

176 6: Probability ⏐ Part C Probability

2.8.2 Example: Contingency tables

A cosmetics company has developed a new anti-dandruff shampoo which is being tested on volunteers. Seventy

percent of the volunteers have used the shampoo whereas others have used a normal shampoo, believing it to be

the new anti-dandruff shampoo. Two sevenths of those using the new shampoo showed no improvement whereas

one third of those using the normal shampoo had less dandruff.

Required

A volunteer shows no improvement. What is the probability that he used the normal shampoo?

Solution

The problem is solved by drawing a contingency table, showing 'improvement' and 'no improvement', volunteers

using normal shampoo and volunteers using the new shampoo.

Let us suppose that there were 1,000 volunteers (we could use any number). We could depict the results of the

test on the 1,000 volunteers as follows.

New shampoo

Normal shampoo

Total

Improvement

***500

****100

600

No improvement

**200

200

400

*700

***300

1,000

* 70%

× 1,000 **

× 700

*** Balancing figure ****

× 300

We can now calculate P (shows no improvement)

P(shows no improvement) =

000,1

400

P(used normal shampoo/shows no improvement) =

400

200

Other probabilities are just as easy to calculate.

P (shows improvement/used new shampoo) =

700

500

P (used new shampoo/shows improvement) =

600

500

Question

Independent events

The independent probabilities that the three sections of a management accounting department will encounter one

computer error in a week are respectively 0.1, 0.2 and 0.3. There is never more than one computer error

encountered by any one section in a week. Calculate the probability that there will be the following number of

errors encountered by the management accounting department next week.

(a) At least one computer error

(b) One and only one computer error

Part C Probability ⏐ 6: Probability 177

Answe

(a) The probability of at least one computer error is 1 minus the probability of no error. The probability of no

error is 0.9

× 0.8 × 0.7 = 0.504.

(Since the probability of an error is 0.1, 0.2 and 0.3 in each section, the probability of no error in each

section must be 0.9, 0.8 and 0.7 respectively.)

The probability of at least one error is 1 – 0.504 = 0.496.

(b) Y = yes, N = no

Section 1 Section 2 Section 3

(i) Error? Y N N

(ii) Error? N Y N

(iii) Error? N N Y

Probabilities

(i)

0.1 × 0.8 × 0.7 =

0.056

(ii)

0.9 × 0.2 × 0.7 =

0.126

(iii)

0.9 × 0.8 × 0.3 =

0.216

Total

0.398

The probability of only one error only is 0.398.

Question

General rule of addition

In a student survey, 60% of the students are male and 75% are CIMA candidates. The probability that a student

chosen at random is either female or a CIMA candidate is:

A 0.85

B 0.30

C 0.40

D 1.00

Answe

P(male) = 60% = 0.6

P(female) = 1 – 0.6 = 0.4

P(CIMA candidate) = 75% = 0.75

We need to use the general rule of addition to avoid double counting.

∴ P(female or CIMA candidate) = P(female) + P(CIMA candidate) – P(female and CIMA candidate)

= 0.4 + 0.75 – (0.4

× 0.75)

= 1.15 – 0.3

= 0.85

The correct answer is A.

You should have been able to eliminate options C and D immediately. 0.4 is the probability that the candidate is

female and 1.00 is the probability that something will definitely happen – neither of these options are likely to

correspond to the probability that the candidate is either female or a CIMA candidate.

178 6: Probability ⏐ Part C Probability

3 Expected values

An expected value (or EV) is a weighted average value, based on probabilities. The expected value for a single

event can offer a helpful guide for management decisions.

3.1 How to calculate expected values

If the probability of an outcome of an event is p, then the expected number of times that this outcome will occur in

n events (the expected value) is equal to n

× p.

For example, suppose that the probability that a transistor is defective is 0.02. How many defectives would we

expect to find in a batch of 4,000 transistors?

EV = 4,000

× 0.02

= 80 defectives

Expected value (EV) =

∑np

Where ∑ = sum of

n = outcome

p = probability of outcome occurring

3.2 Example: Expected values

The daily sales of Product T may be as follows.

Units

Probability

1,000

0.2

2,000

0.3

3,000

0.4

4,000

0.1

1.0

Required

Calculate the expected daily sales.

Solution

The EV of daily sales may be calculated by multiplying each possible outcome (volume of daily sales) by the

probability that this outcome will occur.

Probability

Expected value

Units

1,000

0.2

200

2,000

0.3

600

3,000

0.4

1,200

4,000

0.1

400

EV of daily sales

2,400

In the long run the expected value should be approximately the actual average, if the event occurs many times

over. In the example above, we do not expect sales on any one day to equal 2,400 units, but in the long run, over a

large number of days, average sales should equal 2,400 units a day.

T F

RWAR

Formula to

learn

Part C Probability ⏐ 6: Probability 179

3.3 Expected values and single events

The point made in the preceding paragraph is an important one. An expected value can be calculated when the

event will only occur once or twice, but it will not be a true long-run average of what will actually happen, because

there is no long run.

3.4 Example: Expected values and single events

Suppose, for example, that a businessman is trying to decide whether to invest in a project. He estimates that there

are three possible outcomes.

Outcome Profit/(loss) Probability

Success 10,000 0.2

Moderate success 2,000 0.7

Failure (4,000) 0.1

The expected value of profit may be calculated as follows.

Profit/(loss)

Probability

Expected value

10,000

0.2

2,000

0.7

1,400

(4,000)

0.1

(400

)

Expected value of profit

3,000

In this example, the project is a one-off event, and as far as we are aware, it will not be repeated. The actual profit

or loss will be $10,000, $2,000 or $(4,000), and the average value of $3,000 will not actually happen. There is no

long-run average of a single event.

Nevertheless, the expected value can be used to help the manager decide whether or not to invest in the project.

Question

Expected values

A company manufactures and sells product D. The selling price of the product is $6 per unit, and estimates of

demand and variable costs of sales are as follows.

Variable cost

Probability Demand Probability per unit

Units $

0.3 5,000 0.1 3.00

0.6 6,000 0.3 3.50

0.1 8,000 0.5 4.00

0.1 4.50

The unit variable costs do not depend on the volume of sales.

Fixed costs will be $10,000.

Required

Calculate the expected profit.