Chow T.L. Mathematical Methods for Physicists: A Concise Introduction
Подождите немного. Документ загружается.


This is the binomial series: (a)Ifp is a positive integer or zero, the series termi-
nates. (b)Ifp > 0 but is not an integer, the series converges absolutely for
ÿ1 x 1. (c)Ifÿ1 < p < 0, the series converges for ÿ1 < x 1. (d)If
p ÿ1, the series converges for ÿ1 < x < 1.
Problem A1.16
Obtain the Maclaurin series for sin x (the Taylor series for sin x about x 0).
Problem A1.17
Use series methods to obtain the approximate value of
R
1
0
1 ÿ e
ÿx
=xdx.
We can ®nd the power series of functions other than the most common ones
listed above by the successive diÿerentiation process given by Eq. (A1.9). There
are simpler ways to obtain series expansions. We give several useful methods here.
(a) For example to ®nd the series for x 1sin x, we can multiply the series for
sin x by x 1 and collect terms:
x 1sin x x 1 x ÿ
x
3
3!
x
5
5!
ÿ
ý!
x x
2
ÿ
x
3
3!
ÿ
x
4
3!
:
To ®nd the expansion for e
x
cos x, we can mult iply the series for e
x
by the series
for cos x:
e
x
cos x 1 x
x
2
2!
x
3
3!
ý!
1 ÿ
x
2
2!
x
4
4!
ý!
1 x
x
2
2!
x
3
3!
x
4
4!
ÿ
x
2
2!
ÿ
x
3
3!
ÿ
x
4
2!2!
x
4
4!
1 x ÿ
x
3
3
ÿ
x
4
6
:
Note that in the ®rst example we obtained the desired series by multiplication of
a known series by a polynomial; and in the second example we obtained the
desired series by multiplication of two series.
(b) In some cases, we can ®nd the series by division of two series. For
example, to ®nd the series for tan x, we can divide the series for sinx by the series
for cos x:
526
APPENDIX 1 PRELIMINARIES
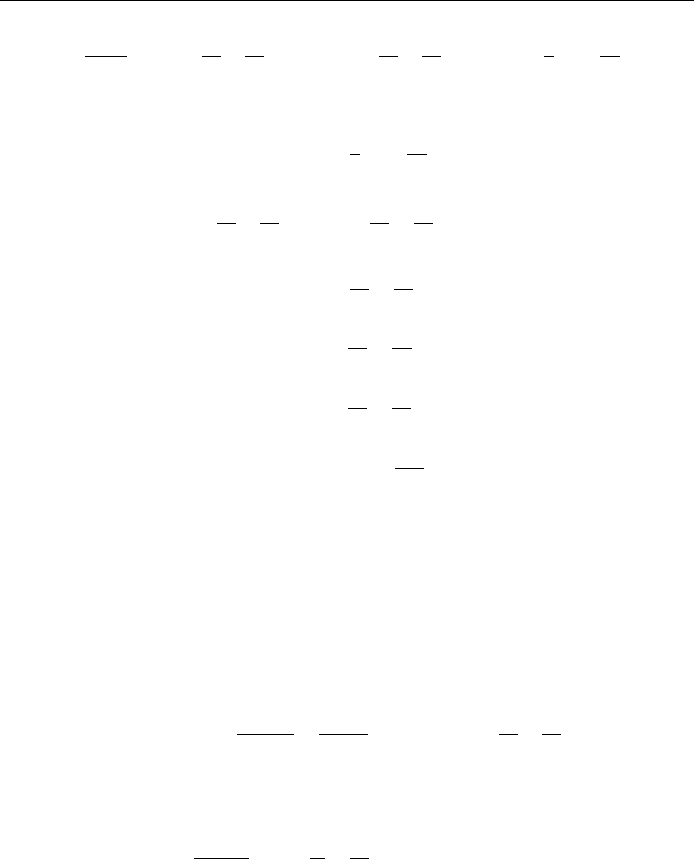
tan x
sin x
cos x
1 ÿ
x
2
2
x
4
4!
x ÿ
x
3
3!
x
5
5!
x
1
3
x
3
2
15
x
5
:
The last step is by long division
x
1
3
x
3
2
15
x
5
1 ÿ
x
2
2!
x
4
4!
x ÿ
x
3
3!
x
5
5!
s
x ÿ
x
3
2!
x
5
4!
x
3
3
ÿ
x
5
30
x
3
3
ÿ
x
5
6
2x
5
15
; etc:
Problem A1.18
Find the series expansion for 1=1 x by long division. Note that the series can
be found by using the binomial series: 1=1 x1 x
ÿ1
.
(c) In some cases, we can obtain a series expansion by substitution of a poly-
nomial or a series for the variable in another series. As an exampl e, let us ®nd the
series for e
ÿx
2
. We can replace x in the series for e
x
by ÿx
2
and obtain
e
ÿx
2
1 ÿ x
2
ÿx
2
2
2!
ÿx
3
3!
1 ÿ x
2
x
4
2!
ÿ
x
6
3!
:
Similarly, to ®nd the series for sin
x
p
=
x
p
we replace x in the series for sin x by
x
p
and obtain
sin
x
p
x
p
1 ÿ
x
3!
x
2
5!
; x > 0:
Problem A1.19
Find the series expansion for e
tan x
.
Problem A1.20
Assuming the power series for e
x
holds for complex numbers, show that
e
ix
cos x i sin x:
527
TAYLOR'S EXPANSION

(d) Find the series for tan
ÿ1
x (arc tan x). We can ®nd the series by the succes-
sive diÿerentiation process. But it is very tedious to ®nd successive derivatives of
tan
ÿ1
x. We can take advantage of the following integration
Z
x
0
dt
1 t
2
tan
ÿ1
t
þ
þ
þ
þ
x
0
tan
ÿ1
x:
We now ®rst write out 1 t
2
ÿ1
as a binomial series and then integrate term by
term:
Z
x
0
dt
1 t
2
Z
x
0
1 ÿ t
2
t
4
ÿ t
6
ÿ
dt t ÿ
t
3
3
t
5
5
ÿ
t
7
7
þ
þ
þ
þ
x
0
:
Thus, we have
tan
ÿ1
x x ÿ
x
3
3
x
5
5
ÿ
x
7
7
:
(e) Find the series for ln x about x 1. We want a series of powers (x ÿ 1)
rather than powers of x. We ®rst write
ln x ln1 x ÿ 1
and then use the series ln 1 x with x replaced by (x ÿ 1):
ln x ln 1 xÿ1 x ÿ 1ÿ
1
2
x ÿ 1
2
1
3
x ÿ 1
3
ÿ
1
4
x ÿ 1
4
:
Problem A1.21
Expand cos x about x 3=2.
Higher derivatives and Leibnitz's formula for nth derivative of a product
Higher deriva tives of a function y f x with respect to x are written as
d
2
y
dx
2
d
dx
dy
dx
;
d
3
y
dx
3
d
dx
d
2
y
dx
2
ý!
; ...;
d
n
y
dx
n
d
dy
d
nÿ1
y
dx
nÿ1
ý!
:
These are sometimes abbreviated to either
f
00
x; f Fx ; ...; f
n
x or D
2
y; D
3
y; ...; D
n
y
where D d=dx.
When higher deriva tives of a product of two functions f x and gx are
required, we can proceed as follows:
DfgfDg gDf
528
APPENDIX 1 PRELIMINARIES
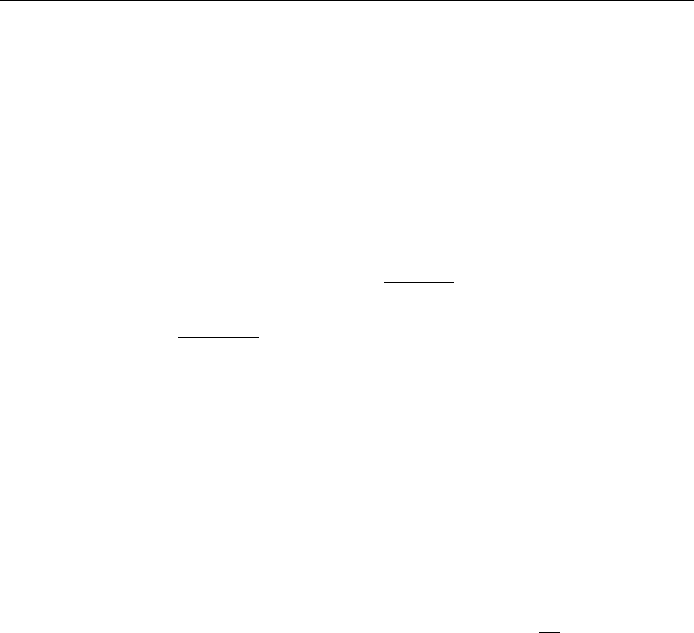
and
D
2
fgDfDg gDf fD
2
g 2Df Dg D
2
g:
Similarly we obtain
D
3
fgfD
3
g 3Df D
2
g 3D
2
f Dg gD
3
f ;
D
4
fgfD
4
g 4Df D
3
g 6D
2
f D
2
g 4D
3
Dg gD
4
g;
and so on. By inspection of these resul ts the following formula (due to Leibnitz)
may be written down for nth derivative of the product fg:
D
n
fgf D
n
gnDf D
nÿ1
g
nn ÿ 1
2!
D
2
f D
nÿ2
g
n!
k!n ÿ k!
D
k
f D
nÿk
gD
n
f g:
Example A1.16
If f 1 ÿ x
2
; g D
2
y, where y is a function of x, say ux, then
D
n
f1 ÿ x
2
D
2
yg1 ÿ x
2
D
n2
y ÿ 2nxD
n1
y ÿ nn ÿ 1 D
n
y:
Leibnitz's formula may also be applied to a diÿerential equation. For example,
y satis®es the diÿerential equation
D
2
y x
2
y sin x:
Then diÿerentiating each term n times we obtain
D
n2
y x
2
D
n
y 2nxD
nÿ1
y nn ÿ 1D
nÿ2
ysin
n
2
x
;
where we have used Leibnitz's formula for the product term x
2
y.
Problem A1.22
Using Leibnitz's formula, show that
D
n
x
2
sin xfx
2
ÿ nn ÿ 1gsinx n=2ÿ2nx cosx n=2:
Some important properties of de®nite integrals
Integration is an operation inverse to that of diÿerentiation; and it is a device for
calculating the `area under a curve'. The latter method regards the integral as the
limit of a sum and is due to Riemann. We now list some useful properties of
de®nite integrals.
(1) If in a x b; m f xM, where m and M are constants, then
mb ÿ a
Z
b
a
f xd Mb ÿ a:
529
PROPERTIES OF DEFINITE INTEGRALS

Divide the interval [a, b] into n subintervals by means of the points
x
1
; x
2
; ...; x
nÿ1
chosen arbitrarily. Let
k
be any point in the subinterval
x
kÿ1
k
x
k
, then we have
mx
k
f
k
x
k
Mx
k
; k 1; 2; ...; n;
where x
k
x
k
ÿ x
kÿ1
. Summing from k 1ton and using the fact that
X
n
k1
x
k
x
1
ÿ ax
2
ÿ x
1
b ÿ x
nÿ1
b ÿ a;
it follows that
mb ÿ a
X
n
rk1
f
k
x
k
Mb ÿ a:
Taking the limit as n !1and each x
k
! 0 we have the required result.
(2) If in a x b; f xgx, then
Z
b
a
f xdx
Z
b
a
gxdx:
(3)
Z
b
a
f xdx
þ
þ
þ
þ
þ
þ
þ
þ
Z
b
a
f x
jj
dx if a < b:
From the inequality
a b c
jj
a
jj
b
jj
c
jj
;
where jaj is the absolute value of a real number a, we have
X
n
k1
f
k
x
k
þ
þ
þ
þ
þ
þ
þ
þ
þ
þ
X
n
k1
f
k
x
k
jj
X
n
k1
f
k
jj
x
k
:
Taking the limit as n !1and each x
k
! 0 we have the required result.
(4) The mean value theorem: If f x is continuous in [a, b], we can ®nd a point
in (a, b) such that
Z
b
a
f xdx b ÿ af :
Since f x is continuous in [a, b], we can ®nd constants m and M such that
m f xM. Then by (1) we have
m
1
b ÿ a
Z
b
a
f xdx M:
Since f x is continuous it takes on all values between m and M; in parti-
cular there must be a value such that
f
Z
b
a
f xdx=b ÿ a; a <<b:
The required result follows on multiplying by b ÿ a.
530
APPENDIX 1 PRELIMINARIES

Some useful methods of integration
(1) Changing variables: We use a simple example to illustrate this common
procedure. Consider the integral
I
Z
1
0
e
ÿax
2
dx;
which is equal to =a
1=2
=2: To show this let us write
I
Z
1
0
e
ÿax
2
dx
Z
1
0
e
ÿay
2
dy:
Then
I
2
Z
1
0
e
ÿax
2
dx
Z
1
0
e
ÿay
2
dy
Z
1
0
Z
1
0
e
ÿax
2
y
2
dxdy:
We now rewrite the integral in plane polar coordinates r;:
x
2
y
2
r
2
; dxdy rdrd . Then
I
2
Z
1
0
Z
=2
0
e
ÿar
2
rddr
2
Z
1
0
e
ÿar
2
rdr
2
ÿ
e
ÿar
2
2a
þ
þ
þ
þ
1
0
4a
and
I
Z
1
0
e
ÿax
2
dx =a
1=2
=2:
(2) Integration by parts: Since
d
dx
uvu
dv
dx
v
du
dx
;
where u f x and v gx, it follows that
Z
u
dv
dx
dx uv ÿ
Z
v
du
dx
dx:
This can be a useful formula in evaluating integrals.
Example A1.17
Evaluate I
R
tan
ÿ1
xdx
Solution: Since tan
ÿ1
x can be easily diÿerentiated, we write
I
R
tan
ÿ1
xdx
R
1 tan
ÿ1
xdx and let u tan
ÿ1
x; dv=dx 1. Then
I x tan
ÿ1
x ÿ
Z
xdx
1 x
2
x tan
ÿ1
x ÿ
1
2
log1 x
2
c:
531
SOME USEFUL METHODS OF INTEGRATION

Example A1.18
Show that
Z
1
ÿ1
x
2
e
ÿax
2
dx
1=2
2a
3=2
:
Solution: Let us ®rst consider the integral
I
Z
1
0
e
ÿax
2
dx
`Integration-by-parts' gives
I
Z
c
b
e
ÿax
2
dx e
ÿax
2
x
þ
þ
þ
þ
c
b
2
Z
c
b
ax
2
e
ÿax
2
dx;
from which we obtain
Z
c
b
x
2
e
ÿax
2
dx
1
2a
Z
c
b
e
ÿax
2
dx ÿ e
ÿax
2
x
þ
þ
þ
þ
c
b
:
We let limits b and c become ÿ1 and 1, and thus obtain the desired result.
Problem A1.23
Evaluate I
Z
xe
x
dx ( constant).
(3) Partial fractions: Any rational function Px=Qx, where Px and Qx
are polynomials, with the degree of Px less than that of Qx, can be written as
the sum of rational functions having the form A=ax b
k
,
Ax B=ax
2
bx c
k
, where k 1; 2; 3; ... which can be integrated in
terms of elementary functions.
Example A1.19
3x ÿ 2
4x ÿ 32x 5
3
A
4x ÿ 3
B
2x 5
3
C
2x 5
2
D
2x 5
;
5x
2
ÿ x 2
x
2
2x 4
2
x ÿ 1
Ax B
x
2
2x 4
2
Cx D
x
2
2x 4
E
x ÿ 1
:
Solution: The coecients A, B, C etc., can be determined by clearing the frac-
tions and equating coecients of like powers of x on both sides of the equation.
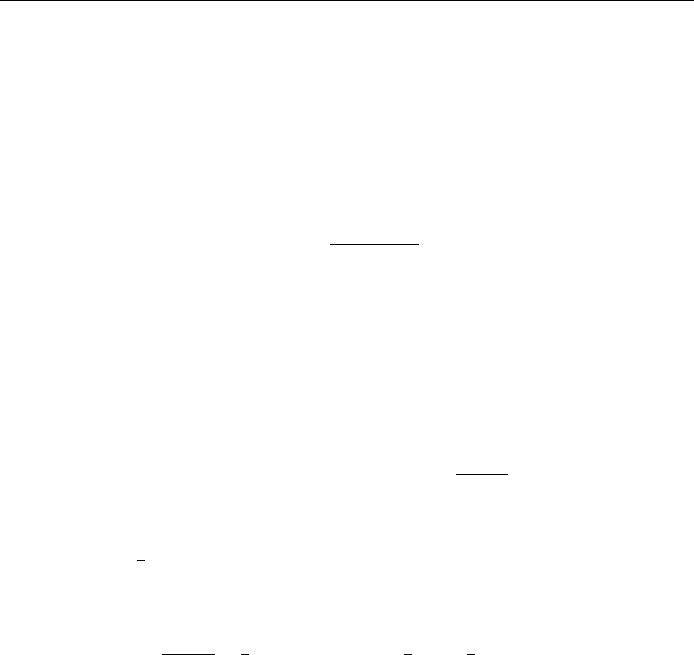
Problem A1.24
Evaluate
I
Z
6 ÿ x
x ÿ 32x 5
dx:
532
APPENDIX 1 PRELIMINARIES
(4) Rational functions of sin x and cos x can always be integrated in terms of
elementary functions by substitution tan x=2u, as shown in the following
example.
Example A1.20
Evaluate
I
Z
dx
5 3cosx
:
Solution: Let tan x=2u, then
sin x=2u=
1 u
2
p
; cos x=21=
1 u
2
p
and
cos x cos
2
x=2ÿsin
2
x=2
1 ÿ u
2
1 u
2
;
also
du
1
2
sec
2
x=2dx or
dx 2 cos
2
x=22du=1 u
2
:
Thus
I
Z
du
u
2
4
1
2
tan
ÿ1
u=2c
1
2
tan
ÿ1
1
2
tan x=2 c:
Reduction formulas
Consider an integral of the form
R
x
n
e
ÿx
dx. Since this depends upon n let us call it
I
n
. Then using integrati on by parts we have
I
n
ÿx
n
e
ÿx
n
Z
x
nÿ1
e
ÿx
dx x
n
e
ÿx
nI
nÿ1
:
The above equation gives I
n
in terms of I
nÿ1
I
nÿ2
; I
nÿ3
, etc.) and is therefore called
a reduction formula.
Problem A1.25
Evaluate I
n
Z
=2
0
sin
n
xdx
Z
=2
0
sin x sin
nÿ1
xdx:
533
REDUCTION FORMULAS

Diÿerentiation of integrals
(1) Inde®nite integ rals: We ®rst consider diÿerentiation of inde®nite integrals. If
f x; is an integrable function of x and is a variable parameter, and if
Z
f x;dx Gx;; A1:11
then we have
@Gx;=@x f x;: A1:12
Furthermore, if f x; is such that
@
2
Gx;
@x@
@
2
Gx;
@@x
;
then we obtain
@
@x
@G x;
@
@
@
@Gx;
@x
@f x;
@
and integrating gives
Z
@f x;
@
dx
@Gx;
@
; A1:13
which is valid provided @f x;=@ is continuous in x as well as .
(2) De®nite integrals: We now extend the above procedure to de®nite integrals:
I
Z
b
a
f x;dx; A1:14
where f x; is an integ rable function of x in the interval a x b,anda and b
are in general continuous and diÿerentiable (at least once) functions of . We now
have a relation sim ilar to Eq. (A1.11):
I
Z
b
a
f x;dx Gb;ÿG a ;A1:15
and, from Eq. (A1.13),
Z
b
a
@f x;
@
dx
@Gb;
@
ÿ
@G a;
@
: A1:16
Diÿerentiating (A1.15) totally
dI
d
@G b;
@b
db
d
@Gb;
@
ÿ
@Ga;
@a
da
d
ÿ
@G a;
@
:
534
APPENDIX 1 PRELIMINARIES

which becomes, with the help of Eqs. (A1.12) and (A1.16),
dI
d
Z
b
a
@f x ;
@
dx f b;
db
d
ÿ f a;
da
d
; A1:17
which is known as Leibnitz's rule for diÿerentiating a de®nite integral. If a and b,
the limits of integ ration, do not depend on , then Eq. (A1.17) reduces to
dI
d
d
d
Z
b
a
f x;dx
Z
b
a
@f x;
@
dx:
Problem A1.26
If I
Z
2
0
sinx=xdx, ®nd dI=d.
Homogeneous functions
A homogeneous function f x
1
; x
2
; ...; x
n
of the kth degree is de®ned by the
relation
f x
1
;x
2
; ...;x
n
k
f x
1
; x
2
; ...; x
n
:
For example, x
3
3x
2
y ÿ y
3
is homogeneous of the third degree in the variables x
and y.
If f x
1
; x
2
; ...; x
n
) is homogeneous of degree k then it is straightforward to
show that
X
n
j1
x
j
@f
@x
j
kf :
This is known as Euler's theorem on homogeneous functions.
Problem A1.27
Show that Euler's theorem on homogeneous functions is true.
Taylor series for functions of two independent variables
The ideas involved in Taylor series for functions of one variable can be general-
ized. For example, consider a function of two variables (x; y). If all the nth partial
derivatives of f x; y are continuous in a closed region and if the n 1)st partial
535
HOMOGENEOUS FUNCTIONS
