Chow T.L. Mathematical Methods for Physicists: A Concise Introduction
Подождите немного. Документ загружается.

correspond respectively to the elements A
0
; B
0
; C
0
; ... of G
0
, then the equation
AB C implies that A
0
B
0
C
0
, etc., and vice versa. Two isomorphic groups
have the same multiplication tables except for the labels attached to the group
elements. Obviously, two isomorphic groups must have the same order.
Groups that are isomorphic and so have the same multiplication table are the
same or identical, from an abstract point of view. That is why the concept of
isomorphism is a key concept to physicists. Diverse groups of ope rators that act
on diverse sets of objects have the same multiplication table; there is only one
abstract group. This is where the value and beauty of the group theoretical
method lie; the same abstract algebraic results may be applied in making predic-
tions about a wide variety physical objects.
The isomorphism of groups is a special instance of homomorphism, which
allows many-to-one correspondence.
Example 12.9
Consider the groups of Problems 12.2 and 12.4. The group G of Problem 12.2
consists of the four elements E 1; A i; B ÿ1; C ÿi with ordinary multi-
plication as the rule of combination. The group multiplication table has the form
shown in Table 12.3. The group G
0
of Problem 12.4 consists of the following four
elements, with matrix multiplication as the rule of combination
E
0
10
01
; A
0
01
ÿ10
; B
0
ÿ10
0 ÿ1
; C
0
0 ÿ1
10
:
It is straightforward to check that the group multiplication table of group G
0
has
the form of Table 12.4. Comparing Tables 12.3 and 12.4 we can see that they have
precisely the same structure. The two groups are therefore isomorphic.
Example 12.10
We stated earlier that diverse groups of operators that act on diverse sets of
objects have the same multiplication table; there is only one abstract group. To
illustrate this, we consider, for simplicity, an abstract group of order two, G
2
: that
436
ELEMENTS OF GROUP THEORY
Table 12.3.
1 i ÿ1 iEABC
11i ÿ1 iEEABC
iiÿ1 ÿi 1orAABCE
ÿ1 ÿ1 ÿi 1 iBBCEA
ÿi ÿi 1 i ÿ1 CCEAB
is, we make no a priori assumption about the signi®cance of the two elements of
our group. One of them must be the identity E, and we call the other X. Thus we
have
E
2
E; EX XE E:
Since each element appears once and only once in each row and column, the
group multiplication table takes the form:
We next consider some groups of operators that are isomorphic to G
2
. First,
consider the following two transformations of three-dimensional space into itself:
(1) the transformation E
0
, which leaves each point in its place, and
(2) the transformation R, which maps the point x; y; z into the point
ÿx; ÿy; ÿz. Evidently, R
2
RR (the transformation R followed by R)
will bring each point back to its original position. Thus we have
E
0
2
E
0
, RE
0
E
0
R RE
0
R; R
2
E
0
; and the group multiplication
table has the same form as G
2
: that is, the group formed by the set of the two
operations E
0
and R is isomorphic to G
2
.
We now associate with the two operations E
0
and R two operators
^
O
E
0
and
^
O
R
,
which act on real- or complex-valued functions of the spati al coordinates x; y; z,
ýx; y; z, with the following eÿects:
^
O
E
0
ýx; y; zýx; y; z;
^
O
R
ýx; y; zýÿx; ÿy; ÿz:
From these we see that
^
O
E
0
2
^
O
E
0
;
^
O
E
0
^
O
R
^
O
R
^
O
E
0
^
O
R
;
^
O
R
2
^
O
R
:
437
ISOMORPHIC GROUPS
Table 12.4.
E
0
A
0
B
0
C
0
E
0
E
0
A
0
B
0
C
0
A
0
A
0
B
0
C
0
E
0
B
0
B
0
C
0
E
0
A
0
C
0
C
0
E
0
E
0
B
0
EX
EEX
XXE

Obviously these two operators form a group that is isomorphic to G
2
. These two
groups (formed by the elements E
0
, R, and the elements
^
O
E
0
and
^
O
R
, respectively)
are the two representations of the abstract group G
2
. These two simple examples
cannot illustrate the value and beauty of the group theoretical method, but they
do serve to illustrate the key concept of isomorphism.
Group of permutations and Cayley's theorem
In Example 12.6 we examined brie¯y the group of permutations of three objects.
We now come back to the general case of n objects (1; 2; ...; n) placed in n boxes
(or places) labeled
1
,
2
; ...;
n
. This group, denoted by S
n
, is called the sym-
metric group on n objects. It is of order n! How do we know? The ®rst object may
be put in any of n boxes, and the second object may then be put in any of n ÿ 1
boxes, and so forth: nn ÿ 1n ÿ 23 2 1 n!:
We now de®ne, following common practice, a permutation symbol P
P
123 n
1
2
3
n
þ!
; 12:1
which shifts the object in box 1 to box
1
, the object in box 2 to box
2
, and so
forth, where
1
2
n
is some arrangement of the numbers 1 ; 2; 3; ...; n. The old
notation in Example 12.6 can now be written as
231
123
231
:
For n objects there are n! permutations or arrangements, each of which may be
written in the form (12.1). Taking a speci®c example of three objects, we have
P
1
123
123
; P
2
123
231
; P
3
123
132
;
P
4
123
213
; P
5
123
321
; P
6
123
312
:
For the product of two permutations P
i
P
j
i; j 1; 2; ...; 6, we ®rst perform the
one on the right, P
j
, and then the one on the left, P
i
. Thus
P
3
P
6
123
132
123
312
123
213
P
4
:
To the reader who has diculty seeing this result, let us explain. Consider the ®rst
column. We ®rst perform P
6
, so that 1 is replaced by 3, we then perform P
3
and 3
438
ELEMENTS OF GROUP THEORY

is replaced by 2. So by the combined action 1 is replaced by 2 and we have the ®rst
column
1
2
:
We leave the other two columns to be completed by the reader.
Each element of a group has an inverse. Thus, for each permutation P
i
there is
P
ÿ1
i
, the inverse of P
i
. We can use the property P
i
P
ÿ1
i
P
1
to ®nd P
ÿ1
i
. Let us ®nd
P
ÿ1
6
:
P
ÿ1
6
312
123
123
231
P
2
:
It is straightforward to check that
P
6
P
ÿ1
6
P
6
P
2
123
312
123
231
123
123
P
1
:
The reader can verify that our group S
3
is generated by the elements P
2
and P
3
,
while P
1
serves as the identity. This means that the other three distinct elements
can be expressed as distinct multiplicative combinations of P
2
and P
3
:
P
4
P
2
2
P
3
; P
5
P
2
P
3
; P
6
P
2
2
:
The symmetric group S
n
plays an impor tant role in the study of ®nite groups.
Every ®nite group of order n is isomorphic to a subgroup of the permutation
group S
n
. This is known as Cayley's theorem. For a proof of this theorem the
interested reader is referred to an advanced text on group theory.
In physics, these permutation groups are of considerable importance in the
quantum mechanics of identical particles, where, if we interchange any two or
more these particles, the resulting con®guration is indistinguishable from the
original one. Various quantities must be invariant under interchange or permuta-
tion of the particles. Details of the consequences of this invariant property may be
found in most ®rst-year graduate textbooks on quantum mechanics that cover the
application of group theory to quantum mechanics.
Subgroups and cosets
A subset of a group G, which is itself a group, is called a subgroup of G. This idea
was introduced earlier. And we also saw that C
3
, a cyclic group of order 3, is a
subgroup of S
3
, a symmetric group of ord er 6. We note that the order of C
3
is a
factor of the order of S
3
. In fact, we will show that, in general,
the order of a subgroup is a factor of the order of the full group
(that is, the group from which the subgroup is derived).
439
SUBGROUPS AND COSETS

This can be proved as follows. Let G be a group of order n with elements g
1
E,
g
2
; ...; g
n
: Let H, of order m, be a subgroup of G with elements h
1
E,
h
2
; ...; h
m
. Now form the set gh
k
0 k m, where g is any element of G not
in H. This collection of elements is called the left-coset of H with respect to g (the
left-coset, because g is at the left of h
k
).
If such an element g does not exist, then H G, and the theorem holds trivially.
If g does exist, than the elements gh
k
are all diÿerent. Otherwise, we would have
gh
k
gh
`
,orh
k
h
`
, which contradicts our assumption that H is a group.
Moreover, the elements gh
k
are not elements of H. Otherwise, gh
k
h
j
, and we
have
g h
j
=h
k
:
This implies that g is an element of H, which co ntradicts our assumption that g
does not belong to H.
This left-coset of H does not form a group because it does not contain the
identity element (g
1
h
1
E. If it did form a group, it would require for some h
j
such that gh
j
E or, equivalently, g h
ÿ1
j
. This requires g to be an element of H.
Again this is contrary to assum ption that g does not belong to H.
Now every element g in G but not in H belongs to some coset gH. Thus G is a
union of H and a number of non-overlapping cosets, each having m diÿerent
elements. The order of G is therefore divisible by m. This proves that the order
of a subgroup is a factor of the order of the full group. The ratio n/m is the index
of H in G.
It is straightforward to prove that a group of order p, where p is a prime
number, has no subgroup. It could be a cyclic group generated by an element a
of period p.
Conjugate classes and invariant subgroups
Another way of dividing a group into subsets is to use the concept of classes. Let
a, b, and u be any three elements of a group, and if
b u
ÿ1
au;
b is said to be the transform of a by the element u; a and b are conjugate (or
equivalent) to each other. It is straightforward to prove that conjugate has the
following three properties:
(1) Every element is conjugate with itself (re¯exivity). Allowing u to be the
identity element E, then we have a E
ÿ1
aE:
(2) If a is conjugate to b, then b is conjugate to a (symmetry). If a u
ÿ1
bu, then
b uau
ÿ1
u
ÿ1
ÿ1
au
ÿ1
, where u
ÿ1
is an element of G if u is.
440
ELEMENTS OF GROUP THEORY

(3) If a is conjugate with both b and c, then b and c are conjugate with each
other (transitivity). If a u
ÿ1
bu and b v
ÿ1
cv, then a u
ÿ1
v
ÿ1
cvu
vu
ÿ1
cvu, where u and v belong to G so that vu is also an elem ent of G.
We now divide our group up into subsets, such that all elements in any subset
are conjugate to each other. These subsets are called classes of our group.
Example 12.11
The symmetric group S
3
has the following six distinct elements:
P
1
E; P
2
; P
3
; P
4
P
2
2
P
3
; P
5
P
2
P
3
; P
6
P
2
2
;
which can be separat ed into three conjugate classes:
fP
1
g; fP
2
; P
6
g; fP
3
; P
4
; P
5
g:
We now state some simple facts about classes without proofs:
(a) The identity elem ent always forms a class by itself.
(b) Each element of an Abelian group forms a class by itself.
(c) All elements of a class have the same period.
Starting from a subgroup H of a group G, we can form a set of elements uh
ÿ1
u
for each u belong to G. This set of elements can be seen to be itself a group. It is a
subgroup of G and is isomorphic to H. It is said to be a conjugate subgroup to H
in G. It may happen, for some subgroup H, that for all u belonging to G, the sets
H and uhu
ÿ1
are identi cal. H is then an invariant or self-conjugate subgroup of G.
Example 12.12
Let us revisit S
3
of Example 12.11, taking it as our group G S
3
. Consider the
subgroup H C
3
fP
1
; P
2
; P
6
g: The following relation holds
P
2
P
1
P
2
P
6
0
B
B
@
1
C
C
A
P
ÿ1
2
P
1
P
2
P
1
P
2
P
6
0
B
B
@
1
C
C
A
P
ÿ1
2
P
ÿ1
1
P
1
P
2
P
1
P
2
P
6
0
B
B
@
1
C
C
A
P
1
P
2
ÿ1
P
2
1
P
2
P
1
P
2
P
6
0
B
B
@
1
C
C
A
P
2
1
P
2
ÿ1
P
1
P
6
P
2
0
B
B
@
1
C
C
A
:
Hence H C
3
fP
1
; P
2
; P
6
g is an invariant subgroup of S
3
.
441
CONJUGATE CLASSES AND INVARIANT SUBGROUPS

Group representations
In previous sections we have seen some examples of groups which are isomorphic
with matrix groups. Physicists have found that the representation of group
elements by matrices is a very powerful technique. It is beyond the scope of
this text to make a full study of the representation of groups; in this section we
shall make a brief study of this important subject of the matrix representations of
groups.
If to every element of a group G, g
1
; g
2
; g
3
; ...; we can associate a non-singular
square matrix Dg
1
; Dg
2
; Dg
3
; ...; in such a way that
g
i
g
j
g
k
implies Dg
i
Dg
j
Dg
k
; 12:2
then these matrices themselves form a group G
0
, which is either isomorph ic or
homomorphic to G. The set of such non-singular square matrices is called a
representation of group G. If the matrices are n n , we have an n-dimensional
representation; that is, the order of the matrix is the dimension (or ord er) of the
representation D
n
. One trivial example of such a representation is the unit matrix
associated with every element of the group. As shown in Example 12.9, the four
matrices of Problem 12.4 form a two-dimensiona l representation of the group G
of Problem 12.2.
If there is one-to-one correspondence between each element of G and the matrix
representation group G
0
, the two groups are isomorphic, and the repres entation is
said to be faithful (or true). If one matrix D represents more than one group
element of G, the group G is homomorphic to the matrix representation group
G
0
and the representation is said to be unfaithful.
Now suppose a representation of a group G has been found which consists of
matrices D Dg
1
; Dg
2
; Dg
3
; ...; Dg
p
, each matrix being of dimension
n. We can form another representation D
0
by a similarity transformation
D
0
gS
ÿ1
DgS; 12:3
S being a non-singular matrix, then
D
0
g
i
D
0
g
j
S
ÿ1
Dg
i
SS
ÿ1
Dg
j
S
S
ÿ1
Dg
i
Dg
j
S
S
ÿ1
Dg
i
g
j
S
D
0
g
i
g
j
:
In general, representations related in this way by a similarity transformation are
regarded as being equivalent. However, the forms of the individual matrices in the
two equivalent representations will be quite diÿerent. With this freedom in the
442
ELEMENTS OF GROUP THEORY

choice of the forms of the matrices it is important to look for some quantity that is
an invariant for a given transformation. This is found in considering the traces of
the matrices of the representation group because the trace of a matrix is
invariant under a similarity transformation. It is often possible to bring, by a
similarity transformation, each matrix in the repres entation group into a diagonal
form
S
ÿ1
DS
D
1
0
0 D
2
þ!
; 12:4
where D
1
is of order m; m < n and D
2
is of order n ÿ m. Under these conditions,
the original representation is said to be reducible to D
1
and D
2
. We may write
this result as
D D
1
D
2
12:5
and say that D has been decomposed into the two smaller representation D
1
and
D
2
; D is often called the direct sum of D
1
and D
2
.
A representation Dg is called irreducible if it is not of the form (12.4)
and cannot be put into this form by a similarity transformation. Irreducible
representations are the simplest representations, all others may be built up from
them, that is, they play the role of `building blo cks' for the study of group
representation.
In general, a given group has many representations, and it is always possible to
®nd a unitary representation ± one whose matrices are unitary. Unitary matrices
can be diagonalized, an d the eigenvalues can serve for the description or classi-
®cation of quantum states. Hence unitary representations play an especially
important role in quantum mechanics.
The task of ®nding all the irreducible representations of a group is usually very
laborious. Fortunately, for most physical applications, it is sucient to know only
the traces of the matrices forming the representation, for the trace of a matrix is
invariant under a similarity transformation. Thus, the trace can be used to iden-
tify or characterize our repres entation, and so it is called the character in group
theory. A further simpli®cation is provided by the fact that the character of every
element in a class is identical, since elements in the same class are related to each
other by a similarity transformation. If we know all the characters of one element
from every class of the group, we have all of the information concerning the group
that is usually needed. Hence characters play an important part in the theory of
group representations. However, this topic and others related to whether a given
representation of a group can be reduced to one of smaller dimensions are beyond
the scope of this book. There are several important theorems of representation
theory, which we now state without proof.
443
GROUP REPRESENTATIONS

(1) A matrix that commutes with all matrices of an irreducible representation of
a group is a multiple of the unit matrix (perhaps null). That is, if matrix A
commutes with Dg which is irreducible,
DgA ADg
for all g in our group, then A is a mult iple of the unit matrix.
(2) A representation of a group is irreducible if and only if the only matrices to
commute with all matrices are multiple of the unit matrix.
Both theorem s (1) and (2) are corollaries of Schur's lemma.
(3) Schur's lemma: Let D
1
and D
2
be two irreducible representations of (a
group G) dimensionality n and n
0
, if there exists a matrix A such that
AD
1
gD
2
gA for all g in the group G
then for n 6 n
0
, A 0; for n n
0
, either A 0orA is a non-singular matrix
and D
1
and D
2
are equivalent representations under the similarity trans-
formation generated by A.
(4) Orthogonality theorem: If G is a group of order h and D
1
and D
2
are any
two inequivalent irreducible (unitary) representations, of dimensions d
1
and
d
2
, respectively, then
X
g
D
i
þ
g*D
j
ÿ
g
h
d
1
ij
ÿ
þ
;
where D
i
g is a matrix, and D
i
þ
g is a typical matrix element. The sum
runs over all g in G.
Some special groups
Many physical systems possess symmetry properties that always lead to certain
quantity being invariant. For example, translational symmetry (or spatial homo-
geneity) leads to the conservation of linear momentum for a closed system, and
rotational symmetry (or isotropy of space) leads to the conservation of angular
momentum. Group theory is most appropriate for the study of symmetry. In this
section we consider the geometrical symmetries. This provides more illustrations
of the group concepts and leads to some special groups.
Let us ®rst review some symm etry operations. A plane of symmetry is a plane in
the system such that each point on one side of the plane is the mirror image of a
corresponding point on the other side. If the system takes up an identical position on
rotation through a certain angle about an axis, that axis is called an axis of sym-
metry. A center of inversion is a point such that the system is invariant under the
operation r !ÿr, where r is the position vector of any point in the system referred to
the inversion center. If the system takes up an identical position after a rotation
followed by an inversion, the system possesses a rotation±inversion center.
444
ELEMENTS OF GROUP THEORY
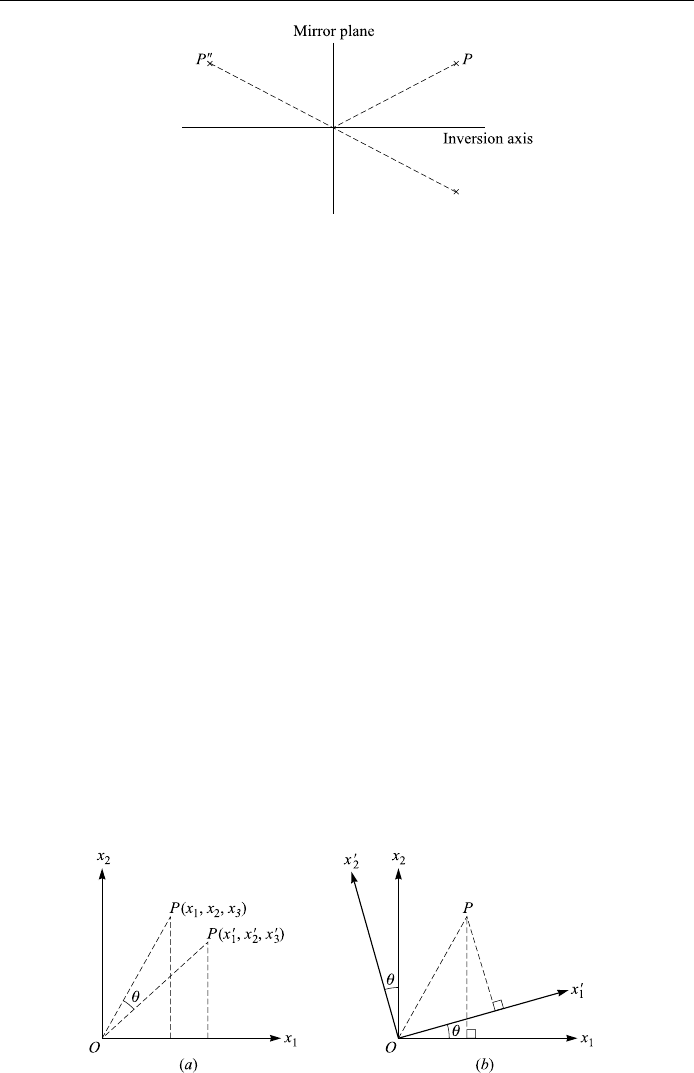
Some symmetry operations are equivalent. As shown in Fig. 12.2, a two-fold
inversion axis is equivalent to a mirror plane perpendicular to the axis.
There are two diÿerent ways of looking at a rotation, as shown in Fig. 12.3.
According to the so-called active view, the system (the body) undergoes a rotation
through an angle , say, in the clockwise direction about the x
3
-axis. In the passive
view, this is equivalent to a rotation of the coordinate system through the same
angle but in the counterclockwise sense. The relation between the new and old
coordinates of any point in the body is the same in both cases:
x
0
1
x
1
cos x
2
sin ;
x
0
2
ÿx
1
sin x
2
cos ;
x
0
3
x
3
;
9
>
>
=
>
>
;
12:6
where the prime quantities represent the new coordinates.
A general rotation, re¯ection, or inversion can be represented by a linear
transformation of the form
x
0
1
11
x
1
12
x
2
13
x
3
;
x
0
2
21
x
1
22
x
2
23
x
3
;
x
0
3
31
x
1
32
x
2
33
x
3
:
9
>
>
=
>
>
;
12:7
445
SOME SPECIAL GROUPS
Figure 12.2.
Figure 12.3. (a) Active view of rotation; (b) passive view of rotation.
