Celletti A. Stability and Chaos in Celestial Mechanics
Подождите немного. Документ загружается.

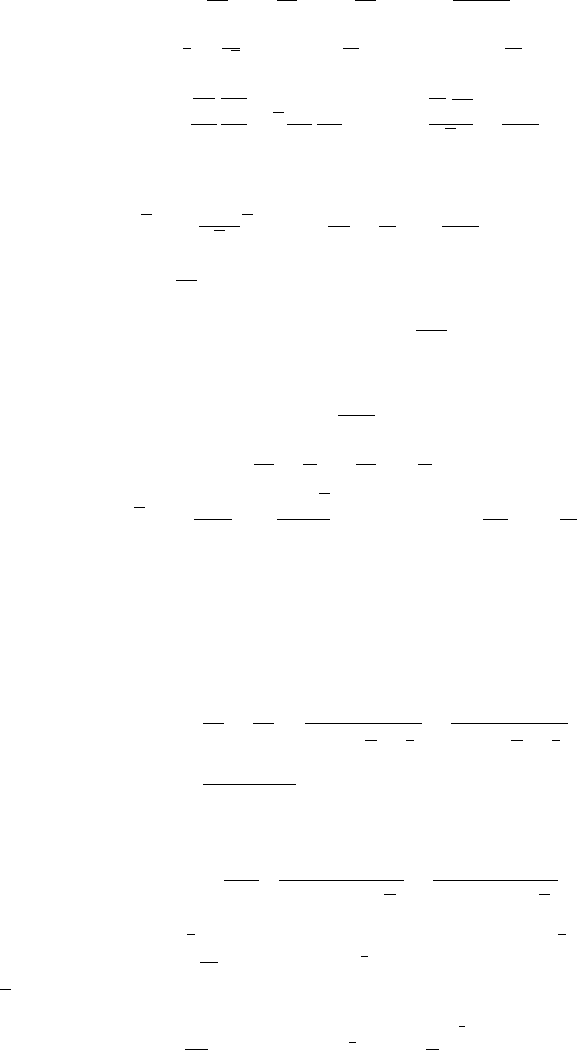
224 10 Regularization theory
w
+2i
w
˙τ
+ w
¨τ
˙τ
2
+ w
2
h
h
= ∇
w
U
1
|h
|
2
˙τ
2
. (10.19)
From the relations ˙τ =
1
g
=
1
kk
and ¨τ = −
˙g
g
2
, it follows that
¨τ
˙τ
2
= −˙g. Taking into
account the expression
−˙g = −
k
dk
dw
dw
dτ
+
k
dk
dw
dw
dτ
˙τ = −
k
w
k
+
k
w
k
,
it follows that (10.19) takes the form
w
+2ikkw
−
|w
|
2
k
k
+ w
2
h
h
−
k
k
=
|k|
4
|h
|
2
∇
w
U. (10.20)
Setting
˜
U(q)=U(q) −
C
J
2
where C
J
denotes the Jacobi integral, by (4.15) one has
|˙q|
2
=2
˜
U(q)=|h
|
2
|w
|
2
1
|k|
4
,
namely
|w
|
2
=2
|k|
4
|h
|
2
˜
U.
Using (10.20) and the relation (
h
h
−
k
k
)=
d
dw
(log
h
k
), one obtains
w
+2ikkw
=
|k|
4
|h
|
2
2
˜
U
d log
k
dw
+ ∇
w
U
−|w
2
|
d
dw
log
h
k
.
Choosing k = h
, one obtains the equation
w
+2i|h
|
2
w
= ∇
w
|h
|
2
˜
U
.
Next we determine α and β by requiring that h regularizes both singularities and
by imposing that the transformation leaves P
1
, P
3
invariant. Due to the expressions
U
c
(w)=
μ
1
r
1
+
μ
3
r
3
=
μ
1
|αw +
β
w
+
1
2
|
+
μ
3
|αw +
β
w
−
1
2
|
|h
(w)|
2
=
|αw
2
− β|
2
|w|
4
,
it follows that
U
c
(w)|h
(w)|
2
=
1
|w|
3
μ
1
|αw
2
− β|
2
|αw
2
+ β +
w
2
|
+
μ
3
|αw
2
− β|
2
|αw
2
+ β −
w
2
|
.
The singularity at q =
1
2
corresponds to the roots of αw
2
+ β −
1
2
w =0,which
give the positions w
1,2
=
1
4α
(1 ± (1 − 16αβ)
1
2
). Since the root of the numerator is
±(
β
α
)
1/2
,werequirethat
1
4α
(1 ± (1 − 16αβ)
1
2
)=±
β
α
1
2
. (10.21)

10.3 The Birkhoff regularization 225
Equation (10.21) is satisfied provided that 16αβ = 1, which gives w
1,2
=
1
4α
.Ifwe
require that P
3
is located at (
1
2
, 0), then we find α =
1
2
and β =
1
8
. The singularity
at P
1
of the first term of U
c
(w) can be removed by applying the same procedure,
which provides the same values for α and β.Theterm
1
|w|
3
is singular for w =0,
which corresponds to the non–realistic solution of q tending to infinity. In summary,
the Birkhoff transformation is given by q =
1
2
(w +
1
4w
), which is equivalent to
q
1
=
1
2
w
1
+
w
1
4(w
2
1
+ w
2
2
)
q
2
=
1
2
w
2
−
w
2
4(w
2
1
+ w
2
2
)
.
10.3.1 The B
3
regularization
Consider the restricted, circular, spatial three–body problem, where it is assumed
that the primaries P
1
, P
3
move on circular orbits on the same plane, while the
small body P
2
is allowed to move in the space. The B
3
regularization allows us
to remove in the spatial case both singularities with the primaries. We introduce
a synodic reference frame rotating with the angular velocity of the primaries. Let
(q
1
,q
2
,q
3
) be the coordinates of P
2
. The equations of motion are given by
¨q
1
− 2˙q
2
=
˜
Ω
q
1
¨q
2
+2˙q
1
=
˜
Ω
q
2
¨q
3
+ q
3
=
˜
Ω
q
3
with
˜
Ω(q
1
,q
2
,q
3
)=
1
2
(q
2
1
+ q
2
2
+ q
2
3
)+
μ
1
r
1
+
μ
3
r
3
and r
1
=
(q
1
+ μ
3
)
2
+ q
2
2
+ q
2
3
, r
3
=
(q
1
− μ
1
)
2
+ q
2
2
+ q
2
3
. In the spatial case,
the Hamiltonian in the synodic reference frame is given by
H(p
1
,p
2
,p
3
,q
1
,q
2
,q
3
)=
1
2
(p
2
1
+ p
2
2
+ p
2
3
)+q
2
p
1
− q
1
p
2
−
μ
1
r
1
−
μ
3
r
3
.
The Birkhoff regularizing transformation in the spatial case can be obtained as
follows. Let us define the coordinate’s transformation q
1
=
1
2
˜q
1
+(
1
2
− μ), q
2
=˜q
2
,
q
3
=˜q
3
, so that the primaries are located at (−1, 0, 0) and (1, 0, 0). Then, we
implement a transformation by reciprocal radii with center at (1, 0, 0) and radius
equal to
√
2:
ˆq
1
=1+
2(˜q
1
− 1)
(˜q
1
− 1)
2
+˜q
2
2
+˜q
2
3
ˆq
2
=
2˜q
2
(˜q
1
− 1)
2
+˜q
2
2
+˜q
2
3
ˆq
3
=
2˜q
3
(˜q
1
− 1)
2
+˜q
2
2
+˜q
2
3
.
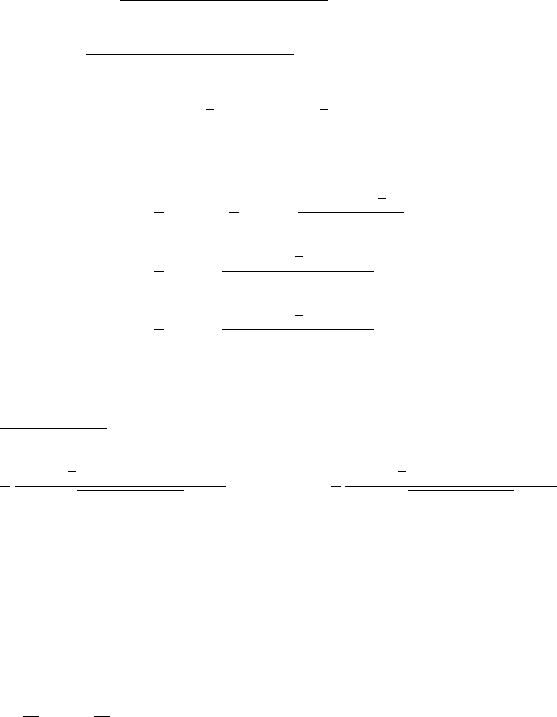
226 10 Regularization theory
This transformation places P
1
at the origin, while P
3
is ejected at infinity. A KS–
transformation is implemented to regularize both singularities, at the origin and
at infinity. To this end, a four–dimensional parametric space is introduced with
coordinates (u
1
,u
2
,u
3
,u
4
) such that
ˆq
1
= u
2
1
− u
2
2
− u
2
3
+ u
2
4
ˆq
2
=2(u
1
u
2
− u
3
u
4
)
ˆq
3
=2(u
1
u
3
+ u
2
u
4
) .
A new transformation by reciprocal radii is implemented, which brings P
1
at
(−1, 0, 0) and P
3
at (1, 0, 0):
u
1
=1+
2(v
1
− 1)
(v
1
− 1)
2
+ v
2
2
+ v
2
3
+ v
2
4
u
k
=
2v
k
(v
1
− 1)
2
+ v
2
2
+ v
2
3
+ v
2
4
,k=2, 3, 4 .
Finally we place P
1
and P
3
at (−
1
2
, 0, 0) and (
1
2
, 0, 0), respectively. The relation
between the physical plane (q
1
,q
2
,q
3
) and the parametric plane (v
1
,v
2
,v
3
,v
4
)is
given by
q
1
=
1
2
− μ +
1
2
v
1
+
v
1
(v
2
4
+
1
4
)
v
2
1
+ v
2
2
+ v
2
3
q
2
=
1
2
v
2
+
v
2
(v
2
4
−
1
4
) − v
3
v
4
v
2
1
+ v
2
2
+ v
2
3
q
3
=
1
2
v
3
+
v
3
(v
2
4
−
1
4
)+v
2
v
4
v
2
1
+ v
2
2
+ v
2
3
.
The fictitious time s is defined through dt = Dds,where
D =
r
1
r
3
v
2
1
+ v
2
2
+ v
2
3
r
1
=
1
2
(v
1
+
1
2
)
2
+ v
2
2
+ v
2
3
+ v
2
4
v
2
1
+ v
2
2
+ v
2
3
,r
3
=
1
2
(v
1
−
1
2
)
2
+ v
2
2
+ v
2
3
+ v
2
4
v
2
1
+ v
2
2
+ v
2
3
.
The equations of motion with respect to the fictitious time are given by
Dq
1
− D
q
1
− 2D
2
q
2
= D
3
˜
Ω
q
1
Dq
2
− D
q
2
+2D
2
q
1
= D
3
˜
Ω
q
2
Dq
3
− D
q
3
= D
3
˜
Ω
q
3
. (10.22)
The singularities appearing in (10.22) through the functions
˜
Ω
q
1
,
˜
Ω
q
2
,
˜
Ω
q
3
are due
to the terms
1
r
3
1
and
1
r
3
3
.SinceD
3
is proportional to r
3
1
r
3
3
, the contributions of
D
3
˜
Ω
q
1
, D
3
˜
Ω
q
2
, D
3
˜
Ω
q
3
do not contain singularities at the attracting centers. We
conclude by remarking that the origin is still a singular point, but it corresponds
to place P
3
at infinity.

A Basics of Hamiltonian dynamics
A.1 The Hamiltonian setting
Consider a mechanical system with n degrees of freedom
1
;letT = T (˙q)bethe
kinetic energy and let V = V (q
) be the potential energy, where ˙q ∈ R
n
, q ∈ R
n
.
The corresponding Lagrangian function is defined as
L(˙q
,q) ≡ T (˙q) − V (q) .
Let us introduce the momenta conjugated to the coordinates through the expression
p
≡
∂L(˙q
,q)
∂ ˙q
. (A.1)
From the Lagrange equations
d
dt
∂L(˙q
,q)
∂ ˙q
=
∂L(˙q
,q)
∂q
,
one obtains
˙p
=
∂L(˙q
,q)
∂q
.
Therefore it follows that
dL =
∂L
∂ ˙q
d˙q +
∂L
∂q
dq = pd˙q +˙pdq = d(p ˙q) − ˙qdp +˙pdq ,
namely
d(p
˙q −L)=−˙pdq +˙qdp . (A.2)
Let us introduce the Hamiltonian function as
H(p
,q) ≡ p ˙q −L(˙q,q) ,
where the variables ˙q
are intended to be expressed in terms of p and q,byinverting
the relation (A.1). From (A.2) one obtains:
dH(p
,q)=−˙pdq +˙qdp ;
1
The number of degrees of freedom is the minimum number of independent coordinates
which are necessary to describe the mechanical system.

228 A Basics of Hamiltonian dynamics
being
dH(p
,q)=
∂H
∂p
dp +
∂H
∂q
dq ,
one finds the equations
˙q
=
∂H(p
,q)
∂p
˙p = −
∂H(p
,q)
∂q
. (A.3)
The equations (A.3) are called Hamilton’s equations. While in the Lagrangian case
one needs to solve a differential equation of the second order, in the Hamiltonian
setting one is led to find the solution of two differential equations of the first order.
In terms of the components of the vectors p
and q, Hamilton’s equations are written
as
˙q
i
=
∂H(p
,q)
∂p
i
˙p
i
= −
∂H(p
,q)
∂q
i
,i=1,...,n .
Example. Given the Lagrangian function
L(˙q, q)=
1
2
˙q
2
+ q ˙q +3q
2
,
the corresponding Hamiltonian function and the solution of Hamilton’s equations
are found as follows.
The momentum conjugated to q is
p =
∂L
∂ ˙q
=˙q + q,
which yields
˙q = p − q.
Therefore
H(p, q)=p ˙q −L
=
1
2
p
2
− pq −
5
2
q
2
.
The corresponding Hamilton’s equations are
˙p = −
∂H
∂q
= p +5q
˙q =
∂H
∂p
= p − q.
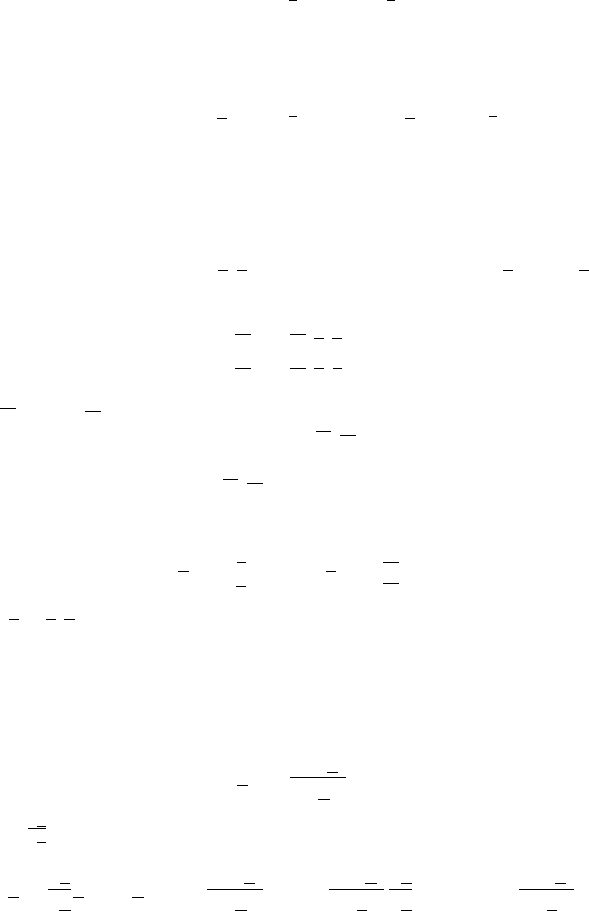
A.2 Canonical transformations 229
Differentiating the second equation with respect to time one has
¨q =˙p − ˙q =6q,
namely
¨q − 6q =0,
whose solution is given by
q(t)=A
1
e
√
6t
+ A
2
e
−
√
6t
,
where A
1
and A
2
are arbitrary constants depending on the initial data. From
p = q +˙q one finds the solution for the momentum:
p(t)=
A
1
+
√
6A
1
e
√
6t
+
A
2
−
√
6A
2
e
−
√
6t
.
A.2 Canonical transformations
Given the Hamiltonian H = H(p,q)withn degrees of freedom (p ∈ R
n
, q ∈ R
n
),
let us consider the coordinate transformation
P
= P (p,q)
Q
= Q(p,q) , (A.4)
where P
∈ R
n
, Q ∈ R
n
. The coordinate change (A.4) is said to be canonical, if
the equations of motion in the variables (P
,Q) keep the Hamiltonian structure,
namely the transformed variables satisfy Hamilton’s equations with respect to a
new Hamiltonian, say H
1
= H
1
(P ,Q).
Let us derive the conditions under which the transformation (A.4) is canonical. We
introduce the notation
x
=
q
p
,z
=
Q
P
and let z
= z(x) be the transformation (A.4). Set
J ≡
0 I
n
−I
n
0
,
where I
n
is the n–dimensional identity matrix; Hamilton’s equations (A.3) can be
written as
˙x
= J
∂H(x
)
∂x
.
Let M =
∂z
∂x
; then, the transformed equations are
˙z
=
∂z
∂x
˙x = M ˙x = MJ
∂H(x
)
∂x
= MJ
∂H(x
)
∂z
∂z
∂x
= MJM
T
∂H(x)
∂z
.

230 A Basics of Hamiltonian dynamics
Therefore, the canonicity condition is equivalent to require that the following rela-
tion is satisfied:
MJM
T
= J ; (A.5)
equation (A.5) implies that the matrix M is symplectic. In that case Hamilton’s
equations with respect to the new variables z
hold, whenever the new Hamiltonian
is defined through the expression H
1
(z)=H(x(z)).
A canonicity criterion is obtained through the introduction of the so–called
Poisson brackets. Let us consider the functions f = f (p
,q), g = g(p,q); the Poisson
bracket between f and g is defined as the quantity
{f,g} =
n
k=1
∂f
∂q
k
∂g
∂p
k
−
∂f
∂p
k
∂g
∂q
k
.
A direct computation shows that the condition (A.5) is equivalent to the following
condition [5]. The transformation (A.4) is canonical if the following relations hold:
{Q
i
,Q
j
} = {P
i
,P
j
} =0, {Q
i
,P
j
} = δ
ij
,i,j=1,...,n .
In the one–dimensional case (n =1)itsufficestoverifythat
{Q, P } =1,
since {Q, Q} and {P, P} are identically zero.
Let us introduce the generating function of a canonical transformation as fol-
lows. Consider the general case of a time–dependent canonical transformation
Q
= Q(q,p,t)
P
= P (q,p,t) . (A.6)
The generating function, that we extend to encompass the time–dependent trans-
formation (A.6), is a function of the form
F = F (q
,Q,t) ,
such that the following transformation rules hold:
p
=
∂F
∂q
P = −
∂F
∂Q
.
If H
1
= H
1
(P ,Q,t) is the Hamiltonian in the new set of variables, then
H
1
(P ,Q,t)=H(p,q,t)+
∂F
∂t
.
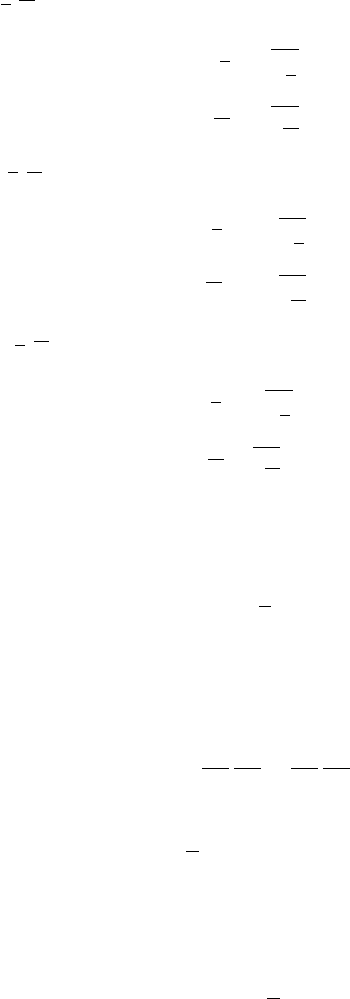
A.2 Canonical transformations 231
Equivalent forms of the generating functions are the following:
(i) F = F (q
,P,t) with transformation rules:
p
=
∂F
∂q
Q =
∂F
∂P
;
(ii) F = F (p
,Q,t) with transformation rules:
q
= −
∂F
∂p
P = −
∂F
∂Q
; (A.7)
(iii) F = F (p
,P,t) with transformation rules:
q
= −
∂F
∂p
Q =
∂F
∂P
.
Example. Let us compute the values of α and β for which the following transfor-
mation is canonical:
P = αpe
βq
Q =
1
α
e
−βq
;
for such values we proceed to find the corresponding generating function.
Let us use the Poisson brackets to check the canonicity of the transformation; in
particular, since the change of variables is one–dimensional, it suffices to verify that
{Q, P }≡
∂Q
∂q
∂P
∂p
−
∂Q
∂p
∂P
∂q
=1.
Therefore one has:
−
β
α
e
−βq
· αe
βq
=1,
which is satisfied for β = −1 and for any α = 0. In this case the transformation
becomes:
P = αpe
−q
Q =
1
α
e
q
. (A.8)

232 A Basics of Hamiltonian dynamics
Let us look for a generating function F = F (q, P), whose transformation rules are
given by
p =
∂F
∂q
Q =
∂F
∂P
.
From (A.8) one has:
p =
P
α
e
q
Q =
1
α
e
q
.
Therefore it should be
∂F
∂q
=
P
α
e
q
, (A.9)
namely F (q, P )=
P
α
e
q
+ f(P ), where f(P ) is a total function of P ; analogously,
from the relation
∂F
∂P
=
1
α
e
q
, (A.10)
one finds F (q, P)=
P
α
e
q
+ g(q), where g(q) depends only on the variable q. Com-
paring the solutions of (A.9) and (A.10) one obtains f(P )=g(q) = 0, thus yielding
F (q,P)=
P
α
e
q
.
A.3 Integrable systems
A Hamiltonian system with n degrees of freedom is said to be integrable if there
exist n integrals, say U
1
, ..., U
n
, which satisfy the following assumptions:
(1) the integrals are in involution: {U
j
,U
k
} =0foranyj, k =1,...,n;
(2) the integrals are independent, which implies that the matrix
⎛
⎜
⎝
∂U
1
∂p
1
...
∂U
1
∂p
n
∂U
1
∂q
1
...
∂U
1
∂q
n
.
.
.
∂U
n
∂p
1
...
∂U
n
∂p
n
∂U
n
∂q
1
...
∂U
n
∂q
n
⎞
⎟
⎠
has rank n;
(3) in place of (2) one can require the non–singularity condition:
det
⎛
⎜
⎝
∂U
1
∂p
1
...
∂U
1
∂p
n
.
.
.
∂U
n
∂p
1
...
∂U
n
∂p
n
⎞
⎟
⎠
=0;
notice that this condition is stronger than the independence of item (2).

A.4 Action–angle variables 233
Having fixed a point (p
0
,q
0
), let α
0
= U(p
0
,q
0
), where U ≡ (U
1
,...,U
n
); for
α
∈ R
n
define the manifold M
α
as
M
α
= {(p,q) ∈ R
2n
: U
1
(p,q)=α
1
,...,U
n
(p,q)=α
n
} .
The integrability of a Hamiltonian system can be obtained through the following
theorem [5].
Liouville–Arnold theorem. Suppose that the Hamiltonian H(p
,q), p, q ∈ R
n
,
admits n integrals U
1
, ..., U
n
, satisfying the above conditions of involution and
non–singularity. Assume that the manifold M
α
is compact in a suitable neighbor-
hood of α
0
. Then, there exists a transformation of coordinates from (p,q) to (I,ϕ)
with I
∈ R
n
, ϕ ∈ T
n
, such that the new Hamiltonian H
1
takes the form
H
1
(I,ϕ) ≡ h(I) ,
for a suitable function h = h(I
).
A.4 Action–angle variables
Consider the mechanical system described by the Hamiltonian H(p,q), where p ∈
R
n
, q ∈ R
n
. When dealing with integrable systems one can introduce a canonical
transformation C :(p
,q) ∈ R
2n
→ (I,ϕ) ∈ R
n
× T
n
, such that the transformed
Hamiltonian depends only on the action variables I
:
H◦C(I
,ϕ)=h(I) ,
for some function h = h(I
). The coordinates (I,ϕ)areknownasaction–angle
variables [5].
The Liouville–Arnold theorem provides an explicit algorithm to construct the
action–angle variables. In particular, let us introduce as transformed momenta the
actions (I
1
,...,I
n
) defined through the relation
I
i
=
)
p
i
dq
i
,
where the integral is computed over a full cycle of motion. If the initial Hamiltonian
is completely integrable, it will depend only on the action variables, say
h = h(I
1
,...,I
n
) . (A.11)
The canonical variables conjugated to I
i
are named angle variables; they will be
denoted as (ϕ
1
,...,ϕ
n
). One immediately recognizes that Hamilton’s equations
associated to (A.11) are integrable. Indeed, let us define the frequency or rotation
vector as
ω
= ω(I)=
∂h(I
)
∂I
;
