Casing/Tubing design manual october 2005 Chevron

Подождите немного. Документ загружается.

With continued load increase, a sinusoidally buckled tube will further deform and

eventually assume the form of a helix. The exact value at which sinusoidal

buckling transforms to helical buckling is a subject of controversy, as illustrated

by the following two equations (7-11 and 7-12), and is a topic of active research

within the industry.

EIw

sin2

(7-11)

()

EIw

sin

1222 −=

(7-12)

Further loading tightens the pitch of the helix and increases the wall contact

force. Eventually, the frictional resistance associated with the wall contact force

may reach a value that no longer permits movement. So-called “lockup” of the

tube occurs, a condition that is assuming increasing importance in determining

the maximum penetration that can be achieved in a horizontal wellbore

extension.

7.6 Buckling in a Curved Wellbore

Kyllingstad and co-workers [He and Kyllingstad, 1993; Kyllingstad, 1995] have

extended Dawson and Paslay's formula to the case of wellbores with curvature.

Given the following input variables:

• Radial clearance,

• Young's modulus,

• Tube cross-sectional moment of inertia,

• Normal force per length,

The critical buckling force to initiate sinusoidal buckling is:

EIN

. (7-13)

For a straight, inclined wellbore,

sin

and Dawson and Paslay's equation

is recovered. For more general wellbores, given the input variables:

• Inclination from the vertical,

• Bearing or azimuth,

• Tube effective weight,

• Effective tension,

7-6 Casing/Tubing Design Manual

October 2005

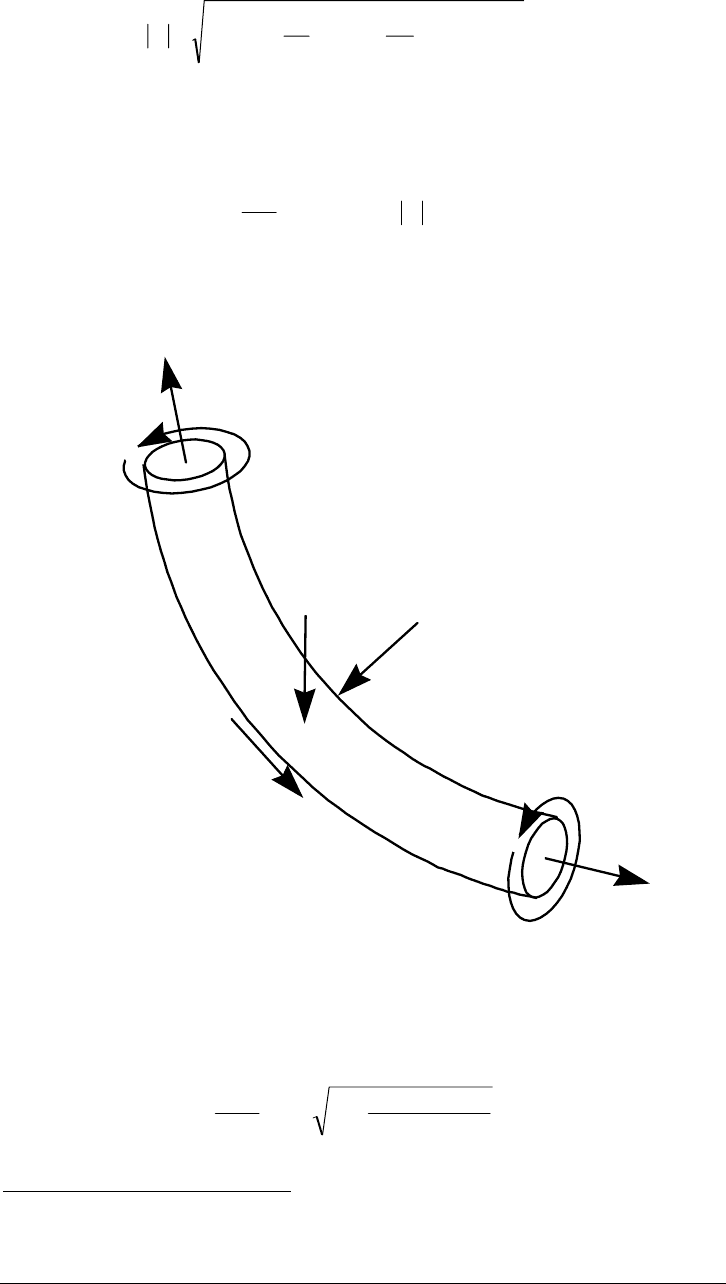
The normal force per unit length of tubular is given by the so-called “soft string”

model [Johancsik et al., 1984]:

eee

⎛

⎝

⎜

⎞

⎠

⎟

⎛

⎝

⎜

⎞

⎠

⎟

sin sin

(7-14)

Where

is length along the tubular string, s

0 at the bit/shoe, and given the

these input variables: coefficient of friction between the tube and its confining

hole,

. The change in effective tension along the tubular is:

=±cos

αµ

.(7-15)

The sign of the friction term is such that friction opposes relative motion between

the tube and its confining hole.

w ds

N ds

= µ|N|

T + dT

M + dM

α + dα,

β + dβ

α, β

Figure 7-1 Soft String Model Forces and Moments

For a 2D build wellbore (without azimuth change), an explicit critical buckling

force equation was proposed [Wu and Juvkam-Wold, 1995],

⎥

⎦

⎤

⎢

⎣

⎡

++=

Rwr

kEI

sin

(7-16)

The soft string model ignores the bending stiffness of the tube. For high values of

curvature, this assumption may be replaced by more detailed numerical analysis.

Casing/Tubing Design Manual 7-7

October 2005

where

is the radius of curvature of the 2D build wellbore.

The corresponding explicit critical buckling force in a 2D drop wellbore (without

azimuth change) took two formulas for a large-curvature drop wellbore

(

FRw

≥ sin

⎥

⎦

⎤

⎢

⎣

⎡

−+=

Rwr

kEI

sin

(7-17)

and for a small-curvature drop (

FRw

≤

sin

⎥

⎦

⎤

⎢

⎣

⎡

−+= 1

sin

Rwr

kEI

(7-18)

The factor of K in equations (7-16) to (7-18) is a subject of controversy with a

value of 2 or 4, and is a topic of further research within the industry.

7.7 Effect of Inclination Increase (Build)

Note the following items in equation (7-14):

• For buckling to occur at all, must be negative

• If the inclination is increasing, dds

is negative (recall that

is measured

upward from the bottom of the string)

Therefore, in a potential buckling situation, a build section results in an increase

in the normal force,

, and, thus, an increase in the critical buckling force. The

increase of buckling force in a build section is obvious in equation 7-16, as it

corresponds to a decrease of wellbore radius of curvature,

sin α = 0

dα/ds = -Curvature

a. Build Section

sin α = 0

dα/ds = -Curvature

b. Drop Section

Figure 7-2 Effect of Wellbore Curvature on Critical Buckling Force

7-8 Casing/Tubing Design Manual

October 2005

7.8 Effect of Inclination Decrease (Drop)

Note the following in equation (7-14):

• For buckling to occur at all, must be negative

• If the inclination is decreasing, dds

is positive (recall

is measured

upward from the bottom of the string)

In a potential buckling situation, therefore, a drop section may result in either (1)

an increase in the normal force,

, and, thus, an increase in the critical buckling

force or (2) a decrease in the normal force and, thus, a decrease in the critical

buckling force. The governing effect depends on the value of the term of

Fd ds

(which is always negative) as compared to the term of w

sin

(which

is always positive).

Increasing the former term from zero lowers the normal force and lowers the

critical buckling force. This decrease of buckling force occurs in a small-curvature

drop section as reflected in equation (7-18). Physically, the tube is being lifted off

the low side of the hole by axial compression.

If, however, the former term is sufficiently large (under the large-curvature drop

section), it will surpass the influence of the weight term and become dominant. In

this instance, the normal force begins to increase, resulting in an increase of

critical buckling force, as the drop rate increases. This increase of buckling force

in a drop section is reflected in equation (7-17). Physically, this corresponds to

the tube having been lifted off the low side of the hole entirely and contacting the

high side of the hole. Further decreases in

(increases in its absolute value)

force the tube to the low side of the hole, producing an effect much like that

observed for a build section.

7.9 Effect of Azimuth Change (Turn)

Note in equation (7-14) that any change in azimuth results in an increase in the

normal force, , and, thus, an increase in the critical buckling force is

suggested.

7.10 Length Change in a Vertical

Wellbore

Below the neutral point the buckled tube assumes a helical configuration, with

the radius of the helix determined by the radial clearance between the tube and

its constraining hole. The pitch of the helix will vary with distance from the neutral

point. The more negative the effective tension, the smaller the pitch. With regard

to axial movement of a free end of the tubular string, helical buckling manifests

itself as a length change. The value of this length change is particularly important

in tubing designs involving a packer and a polished bore receptacle.

Casing/Tubing Design Manual 7-9

October 2005

Given the following input variables:

• Radial clearance, r

• Effective tension at bottom of interval,

• Young's modulus,

• Tube outside diameter,

• Tube wall thickness,

• Tube cross-sectional moment of inertia,

• Tube effective weight, w

• Original length of tube

the length change because of helical buckling is given by,

∆L

EIw

BKL

=−

(7-19)

when the distance from

to the neutral point is less than the length of the

tubular string and,

∆L

EIw

BKL

=− −

⎛

⎝

⎜

⎞

⎠

⎟

⎛

⎝

⎜

⎞

⎠

⎟

(7-20)

when the distance from

to the neutral point is greater than the length of the

tubular string. The moment of inertia of the cross section is:

()

(

)

IDDt=−−

(7-21)

7.10.1 Example Problem

Continuing the previous example, assume the depth of 3,000 m (9,842.5 ft)

marks the lower end of the tubing string. Further, assume the tubing is confined

in 177.8 mm, 38.73-kg/m (7 in., 26 lb/ft) casing having an inside diameter of

159.4 mm (6.276 in.). Compute the length change because of helical buckling.

The procedure is summarized in the following table.

Table 7-4 Example Procedure

Action Variable Metric English

Input variables Fe

-0.0652 MN

10.01 kg/m

73.03 mm

5.515 mm

-14658 lb

6.73 lb/ft

2.875 in.

0.217 in.

To be clear, L is the unsupported length of tube above the point at which F

calculated. The presence of any lateral support defines the extent of tube to be analyzed.

Of course, for casing the cement sheath is the ultimate lateral support, preventing helical

buckling altogether.

7-10 Casing/Tubing Design Manual

October 2005

Action Variable Metric English

3,000 m

159.4 mm

2.068x105 MPa

9,842.5 ft

6.276 in.

30x106 psi

Compute radial clearance

Compute moment of inertia

Note from previous example

that distance to neutral point is

less than L

43.18 mm

6.710x105 mm

1.700 in.

1.61 in

Compute length change

∆

BKL

-69.7 mm -2.87 in.

In this particular case, the buckling length change is small. According to the

severity of the buckling load, however, this component of overall length change

can be significant.

7.11 Length Change in Inclined and

Curved Wellbores

To date, a complete analysis of the length change because of combined

sinusoidal and helical buckling in inclined and curved wellbores has not been

presented. Mitchell [1996] has presented a numerical solution for length change

in an inclined wellbore and concluded that, according to the value of the effective

force, the length change can be much less than that predicted using the model

for helical buckling in a vertical wellbore. Industry research continues in this area.

7.12 Permanent Corkscrewing

Permanent corkscrewing refers to extreme cases of helical buckling when the

combination of the axial, radial, circumferential, and, particularly, bending

stresses accompanying buckling are so severe that the tube body yields. After

the tube yields, subsequent unloading will not completely eliminate all

deformation and the tube will have permanent helical curvature.

To determine if a buckled state is sufficient to result in permanent deformation,

the radial and circumferential stresses are determined. Given the following input

variables:

• Internal pressure,

• External pressure,

• Axial stress,

• Tube outside diameter,

• Tube wall thickness,

these stresses are determined from,

Casing/Tubing Design Manual 7-11

October 2005

(

)

ii oo

ioio

pr pr

pprr

rr r

−

22 2

(7-22)

(

)

−

pr p r

pprr

rr r

ii oo

ioio

22 2

(7-23)

where

r and are the inner and outer radii of the tube, respectively. The total

axial stress is given by,

zzazb

, (7-24)

where

is the axial stress and

is the bending stress associated with

helical buckling. Given the following input variables:

• Effective tension at bottom of interval,

• Tube outside diameter,

• Tube cross-sectional moment of inertia,

The bending stress associated with helical buckling is,

DrF

=±

,(7-25)

where:

rrr

≤

.(7-26)

When

, and

are known, the stress state may be compared to multi-

dimensional yield as defined by the von Mises criterion:

σ σ σ σ σσ σσ σσ

θθ

er zr rz

=++− − −

222

(7-27)

If the stresses, as combined above, equal or exceed

, yield has occurred and

the tube will be permanently corkscrewed.

In the presence of bending due to buckling, it is uncertain whether the highest

value of the right-hand side of

will occur at the inner or outer radius, so both

locations must be checked by successively setting

to and r . r r

i o

Further, it is usually not immediately clear whether adding or subtracting the

bending stress will result in the highest value, so both the inner and outer

longitudinal fibers of the bent tube must be checked by adding and subtracting

the bending stress. These four possibilities correspond to separate calculations

at the most compressed (because of bending) circumferential position on the

inner radius, the most compressed position on the outer radius, the most

extended position on the inner radius, and the most extended position on the

outer radius.

7-12 Casing/Tubing Design Manual

October 2005

7.13 References

1. Dawson, R., and Paslay, P. R.: “Drillpipe Buckling in Inclined Holes,” Journal

of Petroleum Technology (1984) 1734-1738.

2. He, X., and Kyllingstad, A.: "Helical Buckling and Lock-Up Conditions for

Coiled Tubing in Curved Wells," SPE 25370 presented at the 1993 SPE Asia

Pacific Oil & Gas Conference, Singapore, 8-10 February.

3. Johancsik, C. A., Friesen, D. B., and Dawson, R.: “Torque and Drag in

Directional Wells -- Prediction and Measurement,” JPT (1984) 987-92.

4. Kyllingstad, A.: "Buckling of Tubular Strings in Curved Wells," Petroleum

Science & Engineering, Vol. 12 (1995), 209-218.

5. Lubinski, A.: Developments in Petroleum Engineering, Collected Works of

Arthur Lubinski, Volume One, Stefan Miska (ed.), Gulf Publishing Company,

Houston, Texas (1987).

6. Mitchell, R. F.: “Buckling Analysis in Deviated Wells: A Practical Method,”

paper SPE 36761 presented at the 1996 SPE Annual Technical Conference

and Exhibition, Denver, Colorado, 6-9 October.

7. Roark, R. J., and Young, W. C.: Formulas for Stress and Strain, Fifth Edition,

McGraw Hill, New York (1982).

8. Wu, J., and Juvkam-Wold, H. C.: "Buckling and Lockup of Tubulars in

Inclined Wellbores, " Journal of Energy Resources Technology, Vol. 117,

(1995) 208-213.

9. Wu, J., and Juvkam-Wold, H. C.: “The Effect of Wellbore Curvature on

Tubular Buckling and Lockup in Wellbores," Journal of Energy Resources

Technology, Vol. 117, (1995) 214-218.

10. Ziegler, H.: Principles of Structural Stability, Blaisdell, Waltham,

Massachusetts, (1968).

Casing/Tubing Design Manual 7-13

October 2005

Casing/Tubing Design Manual 8-1

April 2007

8 Tube Design

8.1 Introduction..............................................................................................8-1

8.2 Design Factors........................................................................................8-1

8.2.1 Collapse ..................................................................................................8-2

8.2.2 Burst (API)...............................................................................................8-2

8.2.3 Burst (VME).............................................................................................8-2

8.2.4 Burst (Full-Yield)......................................................................................8-3

8.2.5 Tension/Compression .............................................................................8-3

8.2.6 Exception.................................................................................................8-3

8.3 HPHT Wells ................................................................................................8-3

8.4 Casing Design Criteria................................................................................8-5

8.5 References................................................................................................8-10

8.1 Introduction

The design of casing and tubing is the selection of appropriate tubular weight and

grade, achieving the required tube strength necessary to withstand all anticipated

tube loads, by meeting the following relationship:

Tube Design Factor

Tube Load

≤

Tube Strength

The tube load and tube strength have been discussed in the previous chapters.

The tube design factor is discussed in the next section.

The last section is the heart of Chevron design standardization. Nevertheless,

there will be exceptions to the stated rules. Where possible, these exceptions are

treated. Otherwise, deviations from the guidelines on load conditions should only

be practiced with caution.

8.2 Design Factors

Design factors represent the degree to which you are unsure of either the

performance of a tubular and/or the loads to which the tubular is to be subjected.

Variations of design factor values among users can typically be traced to:

• Load assumptions. One user may use a lower design factor, but consistently

base his design on higher design loads. For example, a 1.0 collapse factor

coupled with a load assumption of total evacuation may be just as severe as

a 1.2 design factor coupled with assuming the tubular to be partially filled at

all times.

• An abundance or lack of conservatism. Ideally, design factors should be

based on sound engineering principles and not prejudice. Exceptions to this

statement do exist, particularly in the case of possible risk to citizens who

may live/work close to a proposed wellbore.

8-2 Casing/Tubing Design Manual

April 2007

The design factors that should be normally used are given in Table 8-1.

Table 8-1. Design Factors

Failure Mode Design Factor

Collapse 1.00 or 0.85 on good cement section

Burst (API) 1.10 ~ 1.20

Burst (VME, Full-Yield) 1.20

Triaxial Yield 1.20

Tension/compression 1.60/1.30

8.2.1 Collapse

The collapse design factors of 1.00 or lower are acceptable because the tube

collapse performance determined by the API (Bul 5C3) are derived statistically,

to ensure that only a small percentage of samples will collapse below the rated

pressure

. Further, collapse design loads are normally taken as extreme

instances of external pressure differential (tube evacuation).

8.2.2 Burst (API)

A design factor of 1.10 to 1.20 for tube burst design when API burst strength is

used in tube deign should permit a reasonable design. This is because burst

strength (internal yield pressure) is based on the Barlow equation, a non-

conservative simplification of the Lamé equations and an approximation of the

tube initial yield under the burst pressure. Nevertheless, there is still a good deal

of conservatism in burst design because of the use of specified minimum yield

strength, downrating the tube wall thickness by the maximum allowable 12.5 %,

and use of initial yield as a criterion.

8.2.3 Burst (VME)

A design factor of 1.20 for tube burst design when von Mises (VME) burst

strength is used in tube design should permit a reasonable design.

The VME burst of a tube is based on the accurate initial yield of the tube by the

burst pressure under multi-dimensional stress state. This tube burst criterion

normally gives a slightly higher burst pressure for a tube than the API internal

yield pressure [Wu, 2002]. Even the same conservatism in burst design is

employed with the use of specified minimum yield strength, the downrating of the

tube wall thickness by the maximum allowable 12.5%, and the use of initial yield

as a criterion.

For example, the plastic collapse formula is based on the conception that there is a 95%

probability or confidence level that the collapse pressure will exceed the minimum stated

pressure with no more than 0.5% failures.