Cain G., Meyer G.H. Separation of Variables for Partial Differential Equations: An Eigenfunction Approach
Подождите немного. Документ загружается.

page_262
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_262.html[22/02/2009 23:54:23]
< previous page page_262 next page >
Page 262
Here
u
and
v
are the pressures in the fluid film and in the porous slider. The last term in the Reynolds
equation (9.1) models the lubricant flow in and out of the slider as predicted by Darcy’s law which
governs the fluid flow in the porous slider.
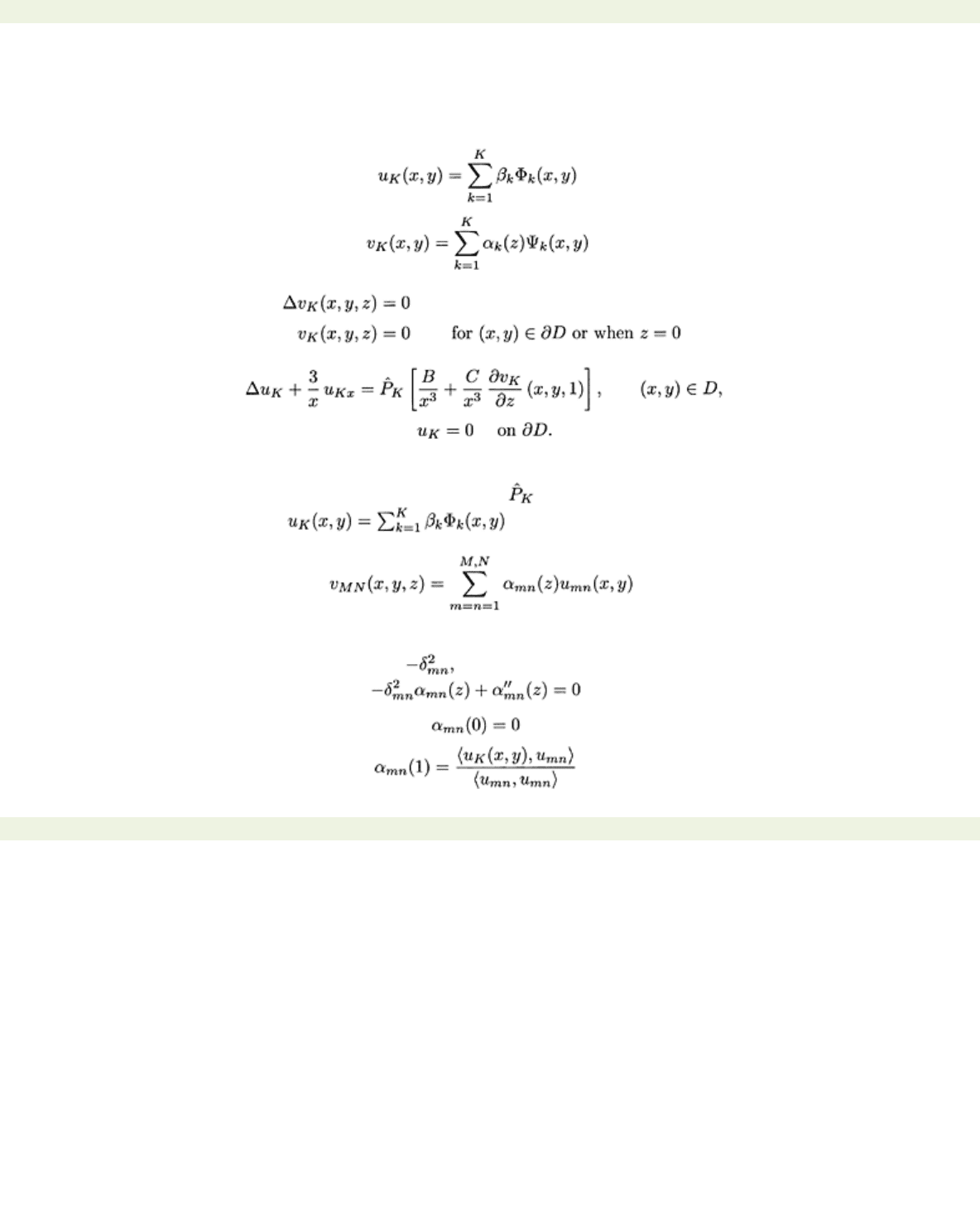
We are going to find an approximate solution
{uK(x, y), vK(x, y, z)}
of the form
such that
and
The continuity condition is approximated by
vK
(
x, y,
1)=
PKuK(x, y)
.
The functions {Φ
k
}, {Ψ
k
} and the projections
PK
and will be introduced below.
Assume for the moment that
is known. Then
v
can be found with an
eigenfunction expansion in the usual way. We write
where
{umn(x, y)}
are the eigenfunctions of the two-dimensional Laplacian found in Example 8.12, and
where
PMN
denotes the orthogonal projection onto the span of these first
MN
eigenfunctions. If the
corresponding eigenvalues are denoted by then
< previous page page_262 next page >

page_263
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_263.html[22/02/2009 23:54:23]
< previous page page_263 next page >
Page 263
where
The solution is
For the remainder of this example it will be convenient to order the eigenfunctions linearly for 1≤
n≤N
and 1≤
m
≤
M
by defining
k=
(
n−
1)
M+m, k
=1,…,
K
=
MN,
so that
n=[k/M]
+1,
m
=
k
−(n−1)
M,
and writing
It follows that
As a consequence
where
Let us now turn to the solution
uK(x, y).
In view of Example 8.5 it is straightforward to verify that the
two-dimensional eigenvalue problem
has count ably many eigenfunctions
with corresponding eigenvalues
< previous page page_263 next page >
page_264
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_264.html[22/02/2009 23:54:24]
< previous page page_264 next page >
Page 264
where and λm is the mth positive root of
f
(λ)=
J
1(λ
x
0)
Y
1(λ
a
)−
J
1(λ
a
)
Y
1(λ
x
0)=0.
These eigenfunctions are orthogonal over
D
with respect to the weight function
w(x, y)=x
3. As above
we shall order them linearly and denote by
the orthogonal projection onto span with respect
to the inner product
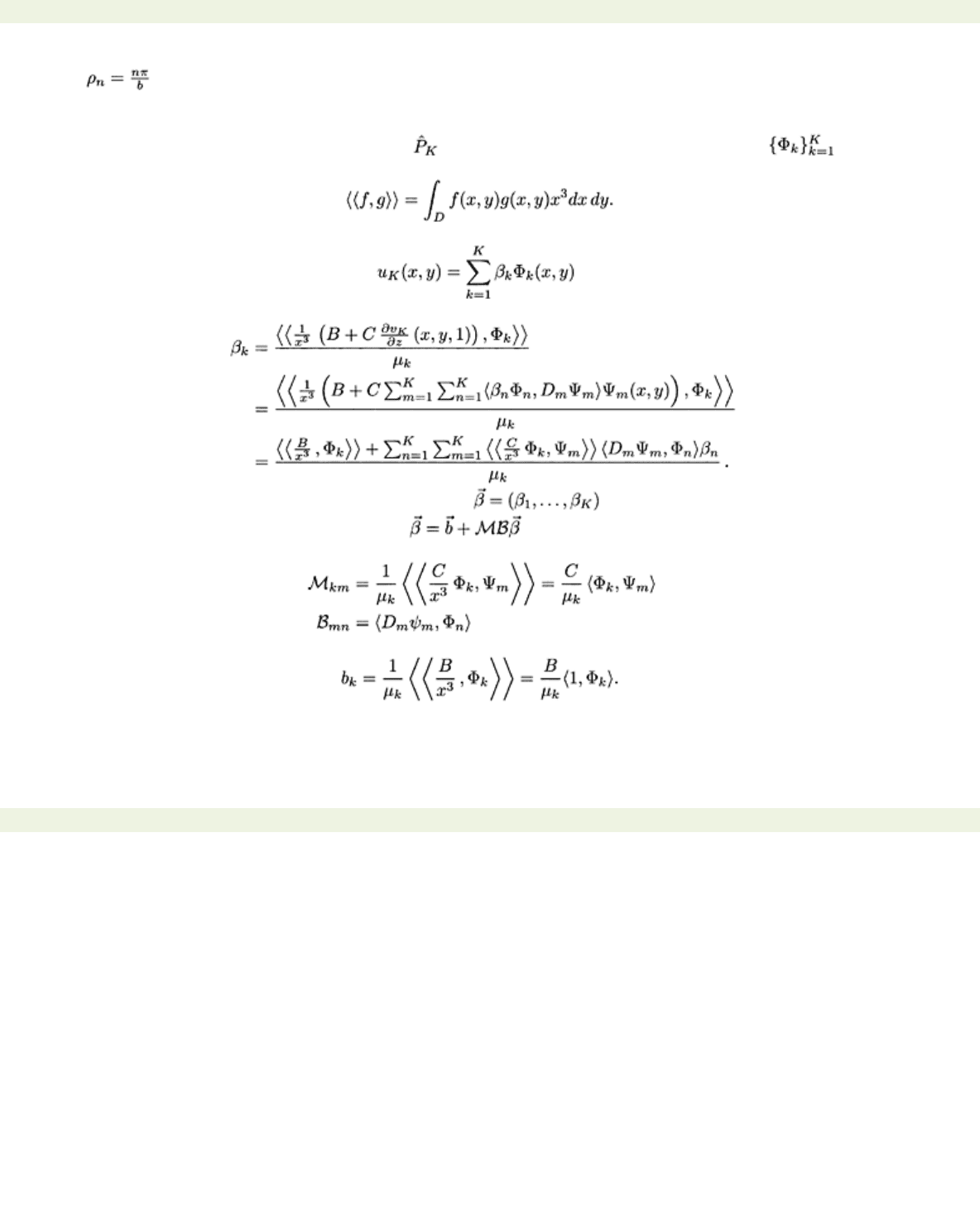
Analogous to our derivation of the Green’s function
GKK
in Example 8.13 we find that
is a solution of (9.1) if and only if for
k=
1,…,
K
These equations can be written in matrix form for as
where
and
We point out that
uK(x, y)
and
vK
(
x, y,
1) belong to different function spaces because they are
expanded in terms of different eigenfunctions. They are linked through the interface continuity
approximation
vK
(
x, y,
1)=
PKuK(x, y)
< previous page page_264 next page >

page_265
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_265.html[22/02/2009 23:54:25]
< previous page page_265 next page >
Page 265
which is an algebraic system for the
2K
degrees of freedom in the expansions but does not imply that
vK
(
x, y,
1) and
uK(x, y)
are the same. In view of our numerical experiments with Example 8.11 a least
squares minimization of
may be a viable alternative for linking
u
and
v
at
z
=1.
9.2 The eigenvalue problem for the Laplacian in
Example 9.5 An eigenvalue problem for quadrilaterals.
Let us consider the representative eigenvalue problem
where
D
1=(0,
a
)×(0,
b
).
We know from Example 8.12 that the eigenvalue problem
has the eigenfunctions
umn(x, y)
=sin λ
mx
sin
ρny
with
and eigenvalues
As in Example 8.12 we look for an eigenfunction of the form
u(x, y, z)=αmn(z)umn(x, y).
Substitution into the three-dimensional eigenvalue problem shows that
< previous page page_265 next page >

page_266
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_266.html[22/02/2009 23:54:26]
< previous page page_266 next page >
Page 266
This eigenvalue problem for
αmn(z)
has solutions
αmnp(z)
=cos
ηpz
whenever
for an integer
p≥1.
Hence for any integer
m, n, p
≥1 we have the eigenfunction
umnp(x, y, z)
=sin λ
mx
sin
ρny
cos
ηpz
with corresponding eigenvalue
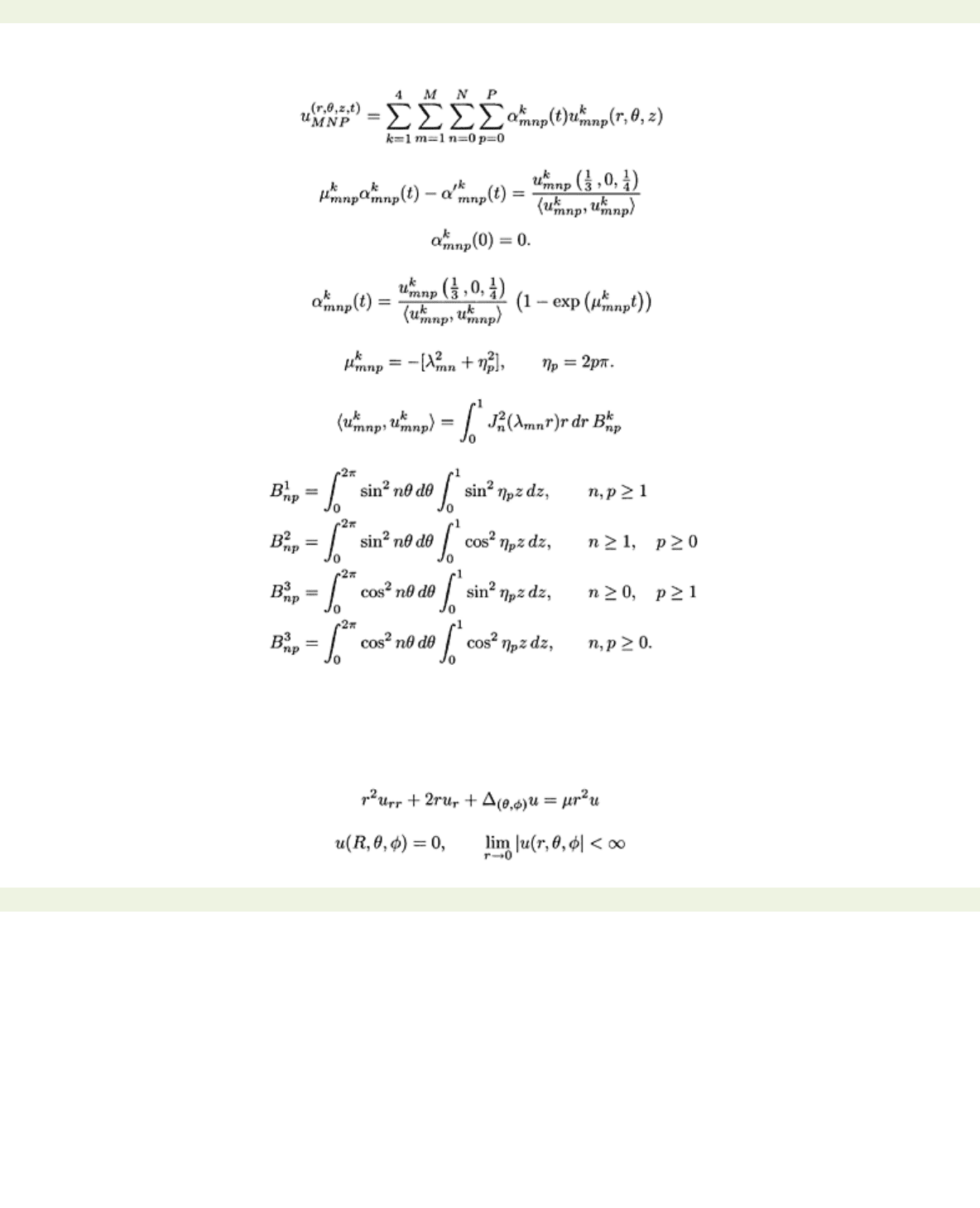
Example 9.6 An eigenvalue problem for the Laplacian in a cylinder.
We consider eigenfunctions which are periodic in
z
with period
c
and whose radial derivatives vanish on
the mantle of a cylinder with radius
R.
Hence we consider
where the base of the cylinder is
From Example 8.14 we know that the functions
satisfy
The boundary condition at
r=R
requires
For each
n
≥0 there are count ably many nonzero solutions {λ
mn
} such that
< previous page page_266 next page >

page_267
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_267.html[22/02/2009 23:54:27]
< previous page page_267 next page >
Page 267
Hence the two-dimensional eigenfunctions are with the corresponding eigenvalues
where
xmn
is the mth nonzero root of the function These roots are available numerically.
When an eigenfunction for the cylinder is written in the form
and substituted into the eigenvalue equation, then must be a solution of
Since does not depend on
i,
we can drop the superscript
i
. Hence we have an eigenvalue problem
for
αmn(z)
which is solved by sines and cosines which are
c
periodic so that
where
Hence to each of the four eigenfunctions
with
k
=2(
i
−1)+
j
corresponds the eigenvalue µ of the Laplacian
The eigenfunctions are orthogonal when integrated over the cylinder, i.e., with respect to the inner
product
Example 9.7 Periodic heat flow in a cylinder.
< previous page page_267 next page >

page_268
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_268.html[22/02/2009 23:54:28]
< previous page page_268 next page >
Page 268
We consider a model problem for heat flow in a long cylinder of radius
R
=1 with regularly spaced
identical heat sources and convective cooling along its length.
As a mathematical model we shall accept
where
D
is the unit disk.
The approximate solution is written in the form
where Φ
q(r, θ, z)
is a z-periodic eigenfunction of the problem
Example 9.6 shows that the eigenfunctions are of the form
with
and as given in Example 9.6. The radial eigenvalues {λ
mn
} differ from those of Example
9.6. Here λ
mn
is the mth positive root of the nonlinear equation
For each
n
there are count ably many roots {λ
mn
} which must be found numerically. The corresponding
radial functions
Jn
(λ
mnr
) are mutually orthogonal in The eigenvalue corresponding to
remains
The approximating problem is
< previous page page_268 next page >
page_269
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_269.html[22/02/2009 23:54:28]
< previous page page_269 next page >
Page 269
with the above initial and boundary conditions. It is solved by
where
Hence
for
Note that
with
Wave propagation in a cylinder is handled analogously. Different boundary conditions at
r=R
and at
z
=0,
c
will require different eigenfunctions of the Laplacian on a cylinder, but all computations are
reasonably straightforward.
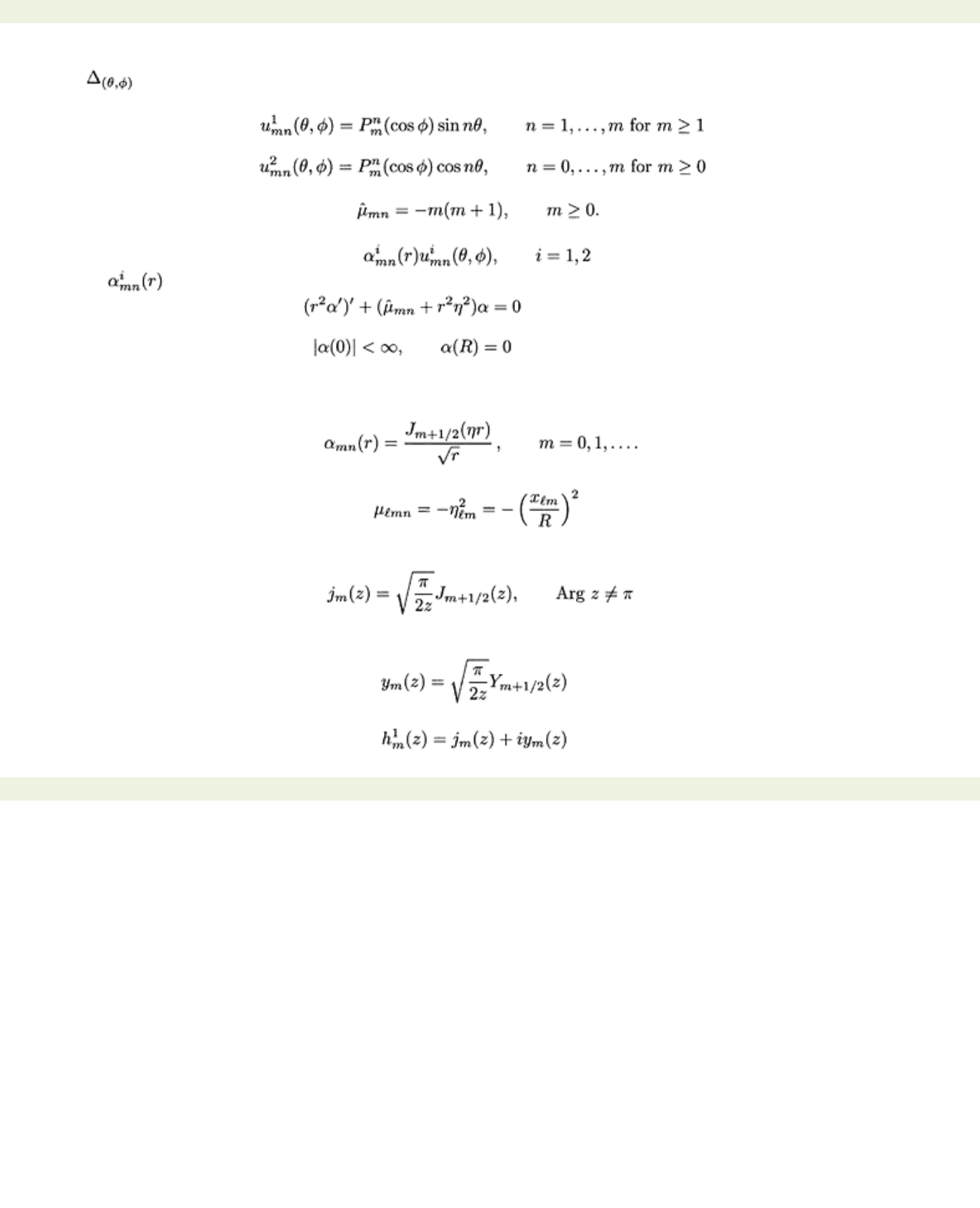
Example 9.8 An eigenvalue problem for the Laplacian in a sphere.
In order to solve heat flow and oscillation problems in a sphere we need the eigenfunctions of the
Laplacian. We shall study the eigenvalues associated with the Dirichlet problem, i.e.
< previous page page_269 next page >
page_270
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_270.html[22/02/2009 23:54:29]
< previous page page_270 next page >
Page 270
where is the Laplacian on the unit sphere considered in Example 8.15. We know from Example
8.15 that its eigenfunctions are
with corresponding eigenvalues
Hence an eigenfunction of the form
results if is a solution of the eigenvalue problem
(9.2)
where for notational convenience we have set
µ=−η
2
.
This differential equation is known from special function theory (or from (3.10)) to have a bounded
solution of the form
It follows that the eigenvalues of the Laplacian in spherical coordinates are given by
where
xℓm
is the
ℓ
th positive root of the Bessel function
Jm+1/2(x).
It is customary in special function theory to call the function
a “spherical Bessel function of the first kind of order m.” Similarly, one can define a spherical Bessel
function of the second kind of order m in terms of Bessel functions of the second kind
and the so-called spherical Hankel function
< previous page page_270 next page >

page_271
file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_271.html[22/02/2009 23:54:30]
< previous page page_271 next page >
Page 271
A discussion of these functions and their behavior at
z
=0 and as may be found, for example, in
[1]. In this application then
xℓm
is also the
ℓ
th positive root of the spherical Bessel function of the first
kind of order
m.
For each eigenvalue
uℓmn
with fixed
ℓ
and
m
we have the eigenfunctions
We verify by direct integration over the sphere that the eigenfunctions corresponding to the same
eigenvalue are all mutually orthogonal. The general theory assures that eigenfunctions with distinct
eigenvalues likewise are orthogonal.
Example 9.9 The eigenvalue problem for Schrödinger’s equation with a spherically
symmetric potential well.
The last example of this book is a classic textbook problem in quantum mechanics (see, e.g., [20]). It is
chosen to remind the reader that eigenfunction expansions are commonplace in quantum mechanics,
that the eigenfunctions of the Laplacian are essential building blocks for the eigenfunctions of the
Schrödinger equation, and that other eigenvalue problems occur naturally which no longer have the
concise structure of Sturm-Liouville problems but which can still be attacked with the algorithms
employed throughout these pages.
The arguments of Example 9.8 need only minor modifications when we consider the eigenvalue problem
for the Schrödinger equation
(9.3)
where
ħ
and
m
are constants and
V(r)
is a nonpositive function given as
Since
V
is discontinuous, the above Schrödinger equation cannot have a classical solution everywhere.
The postulates of quantum mechanics ask for a function
u
which is a classical solution where
V
is
continuous, which is continuous and has continuous gradients at all points, and which decays as
such that
Let us now solve equation (9.3). An eigenfunction expansion solution would be of the form
< previous page page_271 next page >
