Cain G., Meyer G.H. Separation of Variables for Partial Differential Equations: An Eigenfunction Approach

Подождите немного. Документ загружается.

page_202

file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_202.html[22/02/2009 23:53:36]

< previous page page_202 next page >

Page 202

With homogeneous boundary conditions on opposing sides of

∂D

we can apply the eigenfunction

expansion of Chapter 5. Note that the computed solution

uN=wN

will satisfy the given boundary

conditions on

0 and

x=a

exactly and the approximate boundary condition

This approach breaks down when the function

v(x, y)

defined above (and its analogue which

interpolates the data

(

0) and

g(x, b))

is not differentiable. Then, in principle, problem (8.5) can be

solved with the formal splitting

u=u

where

and

F(x, y)=F

(x, y)+F

(x, y).

As in Example 8.1, this formal splitting can introduce discontinuities into the boundary data at the

corners of

∂D

which may not be present in the original problem (8.5). In this case a preconditioning of

the problem is advantageous which assures that the splitting has continuous boundary data. The

exposition of preconditioning for the general case is quite involved and included here for reference. It

presupposes that

is such that the problem (8.5) admits a classical solution with high regularity. This

requires certain consistency conditions on

at the corners of

∂D

which are derived below.

To be specific let us examine the solution

of (8.5) near the corner point

(a, b)

. We wish to find a

smooth function

v(x, y)

so that the new dependent variable

w=u−v,

which solves the problem

w=F

−Δ

v=G(x, y)

< previous page page_202 next page >

page_203

file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_203.html[22/02/2009 23:53:37]

< previous page page_203 next page >

Page 203

can be found with a formal splitting without introducing an artificial singularity at

(a, b)

Suppose for the moment that such a

has been found. If we write

w=w

2 and

G=G

then

1 and

2 solve

and

where

(8.6)

Now we can apply our eigenfunction expansion. The eigenvalue problem associated with

1 is

Its solution is discussed at length in Section 3.1. Let

and denote the eigenvalues and

eigenfunctions for

0 where, depending on

1 and

0=0 or 1. Then the problem for

1 is

approximated by

where

< previous page page_203 next page >

page_204

file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_204.html[22/02/2009 23:53:38]

< previous page page_204 next page >

Page 204

and

This problem is solved by

where

The solution

αn(y)

has the form

αn(y)=d

1 sinh λ

ny+d

2 cosh λ

ny+αnp(y)

Whether one can solve for the coefficients

1 and

2 depends on the data of the problem. For

2>0

any solution we can construct is necessarily unique because the solution of the Robin problem is unique

as shown in Section 1.3.

Similarly,

2 is approximated by the solution

of the problem

where

< previous page page_204 next page >

page_205

file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_205.html[22/02/2009 23:53:39]

< previous page page_205 next page >

Page 205

and

Here and

{ψn(y)}

are the eigenvalues and eigenfunctions of

The solution is

where

Let us now turn to the choice of

. The discussion of the Gibbs phenomenon in Chapter 4 suggests that

the preconditioning should result in boundary data that satisfy the same homogeneous boundary

condition at the corner points as the eigenfunctions used for their approximation. This will be the case

for

h(x, b)

and

h(a, y)

at the point

(a, b)

and

(Remember that the coefficients

ci(x, y)

are constants along

=0,

and

= 0,

Substitution of (8.6) for

h(x, y)

leads to the two equations

< previous page page_205 next page >

page_206

file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_206.html[22/02/2009 23:53:39]

< previous page page_206 next page >

Page 206

and

Thus

(8.7)

Both equations have the same left-hand side and hence can have a solution only if

(8.8)

We shall call the boundary condition consistent at

(a, b)

if equation (8.8) holds. The meaning of (8.8)

becomes a little clearer if we look at two special cases. Suppose that Dirichlet data are imposed on

x=a

and

y=b,

i.e.

u(x, y)=g(x, y)

x=a

and on

y=b

Then

(a, y)=c

(x, b)=

0 and

(a, y)=c

(x, b)

=1. The consistency condition (8.8)

simply implies that

is continuous on

∂D

(a, b)

. Equation (8.7) reduces to the preconditioning

condition known from Example 8.1

v(a, b)=g(a, b).

Suppose next that

Then

1=1 and

2=0 and (8.8) requires that

< previous page page_206 next page >

page_207

file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_207.html[22/02/2009 23:53:40]

< previous page page_207 next page >

Page 207

Hence if

is differentiable on

∂D

(a, b),

then

uxy(x, y)

is continuous on

∂D

(a, b)

. In this case

(8.7) requires

In general it is straightforward to show with Gaussian elimination that if (8.5b) holds and can be

differentiated along

and

y=b,

then the resulting four equations for u(a, b),

ux(a, b), uy(a, b),

and

uxy(a, b)

are overdetermined and consistent only if (8.8) holds. If the data are not consistent, then

preconditioning is generally not possible. On the other hand, if this consistency condition is met, then

we can choose for

v(x, y)

any smooth function which satisfies (8.7). Similar arguments apply to

h(x, y)

at the other three corners of the rectangle. If the data are consistent everywhere, then we look for a

smooth function

v(x, y)

which satisfies four equations like (8.7). It is straightforward to verify that the

function

with

and

satisfies equation (8.7) and

vab=vabx=vaby=vabxy

=0 at (0, 0), (

0), and (0,

Similar functions of the form

(x, y)=C(x−a)

(y−b)

b(x, y)=D(x−a)

va0(x, y)=Ex

(y−b)

with appropriate coefficients

D, D,

and

allow us to express

v(x, y)

v(x, y)=v

(x, y)+va

(x, y)+vab(x, y)+v

b(x, y)

Preconditioning with such a polynomial introduces an additional smooth source term into Poisson’s

equation for

i.e.

=Δ

−Δ

F(x, y)

−Δ

v(x, y),

< previous page page_207 next page >

page_208

file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_208.html[22/02/2009 23:53:41]

< previous page page_208 next page >

Page 208

but its influence on the eigenfunction expansion solution appears to be small because the problems to

be solved with the formal splitting have classical solutions with the same smoothness as the solution

of the original problem. Hence preconditioning of the data to ensure that the subsequent splitting has a

smooth solution is not particularly arduous and greatly improves the accuracy of the approximate

solution.

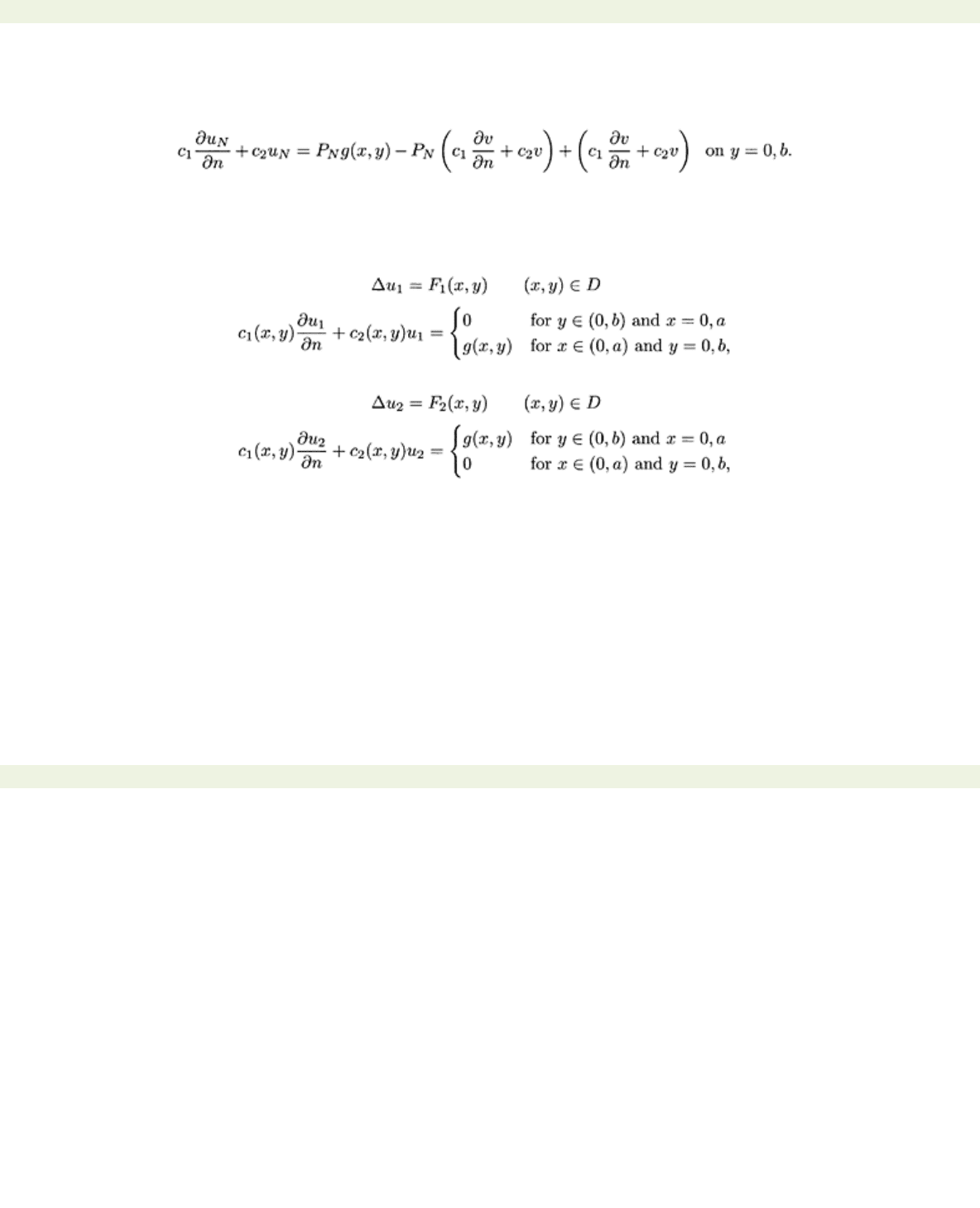
As illustration let us consider the problem

Figure 8.2: (a) A graphical display of the test problem.

A useful mnemonic to visualize the problem and help keep track of various splittings is to graph

geometry and problem as in Fig. 8.2a. It is straightforward to check that these data are consistent at

the corners (1, 0), (1, 1), and (0, 1) but not at (0, 0) where

< previous page page_208 next page >

page_209

file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_209.html[22/02/2009 23:53:42]

< previous page page_209 next page >

Page 209

We shall solve this problem three different ways. First, we write

w(x, y)=u(x, y)−v(x, y)

with

v(x, y)

=(1

−y

)2(1

−x

2/2, (8.9)

and apply the eigenfunction expansion method to

The eigenfunctions are

so that we will see a Gibbs phenomenon in the

approximation of

G(x, y)

0 and

1, and in the approximation of

wy(x, 0)

=0. Our second

solution is obtained from the formal splitting

u=u

2 where

and

It is easy to see that the boundary data for

1 are not consistent at (1, 1) and that those for

2 are not

consistent at (0, 0) and (1, 1). The discontinuity in

at (0, 0) is inherent in the problem. The

discontinuities at (1, 1) are caused by the formal splitting.

The artificial inconsistency at (1, 1) can be removed by preconditioning the data. We observe that the

eigenfunctions

and

ψ(y)

of the formal splitting satisfy the boundary conditions

We define

w=u−v.

< previous page page_209 next page >

page_210

file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_210.html[22/02/2009 23:53:42]

< previous page page_210 next page >

Page 210

Then

should be chosen such that the boundary condition

(

1)=

−1)−

(

1) can be

expanded in terms of

without introducing a Gibbs phenomenon. This requires that

(0, 1)=0

and

(1, 1)=0. Similarly

(0,

)=(1

−y

−v

(0,

)

should satisfy the boundary conditions of

{ψn (y)},

i.e.

(0,0)=

(0,1)=0.

Similar expressions hold along the lines

0 and

1. Altogether we have the conditions

This leads to

(1, 0)=

(0, 1)=0,

y(1, 1)=1

and the inconsistent condition

We observe that the function

satisfies the conditions at (1, 0), (1, 1), and (0, 1). Then the preconditioned problem is

We split the problem by writing

w=w

where

1=0

1(0,

1(1,

)=0

< previous page page_210 next page >

page_211

file:///G|/%5EDoc%5E/_new_new/got/020349878X/files/page_211.html[22/02/2009 23:53:43]

< previous page page_211 next page >

Page 211

(

0)=0,

(

1)=2

(

−1)

and

−

2(0,

)=(1

−y

, w

2(1,

)=0

(

0)=

(

1)=0.

We verify by differentiating the Dirichlet data along the boundary that the data for

1 and

2 are

consistent at the corners except for the point (0, 0) where

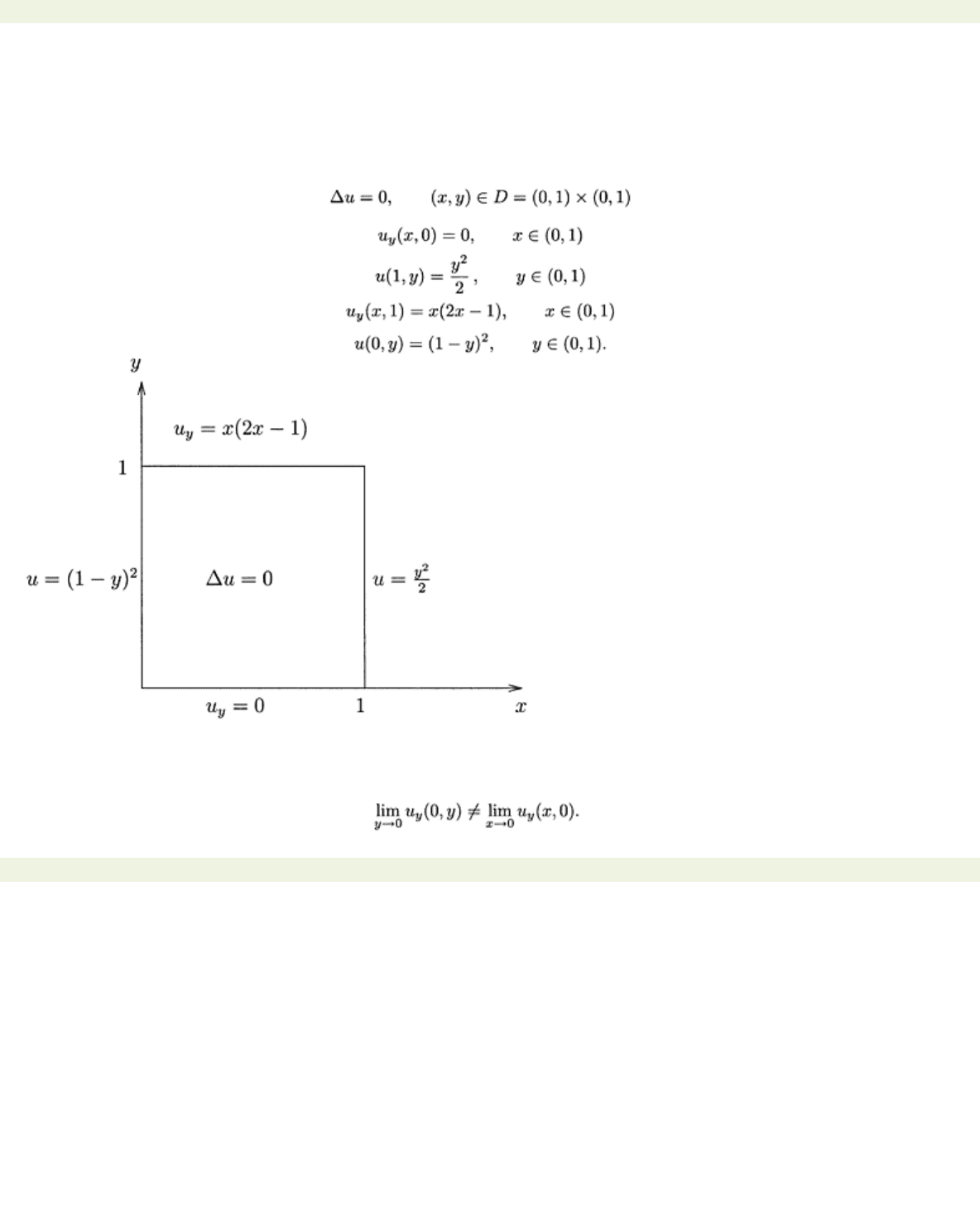
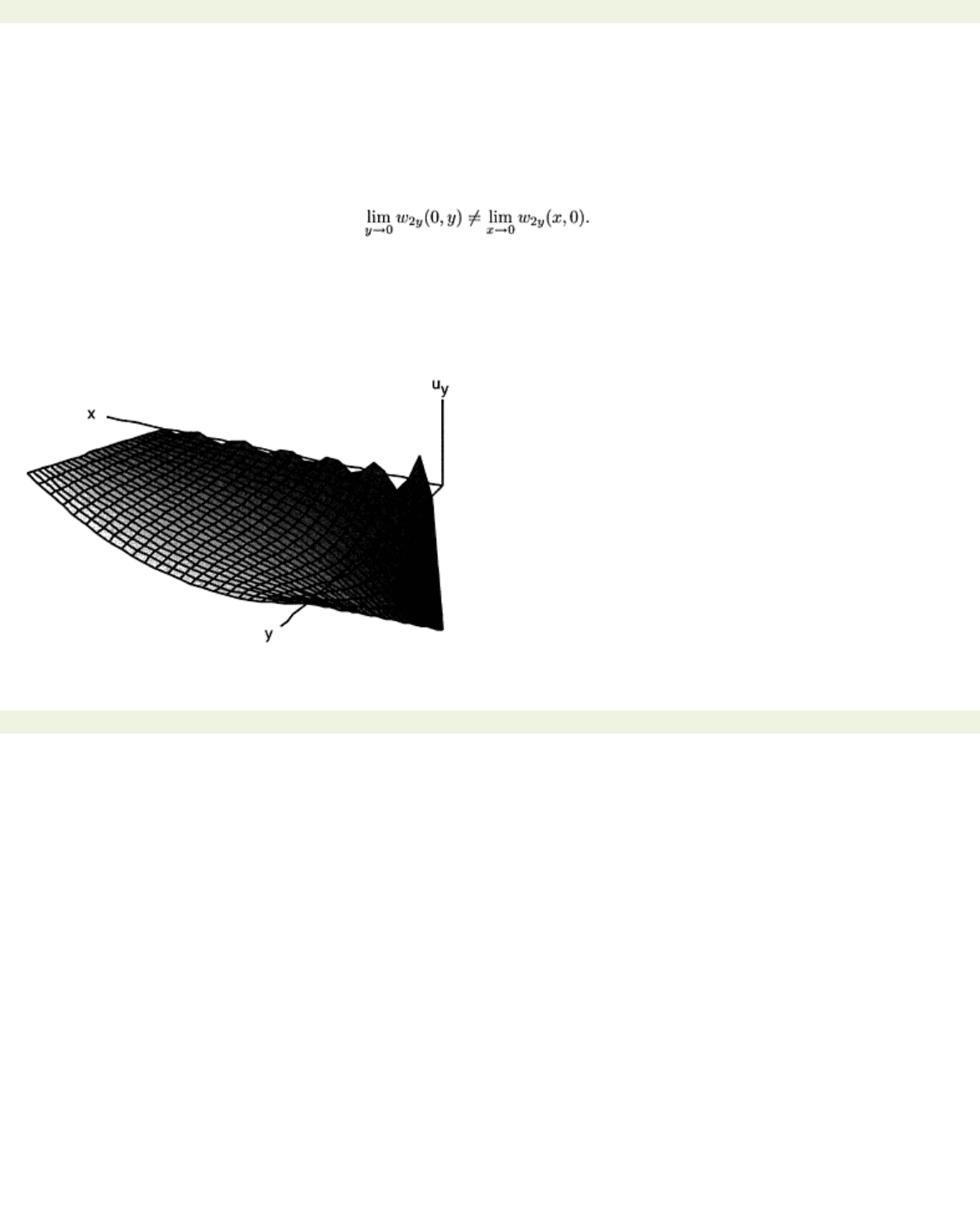
The separation of variables approximations to

1 and

2 are readily obtained. Since the preconditioning

in this problem was carried out to preserve the smoothness of

at three corners, we show in Figs.

8.2b, c, d the surfaces for

obtained with the three formulations. The discontinuity at (0, 0) is

unavoidable, but otherwise one would expect a good solution to be smooth. Clearly it pays to

precondition the problem before splitting it. Zeroing out inhomogeneous but smooth boundary data on

opposite sides with (8.9) and using an eigenfunction expansion preserves smoothness, requires no

preconditioning, and is our choice for such problems.

Figure 8.2 (b) Eigenfunction expansion with exact boundary data at

0, 1 and a Gibbs phenomenon on

< previous page page_211 next page >