Caballero B. (ed.) Encyclopaedia of Food Science, Food Technology and Nutrition. Ten-Volume Set

Подождите немного. Документ загружается.

different types of bran can vary widely. Rice bran

contains approximately 10% phytic acid, whereas

wheat bran and rye bran contain only 5%. The phytic

acid content of different cereals depends on the

botanic subfamily to which the grain belongs.

Wheat and rye are members of the Hordeae sub-

family, whereas rice is a member of the Oryzeae.

The phytic acid content of oat bran (subfamily:

Aveneae) is approximately 6%.

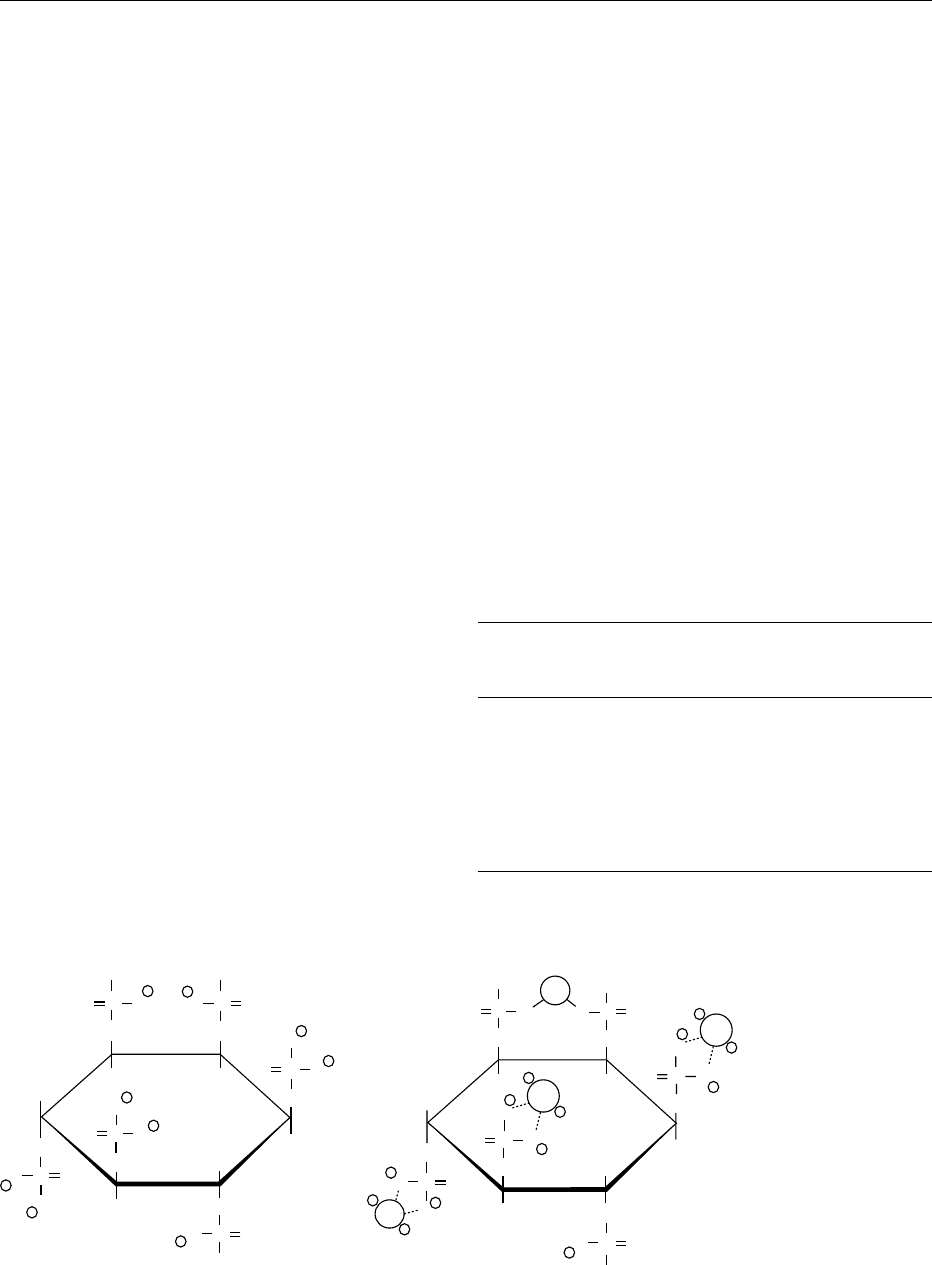
0009 Phytic acid can strongly chelate multivalent metal

ions, especially the positively charged ions zinc, cal-

cium, and iron. The chelates are named phytates.

Such chemical bonds with multivalent cations can

be formed intra- and intermolecularly by one or

several phosphate groups of the pyhtic acid molecule

(Figure 2). These complexes are insoluble.

0010 The distribution of phytic acid in different morpho-

logic layers of seeds can vary significantly. In cereal

grains from the Hordeae subfamily, phytic acid is

normally concentrated in the aleurone cells and, to

a lesser degree, in the germ. The aleurone layer of

wheat grain, for example, contains 85% of the phytic

acid, the embryo 13%, and the endosperm 2%. In

contrast, 80% of the phytic acid content of rice and

maize is concentrated in the pericarp and embryo,

respectively.

0011 Inositol-6-phosphate and inositol-5-phosphate

show the strongest inhibiting effect on mineral bio-

availability, whereas the effect of inositol-4-phosphate

and inositol-3-phosphate, two products of inositol-6-

phosphate hydrolysis, is markedly lower. Phytic acid is

hydrolyzed by the enzyme phytase. This enzyme was

first described in rice bran. In wheat bran, the highest

phytase activity is present in the aleurone layer, where

more than 40% of the phytase activity is located.

Approximately 34% is present in the endosperm,

15% in the scutellum, and 3% in the germ. In rye

bran, the phytase activity is 10–20 times higher than

in the rye flour. The total amount of cereal phytase

activity varies depending on the food origin: phytase

activity is high in wheat and rye grains, medium in

barley, and low to very low in oat and maize grain.

(See Phytic Acid: Properties and Determination;

Nutritional Impact.)

Lignans

0012Lignans are phenolic dimers possessing a 2,3-diben-

zylbutane structure. Such compounds are known to

exist as minor constituents of many plants, where they

form the building blocks for the formation of lignin in

the plant cell wall. The compounds occur mainly in

the glycosidic form. In rye, lignans are predominantly

present in the bran fraction (Table 2). The glycosides

are converted by fermentation in the proximal colon

to mammalian lignans. The two major mammalian

lignans, enterodiol and enterolactone, are the prod-

ucts of colonic bacterial metabolism of the plant lig-

nans secoisolariciresinol and matairesinol.

−

fig0002 Figure 2 Structure of phytic acid and a phytate chelate at neutral pH. From Ingelmann H-J, Rimbach G and Pallauf J (1993)

Phytinsa

ure – ein antinutritiver Faktor? Ernhrungs^Umschau 40: 400–404, with permission.

tbl0002Table 2 Lignan content of whole grains and bran

Secoisolariciresinol

(mgper100g

dry weight)

Matairesinol

(mgper100g

dry weight)

Wheat bran (whole grain) 33 3

Wheat bran 110 0

Rye meal 47 65

Rye bran 132 167

Oat meal 13 0

Oat bran 24 155

Barley (whole grain) 58 0

Barley bran 63 0

Adapted from Mazur and Adlercreutz. Pure and Applied Chemistry

70: 1759–1776.

1846 DIETARY FIBER/Bran

Physiologic Effects of Bran

Stool weight

0013 Stool weight is significantly increased by adding

sources of insoluble fiber to the diet. The physio-

logical effect of dietary fiber is largely determined

by the size of the particles. One gram of fine wheat

bran increases the stool weight by 3.1 g, and 1 g

of coarse bran increases the stool weight by ap-

proximately 6.2 g. Barley bran is more effective in

increasing stool weight than fine wheat bran but

less effective than coarse wheat bran. Comparable

amounts of fiber provided by wheat and oat bran

have the same effect on daily stool output, even

though > 90% of wheat bran fiber and only 50–

60% of oat bran fiber are insoluble. Wheat bran

increases the fecal concentration of sugars and mass

of plant material more than oat bran, whereas oat

bran increases the fecal bacterial mass more. Oat bran

increases the stool weight by providing rapidly fer-

mented soluble fiber in the proximal colon for bacter-

ial growth, which is sustained until excretion by

fermentation of the insoluble fiber. Bacteria and lipids

are major contributors to the increase in stool weight

with oat bran consumption, whereas ingested plant

fiber is responsible for much of the increase in stool

weight with wheat-bran consumption.

0014 Constipated subjects should start increasing diet-

ary fiber by 2–4 g, e.g., with 1–2 tablespoons of bran.

Plenty of liquid should be taken with bran, as it may

cause irritations in the digestive tract. Intake can be

increased to three times a day with a meal. Bran

should be mixed with food in order to absorb water

and increase stool volume. Coarse bran holds water

better than fine bran. Depending on the severity of

constipation, improvement may take a few days to

several months.

Sterol Metabolism

0015 Bile acids Soluble dietary fiber can adsorb bile acids.

Accordingly, oat bran and rye bran increase fecal

excretion of total bile acids, whereas wheat bran has

no or only modest effects. Moreover, oat bran and rye

bran influence the metabolism of bile acids. Rye bran

increases the daily excretion and percentage of conju-

gated primary bile acids (cholic acid, chenodesoxy-

cholic acid) and lowers the percentage of free

secondary bile acids (desoxycholic acid, lithocholic

acid). Rye bran thus decreases the ratio of secondary

bile acids to primary bile acids. Rye bran, but not

wheat bran or barley bran, reduces the ratio of litho-

cholic acid to desoxycholic acid. Oat bran enlarges

the cholic acid pool, increases the relative proportion

of chenodeoxycholate in bile, and reduces the relative

proportion of desoxycholic acid, whereas cholate

remains unchanged.

0016Blood cholesterol levels Since the first study per-

formed in 1963 in humans with fiber-rich rolled

oats, several studies have reported a beneficial effect

of oat bran or oat meal-enriched diets on plasma

cholesterol levels in healthy subjects or mildly hyper-

lipidemic subjects. The daily intakes ranged from 17

to 100 g oat bran, and the duration was 2–12 weeks.

Oat bran and oat gum (80% b-glucan) has been

served in drinks and instant whips, or baked in

bread and muffins. The majority of controlled studies

performed in hyperlipidemic subjects showed a

decrease in plasma total cholesterol, with most de-

creases in the range of 4to10%. It is noteworthy

that decreases in plasma cholesterol are related to

decreases in LDL cholesterol, whereas while HDL

cholesterol does not generally noticeably change.

Consequently, oat bran causes a modest reduction in

blood cholesterol level. Larger reductions are seen in

subjects who have initially higher blood cholesterol

levels (> 5.9 mmol l

1

or 229 mg dl

1

). Studies have

shown clearly that soluble b-glucan in oat fiber has a

cholesterol-lowering effect in animals and humans.

About 3 g of b-glucan per day causes a 10% drop in

LDL cholesterol. In bread and muffins, the baking

process can decrease the solubility and/or molecu-

lar weight of b-glucans, resulting in a weak serum

cholesterol-lowering effect. Approximately 100 g of

oat bran should be ingested daily to have any effect on

plasma cholesterol levels. Oat b-glucan also improves

glucose and insulin regulation. The mechanisms of

action of viscous polysaccharides like b-glucans in

the human gastrointrestinal tract are not completely

understood. Increased luminal viscosity is believed to

cause delayed gastric emptying and reduce the rate of

absorption of nutrients in the small intestine, thereby

attenuating the postprandial glucose and insulin

response to an oral carbohydrate load. Viscous gums

alter bile acid- and cholesterol metabolism, reduce

and alter the site of lipid absorption, and undergo

fermentation in the colon to short-chain fatty acids.

All of these effects might contribute to a reduction in

serum cholesterol levels in humans. Full-fat rice bran

leads to a similar reduction in serum LDL cholesterol

compared with oat bran. Rice bran contains low

amounts of soluble fiber, but rice bran oil has a hypo-

lipidemic effect. Essentially all human studies per-

formed since 1969 have shown no effects of wheat

bran on fasting blood total cholesterol as well as

LDL cholesterol in healthy or hyperlipidemic subjects

following a chronic intake of 14–77 g of wheat bran

per day. Moreover, in rats, wheat bran essentially

does not change fasting blood lipid parameters.

DIETARY FIBER/Bran 1847

(See Cholesterol: Properties and Determination; Ab-

sorption, Function, and Metabolism.)

0017 Female sex hormones A daily intake of 15 g of

wheat bran can significantly reduce circulating levels

of estradiol and estrone in premenopausal women by

approximately 15–20%. The mechanism by which

wheat bran modulates serum estrogen levels most

likely involves their enterohepatic circulation. Estro-

gens are conjugated in the liver to form glucoronides

and sulfoglucoronides, and 20–50% of estrogen

metabolites are excreted in the bile as these polar

biologically inactive substances. Approximately

80% of the estrogens in the gut are reabsorbed, a

process that requires deconjugation by bacterial

enzymes. Wheat bran intake may lower fecal b-glu-

coronidase activity. An additional mechanism may be

the binding of unconjugated estrogens to fiber in the

gut, thus impeding their reabsorption. Oat bran

causes reductions in fecal b-glucuronidase activity to

a lesser extent than wheat bran. Maize bran has no

significant effect on this enzyme.

0018 Vitamin D A high intake of wheat bran can also

influence the metabolism of vitamin D, another ster-

oid substance: an additional intake of 20 g of dietary

fiber per day from wheat bran can reduce the plasma

half-life of tritium-labeled 25-hydroxyvitamin D by

approximately 30%. A dietary fiber-induced decrease

in vitamin D status may diminish active absorption of

dietary calcium. The occurrence of rickets and osteo-

malacia in Asians living in the UK has been partially

associated with a high intake of fiber-rich chapatties

(unleavened bread). However, such an adverse effect

may only be of clinical importance in subjects with

marginal or insufficient vitamin D status and low

dietary calcium intake. (See Vitamins: Overview.)

Effects of Bran on Mineral Metabolism

0019 It has been demonstrated in several human studies

that wheat bran and high-fiber diets decrease intes-

tinal Ca absorption. Wheat bran alters the usual

inverse relationship between calcium load and frac-

tional absorption in humans. This effect is the result

of the high Ca-binding capacity of wheat bran. At

pH 8.0, 1 g of wheat bran can bind approximately

0.45 mmol of calcium. This amount is slightly higher

than the calcium binding of rye bran (0.38 mmol of

Ca per gram of bran) and slightly lower than the

calcium binding of rice bran (0.49 mmol g

1

whereas soy bran only binds 0.19 mmol of calcium

per gram at pH 8.0. In wheat bran, phytic acid and

dietary fiber are both present in high quantities.

Theoretically, each mole of phytic acid can maximally

bind 6 moles of calcium. Phytic acid can form

insoluble calcium salts resulting in a low bioavailabil-

ity of calcium, but wheat bran can bind more calcium

in vitro than expected from the molar binding ratio

of bran phytate:calcium. In vitro calcium binding to

wheat bran is linear over a wide range. Thus, the

amount of calcium binding by wheat bran cannot be

explained by the content of phytic acid alone. Other

bran constituents may influence calcium bioavaila-

bility. Although neutral cellulose has little affinity to

cations, lignin strongly binds calcium. The lignin in

wheat bran is indigestible during the gastrointestinal

transit. However, as the result of an adaptation pro-

cess, wheat bran intake is also associated with a re-

duced renal calcium excretion. Consequently, a high

bran intake does not necessarily increase the risk of a

negative calcium balance.

0020Intakes of high (> 15 g) amounts of bran (wheat,

oat, barley) can reduce zinc absorption and decrease

iron availability. Measures to reduce the phytate con-

tent of cereals like malting and soaking (activation

of endogenous phytase activity) can significantly im-

prove zinc and iron absorption. Nevertheless, in diets

that provide sufficient amounts of trace elements,

decreased absorption does not necessarily influence

the trace element balance and status of human sub-

jects. A reduced endogenous fecal excretion can com-

pensate for the lower absorption. However, if zinc

and iron intakes are marginal or inadequate, the

additional consumption of a fiber concentrate may

contribute to the development of trace element

deficiencies.

0021In animal diets, supplemental phytases of microbial

and cereal sources improve dietary phytate phos-

phorus utilization. Such a measure is environmentally

advantageous in order to replace the content of inor-

ganic phosphorus in those diets.

Beneficial Health Effects of Bran and its

Components

0022In animals, wheat bran causes a significant reduction

in incidence of mammary cancer. A suggested protect-

ive effect of wheat bran on breast cancer in humans

may be due, at least in part, to the effect on circulat-

ing estrogen concentrations and their bioavailability.

Moreover, the lignans especially present in the aleur-

one layer of some cereals (Table 2) may exert anti-

estrogenic effects in humans.

0023Wheat bran and its component phytic acid have

both been shown to decrease early markers of colon

carcinogenesis like certain indices of cell proliferation

and certain aberrant cryt foci parameters. Wheat

bran, dephytinized wheat bran, and phytic acid

induce cell apoptosis, increase cell differentiation,

1848 DIETARY FIBER/Bran

and favorably affect colon morphology, thus indicat-

ing that phytic acid as well as other components of

bran may be preventive against colon cancer. A lower

concentration of the co-carcinogenic secondary bile

acids and a high bacterial production of butyrate may

contribute to a normal metabolism and proliferation

of the colon epithelial cells after bran intake.

0024 Phytic acid inhibits in vitro and in vivo the enzym-

atic degradation of starch and reduces the glycemic

index owing to complexation of the starch molecule,

amylase, and calcium ions, which are essential for the

amylase activity. The reduced rate of glucose absorp-

tion and reduced postprandial increase in blood

glucose may both be beneficial in prophylaxis and

treatment of diabetes mellitus. Protective effects of

phytic acid against lead toxicity have been demon-

strated in the presence of calcium ions. Acute toxic

effects of 1 g of lead per kilogram of diet can be

reduced by 20 g of calcium phytate per kilogram.

Commercial Products

0025 Both fine wheat bran and coarse wheat bran are avail-

able for the consumer. One tablespoon of bran contains

approximately 2 g of dietary fiber. Bran may be used

alone as a cereal, mixed with other cereals, sprinkled on

cereals or included in bran muffins, hamburgers, etc.

Bran is legally regarded as a food. Moisture should not

exceed 14%, and the amount of starch in wheat bran

should not exceed 15% of the dry weight. Since espe-

cially the bran fraction of wheat can accumulate rela-

tively high amounts of cadmium, the cadmium content

should not exceed 50–60 mgkg

1

. Wheat bran tablets

are also obtainable – one tablet equals approximately

2 g of dietary fiber. Moreover, breakfast cereals with a

bran content up to 85% are in the market (such as

Kellogs All-Bran Plus

). These products contain 11 g

of dietary fiber per 40-g portion. Several baked goods

are enriched with wheat bran to increase the fiber

content. Enrichment should at least result in a doubling

of the dietary fiber content, and a good source of diet-

ary fiber should contain 2 g per portion. Although

coarse wheat bran increases the stool weight more

efficiently than fine wheat bran, the latter is often

used to enrich baked goods, as it is easier to process

and is preferred by the consumer.

0026 Pure oat bran flakes are also commercially avail-

able. One portion (25 g) of oat bran contains approxi-

mately 2.3 g of insoluble and 1.95 g of soluble dietary

fiber. Moreover, oat bran-enriched breakfast cereals

and oat flakes containing 80% oat bran are obtain-

able, and oat bran can be used as a fat replacement in

sausages like Frankfurters (6% oat bran, 30% water).

0027 Protex

(Food Engineering International, Inc.) is a

specially processed rice bran derivative that may be

used in baked goods, breakfast cereals, macaroni and

noodle products, and milk-like beverages.

New Potential Sources

0028Psyllium is a new source of dietary fiber. It is made from

ground husks of psyllium seeds and has the ability to

hold water and form bulk. Psyllium contains up to

85% soluble fiber. Psyllium holds water better than

bran, so smaller amounts are needed to be effective.

Moreover, psyllium effectively lowers serum LDL chol-

esterol and blood glucose and insulin levels. In 1998,

the US Food and Drug Administration ruled that psy-

llium, like oat bran, may reduce the risk of heart dis-

ease. Psyllium has several advantages over oat bran. It

is more versatile, since it is a far more concentrated

form of fiber. The US Food and Drug Administration

allows the addition of psyllium to breakfast cereals,

and FDA officials expect more psyllium-containing

foods, from waffles to biscuits, to hit the market.

0029Soy bran is a dietary fiber concentrate that is

obtained during the production of soy protein, and

the insoluble portion is separated by centrifugation.

The concentrate is defatted and contains the soy

hulls. The dietary fiber content is approximately 67–

80% (Table 3). Soy bran is sold in competition

with other dietary fiber concentrates. It is effective

in increasing stool weight (Table 2), and 25 g of soy

fiber significantly reduces total serum cholesterol

levels, especially in patients with blood cholesterol

concentrations above 7.7 mmol l

1

(300 mg dl

1

0030Several other food residues with a high dietary fiber

content are obtained during food processing (Table 4).

They can be used by food technologists, e.g., for the

fiber enrichment of bread (beer draff) or for the pro-

cessing of fiber and b-carotene-rich fruit juices (carrot

husks).

0031Wheat fiber (fiber content 97%) is a concentrate of

cellulose and hemicellulose that is free of phytic acid

tbl0003Table 3 Effect of bran and other fiber-rich foods on stool weight

Efficiency

index

increase in stool weight ðgÞ

fiber intake ðgÞ

Wheat bran, fine

3.1

Wheat bran, coarse

6.2

Bread, whole wheat

grain, fine

5.6

Bread, whole wheat

grain, coarse

8.2

Barley fiber

concentrate

4.2

Oat bran

4.54

Soy bran

1.5–2.3

Carrots, raw

3.6

Daily intake of 14 g of fiber;

daily intake of 30–65 g of fiber.

DIETARY FIBER/Bran 1849

and gluten. This insoluble wheat fiber can be used for

the production of common foods and also for the

preparation of dietetic foods (e.g., for patients with

celiac disease).

0032 The drying of apples results in a dietary fiber con-

centrate that is rich in soluble fiber (pectins). The

combination of wheat and apple fiber influences the

ratio of insoluble to soluble dietary fiber in processed

foods.

See also: Bioavailability of Nutrients; Cereals:

Contribution to the Diet; Dietary Fiber: Properties and

Sources; Effects of Fiber on Absorption; Phytic Acid:

Nutritional Impact; Nutritional Impact

Further Reading

Bartnik M and Jakubczyk T (1989) Chemical composition

and nutritive value of wheat bran. In: Bourne GH (ed.)

Nutritional Value of Cereal Products, Beans and

Starches. World Review of Nutrition and Dietetics 60:

92–131.

Batchelor AJ and Compston JE (1983) Reduced plasma

half-life of radio-labelled 25-hydroxyvitamin D

subjects receiving a high-fiber diet. British Journal of

Nutrition 49: 213–216.

Chen HL, Haack VS, Janecky CW, Vollendorf NW and

Marlett JA (1998) Mechanisms by which wheat bran

and oat bran increase stool weight in humans. American

Journal of Clinical Nutrition 68: 711–719.

Ferguson LR and Harris PF (1999) Protection against

cancer by wheat bran: role of dietary fibre and phyto-

chemicals. European Journal of Cancer Prevention 8:

17–25.

Lairon D (1996) Dietary fibres: effects on lipid metabolism

and mechanisms of action. European Journal of Clinical

Nutrition 50: 125–133.

Rose DP, Goldman M, Connolly JM and Strong LE (1991)

High-fiber diet reduces serum estrogen concentrations

in premenopausal women. American Journal of Clinical

Nutrition 54: 520–525.

Saunders RM (1990) The properties of rice bran as a food-

stuff. Cereal Foods World 35: 632–636.

Wood P (ed.) (1993) Oat Bran. St. Paul, MN: American

Association of Cereal Chemists.

Energy Value

S Brooks, 3W Banting Research Centre, Ottawa,

Canada

R Mongeau, Ottawa, Aylmer (Que

bec), Quebec,

Canada

Caloric Value in Human Nutrition

0001All food contains energy, which is needed by the body

to fuel metabolic processes. This energy is obtained

though the oxidation of macronutrients (carbo-

hydrate, fat, and protein) and is partly captured as

chemical energy in compounds such as adenosine

triphosphate (ATP). These ‘high-energy’ compounds

are used to drive many processes, including the bio-

synthesis of cellular components, the maintenance

of ion concentrations across membranes, the initial

phases of catabolic pathways, cell replication, mech-

anical function, etc. The large amount of energy

released by the hydrolysis of ATP (approximately

7 kcal mol

1

under cellular conditions) is captured

by coupling its hydrolysis to other chemical reactions

to drive them to completion.

0002The energy captured as ATP equivalents is one end

of a long chain of digestive and metabolic processes

that starts as the gross energy of the macronutrients in

ingested food (Figure 1). Significant losses in energy

occur during the digestive process. These can be

identified and quantified as losses due to incomplete

digestion and absorption (loss in fecal energy to

give apparent digestible energy; DE

app

), losses to fer-

mentation gases and urinary nitrogen energy (to

give apparent metabolizable energy), as well as

losses associated with the digestive process itself

and with the absorption and storage of food (to give

net energy). Calculating the metabolizable energy

from a food requires the collection of all excreta

(including methane and hydrogen gasses). For sub-

stances that are fermented, extra information is also

required as discussed later in this chapter. Net energy

values are more difficult to estimate and require some

assumptions about digestive processes (see section on

Factorial Models of Fermentation, eqn (19)).

0003Metabolizable energy values are the commonly

reported food energy values found on product labels.

These were initially determined in the 1900s by a

German scientist who was a pioneer in this area. To

honor his name, these factors are commonly referred to

as ‘Atwater factors.’ Both general and specific Atwater

factors exist. The specific values were measured in

individual foods. The general values (4 for carbohy-

drate, 9 for fat, 4 for protein, and 7 for alcohol) were

calculated from the specific Atwater factors using

tbl0004 Table 4 Different fiber concentrates of food residues

Concentrate Dietary fiber content

(gper100 g dry weight)

Beer draff 60–82

Maize bran 42–79

Soy bran 67–80

Pea seeds 83–90

Sugar-beet fiber 67–72

Citrus fiber, orange fiber 50–60

Cacao seeds 55

Carrot husks 60–68

1850 DIETARY FIBER/Energy Value

average intakes of mixed diets. The more modern

food energy values, as contained in tables such as

the US Department of Agriculture Handbook #8,

represent specific Atwater factors as modified by

Merrill and Watt. Although we refer to these factors

as metabolizable energy values for macronutrients,

the values for carbohydrate and fat are, in reality,

app

values. This is because urinary and gaseous

energy losses were considered as insignificant for

these macronutrients. In the case of the protein

Atwater factors, urinary energy losses were taken into

account, but gaseous energy losses were, again, as-

sumed to be negligible. The Atwater factors for fat,

protein, and carbohydrate represent the total energy

available for metabolism, and this heat should be

equivalent to the energy measured with a whole-body

calorimeter.

0004 Digestible sugars, fats, and protein are readily

absorbed in the small intestine by physiological and

biochemical processes that capture the maximum

energy from these food components. These macronu-

trients are virtually completely absorbed by the small

intestine with little energy finding its way into the

large intestine. In fact, with diets high in digestible

sugars, fats, and protein, most of the energy entering

the large intestine comes from small intestine

secretions and from the sloughing off of dead intes-

tinal cells. It is the relative simplicity of the digestive

process coupled with the high degree of absorption

that allows the comparatively easy metabolic energy

calculation for these macronutrients.

0035Not all food components are readily digested

and absorbed in the small intestine. Nonstarch long-

chain polysaccharides such as those associated with

plant cell walls are not digested by human enzymatic

secretions. Many other carbohydrates are not well

absorbed by the small intestine. These pass into the

large intestine where they are anaerobically fer-

mented by the bacterial population that resides

there. For sake of clarity, the terms ‘undigested’ and

‘resistant’ will be used here to refer to the degradation

processes occurring in the upper gastrointestinal tract

(mostly by host digestive enzymes), and the term

‘fermentation’ and related words will be used to

refer to the bacterial degradation processes occurring

in the lower gastrointestinal tract. The energy losses

associated with fermentation are not as readily

measured, and so calculation of the metabolizable

energy for fermentable food components is not as

straightforward as it is for sugars, fats and protein.

The present chapter outlines different methods

for obtaining the metabolizable energy of food

components not digested and absorbed in the small

intestine.

0005A short note about the methods used to determine

macronutrient content of foods is in order. In some

countries like the USA and Canada, the carbohydrate

content is determined by subtracting the fat, protein,

water, and ash content of the food from the total

weight (difference method). This measurement in-

cludes both ‘available carbohydrate’ (i.e., carbohy-

drate that can be digested in the small intestine) as

well as dietary fiber. Other countries, such as Great

Britain and Australia, measure ‘available carbohy-

drate’ directly using chromatographic methods.

The carbohydrate values from this latter method do

not include dietary fiber. Specific Atwater factors

used in the USA and Canada take into account

some (but not all) of the energy losses associated

with dietary fiber because they rely on the apparent

digestibility of carbohydrate to calculate food

energy. This approach overestimates energyavailabil-

ity from mixed diets. In Europe, the general Atwater

factor for carbohydrate (4kcalg

1

, 16.7kJ) is used

to calculate carbohydrate energy after subtracting

the dietary fiber content. This method underestimates

the energy of mixed diets. When ‘available carbohy-

drate’ is measured directly, one must also measure

dietary fiber and use a dietary fiber-specific energy

value to calculate the energy contribution from diet-

ary fiber.

Ingested energy

Fecal energy

Apparent digestible energy

Gaseous energy

Urinary energy

Apparent metabolizable energy

Waste formation and excretion

Digestive processes

Heat of fermentation

Surface energy

Thermogenesis

(obligatory and nonobligatory)

Net energy

Retained energy

Milk production

Reproduction

Maintenance energy

Locomotory activity

Thermal regulation

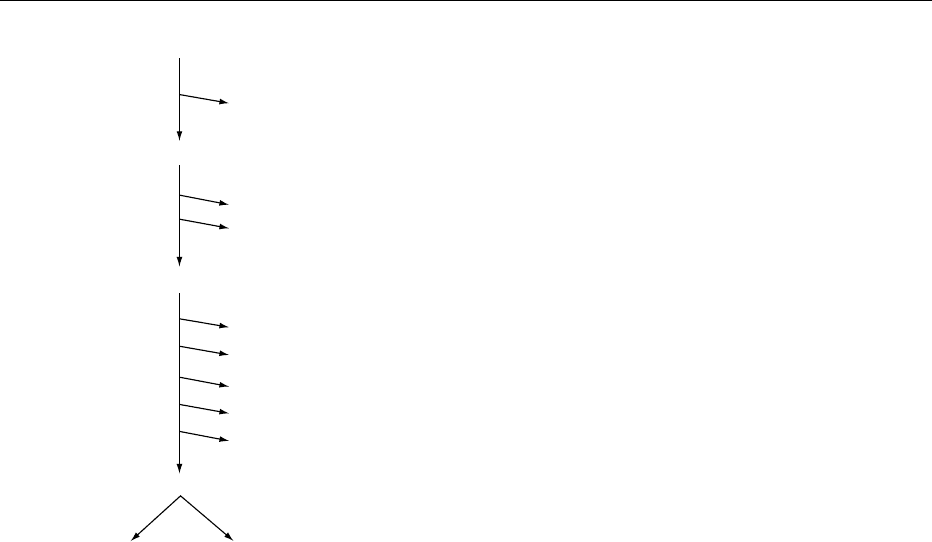
fig0001 Figure 1 Diagrammatic representation of the energy losses

associated with various stages of digestion and the resulting

determined energy values.

DIETARY FIBER/Energy Value 1851

Digestible Energy and Metabolizable

Energy: Calculations

Digestible Energy

000 6 As noted above, the digestible energy of a substance is

the difference between the ingested energy and the

energy excreted in the feces. This is, in principle, a

simple definition but gives rise to two different digest-

ible energy values depending on how the measure-

ment is made: the apparent digestible energy and the

partial digestible energy. We begin by defining IM

the ingested mass of a measured substance FM

as the

amount of the substance of interest. This substance

can represent any dietary material but for the pur-

poses of this article, we will assume that it escapes

digestion in the small intestine and reaches the large

intestine where it may (or may not) be fermented. If

we further define recovered in the feces, the apparent

digestibility (D

app

) can be calculated as:

app

¼ðIM

 FM

Þ=IM

:ð1Þ

The apparent digestible energy value (DE

app

)is

simply the apparent digestibility multiplied by the

heat of combustion ( DH

) of the material:

app

¼ D

app

H

:ð2Þ

0007 The DE

app

takes into account only the energy loss

associated with incomplete digestion of a single food

component and not any potential interaction of the

component with macronutrients. If the food component

is fermented, part of its energy value will be captured

and utilized by the host, part of the remaining energy

will appear in the feces, and part will be lost to other

processes (discussed below). Thus, the DE

app

overesti-

mates the true digestible energy.

0008 Partial digestible energy values (DE

part

) describe

the influence of a dietary substance on the energy

digestibility of the whole diet. As such, they represent

a more complete picture of the energy intake and

excretion associated with ingestion of a particular

food component. Calculating DE

part

values requires

measurement of overall energy balance. The first step

is to define the energy digestibility (D

energy

) of the

overall diet as:

energy

¼ðIE

 FE Þ=IE

, ð3Þ

where IE

is the total ingested energy, and FE is the

fecal energy. Partial digestible energy values are deter-

mined by adding a varying amount of a food com-

ponent (asa supplement) to an unchanging basal diet.

The energy digestibilities of the unsupplemented and

supplemented diets are then determined (eqn (3)) and

are plotted as a function of the fraction of ingested

energy that is derived from the supplement (IE

/IE

Ifthe supplement has a lower digestibility than that of

the overall diet, the graph should be a straight line

with a negative slope – the energy digestibility of the

overall diet will decrease with increasing amounts of

the supplement. The partial indigestibility of the sup-

plement (S

) is given by:

¼ D

energy

=ðIE

=IE

Þþð1  D

energy,0

Þ, ð4Þ

where D

energy,0

is the energy digestibility of the basal

(unsupplemented) diet. The DE

part

is obtained by

multiplying the partial digestibility (1  the partial

indigestibility) of the supplement by its heat of com-

bustion.

part

¼ H

ð1  S

Þ: ð5Þ

0009Partial and apparent digestibilities can differ by

a considerable amount. In general, DE

part

<DE

app

because DE

part

includes energy losses that are

not accounted for by DE

app

measurements. This is

illustrated by an experiment that compared the

app

and DE

part

values for the fiber component of

hard red wheat bran (bran is approximately 40%

fiber). Measurement of the diet and fecal fiber

content as well as the diet and fecal energy content

gaveaDE

app

¼1.82+ 0.17kcalg

1

(7.6kJg

1

)anda

part

¼0.45+ 0.10kcalg

1

(1.9kJg

1

). The DE

app

value shows that, for every gram of wheat bran fiber

ingested, 4.151.82¼2.33kcal (9.7kJ) of energy

was lost to feces in the form of fiber. The DE

part

value shows that 1.820.45¼1.37kcal (5.8kJ) of

extra energy was lost to the feces in addition to that

lost from fecal fiber excretion. Thus, only 0.45/

1.82100%¼25% of the energy of the fermented

wheat fiber was potentially retained by the rats (see

below for other calculations on the metabolizable

energy value of wheat fiber). These calculations

show the importance of determining the total energy

losses in a digestion experiment involving ferment-

able substances.

Metabolizable Energy

0010Like digestible energy values, it is possible to define

two different metabolizable energy values. Livesey

(1993) calls the Atwater metabolizable energy values

‘apparent metabolizable energy’ values (ME

app

) be-

cause they do not take into account all the energy

losses normally associated with metabolizable energy

measurements:

ME ¼ IE ðFE þ UE þ GEÞð6Þ

where UE is the urinary energy and GE is the gaseous

energy. If a strict parallel between digestible energy

and metabolizable energy is to be made, one could

also define ME

app

as DE

app

 (UE þ GE). In essence,

1852 DIETARY FIBER/Energy Value

this is the definition used by Atwater with some as-

sumptions. For fat and carbohydrate, it was assumed

that GE and UE were negligible. For protein, it was

assumed that GE was negligible but UE was not.

0012 Partial metabolizable energy values (ME

part

) can

also be calculated in a fashion analogous to DE

part

values. In this case, we define the total energy loss as:

losses

¼ FE þ GE þ UE: ð7Þ

Once again, we define the energy metabolizability of

the total diet as:

energy

¼ðIE



losses

Þ=IE

ð8Þ

and use this value to define a partial energy ‘non-

metabolizability’ (S

¼ M

energy

=ðIE

=IE

Þþð1  M

energy,0

Þ, ð9Þ

where M

energy,0

is the energy metabolizability of the

basal (unsupplemented) diet. The ME

part

is then given

by:

part

¼ H

ð1  S

Þ: ð10Þ

Equations that minimize the error structure for ex-

perimentally determining DE

part

and ME

part

are also

available.

0013 LikeDE

part

values,ME

part

valuesdescribethe influ-

ence of a part of the diet on the energy metabolizabil-

ity of the whole diet. For protein and fat, it is thought

thatME

part

>ME

app

because fecal excretion of bacter-

ial nitrogen and fat will decrease the ME

app

value.

Note that energy losses to bacterial fat and nitrogen

are not simply due to the loss of macronutrient mass.

Energy is required for the de novo bacterial synthesis

of fat- and protein-containing macromolecular struc-

tures,whicharethenexcretedin the feces.Thisenergy

is also lost. For carbohydrates, it is thought that

part

and ME

app

are equivalent since bacteria do

not contain appreciable amounts of carbohydrate.

For carbohydrates that escape digestion in the small

intestine and are partially or completely fermented,

like those in dietary fiber, ME

app

>ME

part

because of

theextraenergylossesthatoccurduringfermentation.

This energy loss is the subject of this chapter.

Metabolizable or Net Energy?

0014 The discrepancies between ME

app

and ME

part

energy

values mean that a more accurate system for measur-

ing food metabolizable energy must be employed for

diets containing appreciable amounts of undigested

and fermented carbohydrates as the greatest differ-

ences occur with these food components. Many

methods can be used to obtain the ME values of

food components that are not digested by the small

intestine. Whole-body calorimetric measurements (in

a chamber where an individual can be placed for

experimentation) can be used to determine the energy

value directly,estimates of the metabolizability can be

obtained from mixed diets, body energy measure-

ments can be made in laboratory animals and related

back to the metabolizable energy of the food, and

theoretical calculations can provide a general model

for estimating metabolizable energy.

0015Large differences exist between the energy losses

associated with the digestion of macronutrients (fat,

carbohydrate, and protein) and undigested and fer-

mented carbohydrates. Figure 2 shows an example of

thevariousprocesses that occurinthegastrointestinal

tract during the consumption of a partially digested

andpartially fermentedfood.Because the finalenergy

value is net of all major losses of energy, many re-

searchers have called it a net metabolizable energy

(NME). This should not be confused with the net

energy values used for livestock, which represent dif-

ferent energy measurements. Also, it is not the net

energy of Figure 1, which incorporates many more

energy losses. The NME is a more practical measure

of food energy for undigested and fermented sub-

strates because it takes into account energy losses

that do not occur for fat, carbohydrate, and protein.

Use of this value would allow comparisons between

the energy values of different food components.

Mathematical Models for Undigested and

Fermented Compounds: Sugar Alcohols

0016It is technically difficult and expensive to measure all

stages of the fermentation process and determine the

Oral ingestion

Urine

Small intestine

Absorption

Available

energy

Large intestine

Feces Fermentation

Increased

bacterial

mass

Heat

and CH

Absorption

Short-chain

fatty acids

Obligatory

heat loss

fig0002Figure 2 Scheme showing various stages of digestion of a

macronutrient that is partially digested in the small intestine

and partially fermented in the large intestine. The letters repre-

sent fractions of the initial amount that proceed down various

pathways (see text).

DIETARY FIBER/Energy Value 1853

energy losses associated with each step. This is true of

human whole-body calorimetric measurements since

analysis requires a complete energy balance profile

for each subject (see below). A more simplistic ap-

proach involves the construction of a mathematical

model of the digestive events in the large intestine.

This approach has most commonly been applied to

the energy value of sugar alcohols but can also be

extended to other partially or completely fermentable

carbohydrates.

0017 Common dietary sugars (such as glucose, galact-

ose, and fructose) have a ketone or aldehyde group

that allows cyclization to a hexose or pentose ring

structure. Reduction of this carbonyl group to an

alcohol produces a sugar alcohol that cannot cyclize.

The resulting sugar alcohols are not well absorbed in

the small intestine so that a significant percentage

can pass intact into the large intestine. Isomalt (Pala-

tinit

), an equimolar mixture of two disaccharide

alcohols ( a-d-glucopyranosyl-1,1-d-mannitol and a-

d-glucopyranosyl-1,6-d-sorbitol), is an example of a

sugar alcohol that is virtually unabsorbed in the small

intestine. It passes intact into the large intestine,

where it is rapidly and completely fermented by the

bacterial population that resides there. As such, it

represents a model for a theoretical dietary fiber

that is completely fermented in the large intestine

and can be used as a basis for constructing a math-

ematical model.

0018 A good mathematical model seeks to define all

losses that occur during fermentation of ingested

material and assign reasonable factors to these losses.

Figure 2 presents the scheme for such a model.

Fermentable substances provide carbon units for

bacterial growth and reproduction and serve as

metabolizable substrates to meet the colonic bacterial

population’s energy requirements for maintenance

and growth. Four major products result from anaer-

obic colonic fermentation: increased bacterial mass

(factor a, Figure 2), heat energy loss due to fermenta-

tion (factor b, Figure 2), methane and hydrogen gas

(factor c, Figure 2), and short-chain fatty acids

(SCFAs). Humans can only use SCFAs as an energy

source. Thus, the metabolic energy yield of the sugar

alcohol has been reduced by the losses to bacterial

mass, as well as the heat and gas produced during

fermentation. It has also been reported that SCFAs

may produce significantly less ATP per gram of

absorbed SCFA than glucose, so an additional energy

loss to this difference in metabolic efficiency should

also be included (factor d, Figure 2).

0019 The fermentation model of Figure 2 can be

expressed as an equation that incorporates the four

major processes by which energy is lost during fer-

mentation. The equation describing the scheme of

Figure 2, as it applies to sugar alcohols, was first

developed for the Nutrition Council of Holland and

has been recognized by several authors as an alterna-

tive method for calculating the energy value of sugar

alcohols. For partly fermentable substances, it can be

written as:

NME ¼H

½ðA  BÞþ a ð1  A  CÞ b,

ð11Þ

where, DH

is the heat of combustion of the substance

in question, A represents the fraction of the ingested

substance absorbed in the small intestine, B repre-

sents the fraction of absorbed substance that is

metabolized, and C represents the fraction of the

ingested substance that is excreted intact in feces

(Figure 2). The factor a represents the proportion of

energy derived from fermentation of the substance. It

can be calculated from eqn (12):

a ¼ð1  a  b  c Þd :ð12Þ

The factor b was not present in the original equation

and represents the extra energy lost due to the pres-

ence of the fermentable carbohydrate. This can come

about through binding to macronutrients, changes in

osmotic balance, alterations in transit time, changes

in intestinal viscosity, inhibition of digestive enzymes,

inhibition of macronutrient uptake, or increased

sloughing of intestinal cells. As discussed above (see

section on DE

part

and ME

part

), measuring the appear-

ance of an undigested food component in the feces is

not sufficient to account for all of the energy losses

that can occur when a diet is supplemented with a

fermentable carbohydrate. In a practical sense, it is

impossible to differentiate between any ‘extra’ energy

lost to the feces (b) and the energy lost due to in-

creased bacterial mass that results from fermentation

(factor a). The solution is to set b ¼ 0 in eqn (11) and

measure total fecal output.

0020Using the mathematical model of eqns (11) and

(12) requires a knowledge of factors A, B,andC,

which are obtained from experimental evidence. For

sugaralcohols, valuesfor factorA have been obtained

from ileostomy patients or by direct sampling using

multiple lumen tubes. Factor B (the amount of sugar

alcohol metabolized) has been estimated indirectly by

measuring urinary sugar alcohol content (the amount

of sugar alcohol not metabolized).

0021In the original factorial model paper, the Nutrition

Council of Holland held a to be constant for all sugar

alcohols and estimated it at 0.5. This value included

fermentation energy losses resulting from increased

microbial mass excreted in the feces (a 0.2), loss

of H

and CH

(c ¼0.03–0.08), heat produced by

microbes (b ¼0.02–0.05), and higher heat loss during

utilization of short-chain fatty acids as compared

1854 DIETARY FIBER/Energy Value

with glucose (d ¼0.80–0.85). Summing these factors

gives a value of 0.40–0.53. The value of 0.5 was

adopted because the Nutrition Council of Holland

did not want to suggest that the value was known

with high precision (they could have used 0.46–0.47

as the average) and because they wanted to include

some energy lost to increased cell proliferation in the

large intestine caused by increased fermentation.

Thus, the slightly higher value of 0.5 was chosen as

an estimate of the total losses during fermentation. In

a later review of the value of a, it was determined that

the individual factors that contribute to a should give

avalue closerto 0.6. However, in the same review, the

author noted that the factorial procedure overesti-

mates energy values to the extent that it takes no

account of osmotic effects of the sugar alcohol.

Thus, the results of energy values calculated by the

Dutch method are probably fairly accurate. Many

researchers agree that the value of 0.5 represents a

reasonable estimate of the fermentation process.

0022 For completely fermentable substrates that are not

absorbed by the small intestine, like sugar alcohols,

the MNE value is 0.5DH

because A ¼0 and C ¼0.

As indicated above, the value of b is set to 0, and any

‘extra’ energy lost due to ingestion is incorporated

into the factor a, which has been estimated at 0.5.

Thus, for the sugar alcohol isomalt (which is com-

pletely fermented and not absorbed by the small in-

testine), the energy value is approximately 1.9kcal

1

(8kJ g

1

, approximately half the heat of combus-

tion). For completely fermentable dietary fibers, the

0.5  DH

rule of thumb represents an upper limit of

the NME since dietary fiber is known to bind fats and

cholesterol as well as inhibit carbohydrate absorp-

tion. Thus, dietary fiber may interact with other

macronutrients to decrease their metabolizable

energy values. This will give a lower NME value for

dietary fiber since there will be a higher excretion of

fecal energy.

0023When all the energy losses are included, the energy

value of dietary fiber can be small compared with the

ingested value. For example, measurements with hard

red spring wheat fiber give NME values of approxi-

mately 0.3kcalg

1

, even though the total ingested

energy is approximately 4kcalg

1

. In the case of

hard red spring wheat bran, much of the energy is

lost to excretion; wheat bran dietary fiber is incom-

pletely fermented so that approximately 60% is lost

directly to the feces. In addition, a significant loss of

energy comes from dietary fiber interactions with

macronutrients plus other losses due to the presence

of wheat bran fiber (b). Finally, energy is lost during

the fermentation process itself. The energy value of

hard red spring wheat fiber is not typical of other

dietary fibers, as shall be shown in the following

sections (Table 1).

Whole-body Calorimetric Measurements:

Lactitol as a Model

0024Indirect calorimetry (a type of whole body calorim-

etry where all gas consumption and gas exhalation

are continuously monitored) canbe used todetermine

energy expenditure through the use of equations re-

lating total O

consumption, CO

expiration, and

urinary nitrogen excretion to energy utilization. One

such equation is:

Heat production ¼ 16:175O

þ 5:021 CO

 5:987 urea N  4:5H

: ð13Þ

In addition to total energy production, indirect calor-

imetry provides information on macronutrient util-

ization through the nonprotein respiratory quotient

tbl0001 Table 1 Potential error associated with oxidation of 50 g of a carbohydrate versus fermentation and oxidation of the released short-

chain fatty acids

Substrate RQ Oxidation substrates (mol)

consumed (mol) H

eqc

kcalper 50 g

50 g of sucrose 1.00 0.146 CHO 1.753 5.012 250.6

50 g of lactitol 0.943 0.409 (CHO) þ 0.099 (fat) 1.113 (CHO) þ 0.269 (fat) 4.981 (CHO) þ4.682 (fat) 153.1

50 g of lactitol 0.943 0.508 SCFA 1.382 4.663 144.5

The table illustrates the potential error involved in measuring the apparent metabolic energy value of a completely fermentable supplement (lactitol) by

indirect calorimetry. This was done by calculating the apparent metabolic energy value using two different methods. It was assumed that lactitol was

fermented to a mixture of SCFAs followed by complete oxidation to CO

and water plus fermentation gases. In an indirect calorimeter, oxidation of SCFAs

can be mistaken as oxidation of fat and carbohydrate in a 0.195:0.808 ratio. Oxidation of these substrates would give 153.1 kcal per 50 g. Oxidation of the

SCFA directly would give 144.5 kcal per 50 g of original lactitol. Thus, an error of approximately 6% can occur when indirect calorimetry is used to

measure the metabolic energy value of lactitol. The yield of SCFAs from lactitol and the proportion of acetate, propionate, and butyrate (115.1:35.38:24.5

per 100 mol of glucose) represent weighted averages of literature values for ruminants. As such, these calculations approximate the actual error

associated with indirect calorimetry, which varies from individual to individual.

Equivalent mol of sucrose or SCFAs obtained from 50 g of substrate.

values from Livesey G and Elia M (1988) Estimation of energy expenditure, net carbohydrate utilization, and net oxidation and synthesis by indirect

calorimetry: evaluation of errors with special reference to the detailed composition of fuels. American Journal of Clinical Nutrition 47: 608–628. The H

value for glucose was used for carbohydrate and the H

value for the fat mixture of an omnivore diet were used for fat. The H

value for SCFAs

represents a weighted average.

DIETARY FIBER/Energy Value 1855