Burton T. (et. al.) Wind energy Handbook

Подождите немного. Документ загружается.

ﬂuctuations will be signiﬁcantly smaller during operation at the lower rotationa l

speed, it would be advisable to select a somewhat lower tower natural frequency

than this to minimize overall fatigue damage.

Once a satisfactory tower design – in terms of strength and natural frequency–

has been evolved for a given turbine, it is a straightforward matter to scale up the

machine to larger rotor sizes, provided all the tower dimensions are scaled

similarly, the hub-height wind speed is unchanged, and the tip speed is maintained

constant. It can be shown that in these circumstances the tower natural frequency

varies inversely with rotor diameter, as does the rotational speed of the rotor, so

that the dynamic magniﬁcation factors are unchanged. Similarly, tower stresses due

to extreme wind loading are the same as before.

The situation is less straightforward if the tower height is to be varied for a

particular turbine. Assuming, as before, that the extreme hub-height wind speed

remains the same, and that the wind loading on the tower is negligible compared

with the wind loading on the rotor, then the tower base overturning moment is

simply proportional to hub height H. Constant stresses can be maintained at the

tower base by scaling all cross section dimensions up in proportion to the cube root

of the hub height. If the same scaling is maintained all the way up the tower, then

the tower natural frequency will vary as

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

4=3

¼ 1=H

5=6

, neglect-

ing tower mass, where I

is the second mome nt of area of the tower base cross

section. Thus doubling the tower height would result in a 44 percent reduction in

natural frequency. Alternatively, if the tower base overturning moment were

assumed to vary as H

1:5

to allow for the effect of wind shear on hub-height wind

speed and the contr ibution of wind loading on the tower, then constant tower base

stresses could be maintained by scaling the cross section dimensions up by

ﬃﬃﬃﬃﬃ

.On

this basis, tower natural frequency would vary as 1=

ﬃﬃﬃﬃﬃ

. The practical conse-

quences of ‘tuning’ the tower natural frequency are discussed with respect to

tubular towers in the next section.

7.9.3 Steel tubular towers

In the absence of buckling, a waisted conical shell, with a semi angle of 458 below

the critical zone for tip clearance, would be the most efﬁcient structure for

transferring a horizontal rotor thrust acting in any direction to ground level.

However, apart from the practicalities of transport and erection, instability of thin-

walled shells in compression precludes such a design solution, and the steel tubular

towers in common use have a very modest taper. It can be noted in passin g that the

manufacture of gently tapering towers has only been made possible by the develop-

ment of increasingly sophisticated rolling techniques, and that early tu bular towers

were constructed from a series of cylindrical tubes of decreasing diameter with

short ‘adaptor’ sections welded between them.

A tapered tower is generally fabricated from a series of pairs of plates rolled into

half frusta and joined by two vertical welds. The height of each frustum so formed

is limited to 2 or 3 m by the capacity of the rolling equipment. Care has to be taken

in the execution of the horizontal welds to minimize local distortion, which

weakens the tower under compression loading.

TOWER 455

Assuming that a tower design with a uniform taper is to be adopted, the key

design parameters to establish are the diameter and wall thickness at the tower

base. The tower top diameter, on the other hand, is governed by the size of the yaw

bearing, and wall thicknesses at intermediate heights can generally be interpolated

between the tower base value and a sensible minimum at the top of about one

hundredth of the local radius.

The main considerations determining the tower dimensions at the base are

buckling of the shell wall in compression, strength under fatigue loading and

stiffness requirements for ‘tuning’ the natural frequency. These are dealt with in

separate sub-sections below.

As machines get larger, another important consideration is the maximum tower

base diameter that can be accommodated on the highway when tower sections are

transported overland. In the ﬂat terrain of North Germany and Denmark, this limit

is generally 4.0–4.2 m, but elsewhere it will often be less.

Design against buckling

Given perfect geometry, the strength of a cylindrical steel tube in axial compression

is the lesser of the yield strength and the elastic critical buckling stress, given by



¼ 0:605Et=r (7:68)

where r is the cylinder radius and t is the wall thickness. Yield strength governs for

r=t less than 0:605E= f

, which equates to 506 for mild steel, with f

¼ 245 MPa.

However, the presence of imperfections, particularly those introduced by welding,

means that the tower-wall compression resist ance is signiﬁcantly reduced, even at

the rela tively low tower-wall radius to thickness ratios normally adopted. There is

quite a wide disparity between the provisions of different national codes, with some

making an explicit link between compression resistance and tolerances on imperfec-

tions and others not. The recommendations produced by the European Convention

for Constructional Steelwork (ECCS, 1988) contain relatively straightforward em-

pirical rules for the design of thin-walled cylinders in compression, which are based

on sets of experimental results from several sources. These will eventually be

superseded by the provisions of Part 1–6 of Eurocode 3 ‘Supplementary rules for

shell structures’, but in the meantime the ECCS rul es relating to cylinders subject to

bending loads are set out here.

The ﬁrst step is to calculate a critical stress reduction coefﬁcient for axial loading,

, from which a parallel coefﬁcient for bending loading, Æ

, is derived:

0:83

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

1 þ 0:01r=t

for r=t , 212, Æ

0:70

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

0:1 þ 0:01r=t

for r=t . 212 (7:69)

and

¼ 0:1887 þ 0:8113Æ

(7:70)

456 COMPONENT DESIGN

These values apply if the out-of-plane deviations, w, of the cylinder measured with

either

(a) a rod of length L ¼ 4

ﬃﬃﬃﬃ

placed vertically, away from welds, or

(b) a circular template of the same length placed horizontally, away from welds, or

do not exceed 1 percent of the respective rod or template length. The value of Æ

halved if the maximum value of the imperfection ratio, w=L, is 2 percent, and may

be interpolated for intermediate values of w=L.

The buckling strength, 

, is then given in terms of the yield strength and the

elastic critical buckling stress (Equation (7.68)) as follows



¼ f

1  0:4123





0:6

for Æ



. f

=2 and 

¼ 0:75Æ



for Æ



, f

(7:71)

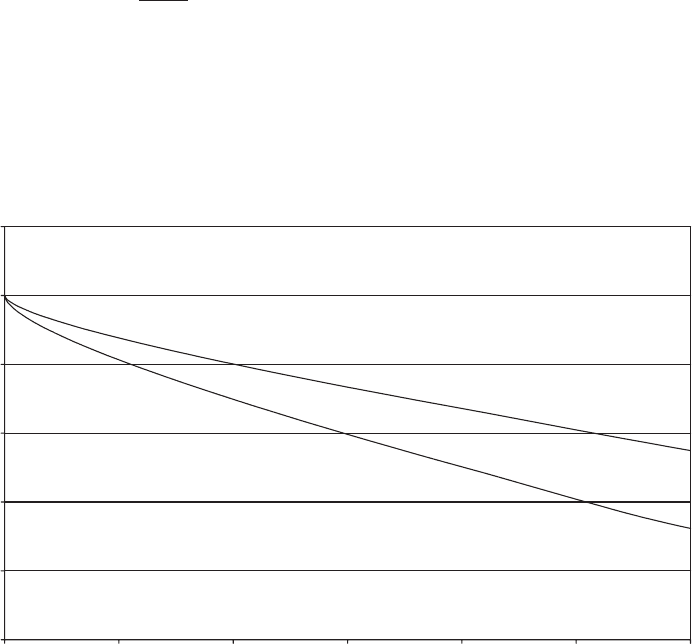
The buckling strength of a mild-steel cylinder in bending as a fraction of the yield

strength is plotted against the radius to wall-thickness ratio for imperfection ratios

(w=L) of 1 percent and 2 percent on Figure 7.39.

The effect of the choice of tower base diameter on total tower weight is best

illustrated by reference to a concrete example. Consider the design of a 50 m hub

0.2

0.4

0.6

0.8

1.2

0 50 100 150 200 250 300

Radius to thickness ratio, r /t

Buckling strength/yield strength

Imperfection to length ratio, w/L = 0.01

Imperfection to length ratio, w/L = 0.02

Figure 7.39 Buckling Strength in Bending of Thin-walled Mild-steel Cylinder for Varying

Radius to Thickness Ratio and Different Levels of Imperfection Based on ECCS Rules

TOWER 457

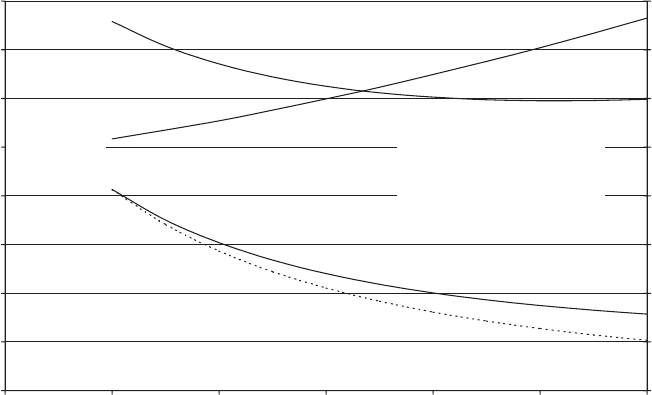
height tower in mild steel for a 60 m diameter, three-bladed, stall-regulated turbine

at a site with a 60 m/s extreme wind speed. The tower base wall thickness required

to resist the overturning moment produced by this wind speed has been calculated

for a range of tower base diameters with the aid of Eq uation (7.71) and plotted on

Figure 7.40. Corresponding tower weights have also been plotted, based on a tower

top diamete r and wall thickness of 2.25 m and 11 mm respectively and assuming an

idealized linear wall-thickness variation between tower top and tower base. It can

be seen that the tower weight reaches a minimum value at about 4.5 m diameter,

indicating that beyond this point the reduction in cross-sectional area for constant

section modulus is offset by the effects of the reducing buckling strength and the

increasing wind loading on the tower itself. The weight penalty resulting from

restricting the tower base diameter to 4.0 m for transport purposes would, in this

case, be negligible.

Fatigue design

Clear rules for the fatigue design of steel-welded structures are given in Eurocode

3, where a family of S– N curves is deﬁned for different weld details. On a log–log

plot these curves in fact consist of two straight lines, with slopes of 1/5 and 1/3 for

numbers of cycles above and below 5 3 10

respectively. In addition, there is a cut-

off limit at N ¼ 10

cycles, so that stress cycles with a stress rang e smaller than that

deﬁned at 10

cycles are deemed not to cause any fatigue damage at all.

Excluding the tower doorway (which is considered later) the critical weld details

on a steel tubular tower are likely to be at welded attachments for intermediate

2 2.5 3 3.5 4 4.5 5

Tower base diameter (m)

Tower base wall thickness (mm) and tower weight (tonnes)

0.1

0.2

0.3

0.4

0.5

0.6

0.7

0.8

Tower natural frequency (Hz)

Tower base

wall thickness (mm)

Tower weight

(tonnes)

Natural frequency

(Hz)

Tower base wall thickness to maintain

constant section modulus cf 2.5 m dia (dotted line)

Steel yield stress = 245 MPa

No of blades = 3, Blade area = 50 sq m

Tower head mass = 75 tonnes

Tower top diameter = 2.25 m

Tower top wall thickness = 11 mm

Shear exponent = 0.11

Dynamic magnification factor = 1.12

Figure 7.40 Variation in Tower Base Wall Thickness with Diameter Required for Support of

60 m Diameter Stall-regulated Wind Turbine at 50 m Height in 70 m/s Extreme Wind

458

COMPONENT DESIGN

platform and cable support members and the horizontal welds to the tower base

ﬂange and intermediate bolted ﬂanges. Assuming a full penetration butt weld is

provided, the detail category number for the horizontal welds is 71 (where the

number 71 indicates the stress range applicable at 2 3 10

cycles in MPa). The detail

category number for longitudinal welded attachments reduces as the length of the

attachment increases, but if the attachment length can be restricted to 100 mm, the

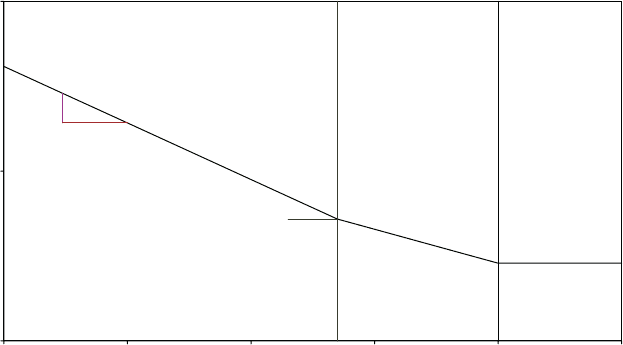
detail category number of 71 applies here as well. The S– N curve for this detail

category is shown in Figure 7.41.

Eurocode 3 lays down different partial safety factors for fatigue strength, ª

according to the consequences of fail ure and the ease of inspection. If the local

failure of a component does not lead rapidly to the failure of the structure, then it is

termed ‘fail-safe’, and ª

¼ 1:0 provided the joint detail is accessible for inspec-

tion. On the other hand, ª

¼ 1:25 for an accessible non ‘fail-safe’ component. The

factors increase to 1.15 and 1.35 respectively for joint details that are difﬁcult to

access. In a welded tubular structure, there is no barrier to the propagation of a

fatigue crack that has reached a critical length, so the welds have to be considered

non-fail-safe. Given that the tower welds are visible and accessible with relative

ease from inside the tower, a partial safety factor for fatigue strength of 1.25 would

appear reasonable.

The derivation of fatigue load spectra and the combination of stress ranges due to

and M

load spectra are discussed in Section 5.12.6.

Relative criticality of extreme and fatigue loads

The relative criticality of buckling failure (under extreme loads) and fatigue loads

depends on a variety of factors. However, fatigue is more likely to be critical on

pitch-regulated machines than on stall-regulated ones, because of the increased

100

1000

Number of cycles,

Stress range (MPa)

29 MPa

52 MPa

414

= 3

= 5

5 x 10

Figure 7.41 Eurocode 3 Fatigue Strength for Detail Category 71 (Butt-welded Joint)

TOWER 459

rotor thrust ﬂuctuations above rated and the reduced extreme loading at standstill.

Fatigue is also more likely to be critical at low wind-speed sites, because the

percentage reduction in extreme loads is less than the percentage reduction in

fatigue equivalent load.

Tuning of tower natural frequency

Considerable scope exists, at least in theory, for adjusting the tower natural

frequency to a suitable value by varying the base diameter, while maintaining the

necessary strength against extreme and fatigue loading. The effect on natural

frequency of varying tower base diameter by a factor of two, for a case where

extreme loading governs, is illustrated for a 60 m diameter stall-regulated machine

at 50 m hub-height in Figure 7.40. The frequency increases from 0.517 Hz for a

2.5 m base diame ter to 0.765 Hz for a 5.0 m diameter. Now the rotatio nal speed of a

60 m diameter turbine to yield a 60 m/s tip speed is about 19 r.p.m. If we assume

that the machine is two speed, with a lower rotational speed of 19 3 2=3 ¼

12:67 r:p:m:, then the lower blade passing frequency will be 0.633 Hz, right in the

middle of the available tower natural frequency range. Adopting a þ15%/15%

frequency exclusion zone, the tower natural frequency is required to be less than

0.538 Hz or more than 0.728 Hz. However, a frequency of 0.728 Hz would require a

diameter of about 4.7 m (without making the tower wall thicker than necessary for

the strength requirement), which is likely to be ruled out by transport considera-

tions. Thus the only strength limited design option is one with a base diameter of

2.75 m, with a weight penalty of about 10 T compared with the 60 T optimum

design, giving a natural frequency of about 0.535 Hz. Alternatively a 4 m base

diameter could be chosen and the wall thickness increased by 37% to 27.5 mm to

give a frequency of about 0.728 Hz. However, the weight penalty in this case is over

15 T.

The above case study illustrates the fact that it is not always economic to satisfy

the natural frequency requirements for a particular co mbination of turbine and hub

height. In these circumstances it may well be preferable to change the hub height.

For example, a hub height of 55 m would work much better for the case described,

with a tower base diameter of 3.5 m yielding a natural frequency of 0.535 Hz and a

tower weight of 74 T.

Joints between tower sections

Towers are normally fabricated in several sections for transport reasons, so joints

are required. Welding on site is an expensive operation, so bolted joints are almost

always used, although sleeved joints, in which each tapered tower section is

threaded over the one beneath and forced into place by jacking, have been used

successfully.

The structurally most effective joint is made with friction grip bolted splice plates

oriented vertically and sandwiching the walls of the abutting tower sections be-

tween them. Provided the grip force is adequate, the joint will not slip even under

460 COMPONENT DESIGN

the extreme load, with the result that the bolts are not subject to fatigue loads.

Unfortunately, apart from the effect of splice plates on the external appearance,

there are practical difﬁculties of joint assembly, because bolting requires the

provision of some form of personnel access on the outside of the tower. Neverthe-

less splice plates are used on some towers.

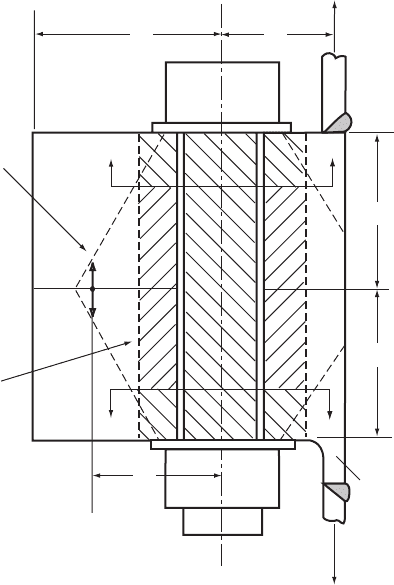
The most popular bolted arrangement is the internal ﬂanged joint as illustrated in

Figure 7.42. The ﬂanges are butt welded to the ends of the mating sections, with the

ﬂange outer edge ﬂush with the tower wall. Alternatively the ﬂange may be formed

with a stu b section of tower wall already attached. Such ﬂange s, which are termed

weld neck ﬂanges, provide a smoother transition from wall to ﬂange (as illustrated

in the lower half of Figure 7.42) and result in a higher butt-weld fatigue category.

After assembly, each bolt is torqued or tensioned to induce a preload between the

ﬂanges in order to minimize in-service bolt fatigue stresses. The bolt should be

initially sized to resist the prying force induced by the extreme tower-wall tensile

stresses – taking the fulcrum adjacent to the ﬂange inner edge – and then checked

for fatigue.

The fatigue calculation for the bolts in a ﬂanged joint depends on the relationship

between the bolt load and tower wall stress, which only remains linear while

contact is maintained over the full ﬂange width. The VDI Guideline (Verein

Boundary of

compressed

volume

Weld nec

Equivalent

cylindrical

annulus

Figure 7.42 Bolted Flange Joint

TOWER 461

Deutscher Ingenieure, 1986), VDI 2230, gives a method for calculating the bolt load

increment as a proportion of the load increm ent in the ‘tributary’ width of tower

wall under these conditions. The axial loading on the ﬂanged joint and the effect of

the moment due to the eccentricity of loading are considered separately. The axial

load is assumed to be shared between the bolt and the preloaded ﬂanges in

proportion to the stiffnesses of the load paths, which, in the case of the ﬂanges, is

based on a reduced cross-sectional area related to the volume compressed by the

preload according to

ers



 d





 d

ðÞ

x þ 1

ðÞ

1

where x ¼

ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃ

(7:72)

and d

is the washer face diameter on the bolt head and nut, d

is the bolt hole

diameter, l

is the clamping length between bolt head and nut, and D

is twice the

distance from the bolt centreline to the nearest ﬂange edge, or the bolt spacing,

whichever is the less.

The Guideline recognizes that the effective plane of introduction of the external

load will not necessarily be immediately under the bolt head or nut, but may lie

nearer the ﬂange mid-plane, giving the load paths distinguished by different cross

hatching in Figure 7.42. Stresses due to the eccentricity of the tower wall load to the

ﬂange contact area are dealt with by ordinary bending theory applied to the whole

contact area.

The VDI 2230 method outlined above no longer applies once a gap has opened

up between the ﬂanges at the outer edge. For larger ﬂuctuations in the externally

applied load, Z, the fulcrum model can be used, although it is inevitab ly conserva-

tive at low loads. The axial load, P, applied to the bolt/ﬂange combination is

calculated on the basis that a fulcrum exists at X, a distance x from the bolt, so that

P ¼ Z(1 þ b=x), and the load share between the bolt and the compressed volume of

ﬂange is calculated according to the relative stiffnesses as before.

In Figure 7.43, the two linear relationships between bolt load increment and

externally applied load are compared with experimental results for a particular test

specimen with a single ﬂange bolt. It is assumed that the planes of introduction of

the load on the bolt/ﬂange combination are immediately under the bolt head and

nut in each case. Line OA shows the VDI 2230 model, with the point A representing

the limit of its validity. Line OB shows the fulcrum model, with B representing the

point at which the preload between the ﬂanges at the position of the bolts

disappears. Thereafter, the bolt load varies as Z(1 þ b=x), i.e. along line BC for

x=a ¼ 0 :7. It may be noted from Figure 7.43 that a value of x=a of 0.8 results in better

agreement with the test results at high loads, but these are not of interest for design

purposes.

Schmidt and Neuper (1997) have proposed a more sophisticated model identiﬁed

as ‘Model C’, which combines aspects of the two models already described and

gives a bolt load characteristic consisting of the three straight lines OA, AB and BC

(see Figure 7.43). Clearly this agrees much better with the experimental results, but

it adds to the complexity of the fatigue load calculation.

Uniformity of bolt loading around the tower clearly depends on the accuracy of

the mating ﬂange surfaces. Schmidt, Winterstetter and Kramer (1999) have investi-

462 COMPONENT DESIGN

gated the effects of various imperfections using a ﬁnite-element model and made

tentative suggestions regarding permitted tolerance levels.

Tower tie-down

The tower is normally ﬁtted with a base ﬂange, which can either be attached to the

foundation by screwed rods cast into the concrete or bolted to an embedded tower

stub. This sub section is concerned with the former arrangement.

The screwed rods are normal ly anchored in some way at their base, and their

capacity to resist overturning moment is determined by the pull-out resistance of

the semi-circle of bolts on the upwind side. As this is governed by the concrete

shear strength, the rods have to be anchored quite deep into the concrete, so that

their length is typically similar to the tower base radius.

The fatigue loads in the tie-down rods can be considerably reduced by pre-

tensioning. The share of tower-wall uplift loads taken by the rods can be based on an

estimate of the relative stiffnesses of the rod and the loaded volume of the concrete,

assuming a dispersion angle of about 308 in the radial direction. The screwed rods

should be sheathed, so that the pre-tension is applied over the full length.

Tower doorways

A doorway is required for access at or near the tower base, and additional

doorways are sometimes required for a transformer in the tower base or for

0.1

0.2

0.3

0.4

0.5

0.6

0.7

0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8

Externally applied load/Bolt preload Z /F

Bolt load increment/Bolt preload (F-F

)/F

Open joint model

with x = 0.7a

Open joint model

with x = 0.8a

Line OA: VDI 2230 model - applicable before flange separation begins

Line OB: Fulcrum model with bolt load share in proportion to VDI 2230 stiffnesses

Line OABC: Schmidt and Neuper Model C

Line BC: Open joint model with x = 0.7a

Heavy line: Experimental results

Joint Geometry:

a = 50 mm, b = 45 mm

t = 50 mm, bolt diameter = 30 mm

bolt spacing = 90 mm

Figure 7.43 Flange Joint Bolt Load Variation with Externally Applied Load – Experimental

Results and Engineering Models Compared

TOWER 463

maintenance access to the blade tip mechanism. Typically they have vertical sides

with sem i-circular ends at top and bottom. Vertical stiffeners have to be provided

as standard down each side to compensate for the missing section of wall and to

resist compression buckling, but attention has to be paid to the weld detail at the

stiffener ends, where stress concentration due to the opening is likely to be an

additional factor.

The weld detail at the stiffener end can be eliminated by reinforcing the inside

edge of the doorway with a continuous ﬂange all the way round. The detail

category of the ﬂange to tower wall butt weld under transverse loading is then 71,

but there is no stress concentration factor to contend with at the top and bottom of

the doorway.

7.9.4 Steel lattice towers

Steel lattice towers are usually assembled from angle sections, with bolting used for

attaching the bracing members to the legs and splicing the leg sections together.

Typically the towers are square in plan with four legs, facilitating the attachment of

the bracing members.

One of the advantages of lattice towers is that material savings can be obtained

by splaying the legs widely apart at the base, without jeopardizing stability or

posing transport problems. The latitude for doing this higher up is limited by tip

clearance considerations, so waisted tower designs are common. A more elegant

tower design results if the legs are rolled to a gentle concave curve, however.

The loads in the legs (or ‘chords’) res ult from the tower bending moments, while

the loads in the bracing (or ‘web’) members result from a combination of tower

shear and torsional loads. In eac h case member buckling under extreme loads has

to be considered, and fatigue loading at the joints. Two devices are employed to

improve member stability: the web members are arranged as pairs of intersecting

diagonals rather than adopting a single triangulated system, so that the tension

diagonal can stabilize the compression diagonal at each intersection, and the web/

chord intersection points on either side of each chord member are staggered

vertically to reduce the spacing of chord supports restraining ﬂexure about the

minor axis. Note that care with detailing is needed at the waist, if present, in order

to ensure adequate lateral restraint for the chords at the change of direction.

Fatigue loading of bolts is avoided by the use of friction grip bolts. Accordingly

galvanizing is normally used for corrosion protection rather than painting, in order

to achieve an adequate coefﬁcient of friction.

7.10 Foundations

The design of wind-turbine foundations is largely driven by the tower base over-

turning moment under extreme wind conditions. A variety of slab, multi-pile and

mono-pile solutions have been adopted for tubular towers, and these are discussed

in turn below.

464 COMPONENT DESIGN