Brewster H.D. Mathematical Physics
Подождите немного. Документ загружается.


32
Mathematical
Basics
1={xE
JRnlak~xk<hk,k=l,
...
,n}
for some a
k
< h
k
•
The measure
of
the set I is defined to be
J.L(l)
= (hI - a
I
) ....
(h
n
-
an):
The Lebesgue integral
of
a characteristic function
of
the set I is defined
fXl
dx=
J.L(I)
Definition: A fiinite linear combination
of
characteristic functions
of
semi open intervals
is called a step function.
Definition: The Lebesgue integral
of
a step function is defined by linearity
N N
fI
CJ.kX1k
dx = I
CJ.kJ.L(Ik)
k=I k=I
Definition: A
function!
JRn
~
JR
is Lebesgue integrable if:3 a sequence
of
step functions
Uk}
such that
00
f=
Ifk,
k=I
which means that two conditions are satisfied
•
00
00
•
f(x)
=
Ifk(x)
'v'x
E
JRn
such that
Ilfk(X)1
<
00
k=I
k=l
The Lebesgue integral
offis
then defined
by
00
ff
dx
= I
ffk
dx
k=I
Proposition: The space,
Ll(JRn),
of
all Lebesgue integrable functions on
Rn
is a vector space and J is a linear functional on it.
Theorem:
Iff,
g E
Ll(JRn)
andf~
g, then J fdx
~
Jgdx.
Iff
E
Ll(JRn),
then
lIf
E
Ll(JRn)
and
If
dxl
~
itdx
~
Jgdx.
Theorem:
If
Uk}
is a sequence
of
integrable functions and

Mathematical
Basics
33
then
Definition: The LI-norm in
LICll~n)
is
defined by
Illil
=
JIJI
dx
Definition: A function f is called a null function is it is integrable and
Illil
=
O.
Two functionsfand
g are said to be equivalent
iff-
g is a null function.
Definition: The equivalence class
off
E
LIC]Rn),
denoted by
[I),
is
the
set
of
all functions equivalent to f
Remark: Strictly speaking, to make L
IC]Rn)
a normed space one has to
consider instead
of
functions the classes
of
equivalent functions.
Definition: A set X c
]Rn
is called a null set
Cor
a set
of
measure zero)
if
its characteristic function is a null function.
Theorem:
• Every countable set is a null set.
• A countable union
of
null sets is a null set.
• Every subset
of
a null
set
is a null set.
Definition:
Two
integrable
functions,/,
gELIC]Rn),
are said to be equal
almost
everywhere,
f = g
a.e.,
if
the
set
of
all
x E
Rn
for
which
fCx)
*"
g(x) is a null set.
Theorem:
f=
ga.e
~
IIf
-gll=
Jlf
-gl=O
Theorem:
The
space
LIC]Rn)
is complete.
HILBERT
SPACES
GEOMETRY
OF
HILBERT
SPACE
Definition: A complex vector space V is called an inner product space
Cor
a pre-Hilbert space
if
there is a mapping
C.,.):
V
$;
V 4
C,
called an inner
product,
that
satisfies: Vx,y, Z E
V,
Va
E C:
•
Cx,
x)
~
0
•
Cx,
x)
= 0
¢:}
x = 0
• (x, y + z) =
Cx,
y)
+ (x, z)
• (x,
$;y)
=
$;Cx,
y)
• (x,
y)
= (y, x)*
Definition:
Let
V be an inner product pace.
•
Two
vectors x, y E V are said to be orthogonal
if
Cx,
y)
= 0,
•
A collection,
{xd:1'
of
vectors in V is called an orthonormal set
if
(Xi'
x)
=
By,
i.e.
(Xi'
x)
= 1
if
i = j and
(Xi'
x)
= 0
if
i
*"
j.
Theorem: Every inner product space is a normed linear space with the

34
Mathematical
Basics
norm
IIxll
= ,J(x,
x)
and
a
metric
space
with
the
metric
d(x,
y)
=,J(x-
y,x-
y).
Theorem: (Pythagorean Theorem) Let V be an inner product space and
be an orthonormal set in
V.
Then
"Iix
e V
N N 2
IIxl12
=
LI(x,x
n
)1
2
+
x-
L(X,Xn)X
n
n=1 n=1
Theorem: (Bessel inequality) Let
Vbe
an inner product space and {xn}
:=1
be an orthonormal set in
V.
Then "Iix e V
Theorem: (Schwarz inequality)
Let
V be an inner product space. Then
"Iix,ye V
l(x,y)1
~
IlxllllYll·
Theorem: (Parallelogram Law)
Let
V be an inner product space. Then
"Iix,yeV
IIx
+ yll2 +
IIx
-
yll2
=
211xl12
+
211Y112.
Definition: A sequence {xn}
of
vectors in an inner product space V is
called strongly convergent to
xe
V,
denoted by
xn
w)
x,
if
Ilxnll
b~O)
0
and weakly convergent to x e
V,
denoted by XII
w)
x,
if
(xn-x,y)
n-+O
)0
"IiyeV.
Theorem:
•
•
Xn-7X
Definition: A complete inner product space is called a Hilbert space.
Definition: A linear transformation
U:
HI
-7
H2
from a Hilbert space
HI
onto the Hilbert space
H2
is called unitary
if
if
it preserves the inner product,
i.e.
"Iix,
ye
HI
(Ux, UY)m =
(x,y)H
I
·
Definition: Two Hilbert spaces
HI
and
H2
are said to be isomorphic
if
there is a unitary linear transformation U from
HI
onto H
2
.

Mathematical
Basics
35
Definition:
Let
HI
and
H2
be
Hilbert
spaces.
The
direct
sum
HI
$
H2
of
Hilbert spaces
HI
and
H2
is the set
of
ordered pairs z =
(x,
y) with
XE
HI
and yE
H2
with inner product
(zI'
z2)HlE9
m = (xl' X
2
)Hl +
(YI'
Y2)H2
EXAMPLES
OF
HILBERT
SPACES
Finite Dimensional Vectors.
eN
is the space
of
N-tuples X = (xl' ... , X N)
of
complex numbers. It is a Hilbert space with the inner product
N
(X,y) =
LX;Yn.
n=I
Square Summable Sequences
of
Complex Numbers.
P.
is the space
of
sequences
of
complex numbers X =
{xn};;':1
such that
00
Llxnl2
<00.
n=I
It is a Hilbert space with the inner product
00
(x,
y)
=
LX;Yn
n=I
Square Integrable Functions on R
L2(R)
is the space
of
complex valued
functions such
thar
kl
f
(X)2I
dx
<
00
It is a Hilbert space with the inner product
(f,
g)
=
k.f"
(x)g(x)dx
Square Integrable Functions on
Rn.
Let be
an
open set in
Rn
(in particular,
can be the whole
]Rn). The space
L2(a)
is the set
of
complex valued functions
such that
1If(x)1
2
dx <
00
where x =
(xl'
... ' x
n
)
E and dx = dx
I
..• dx
n
.
It is a Hilbert space with the inner
product .
(f, g) =
bf*(x)g(x)dx
Square Integrable Vector Valued Functions. Let
be
an
open set in
]Rn
(in particular, a can be the whole
]Rn)
and V be a finite-dimensional vector
space.
The
space
L2(
V,
a)
is
the
set
of
vector
valued
functions
f=
ifI,···,f
N
)
on a such that
N r 2
Lhl/;(x)1
dx <
00.
;=1

36
It is a Hilbert space with the inner product
N
(f,
g)
=
I1h\x)g;(x)dx
i=1
Mathematical
Basics
Sobolev Spaces: Let Q be an open set
in
~n
(in particular, Q can be the
whole
JRn)
and
Va
finite-dimensional complex vector space. Let cm(V,
Q)
be
the space
of
complex vector valued functions that have partial derivatives
of
all orders less or equal to
m.
Let a =
(a
l
, ... , an)' a E
N,
be
a multiindex
of
nonnegative integers, a
i
~
0, and let
1$;1
= a
l
+
...
+ an. Define
ThenfE
em
(V,
Q)
iff
IDo.
h(x)l<
00
Va, lal:s;
m,
Vi
=
1,
...
,N,
Vx EO.
The space
Jf1l(
V,
0)
is the space
of
complex vector valued functions such
that
DO.
f E L
2(
V,
0)
Va,
lal
$;
m, i.e. such that
N 2
I
11
D
o.
h(X)1
dx<
00
Va, lal:s;
m.
i=1
It
is
a Hilbert space with the inner product
N
(f,
g)
= I I 1
(DO.
hex»~
*
DO.
gj(x)dx
o.,lalsm
;=1
Remark. More precisely, the Sobolev space Jf1l(V,
0)
is the completion
of
the space defined above.
PROJECTION THEOREM
Definition: Let
Mbe
a closed subspace
of
a Hilbert space H. The set,
AP-,
of
vectors
in
H which are orthogonal to M is called the othogonal complement
ofM.
Theorem: A closed subspace
of
a Hilbert space and its orthogonal
complement are Hilbert spaces.
Theorem: Let M be a closed subspace
of
a Hilbert space H. Then
Vx E H 3 a unique element Z E M closest to
x.
Theorem: (Projection Theorem) Let M be a closed subspace
of
a Hilbert
space
H.
Then
Vx
E H 3 Z E M
and
3w
E
M.l..
such
that
x = Z +
w.
That
is
H=MtBM.l..

Mathematical
Basics
37
Remark: The set, L(H,
H~,
oflinear
transformations from a Hilbert space
H to
H'
is a Banach space under the norm
liT
II
= sup
\\Tx\\H'
Il
x
ll
H
=l
Definition: The space
11*
= L(H,
C)
of
linear transformations from a
Hilbert space
H to C
is
called the dual space
of
H.
The elements
of
H* are
called continuous linear functionals.
Theorem: (Riesz Lemma) Let H be a Hilbert pace.
Then
'\IT E
H*
:3YrE
H such that '\Ix E H
T (x) =
(yp
x), and
liT
II
H
* =
IlYr
IIH
ORTHONORMAL
BASES
Definition: Let S
be
an orthonormal set
in
a Hilbert pace H.
If
there
is
no
other orthonormal set that contains S as a proper. subset, then S is called
orthonormal basis (or complete orthonormal system) for
H.
Theorem:
Every Hilbert space has an othonormal basis.
Theorem: Let S = L
(xa,y)xa
be an orthonormal basis for a Hilbert
aeA
space
H.
Then '\Iy E H
Definition: Let S ={xa}aeA be an orthonormal basis for a Hilbert space
H. The coefficients
(xa'
y)
are called the Fourier coefficients
of
yE
H with
respect to the basis
S.
Definition: A metric space which has a countable dense subset is said to
be separable.
Theorem: A Hilbert space H
is
separable iff it has a countable orthonormal
basis
S.
If
S contains finite number, N,
of
elements, then H is isomorphic to
CN.
If
S contains countably many elemt:nts, then H
is
isomorphic to
P.
TENSOR PRODUCTS OF
HILBERT
SPACES
Let
HI
and
H2
b Hilbert spaces. For each
<PIE
HI
and
<P2
E
H2
let
<PI
®
<P2
denote the conjugate bilinear form on
HI
X
H2
defined by
(<PI
®
<P2
) (
\jJ
1 ,
\jJ
2 ) =
(\jJ
1 ,
<PI
) HI (\jJ 2 ,
<P2
) H 2
where
"'1
E
HI
and
"'2
EH
2
·
Let E be the set
of
finite linear combinations
of
such bilinear forms. An inner product on E can be defined by
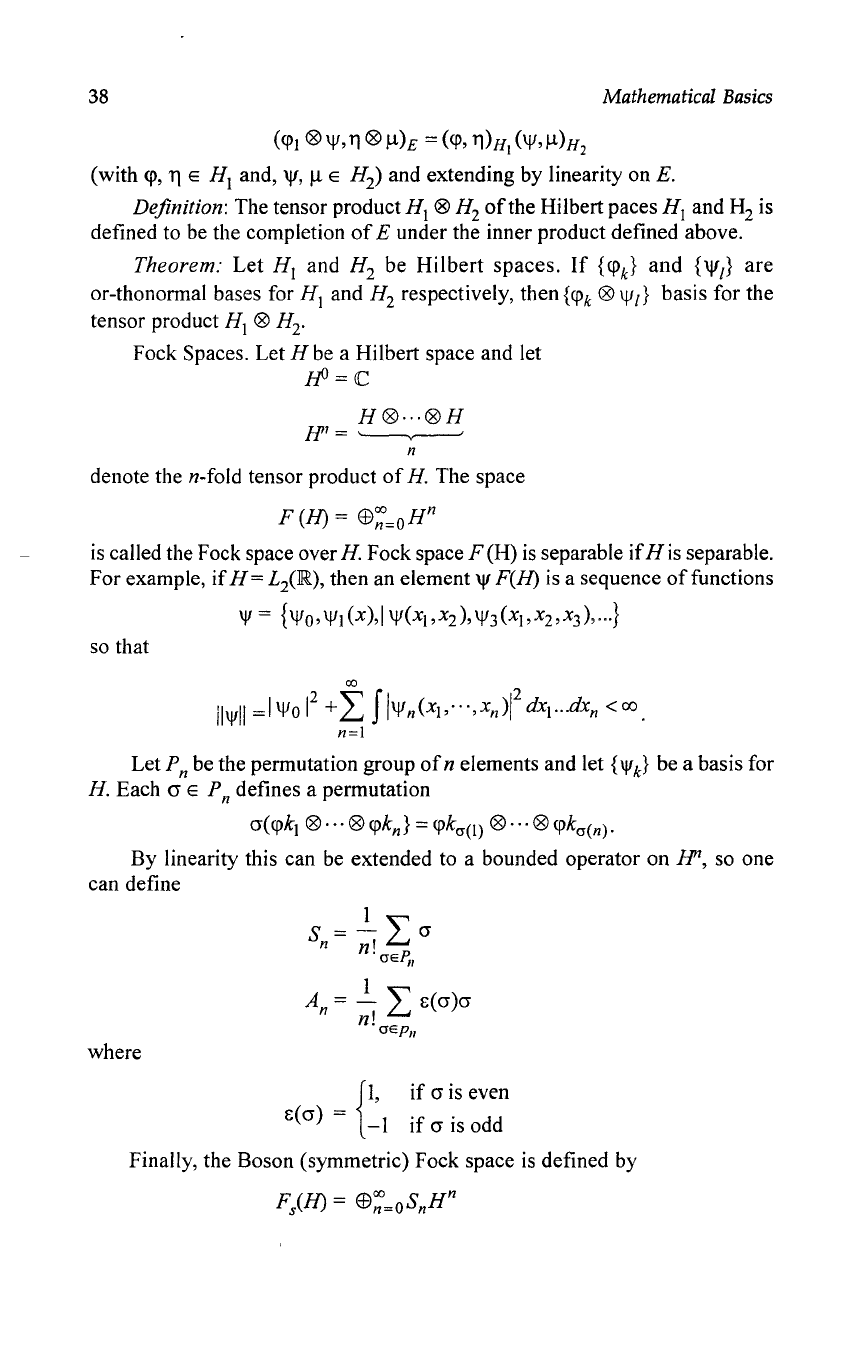
38
Mathematical
Basics
(<PI
®\jf,T)®)l)£
=(<P,T))H
t
(\jf,)l)H
2
(with
<p,
11
E
HI
and, 'If,
Jl
E H
2
) and extending by linearity on E.
Definition: The tensor product
HI
®
H2
of
the Hilbert paces
HI
and
H2
is
defined to be the completion
of
E under the inner product defined above.
Theorem:
Let
HI and
H2
be
Hilbert
spaces.
If
{<!>k}
and
{'If
I} are
or-tho normal bases for HI and
H2
respectively, then
{<Pk
® \jf/} basis for the
tensor product HI
® H
2
.
Fock Spaces. Let H be a Hilbert space and let
lfJ = C
H®···®H
Ifl
=
'--v-----'
n
denote the n-fold tensor product
of
H. The space
F
(ll)
=
(B~=oHn
is called the Fock space over
H.
Fock space F (H)
is
separable
if
H
is
separable.
For example,
if
H=
LiR),
then an element 'If
F(ll)
is a sequence
of
functions
'I' = {\jfo,
'1'1
(x),1
\jf(xI,
X2),
'1'3
(XI'
x2,
X3)'
...
}
so that
00
11'1'11
=1'1'0
12
+ I
fl\jfn(XI,··,x
n
)1
2
dXI···dxn
<00.
n=I
Let P n be the permutation group
of
n elements and let {'I'
k}
be a basis for
H. Each
cr
E P n defines a permutation
cr(<PkI
® ... ®
<pk
n
}
=
<Pkcr(I)
® ... ®
<pkcr(n).
By
linearity this can be extended to a bounded operator on Jl'l, so one
can define
s=~Icr
n
n'
.
crep'/
A =
~
"
E(cr)cr
n , .L.
n.
creplI
where
_ {I,
if
cr
is even
E(cr)-
-1
ifcrisodd
Finally, the Boson (symmetric) Fock space
is
defined by
Fill)
=
(B~=OSnHn

Mathematical
Basics
and the Fermion (antisymmetric) Fock space is defined by
Fill)
=
(f)~=OAnHn
39
In the case
II
=
L2(IR),
'l'nE
Sji"
is a function
of
n variables symmetric
under any permutations
of
variables,
and",
n E
A/f1
is
a function
of
n variables
that is odd function under interchanges
of
any two variables.
ASYMPTOTIC
EXPANSIONS
ASYMPTOTIC
ESTIMATES
Let M be a set
of
real or complex numbers with a limit point
a.
Letj,
g:
M
-4
IR
(orj,g: M
-4
C) be some functions on
M.
Definition: The following are asymptotic estimates
•
I(x)
- g(x) (x
-4
a, x E Ai)
If
lim
I(x)
= I
x-+a.
xeM
g(x)
.
•
I(x)
= o(g(x)) (x
-4
a,
x E Ai)
.
I(x)
If
11m
--
= 0
x-+a,
xeM
g(x)
.
•
I(x)
= o(g(x)) (x E Ai)
If3C:
l/{x)1
~
CIg(x)1
\I
x E M
•
I(x)
= O(g(x)) (x
-4
a,
XE
M)
If
3C
and a neighborhood U
of
a such that:
If(x)1
~
qg(x)1
\Ix
E M n U
ASYMPTOTIC SEQUENCES
Definition: Let
<l'n:
M
-4
IR,
n E N, and a be a limit point
of
M.
Let
<l'n(x)
'*
0 in a neighborhood
Un
of
a.
The the sequence
{<I'n}
is called
asymptotic sequence at
x
-4
a,
x E M
if
\In
E N
<l'n+l(x)
=
o(<I'n(x))
(x
-4
a,
x E M)
Examples:
I. Power asymptotic sequences
(a)
{(x
- a)n}, x
-4
a.
(b)
{x-
n
},
x
-4
00.
2.
Let {An} be a decreasing sequence
of
real numbers, i.e.
An
<
An+l'
and let 0 < E
~
n/2. Then the sequence
{e
AnZ
} , z
-400,
larg
zl
S
~
- E
is an asymptotic sequence.

40
Mathematical
Basics
Properties
of
Asymptotic Sequences
I.
Any
subsequence
of
an
asymptotic sequence
is
an asymptotic
sequence.
2.
Let/(x):;:.
0 for x E M in some neighborhood
of
a
and
{<Pn}
be
an asymptotic sequence
at
x
~
a,
x E
M.
Then the sequence
{fix)
<Pn(x)}
is
an asymptotic sequence as x
~
a,
XE
M.
3.
Let {<pn(x)},{\Jfn(x)} be asymptotic sequences as x
~
a, x E M.
Then the sequence {<pix)},{\Jfn(x)}
is
an asymptotic sequence as x
~a,XE
M.
Asymptotic Series
Let!
M
~
lR
and
a be a limit point
of
M.
Definition: Let
{<Pn}
be an asymptotic sequence as x
~
a, x E M. We say
that
the
function/is
expanded in
an
asymptotic series
00
lex)
- L
an<pn(x),
(x
~
a, x
EM),
n=O
where
an
are constants,
if
\iN
~
0
00
RJlx)
=/(x)-
L
an<Pn(x)
=o(<PN(x», (x
~
a,
XE
M)
n=O
This series
is
called asymptotic expansion
of
the
function f with
respect
to
the asympotic sequence
{<Pn}'
RN
(x)
is
called the rest
term
of
the asymptotic series.
Remarks:
I. The condition
RN
(x) =
o(<p
N
(x»
means, in particular, that
lim
RN(x) = 0 for any fixed N
x~a
2.
Asymptotic series could diverge. This happens
if
lim
RN
(x)
:;:.
0 for some fixed x
N~oo
3.
There are three possibilities:
(a) Asymptotic series converges to /
(x),
(b) Asymptotic series converges
to
a function g(x)
:;:.
lex),
(c) Asymptotic series diverges.
Theorem: Asymptotic expansion
of
a function with respect
to
an
asymptotic sequence
is
unique.
Remark:
Two
different
functions
can
have
the
same
asymptotic
expansion.
For
example,f(x) =
eX
andg(x)
=
e"l:
+
e-
1/x
have the same asymptotic
expansion with respect
to
the asymptotic sequence {xn}:

Mathematical
Basics
41
Theorem:
Asymptotic
series
can
be
added
and
multiplied
by
numbers, but, cannot be multipied by asymptotic series.
Theorem: One can multiply
and
divide power asymptotic series.
Definition: Let f M X S
~
lR
be
a function
of
two variables
and
a
be a limit
point
of
M
and
{<i>n}
be
an
asymptotic sequence as x
~
a.
Let for any xed
YES
the function f
is
expanded in an asymptotic
series
00
f(x,y)
~
Lan(Y)<i>n(x),
(x~a,xEM).
n=O
This asymptotic expansion
is
called uniform with respect
to
the
parameter
YES,
if
the relation
00
RN(x,y)
=
f(x,y)-
L
an
(y)
<i>n
(x) =
O(<i>N(x»,
(x
~
a, x E
M)
n=O
is
valid uniformly with respect to
YES.
Theorem: A uniform asymptotic expansion can be integrated with respect
to the parameter term by term.
Remark: One cannot, in general, differentiate asymptotic series, neither
with respect to
x nor with respect to a parameter.
Asymptotics of
Integrals:
Weak
Singularities
Let us consider the integrals
of
the form
F (e) = r
f(x,e)dx
where
a > 0
and
e > 0 is a
small
positive
parameter.
Here
f E
('Xl([0,
a] x (0,
eoD
is
a smooth function for 0
:s;
x
:s;
a, 0 < e
:s;
eo,
with
some
eo.
Then the integral converges for e >
o.
Letfhave
a singularity when e =
0,
i.e. g(x) =
f(x,
0)
has a singularity at
some
0
:s;
x
:s;
a.
If
this singularity
is
of
power
or
logarithmic type then
we
say
that
the integral F
(e)
has a weak singularity.
This Definition
is
obviously extended for unbounded intervals. Let
F(e) = r
f(x,e)dx
where
a>
0 and e > 0
is
a small parameter. HerefE
CX'([a,
1)
x
(0,
eol)
is
a
smooth function for a
:s;
x:s;
1,0 < e
:s;
eo,
with some
eo.
Let the integral converge
for
e > 0 and diverge for e =
o.
If
the function g(x) = f (x,
0)
is
of
power
or
