Brewster H.D. Mathematical Physics
Подождите немного. Документ загружается.


62
Laplace
and
Saddle
Point Method
the minimax property
of
the contour
y*
it follows that the point
Zo
is the saddle point
of
the function Re S (z). Let z = x + iy. Since the
saddle point
is
a stationary point, then
a a
ax
ReS(zo) =
By
ReS(zo).
Then from Cauchy-Riemann conditions
it
follows that
S'(zo)
=
O.
Definition: A point
Zo
E C is called a saddle point
of
the complex valued
function
S:
C
~
C
if
S'(zo)
=
O.
2. A saddle point
Zo
is said to be
of
order n
if
S'(zo)
= ... =
S<n)(zo)
=
0,
S<n+I)
(zo)
'*
O.
3. A first order saddle point is called simple, i.e. for a simple saddle
point
S"(zo)
'*
O.
4. The number Re S
(zo)
is called the height
of
the saddle point.
To compute the asymptotics at an interior saddle point, we replace the
contour
y*
by a small arc
i1
containing the point
zOo
Then we expand the
function
S
in
the Taylor series in the neighborhood
of
Zo
and neglect the terms
of
third order and higher, i.e. we replace S by
S(z)
=S(zo)
+~S"(Zo)(Z-
zO)2
+
O«z-
zO)3).
Finally, by changing the variables and evaluating the integral by Laplace
method we obtain the asymptotics as
A.
~
00
F
(A.)
= J
-s:;zo)
A.
-1/2
exp[A.S(zo)]
!(zo)
+
O(A.
-I)]
.
The saddle point method consists
of
two parts: the toplogical part and
the analytical part.
The topological part consists
of
the deformation
of
the contour to the
minimax contour
y*
that is most suitable for asymptotical estimates. The
analytical part contains then the evaluation
of
the asymptotics over the contour
The analytical part is rather straightforward. Here one can apply the same
methods and as in the Laplace method; in many cases one can even use the
same formulas.
The topological part is usually much more complicated since it is a global
problem.
It could happen, for example, that a contour
y*
where the minimax
min.,.e
r
max
zEy
Re
S(z)
is
attained does not exist at all! Next, strictly speaking we
need to look for a contour where
min.,.e
r
maxZEY!(z)
exp[Re S (z)] is attained
what makes the problem even more complicated.
Thus,
if
one can find the minimax contour, then one can compute the

Laplace
and
Saddle
Point Method
63
asymptotics
of
F
(A)
as A
~
00.
Unfortunately, there is no simple algorithm
.that would always enable one
tOA
find the minimax contour. Nevertheless,
under certain conditions one can prove that such a contour exists and,
in
fact,
find one.
Level
Curves
of
Harmonic
Functions
Lemma: Let
S:
C
~
C be holomorphic·at
Zo
and
S'(zo)
:;c
O.
Then in a
small neighborhood
of
the point
Zo
the arcs
of
the level curves
Re
S(z)
= Re S
(zo),
1m
S(z)
=
1m
S (zo)'
are analytic curves. These curves are orthogonal at
zOo
Let
<p(z)
= S (z) S
(zo).
Since
S'(zo)
:;c
0 the function w =
<p(z)
is
is
a one-
to-one holomorphic, in fact, conformal, mapping
of
a neghborhood
of
the point
z =
Zo
onto a neighborhood
of
the point w =
O.
The inverse function z =
<p-l(w)
is holomorphic in a neighborhood
of
the origin w =
O.
Let w = u +
ivand
(u, v) =
<p-l(w).
The arc
of
the level curve Re S (z) = Re
S(zo)
is mapped onto an open
interval on the imaginary axis. It is defined by
z = (0,
v)
and is analytic. The
same is
true
for the level curve
1m
S (z) =
1m
S(zo).
It
is defined
by
z = ",(u, 0) and is analytic as well. The tangent vectors to the level curves at
Zo
are determined by
8\v(u, 0) I =
(Bw<p-l)(O),
8\v(O,V)1
=
i(Bw<p-l)
(0)
au
u=O
av
v=O
and are obviously orthogonal. One could also conlude this from the fact that
the map is conformal and, therefore, preserves the angles.
Lemma: Let
Zo
be a simple saddle point
of
the function S. Then in a small
neighborhood
of
the point
Zo
the level curve Re S (z) = Re S(zo) consists
of
two analytic curves that intersect orthogonally at the point
Zo
and separate the
neighborhood
of
Zo
in
four sectors. The signs
of
the function Re
[S
(z) -
S(zo)]
in adjacent sectors are different.
In a neighborhood
of
a simple saddle point the there is a one-to-one
holomorphic function
z = ",(w) such that ",(0) =
zO'
",'(0)
*-
0, and S (",(w» =
S(zo)
+
wZ.
In the complex plane
of
w the level curve Re S (z) = Re S (zo) takes the
form Re
wZ=
o.
Its solution consists
of
two orthogonal lines w± =
(1
± i)twith
- E < t < E that intersect at the point w =
o.
The level curves
~
= (w±) have
listed properties.
Lemma: Let
Zo
be a saddle point
of
the function S
ofbrder
n.
Then in a
small neighborhood
of
the point
Zo
the level curve Re S (z) = Re S
(Zo)
consists
of
(n + 1) analytic curves that intersect at the point
Zo
and separate the
neighborhood
of
Zo
in 2(n +
1)
sectors with angles 1t(n +
1)
at the vertex. The
signs
of
the function Re
[S
(z) - S (zo)] in adjacent sectors are different.

64
Laplace
and
Saddle
Point
Method
Definition:
Let
S be a complex valued function and y be a simple curve
with the initial point
zOo
The
curve y is called curve
of
steepest descent
of
the function Re S
if
1m
S (z) = const and Re S
(z)
< Re S
(zo)
for z E
y,
z '#
zOo
If
1m
S (z) = const and
Re
S (z) > Re S
(zo)
for z E
y,
z '#
zo'
then the curve y is called curve
of
steepest
ascent
of
the function Re S.
Lemma:
1.
If
Zo
is not a saddle point, then there is exactly one curve
of
steepest descent. -
2.
If
Zo
is a simple saddle point, then there are 2 curves
of
steepest
descent.
3.
If
Zo
is
a saddle point
of
order n, then there are (n + 1) curves
of
steepest descent.
4. In a neighborhood
of
a saddle point
Zo
in each sector
tn
which Re
[S
(z) -
S(zo)]
> 0 there is exatly one curve
of
steepest
d~scent.
This is proved by a change
of
variables in a neighborhoo</l
of
z00
Remarks:
Let
S:
D
-7
C be a nonconstant holomorphio function in a
domain
D.
Let
z =
x+iy
and
S
(z)
=
u(x,
y)
+
iv(x,
y).
Then
both
u:
D
-7lR
and
v:
D
-7lR
are harmonic functions in D. Harmonic functions do
not have maximum
or
minimum points in the interior
of
D.
They
are attained
only at the boundary
of
the domain D.
All
critical
points
of
harmonic
functions,
i.e.
the
points
where
au
=
av
= 0 are saddle points.
These are exactly the points where
S'
(z) =
O.
That
is
why
such points are
called saddle points
of
the function S. In the simplest case S =
z2
the surface u
=
x2
-
y2
is hyperbolic paraboloid (saddle).
Definition:
Two
contours
YI
and
Y2
are called equivalent
if
(j(z)exp[AS(z)]dz
= t
j(z)exp[AS(z)]dz
Lemma:
Let
S
andjbe
holormorphic functions on a finite contour
Let
the
points where
max
zEy
Re S (z) is attained are neither saddle points nor the
endpoints
of
the contour
y.
Then there is a contour y' equivalent to the contour
y and such that
max
ReS(z) < max
Re
S(z)
ZEY'
ZEY
•
Theorem:
Let
F
(A)
be a Laplace integral
if
there exists a contour
y*
such
that:
i)
it is equivalent to the contour
yand
ii)
the integral F
(A)
attains the
minimax
min
YE
r
max
zEy
Re S (z) on it. Then among the points where
maxZEY*
Re S (z) is attained there are either endpoints
of
the contour
or
saddle points
z)
such that in aneighborhood
of
Zj the contour
y*
goes through two different
sectors where
Re
S (z) < Re S
(z).

Laplace
and
Saddle
Point Method
Analytic
Part
of
Saddle
Point
Method
65
In this section
we
always assume that y is a simple smooth (or piece-wise
smooth) curve in the complex plane, which may be
y finite or infinite.
The
functions f and S are assumed to be holomorphic on
y.
Also, we assume that
the integral
F
(A.)
converges absolutely.
First
of
all,
we
have
Lemma:
Ifmax
zE
1.
Re
S(z);:::
C, then
F
(A.)
= O(e
c
A.),
(A.;:::
I).
Theorem: Let
Zo
be the initial endpoint
of
the curve
y.
Let f and S are
analytic at
zO'
Re S
(zo)
> Re S
(z)
'liz E
y,
and
S'(zo);;j:.
o.
Then, as
A.
~
00
there
is asympotic expansion
where
00
F
(A.)
- exp[AS(zo)] I
akA
-k-J
k=O
(
1
o)k[f(Z)l
a
k
= - - S'(z)
oz
S'(z)..J
Z=Zo
The proof is by integration by parts.
Theorem: Letzo be an interior point
of
the curve
y.
Letfand
S are analytic
at
zO'
Re S
(zo)
> Re S (z)
'IIzE
y.
Let
Zo
be a simple saddle point
of
S such that
in a neighborhood
of
Zo
the contour y goes through two different sectors where
Re
S (z) < Re S (zo). Then, as A
~
00
there is asympotic expansion
00
F (A) - exp[AS(zo)] I
ak
A
-
k
-
J12
k=O
The branch
of
the square root
is
choosen so that arg
~_Sw
(zo
is equal to
the angle between the positive direction
of
the tangent to the curve y at the
point
Zo
and the positive direction
of
the real axis.
In a neighborhood
of
Zo
there is a mapping z = 'V(w) such that
w
2
S('V(w»
=S(zo)-2.
After this change
of
variables and deforming the contour to the steepest
descent contour the integral becomes
F
(A.)
= exp[AS (zo)] r e-
Aw2
12
f(\jJ(w»\jJ'(w)dw+ 0(1.-
00
)
Since
bothf
and
'V
are holomorphic there
is
a Taylor expansion
00
f(\jJ(w»\jJ'(w) = I
Ck
wk.
k=O

66
Laplace
and
Saddle
Point Method
Then the coefficients a
k
are easily computed
in
terms
of
c
k
ak
=2
k
+
1/2
r(
k
+~
)c2k
Theorem:
Let
y be a finite contour and f and S be holomorphic in a
neighborhood
of
Ao
Let
max=EY
ReS(z) be attained at the points Zj and these
points are eitherothe endpoints
of
the curve or saddle points such that
in
a
neighborhood
of
a
sadodle
points the contour goes through two different sectors
where Re S (z)
< Re S
(z)
Then as A --7
00
the integral F
(A)
is
asymptotically equal to the sum
of
contributions
of
the points z)O
Remark: The integral over a small arc containing a saddle point
is
called
the contribution
of
the saddle point to the integral.
Proposition: Let f and S be holomorphic functions on and
1m
S (z) =
const on
Yo
If
has y finite number
of
saddle points, then as Jlambdal --7
00
, larg
AI
::;;
n/2
- E < n12, the asymptotic expansion
of
the integral F
(A.)
is equal to
the sum
of
contributions
of
the saddles points and the endpoints
of
the contouro

Chapter 3
Free
Fall
and Harmonic Oscillators
THE
SIMPLE
HARMONIC
OSCILLATOR
The next physical problem
of
interest
is
that
of
simple harmonic motion.
Such motion comes up
in
many places
in
physics and provides a generic first
approximation to models
of
oscillatory motion.
This
is
the beginning
of
a major thread running throughout our course.
You have seen simple harmonic motion
in
your introductory physics class.
We will review SHM (or
SHO
in
some texts) by looking at springs and pendula
(the plural
of
pendulum).
Fig. Spring-Mass System
Mass-Spring Systems
We begin with the case
of
a single block on a spring. The net force in this
case is the restoring force
of
the spring given by Hooke's Law,
Fs
=-kx,
where
k>
0
is
the spring constant. Here x
is
the elongation, or displacement..
of
the spring from equilibrium. When the displacement
is
positive, the spring
force
is
negative and when the displacement
is
negative the spring force
is
positive. We have depicted a horizontal system sitting on a frictionless surface.
A similar model can be provided for vertically oriented springs. However,
you need to account for gravity to determine the location
of
equilibrium.
Otherwise, the oscillatory motion about equilibrium
is
modelled the same.
From Newton's Second Law,
F
=mx,
we ,obtain the equation for the
motion
of
the mass on the spring.
mx
+
kx
=
o.
For now we note that two solutions
of
this equation are given by
68
where
Free
Fall
and
Harmonic
Oscillators
x(t) = A cos
cot
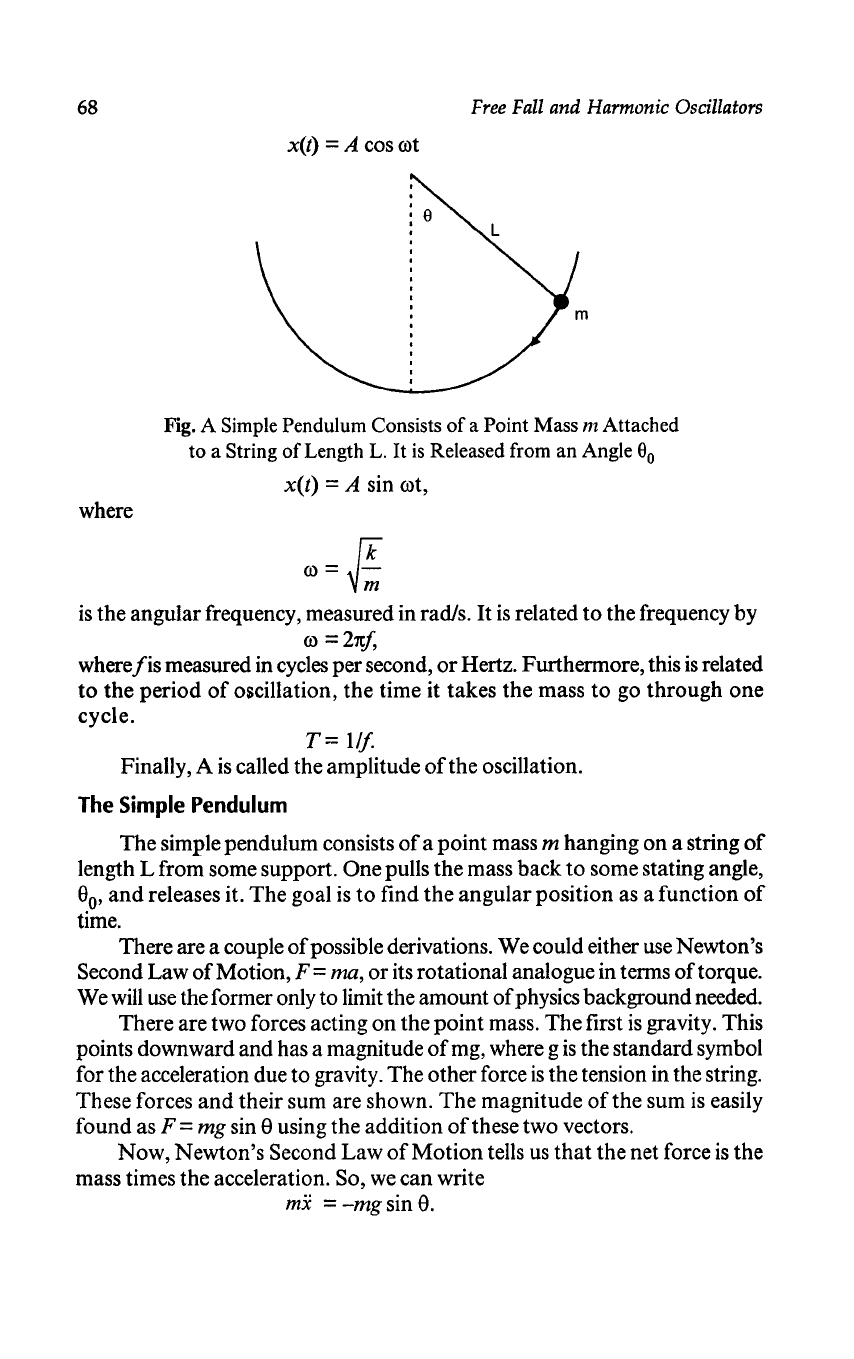
Fig. A Simple Pendulum Consists
of
a Point Mass m Attached
to a
String
of
Length L.
It
is
Released from
an
Angle 9
0
x(t)
= A sin
cot,
co=~
is
the angular frequency, measured in rad/s.
It
is
related
to
the frequency by
co
=
2rcf,
where/is
measured in cycles per second,
or
Hertz. Furthermore, this
is
related
to
the
period
of
oscillation,
the
time it takes
the
mass
to
go
through
one
cycle.
T=
Ilf
Finally, A is called the amplitude
ofthe
oscillation.
The
Simple
Pendulum
The
simple pendulum consists
of
a
point
mass m hanging
on
a string
of
length L from some support. One pulls
the
mass back
to
some stating angle,
9
0
,
and
releases it.
The
goal is
to
find
the
angular
position as a function
of
time.
There are a couple
of
possible derivations. We could either use Newton's
Second
Law
of
Motion, F = ma,
or
its rotational analogue in terms
of
torque.
We
will
use the former only
to
limit the amount
of
physics background needed.
There are two forces acting
on
the
point
mass.
The
first
is
gravity. This
points downward and has a magnitude
of
mg, where g
is
the standard symbol
for the acceleration due to gravity. The other force
is
the tension in the string.
These forces
and
their sum are shown.
The
magnitude
of
the
sum
is
easily
found as
F = mg sin S using the addition
of
these two vectors.
Now,
Newton's Second
Law
of
Motion
tells us
that
the net force
is
the
mass times the acceleration. So,
we
can write
mx
=
-mg
sin
S.

Free
Fall
and
Harmonic
Oscillators
9
mgl,;n
8
T
mg
Fig. There are two Forces Acting
on
the Mass, the Weight mg
and
the Tension
T.
The
net Force
is
found
to
be F =
mg
sin e
69
Next, we need
to
relate x
and
a.
x
is
the
distance traveled, which
is
the
length
of
the arc traced
out
by
our
point mass.
The
arclength
is
related
to
the
angle,
provided
the
angle is measure in radians. Namely, x
=,a
for,
=
L.
Thus, we
can
write
mLa
=
-mg
sin
a.
Cancelling the masses, this then
gives
us
our
nonlinear pendulum equation
La
+ g sin a =
o.
There are several variations
of
equation which will
be
used in this text.
The first
one
is
the linear pendulum. This
is
obtained
by
making a small angle
approximation.
For
small angles we
know
that
sin a 7t
a.
Under
this
approximation becomes
La
+g9
=0.
We note that
th~
equation
is
of
the same form as the mass-spring system.
We define
ro
= g / L
and
obtain
the
equation
for
simple
harmonic
motion,
a +
ro
2
a =
o.
SECOND
ORDER
LINEAR
DIFFERENTIAL
EQUATIONS
In
the last section
we
saw how second order differential equations naturally
appear
in
the
derivations
for
simple oscillating systems.
In
this section we
will look
at
more
general second order linear differential equations.
Second order differential equations are typically harder
than
first order.
In
most cases students are only exposed
to
second
order
linear differential
equations. A general form
is
given by
a(x)y"(x)
+ b(x)y'(x) + c(x)y(x) =
f(x).
One
can
rewrite this equation using
operator
terminology. Namely,
we
first define the differential operator L = a(x)D2 + b(x)D + c(x), whereD = !.

70
Then equation becomes
Ly=f
Free
Fall
and
Harmonic
Oscillators
The solutions
of
linear differential equations are found by making use
of
the I inearity
of
L. An operator L
is
said to be linear
if
it satisfies two properties.
1.
L(YI +
Y2)
= L(YI) + L(Y2)'
2.
L(ay) = aL(v) for a a constant.
One typically solves by finding the general solution
ofthe
homogeneous
problem,
Ly" =
0,
and a particular solution
of
the nonhomogeneous problem,
Lyp = f Then the general solution
is
simply given as y = y + h + yp' This
is
found to be true using the linearity
of
L. Namely,
Ly =
L(Yh
+ yp) =
LYh
+
Lyp
=
0+
f=
f
There are methods for finding a particular solution, yp(x),
of
the equation.
These range from pure guessing to either using the Method
of
Undetermined Coefficients or the Method
of
Variation
of
Parameters.
Detennining solutions to the homogeneous problem
is
not laways so easy.·
However, others have studied a variety
of
second order linear equations and
have saved us the trouble in the case
of
differential equations that keep
reappearing in applications. Again, linearity is useful.
If
YI
and
Y2
are solutions
of
the homogeneous equation, then the linear
combination
clYI + c
2
Y2
is also a solution
of
the homogeneous equation.
In
fact,
if
y I and
Y2
are linearly independent, namely,
c
i
YI
+ c
2
Y2
= °
<=>
c
i
= c
2
= 0,
then clYI + c
2
Y2
is the general solution
of
the homogeneous problem.
Linear independence is established
if
the Wronskian
of
the solutions in
not zero.
W (YI'
Y2)
= YI(x)y'2(x) - y'l (x)Y2(x)
;;f:.
0.
CONSTANT
COEFFICIENT
EQUATIONS
The
simplest
and
most
taught
equations
are
those
with
constant
coefficients. The general form for a homogeneous constant coefficient second
order linear differential equation is given as
ay" (x) + by' (x) + cy(x) =
0.
Solutions are obtained by making a guess
of
y(x) = e
rx
and determining
what possible values
of
r will yield a solution. Inserting this guess into leads
to the characteristic equation
ar2
+ br + C =
0.
The roots
of
this equation lead to three types
of
solution depending upon
the nature
of
the roots.
1.
Real, distinct roots r
I
,
r
2
.
In this case the solutions corresponding
to each root are linearly independent. Therefore, the general solution
is simply
y(x) = cIerl
x
+ c
2
e
r
2
x
.

Free
Fall
and
Harmonic
Oscillators
71
2.
Real, equal
roots
rl
=
r2
= r =
-~.
In
this
case
the
solutions
corresponding to each root are
lin~~rly
dependent. To find a second
linearly independent solution, one uses what is called the Method
of
Reduction
of
Order. This gives the second solution as xe
rx
. Therefore,
the general solution is found as
3. Complex conjugate roots
In
this case the solutions corresponding to
each root are linearly independent. Making use
of
Euler's identity,
e
i9
= cos (e) + i since), these complex exponentials can be rewritten
in
terms
of
trigonometric functions. Namely, one has that e
UX
cos(~x)
and
eUX
sin(~x)
are two linearly independent solutions. Therefore the
general solution becomes
y(x) =
eU'(c
l
cos(~x)
+ c
2
sin(~x)).
The solution
of
constant coefficient equations now follows easily. One
solves the characteristic equation and then determines which case applies. Then
one simply writes down the general solution. We will demonstrate this with a
couple
of
examples.
In
the last section
of
this chapter we review the class
of
equations called the Cauchy-Euler equations. These equations occur often and
follow a similar procedure.
Example:
y"
-
y'
- 6y = 0
yeO)
= 2, y'(O) =
O.
The characteristic equation for this problem
is
1.2
-- r - 6 =
O.
The roots
of
this equation are found as r =
-2,
3. Therefore, the general solution can be
quickly written down.
y(x) = c
l
e-
2x
+ c
2
e
3x
.
Note
that
there are
two
arbitrary constants in
the
general solution.
Therefore, one needs two pieces
of
information to find a particular solution.
Of
course, we have them with the information from the initial conditions. One
needs
y'(x) =
-2c
l
e-
2x
+
3c
2
e
3x
in order to attempt to satisfy the initial conditions. Evaluating y and
y'
at x =
o yields
2
= c
l
+ c
2
0=
-2c
l
+
3c
2
These two equations
in
two unknowns can readily be solved to give c
l
=
6/5 and c
2
= 4/5. Therefore, the solution
of
the initial value problem
is
y(x) =
6 4
"5
e-
2x
+
"5
e
3x
. You should verify that this is indeed a solution.
Example:
y"
+ 6y' + 9y =
O.
In this example
we
have r2 + 6r + 9 =
O.
There
is
only
one
root,
r =
-3.
Again, the
~olution
is found as y(x) = (c
l
+ c
2
x)e--
3x
.
Example:
y"
+ 4y =
O.
