Braibant S., Giacomelli G., Spurio M. Particles and Fundamental Interactions: An Introduction to Particle Physics
Подождите немного. Документ загружается.

26 2 Particle Interactions with Matter and Detectors
γ
γ
γγ
γ
γ
γ
γ
γγ
γ
e
+
e
+
e
+
e
+
e
+
e
+
e
+
e
+
e
+
e
–
e
–
e
–
e
–
e
–
e
–
e
–
e
–
e
–
e
–
e
+
Particle mean
energy
Medium
depth
E
0
E
0
/2
E
0
/4
E
0
/8
E
0
/16
R
2R
3R
4R
5R
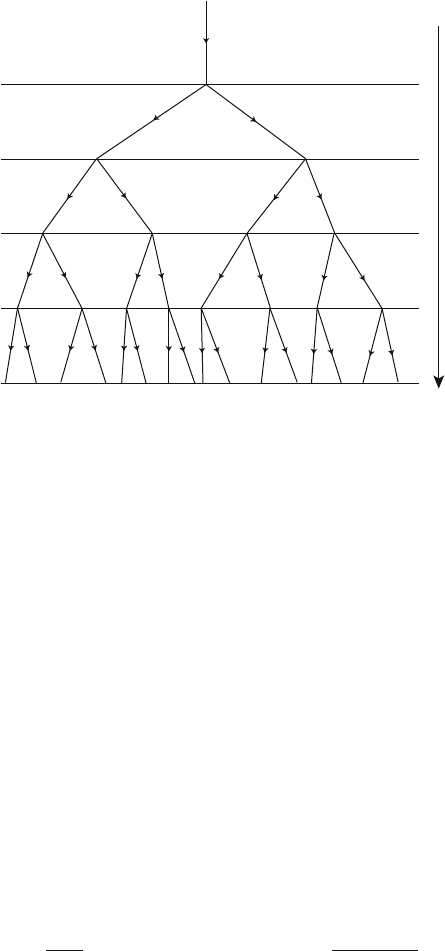
Fig. 2.8 Simplified scheme of an electromagnetic cascade development initiated from a
electrons and positrons go below the critical energy, that is, when they lose energy
only through ionization and excitation.
The cascade development is a statistical process. It can be visualized in a simple
way with the following intuitive method. The primary photon of energy E
0
converts
into an e
C
e
pair after a radiation length L
rad
and the electron or positron mean
energy is E
0
=2 (see Fig. 2.8). In the next radiation length, the electron and positron
both emit one bremsstrahlung photon having approximately half the energy of the
charged particle that emitted it. At this point, after two L
rad
, one has two photons
and an e
C
e
pair. In the following radiation length (at three L
rad
), each of the two
photons is converted into an e
C
e
pair, while the previous e
C
e
pair has radiated
two photons. Now, the number of particles is therefore 8 D 2
3
,ofwhichsixare
e
C
;e
and two are s; the mean energy of any of these particles is E
0
=8. Continuing
with the cascade, after t radiation lengths, the number of ; e
;e
C
particles is
N ' 2
t
, each of them with a mean energy E
N
' E
0
=2
t
. The same result would
be obtained for a cascade initiated from an e
instead of a . Note that we have
measured the medium thickness in radiation lengths, t D x=L
rad
.
One can now ask what is the maximum penetration of the cascade. Measuring
the energy in unit of critical energy, E=E
c
, one has
E
t
max
'
E
0
2
t
max
D E
c
; from which: t
max
'
ln.E
0
=E
c
/
ln 2
: (2.25)
For energies lower than E
c
, the dominant mechanism of energy loss of the electrons
is not bremsstrahlung, but the “continuous” processes of excitation-ionization which
2.4 Electromagnetic Showers 27
0.000
0.025
0.050
0.075
0.100
0.125
0
20
40
60
80
100
(1/E
0
) dE/dt
t = depth in radiation lengths
Number crossing plane
30 GeV electron
incident on iron
Energy
Photons
×1/6.8
Electrons
0 5 10 15 20
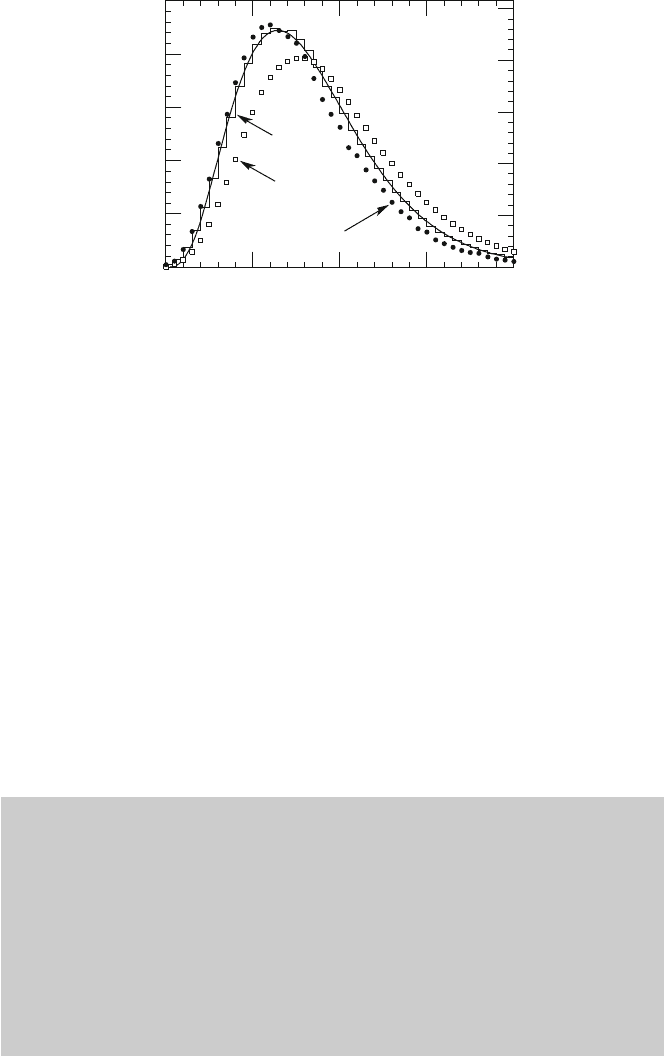
Fig. 2.9 Simulation of an electromagnetic cascade started by a 30 GeV incident electron on iron.
The left scale shows the percentage of the deposited energy per radiation length. The black dots
are the total number of electrons with energy greater than 1.5 MeV; the squares are the number of
photons with E
>1:5MeV (right scale)[P08]
do not allow the multiplication of the number of particles. The maximum number
of particles present in the cascade at a given instant is therefore
N
max
' E
0
=E
c
: (2.26)
This simple model only gives a qualitative idea: the number of particles in a cascade
increases exponentially up to the maximum. When the maximum number has been
reached, the number of particles subsequently decreases gradually. The detailed
analysis of the form of an electromagnetic cascade requires the use of Monte
Carlo methods. Figure 2.9 shows the result of the simulation of an electromagnetic
cascade. Note that the electromagnetic cascade is fully contained in about 20–25
radiation lengths.
Monte Carlo Simulations. Monte Carlo techniques are methods of statistical
simulations based on the use of sequences of pseudo-casual numbers for
the resolution of problems, in particular for estimating the parameters of an
unknown distribution. Monte Carlo methods are particularly useful when the
complexity of the problem makes it impossible or very difficult to reach an
analytical solution or a solution with traditional numerical methods. In the
case of particle detector simulations, sequences of pseudo-casual numbers
are used to produce and follow the parameters of some particles, statistically
varying event by event (the vertex from which a particle is originated, the

28 2 Particle Interactions with Matter and Detectors
energy/momentum of the particle, the point of impact in the detector, the
statistical fluctuations in the energy loss, etc.,) in order to reproduce a
situation as close as possible to reality.
2.5 Neutron Interactions
The neutron, as the photon, has no electric charge; but it has a magnetic dipole
moment, through which it can interact electromagnetically. Neutron interaction with
matter is dominated by the strong interaction; in practice, the cross-section varies
with the energy (speed) of the neutrons. Different energy regions may be considered:
High energy neutrons for kinetic energies T
n
> 100 MeV. In this region, neutrons
behave as protons, with a total cross-section between 40 and 60 mb (see Sect. 7.3).
The study of the neutrons in this energy range is part of the typical studies of particle
physics. The study at smaller energies is included in the nuclear physics field and
may have important technical and engineering implications.
Fast neutrons with 200 keV <T
n
< 40 MeV.
Epithermal neutrons with 0.1 keV <T
n
< 100 keV.
Thermal or slow neutrons when they have kinetic energies comparable to the
energies typical of the thermal motion in materials, that is, T
n
KT .1=40/ eV.
Cold and ultra-cold neutrons for kinetic energies of the order of milli-eV (meV)
and micro-eV (eV).
For energies smaller than 100 MeV, the neutrons are subject to different pro-
cesses:
1. Elastic collision with a nucleus, n C A ! n C A. The elastic scattering is the
most important process for MeV energies.
2. Inelastic collision with a nucleus excitation, nA ! nA
, etc. The excited nucleus
de-excites with the emission of rays. Also, these processes are important for
MeV energies.
3. Radiative neutron capture from a nucleus: n C .Z; A/ ! C .Z; A C 1/;the
cross-section for this process is inversely proportional to the speed and therefore
becomes large at low energies.
4. Nuclear capture reactions of the type .n; p/; .n; d /; .n; ˛/, etc. This cross-
section has a dependence of the type 1=v and therefore the processes become
important for thermal neutrons.
5. Nuclear fission, that is, the neutron capture with breakup of the nucleus (heavy)
in two fragments and emission of a few neutrons (thermal and fast). Fission is
more probable for slow neutrons.
2.6 Qualitative Meaning of a Total Cross-Section Measurement 29
In nuclear physics and for engineering purposes, it is usually necessary to
slow down the fast neutrons. The most important process for obtaining neutron
deceleration is through elastic collisions with nuclei with which other processes
do not occur. The material which is widely used is
12
C for which about 110 elastic
collisions are needed to actually slow down a neutron of 1 MeV to thermal energies
of (1/40)eV. In hydrogen, about 17 collisions are needed.
2.6 Qualitative Meaning of a Total Cross-Section Measurement
If the collisions between two particles were analogous to those between two billiard
balls, elastic collisions and the interaction probability would not depend on the
speed of the incident particle, but would remain constant for any speed. However, if
the balls collide at very high speeds, the billiard balls can break, particularly in the
case of central collisions. Collisions in which the billiard balls break are referred
to as inelastic collisions. With an increase in speed, the rate of inelastic collisions
increases, while the elastic collision rate decreases, though the total probability of a
collision remains the same.
The situation at the level of atomic and nuclear collisions and of particles is more
complicated and less intuitive. Indeed, we have elastic collisions, inelastic collisions
in which the complex system breaks, inelastic processes in which the internal system
is modified, as, for example, in the case of an atom in which an electron is brought
in a more external orbit, and finally, high energy collisions in which the energy is
transformed into mass and new particles are created. The probability of every type
of collision can be measured through a quantity called the cross-section, which is of
enormous importance in high energy physics. The total cross-section is the sum of
elastic and inelastic cross-sections, which may also have different contributions. In
conclusion, several reasons exist as to why one expects that the total cross-section
may strongly vary with the energy of the incident particles.
If the projectiles would have dimensions much smaller than those of the targets, if
there were no wave-like effects and if the forces would have a short range of action,
the cross-section would represent the transverse area (section) of every target. If the
projectiles have comparable dimensions to those of the targets, then one would
measure a quantity that depends on the dimensions of both the projectile and the
target. This is the case of the greatest part of the collisions between particles.
The fact that particles are also waves implies that at the edges of every object
involved in the collision, an elastic diffractive effect occurs. Moreover, in the
submicroscopic world, it is not always possible to clearly separate the effects due
to the type of interaction from those due to the effective dimensions of the objects.
The cross-sections of neutrinos, photons and mesons on protons are very different
because the interactions are respectively caused by the weak, electromagnetic and
strong interaction and because the mesons are, in reality, complex objects. One
may hope to gather the essence of the interactions eliminating spurious effects,
considering in detail the total cross-sections in the limit of the highest energies (that
30 2 Particle Interactions with Matter and Detectors
is, where the associated wavelength is very small) and in the case of the collisions
between the “most elementary” constituents that we presently know. The unit used
to measure the cross-section is the cm
2
; in practice, the atomic cross-sections are
measured in barn (b), 1 barn D 10
24
cm
2
. The cross-section of collisions between
hadrons are of the order of a few tens of mb, 1 mb D 10
3
b D 10
27
cm
2
.Weshall
return to the meaning of the cross-section later.
2.7 Techniques of Particle Detection
The subatomic particles are too small to be observed through optical methods,
though they can be indirectly “observed” through the mechanisms of transfer of
energy in matter. In the previous paragraphs, we have seen that the fast charged
particles ionize and excite, along their trajectories, the atoms of the traversed
medium. This is the principle of operation of all types of detectors. The information
is transformed into electric signals that are then analyzed with electronic methods.
The ionization detectors directly exploit the produced ionization, gathering the
ionization electrons and positive ions (usually in a gas), and transforming them into
electronic signals. In the first half of the 1900s, the ionization chamber, proportional
counter and the Geiger-M¨uller counter were developed. These detectors have
undergone only a few changes and are still used in the laboratory. The multiwire
proportional chamber (MWPC), the drift chamber,thetime projection chamber
(TPC) and others were invented at the beginning of the 1960s. These detectors are
all based on the principle of the proportional counter, though they are larger and
more sophisticated.
In the scintillation counters, the light emitted in the de-excitationof the atoms and
in the molecules excited by the passage of a fast charged particle is used. Various
types of scintillation counters are used in experiments.
In some types of detectors, for example, bubble chambers, along the path of the
particle, the ionization induces a variation of state of the medium; in others, as in
nuclear emulsions, the ionization of the medium activates a chemical process which
is then completed with film development.
In order to be detected, the neutral particles, as the photon, must interact and pro-
duce charged particles (these last particles are those that are actually “observed”).
In the following, the simpler types of detectors are schematically described,
distinguishing the electronic detectors from other types. Detailed descriptions and
information can be found in specialized sites and books [2w2].
2.7.1 General Characteristics
A detector sensitive to all types of radiation for all energies does not exist. Every
detector is sensitive to some type of radiation in a given energetic range. A detector

2.7 Techniques of Particle Detection 31
Table 2.2 Typical values of some of the parameters of particle detectors
Time Dead Spatial Typical
resolution time resolution volume
Detector (s) (s) (cm) (cm
3
)
Ionization chamber, prop. counter. 10
9
10
8
a
1–10
5
Limited streamer tubes 10
8
–10
7
10
4
/m
c
110
2
–10
6
Multiwire proportional chamber 10
9
10
8
10
1
10
3
–10
6
Drift chamber 10
9
–10
8
10
7
–10
5
10
2
10
4
–10
6
Time projection chamber 10
9
–10
8
10
5
–10
4
10
2
10
6
–10
7
Geiger-M¨uller tube 10
6
10
3
a
1–10
4
Semiconductor counter
b
10
8
10
6
10
3
–10 10
Spark chamber 0:2 10
9
10
8
a
1–10
5
Cherenkov counter 10
9
10
8
a
1–10
5
Nuclear emulsion 5 10
5
10–10
4
Nuclear track detector 3 10
4
10–10
6
Cloud chamber 10
2
100 0.05 10
5
Bubble chamber 10
3
1 10
3
–0.1 10
4
–10
7
Scintillation chamber 10
7
10
3
0.05 10
4
–10
6
Streamer chamber 10
8
10
3
0.1 10
6
TRD 10
8
10
6
1 10
5
–10
6
Cryogenic counter 10
5
10
4
10 10
2
a
Depends on apparatus dimensions and segmentation
b
The spatial resolution is given for a single counter for a microstrip detector
c
Dead time per meter of a single crossed streamer tube
possesses specific operational characteristics which are illustrated in the following
in a schematic way (see Table 2.2).
Detector efficiency : Detector efficiency is the probability that a detector
records a radiation that traverses it; it is given by the ratio between the number of
recorded events N
rec
and the N particles that traverse the detector, D N
rec
=N
(with 0 1). It is usually studied through simulation methods (Monte
Carlo methods) based on the knowledge of the detecting process of the geometry
and the mass of the detector of the intrinsic background, for example. It can be
experimentally measured using a beam of known particles.
Response time of the detector: It is connected to the intrinsic time that the detector
takes to form an electronic signal after the arrival of the radiation (excluding the
delays introduced, for example, by the cables). To obtain a good response, the pulse
rise time should be as brief as possible. The response time is usually a Gaussian
distribution for which the half-width at half-maximum can be considered as the
time resolution
t
. Time resolutions are below 1 ns for scintillation counters and
Cherenkov detectors, 1 ms for bubble chambers; for some detectors, a response time
is not defined (nuclear emulsions and nuclear track detectors) (see Table 2.2). The
duration of the signal is important because during this time, a second event may
not be recorded. The dead time is the time between the passage of a particle and

32 2 Particle Interactions with Matter and Detectors
the moment at which the detector is ready to record the passage of the next particle
(during the dead time, the apparatus is not sensitive). The length of the signal, the
electronics used, and the recovery time of the detector (see the example of the Geiger
counter) influence the dead time. They vary from 10
8
to 100s.
Spatial resolution: It is the precision with which the passage of a charged particle
is located in space. It varies from about 1 m for nuclear emulsions to 5–10 mfor
silicon microstrip detectors, to many centimeters for a Cherenkov counter.
Energy resolution: Energy resolution is connected to the possibility of a detector
to distinguish two close energies. If the signals are separate in time, the energy
resolution is the half-width of the energy distribution, measured, for example, in
a “test beam” with particles of known energy. If the two signals are too close in
time, a more refined analysis must be performed. Besides, for scintillators, it is
necessary to take into account the asymmetrical distribution with a long tail towards
high energies, the so-called Landau tail.
2.8 Ionization Detectors
We shall not describe all of the ionizing particle detectors based on the discharges
in gas in detail. One should remember that to create a electron-positive ion pair in
a gaseous medium, it is necessary to provide a mean energy of about 30 eV, a value
which depends on the gas and not on the properties of the ionizing particles. One
should also remember that the ionization potential varies from about 10 eV in the
more complex molecules to about 24eV in the noble gases (see Table 2.3).
One simpler detector that uses a gas is the ionization chamber (or counter)
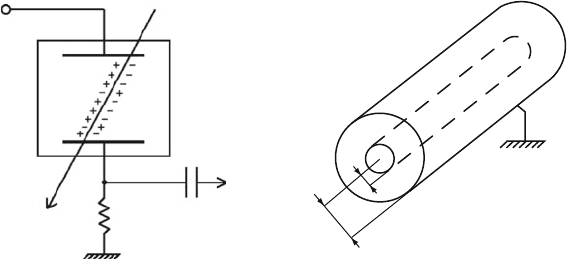
(see Fig. 2.10a); it is usually made of a vessel within which two electrode plates
are inserted, filled with a noble gas at a pressure close to the atmospheric one.
In absence of ionizing particles that cross the chamber, there is no appreciable
continuous electric current. If a fast charged particle crosses the chamber, the ions
produced by the particle passage are all collected in two electrodes; this process
creates a small electric current (current pulse) for a short time of the order of a
Table 2.3 Excitation
potential, ionization potential
and mean energy to produce
an electron-ion pair in various
gases. In general, the lower
the values, the more sensitive
the detector
Excitation Ionization Mean energy
potential (eV) potential (eV) (eV)
H
2
10.8 15.4 37
He 19.8 24.6 41
N
2
8.1 15.5 35
Ne 16.6 21.6 36
Ar 11.6 15.8 26
Xe 8.4 12.1 22
CO
2
10.0 13.7 33
C
4
H
10
10.8 23
2.8 Ionization Detectors 33
Ionizating particle
H
A.T.
ab
C
R
r
1
r
2
+
Fig. 2.10 (a) Ionization chamber: geometry and operating scheme. The path of a fast charged
particle producing positive ions and electrons is indicated. The voltage drop in point H passes
through the capacitor C and proceeds towards the counting electronic system. (b) Coaxial
cylindrical electrodes. The electric field has a dependence of the type E.r/ D V=Œr ln.r
2
=r
1
/
1=r . The ion multiplication happens in the region of the intense electric field close to the internal
conductor
few nanoseconds. In the scheme of Fig. 2.10a, the electric current pulse becomes a
voltage pulse in point H because the electric current decreases the voltage in H. This
pulse can pass through the capacitor C andissenttoanamplifierandtoacounting
electronic system.
For the proportional counters (Fig.2.10b), the operating voltage is such that the
electrons, produced by the ionization of the gas at the passage of the particle, are
multiplied in a proportional way to the initial ionization, resulting in a measurable
avalanche of electric charges.
From a historical point of view, the Geiger counter is the most famous gas
detector. The Geiger counter has a cylindrical electrode structure with a wire
anode and the cathode that serves as an external wall. The generator’s resistance
R is usually quite large (10–100 M˝). The Geiger counter works in the quasi-
independent Townsend discharge regime. The discharge regards the whole tube
because in the point where the initial ionization avalanche (streamer) happens, many
photons are emitted. These photons ionize the molecules of the particular gas used
and the ionized zone propagates along the whole wire up to involving the whole
counter. The operating voltages are about 1–3kV.
With a Geiger counter, high current pulses for a duration of a few microseconds
are obtained. After a discharge, it takes quite some time before the tube is ready to
detect the next particle: the dead time is of the order of milliseconds.
The electronic detectors presented (and others not mentioned here, e.g., the
limited streamer tubes and the resistive plate chambers) cannot provide precise
information on the trajectory of a particle unless a thin segmentation is realized.
Until 1968, precise information on the particle trajectory was obtained using
photographic methods applied to bubble chambers, spark chambers, etc. In 1968,

34 2 Particle Interactions with Matter and Detectors
ab
Incident particle
Sensitive anode wires
Cathode
plates
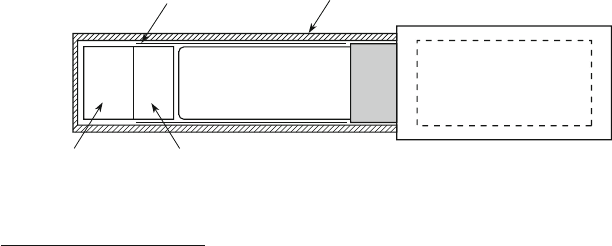
Fig. 2.11 (a) Configuration of a multiwire proportional chamber. Every wire performs as a single
proportional counter. The signals from the wires closest to the trajectory are positive while those
from more distant wires are negative. (b) Configuration of the electric field lines for a MWPC
[2C70]
Charpack (Nobel laureate in 1992) invented the multiwire proportional chamber.
This was the beginning of a technology which allowed electronic detectors to
measure positions with resolutions of 50–100 m, energy loss dE=dx sampled
many times along the trajectory, elevated time resolutions, etc., thus leading the
way to many new applications.
The basic configuration of a multiwire proportional chamber (MWPC) is shown
in Fig. 2.11a: it consists of a plane of parallel wires (anodes) typically with 2 mm
spacing placed in the medium between two cathode plates typically 1.5 cm apart.
The electric field lines are illustrated in Fig. 2.11b. The passage of an ionizing
particle produces positive ions and electrons in the gas inside the chamber. These
last ones drift toward the nearest wire: part of the path happens where the electric
field is constant: they have a motion with a constant speed because of the energy loss
in the collisions (viscous motion). In the zone close to the wire, where the potential
has a 1=r dependence, a multiplication of electrons occurs. In the closest wire (or in
the closest wires in the case of particles with nonperpendicular incidence in the
chamber), a (proportional) positive pulse is produced. However, in the adjacent
wires, negativepulses are generated. Every wire corresponds to a single proportional
counter with separate acquisition electronics (an amplifier, an ADC converter, a
logic signal for TDC, etc.).
This chamber can provide a spatial coordinate, for example, x, with a precision of
a fraction of the wire spacing d, typically
x
' d=
p
12 ' 1 mm. One can operate
a second chamber with wires rotated by 90
ı
in order to obtain information on the y
coordinate with the same precision. A third chamber with wires at 45
ı
is sometimes
used to eliminate any ambiguity in the reconstruction. The response time is only a
few ns, while the chamber efficiency is of the order of 98–99%.
Instead of using a second chamber for the y coordinate, the method of the charge
division can be used: the collected charge at the extremity of a resistive wire (e.g., at
2.9 Scintillation Counters 35
the left side) is proportional to the distance between the point in which the particle
is passed and the extremity of the wire. If Q
L
;Q
R
are the electric charges collected
respectively at the left and right parts of the wire, one has y D `Q
L
=.Q
L
C Q
R
/,
where ` is the wire length. The precisions are not so good (up to 1%ofthewire
length) and it is difficult to maintain a good stability in time. An evolution of the
multiwire proportional chamber is the drift chamber: the information on the y
coordinate is obtained through the measurement of the secondary electron drift time,
t D d=v with respect to a “trigger” signal.
2.9 Scintillation Counters
Figure 2.12 shows the scheme of a scintillation counter consisting of: (1) a
scintillator material whose atoms and molecules are excited by the passage of a
charged particle; in the de-excitation, a certain quantity of light is emitted, i.e., a
certain number of photons. The scintillator is coupled (2) directly or through a light
guide to (3) a photomultiplier; a photomultiplier is made of a photocathode where
the light photons are converted into electrons through the photoelectric effect, and
by a multiplier of electrons (constituted by a series of dy nod es) which produces
an electric signal which may then be amplified by an electronic amplifier.The
photomultiplier is connected to (4) a base containing an adequate circuit providing
the appropriate voltages for the photomultiplier dynodes. (5) A cage made of
ferromagnetic material (mu-metal, an alloy of 80% nickel and 20% iron) minimizes
the effects of the magnetic fields, including the Earth one.
A scintillator (see Table 2.4) must have a good efficiency to convert the deposited
energy in light. Besides, the light must be emitted quickly.
2
Finally, the scintillator
must be transparent to the radiations that it emits and the light must be emitted in a
spectral band compatible with the photomultiplier response.
Steel
screen
Mu metal
screen
Photomultiplier
Light guideScintillator
Base
Fig. 2.12 Scheme of a scintillation counter
2
In this case, one speaks about fluorescence; when the light is emitted in a relatively long
time, one speaks about phosphorescence;thetermluminescence includes both fluorescence and
phosphorescence.
