Braibant S., Giacomelli G., Spurio M. Particles and Fundamental Interactions: An Introduction to Particle Physics

Подождите немного. Документ загружается.

4.1 The Interaction Between Electric Charges 77

γγ

ab c

de f

–

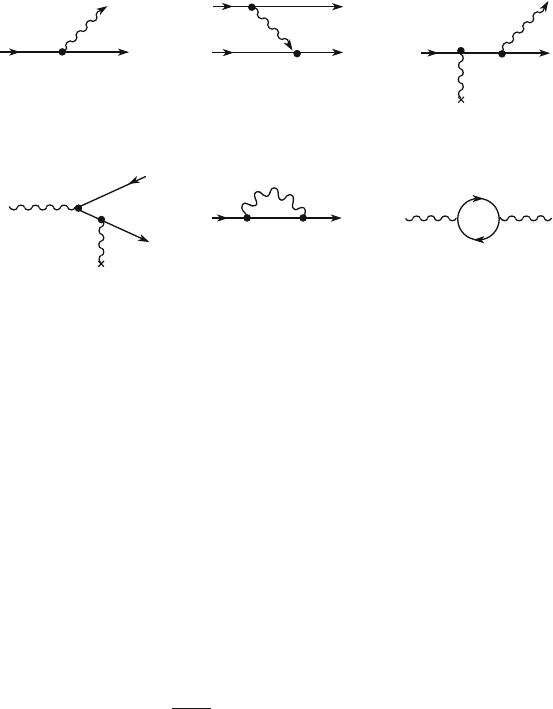
Fig. 4.2 Feynman diagrams of some of the most important processes due to the EM interaction.

(a) emission of a photon by an electron (this fundamental vertex cannot occur if the electron

is isolated; the corresponding contribution to the amplitude squared is proportional to ˛

);

(b) elastic e



collision; (c) bremsstrahlung; (d) pair creation; (e) emission and absorption of

a virtual photon by an electron; (f) creation of a virtual e



pair and subsequent annihilation

(these last two diagrams give rise to closed lines, with virtual particles not directly observed, see

text)

process takes place (experimentally measured through the process cross-section)

is described by the process matrix element. This quantity remains the same if an

incident electron with momentum p is replaced by an outgoing antielectron with

momentum p; there is clearly a relation between the cross-sections of different

processes.

Figure 4.2 shows the Feynman diagrams for the most important processes due

to the electromagnetic interaction. Figure 4.2a represents the emission of a photon

by an electron (see also Problem 4.2) The photon couples to the electron with an

amplitude proportional to

. It is a ﬁrst order process since only one vertex

is present. The elastic collision between two electrons, shown in Fig. 4.2b, is a

second order process with two vertices. The virtual photon contributes with a term

1=q

, called propagator,whereq is the momentum transferred from an electron

to another. Figure 4.2cshowsabremsstrahlung process, i.e., the emission of a

photon by an incident electron accelerated in the Coulomb ﬁeld of a nucleus,

indicated as x. Because three vertices are present (including the nucleus x), it

corresponds to a third order process and the cross-section is proportional to Z

Figure 4.2dshowsthee



pair creation by a photon interacting with the Coulomb

ﬁeld of a nucleus. This is also a third order process. The diagrams (c) and (d)

are similar: (d) can be deduced from (c) by replacing the incoming electron line

with that of the outgoing positron and by changing the photon  from outgoing to

incoming.

78 4 The Paradigm of Interactions: The Electromagnetic Case

4.1.2 The Quantum Theory of Electromagnetism

The experiments carried out at the beginning of the 1900s on the emission of, for

example, blackbody radiation, photoelectric effect, Compton effect, showed that the

electromagnetic ﬁeld is quantized; the quantum of the EM ﬁeld is the photon, with

energy E D h,momentump D h=c,spins D 1„. Because it always moves at

the light speed c, the photon only has two polarization states (e.g., right-handed or

left-hand circular polarization). Classically, this is equivalent to the fact that only

transverse EM waves propagate in vacuum. A virtual photon (by deﬁnition, the

relation E

D p

C m

does not hold for a virtual particle with a rest mass m)

can have a mass different from zero and a third polarization state, the longitudinal

one. Classically, this occurs with electromagnetic waves moving in a waveguide.

The theory of quantum electrodynamics (QED) developed in the 1940s and

1950s by Feynman, Schwinger, Tomonaga (Nobel laureate in 1965) and others,

describes the interaction of charged Dirac ﬁelds (D charged fermions) with the

quantized electromagnetic ﬁeld (second quantization). The theory predicts, in a

large energy range and with high precision, many phenomena, such as, the cross-

sections and transition probabilities.

One of the most important QED properties is its renormalizability. This means

that terms producing inﬁnite quantities (e.g., the so-called “self-energy” terms due

to the diagrams shown in Fig. 4.2e,f) may be all included in the electron mass m

and

electric charge e

. Mass and charge can then be overridden by the experimentally

measured values.

A second important property of QED is the gauge invariance. To intuitively

understand the meaning, remember that in electrostatic, the interaction energy

(experimentally measurable) depends on the electrostatic potential difference, and

not on its absolute value. The interaction energy is therefore invariant under any

change of scale (or “gauge”) of the potential (the potential is known but an

additive constant, the “global gauge”). We shall discuss in Sect. 6.9 the local gauge

invariance, which leads to the electric charge conservation. It is believed that the

theories of fundamental interactions should be renormalizable local gauge theories.

The “constant” ˛

is known with high accuracy from experiments at low

energy. We shall see in Chap. 11 that ˛

is not really constant, but increases

logarithmically with the c.m. energy; for example, it is equal to 1/137 at “zero”

energy and to 1/128 at E

s D 91:2 GeV.

4.2 Some Quantum Mechanics Concepts

In this section, we shall recall some quantum mechanics concepts. A few sim-

ple rules were established to go from classical equations to quantum equations

through the substitution of energy E and momentum p with the corresponding

operators:

4.2 Some Quantum Mechanics Concepts 79

E ! i„

; p !i „r

(4.7)

where r is the gradient operator. The operators @=@t and r are applied on wave

functions. In the covariant form, one has p



!Ci„@=@x



DCi„@



,where

the coordinates of the space-time four-vector x



are indicated as x

D ct; x

x; x

Dy; x

Dz.

4.2.1 The Schr

odinger Equation

Classically, a free particle kinetic energy is E D p

=2m. Applying the substitution

(4.7), one obtains the nonrelativistic Schr

odinger equation:

i„

„

D 0: (4.8a)

The Schr

odinger equation describes the time evolution of the wave function ;it

can also be written as

i„

D H (4.8b)

where H is the system Hamiltonian. For steady states (i.e., not time dependent), the

equation used to derive the energy eigenvalues E is

H D E : (4.8c)

The probability density ﬂux is deﬁned as

j D

i„



r  r



/; (4.9)

satisfying the continuity equation

@

C rj D 0 ) @



D 0 (4.10)

where  Dj j

is the probability density to ﬁnd the particle in a unit volume

(j j

d v is the probability to ﬁnd the particle in a volume dv).

The solution of the Schr

odinger equation:

D Ne

.ipriEt/=„

(4.11)

describes a free particle with energy E and momentum p, with  DjN j

and

j D pjN j

=m.

80 4 The Paradigm of Interactions: The Electromagnetic Case

4.2.2 Klein–Gordon Equation

Let us consider the relativistic relation between energy and momentum, E

C m

. Using the substitution (4.7), one obtains the Klein–Gordon equation:



r



 C

„

 D 0 (4.12)

which describes the propagation of a relativistic free particle of mass m.Incovariant

notation and using the deﬁnition  D @



D @



, one has

 C

„

 D 0;





„



 D 0: (4.13)

If m D 0, it corresponds to the equation describing the propagation of an electro-

magnetic wave;  is now interpreted as the potential U in the coordinate space, or

as the wave amplitude of associated photons which propagate the interaction.

For a static potential in (4.12), the time dependence disappears and by replacing

.r;t/ ! U.r/, one obtains

U D

„

U: (4.14)

For a spherically symmetric potential generated by a point source, U D U.r/ D

U.r/, one has r

U.r/ D

/; r is the distance from the point source placed

in the origin of the system reference frame. It can be veriﬁed that the solution is of

the type

U.r/ D

4r

r=R

(4.15)

where R D„=mc is a quantity with the dimensions of a length, g is an

integration constant and is interpreted as the “intensity strength” of the point source.

The potential (4.15) was initially considered as the “strong” interaction potential

between nucleons in the Yukawa model (see Sect. 7.1.1). The mediators of a Yukawa

interaction have a mass m. The EM case instead corresponds to m D 0,sothat

U.r/ D 0 with a solution U D Q=r,whereQ is the electric charge at

the origin. By analogy, the constant g=4 in the case of the “strong” potential

(4.15) can be considered as the “charge” of the particle that generates the strong

ﬁeld. The factor R in (4.15), related to the mass m of the interaction mediator

boson, is interpreted as the interaction range. According to the original Yukawa

model and since R ' 1:2 fm for a static interaction between two hadrons, one

has mc

D„c=R ' 100 MeV (remember „c = 197.327MeVfm). The predicted

boson was identiﬁed as the  meson. The fact that hadrons are composite objects

complicates this simple interpretation.

4.2 Some Quantum Mechanics Concepts 81

4.2.3 Dirac Equation

The next conceptual step occurred in 1928 when Dirac introduced the quantum

equation which describes the relativistic behavior of point-like particles (as the

electron) with semi-integer spin s D

„:

.i„



 mc/ D 0: (4.16)

Dirac formulated Eq. 4.16 assuming that it was the quantum analogous of the

relation E D˙

C m

.Inthisway,(4.16) contains, contrary to the Klein–

Gordon equation, only ﬁrst derivatives with respect to space and time. The 



matrices are deﬁned in Appendix A.4 where the derivation of the Dirac equation, its

general properties and solutions are also presented in details. The Dirac equation

solutions are four component wave functions called spinors. They can be

considered as the “Dirac ﬁeld,” in analogy with the “electromagnetic ﬁeld” (the

photon) for the Maxwell equations. The Dirac theory explains all the electron

properties known from atomic physics, in particular, the value of the gyromagnetic

ratio between the spin s and the electron magnetic moment 

D g

s, in units of

the Bohr magneton 

The “Dirac sea.” The Dirac equation admits solutions with negative energy.

The states with positive energy and those with negative energy are symmetric

with respect to zero. The existence of negative energy states would destabilize

matter. The hydrogen atom would lose its electron, which would immediately

fall in a negative energy state by emitting a photon of positive energy, in this

way respecting the energy balance. Dirac solved the problem by assuming

that all the negative energy states were ﬁlled, forming the so-called Dirac

sea. The Pauli exclusion principle ensures the stability of the hydrogen atom:

the electron cannot move into a negative energy state because all levels are

already occupied.

In the Standard Model (Chap. 11), the wave functions of all “elementary” spin

s D 1=2 particles obey Eq. 4.16. Here, “elementary” means a particle without a

known internal structure. For example, the proton is not an elementary particle, and

the correct value of its magnetic dipole moment is not directly obtained from the

Dirac equation (see Sect. 7.14.4).

The application of the Dirac theory to the neutrino is currently not veriﬁed by any

experimental evidence. In 1937, E. Majorana obtained a relativistic wave equation

for neutral particles different from that of Dirac. This suggests that neutrinos could

either be Majorana or Dirac particles. The determination of the neutral particle

nature (Dirac or Majorana particle) [Be08] is nowadays one of the most interesting

open experimental questions [4A08].

82 4 The Paradigm of Interactions: The Electromagnetic Case

4.3 Transition Probabilities in Perturbation Theory

In this section, we shall use a perturbation theory in nonrelativistic quantum

mechanics

[P87] to calculate the transition probability (denoted W ) from an initial

to a ﬁnal state. As discussed in the next chapters, this quantity is used to compare

theoretical predictions with experimental data not only for the electromagnetic case,

but also for the weak and strong interactions.

Let us consider the transition probability in a decay process of a particle, or

an interaction (collision) between particles. In the latter case, the cross-section is

the key experimental parameter used to describe the process. The system initial

state is assumed to be well deﬁned: it is, for instance, described by a stationary

wave function , with deﬁned energy E

. This wave function (valid for t<0)is

one of the possible eigenstates of the free Hamiltonian system H

. Factorizing the

wave function (4.11) in a time-dependent term and a time-independent part 

, one

can write

.t < 0/ D 

iE

t=„

: (4.17)

The set of 

functions represents a complete set of eigenfunctions (orthonormal to

each other) of the H

Hamiltonian system, i.e.,



D E



: (4.18)

The transition to one of the allowed possible ﬁnal states (some can be excluded by

the conservation law) is caused by the action of an energy potential term V ,which

starts to be effective for t  0. V is linked to a potential U by a constant: V D g

U .

In the electromagnetic case, g

is the electric charge of the particle described by

the wave function . When the potential V “turns on,” the particle has a ﬁnite

probability to move to a different allowed quantum state 

. At the time t  0,the

total wave function can be expressed as a superposition of possible states, that is,

.t/ D

nD0

.t/

iE

t=„

: (4.19)

The square of the coefﬁcient c

.t/ represents the probability amplitude of ﬁnding

the system in the 

state. At t =0,c

.0/ D 1andc

.0/ D 0 for n ¤ m.At  0,

the wave function (4.19) must satisfy the nonrelativistic Schr

odinger equation for

the Hamiltonian H D H

C V :

H D .H

C V/ D i„@ =@t: (4.20)

The student unfamiliar with the formalism can consider only the calculation ﬁnal results, Eqs. 4.28

and 4.29.

4.3 Transition Probabilities in Perturbation Theory 83

Placing Eqs. 4.19 and 4.18 in Eq. 4.20, one obtains

i„

nD0







iE

t=„

nD0

.t/

iE

t=„

(4.21)

(the term coming from the time partial derivative of the function is canceled

since H



iE

). Multiplying Eq. 4.21 by the complex conjugate

function



(where k is a generic eigenstate of H

) and using the normalization

property 





D 0 for n ¤ k, one gets

i„





nD0

.t/M

i.E

E

/t=„

; (4.22)

where the quantity M

is the matrix element for the transition probability from the

state n into the state k, induced by the potential V ,thatis,





V

d: (4.23)

Note that M

has the dimension of an energy since V (as H ) is an operator with

the dimension of an energy, d D d

x D dv is the volume element, and the wave

functions  have the dimension of a volume

1=2

In perturbation theory, the potential U is assumed to be so weak and the transition

probabilities so small that during the considered time t, the coefﬁcients are such

that c

.t/ ' 1 and c

.t/ ' 0 for n ¤ m. In other words, this means that the

possibility of two or more transitions is extremely unlikely and therefore negligible.

This condition is expressed as

' 0 for n ¤ m: (4.24)

Integrating (4.22) over time, assuming a time-independent potential V ,

.t/ D

i„

i.E

E

/t=„

dt D M

1  e

i.E

E

/t=„

 E

D 2iM

i.E

E

/t=„



sinŒ.E

 E

/t=2„

 E



: (4.25)

The square of the coefﬁcient c

.t/ represents the probability amplitude of ﬁnding

the system in the state 

. In general, the experimentally observed ﬁnal state is a

superposition of different ﬁnal states. This is the case, for example, of a particle

decaying in three particles (three-body decay e.g., the neutron, Chap.8). The total

energy E

available in the ﬁnal state is a constant, but the three particles can share

84 4 The Paradigm of Interactions: The Electromagnetic Case

the total energy in an extremely large number of ways, provided that the sum is E

The total transition probability per time unit towards one of the possible allowed

states is the sum of all the probability amplitudes (4.25), that is,

W D

.t/j

!

1

.t/j



 dE: (4.26)

The sum over discrete states is replaced by an integral on continuous energy states,

as indeed we shall do when examining the above mentioned three-body decay.

This is justiﬁed by the fact that the ﬁnal state system can be represented by the

superposition of a large number of quantized states with a tiny energy difference

between contiguous levels allowed by energy conservation. The quantity dN=dE is

the density of states per energy interval unit.

Equation 4.26 can be integrated with some approximations; by deﬁning the

quantity x D .E

 E

/t=2„, and placing Eq. 4.25 in (4.26), one obtains

W D

„

1





sin

dx: (4.27)

The function sin

x=x

is already known from basic physics studies (diffraction

of electromagnetic waves). This is not surprising since we are also analyzing a

superposition of waves. The function has a main maximum at x D 0 and vanishes at

the ﬁrst minimum at x D˙. The contribution to the integral for jxj >is small

(of the order of 10%). Practically, Eq. 4.27 can be further simpliﬁed by assuming

that dN=dE does not vary dramatically in the range ŒI (where sin

x=x

not negligible) and extracts this term from the integral. Using the fact that

1

sin

dx D ;

the transition probability W becomes

W D

2

„

jM

(4.28)

where the index i represents the initial state and f represents the ﬁnal states. From

the dimensional analysis, W has the dimension of [time]

1

and is measured in (s

1

The Plank’s constant has the dimension of ŒEnergy  Time, M has the dimension

of ŒEnergy and dN=dE has the dimension of ŒEnergy

1

(see Appendix A.2). The

matrix elements for the transition between the states i and f is given by





V

d (4.29)

4.4 The Bosonic Propagator 85

where 

and 

are respectively the spatial part (time-independent) of the initial

and ﬁnal wave functions. dN=dE

is the ﬁnal state energy density (in the literature,

it is sometimes referred to as 

Equation 4.28 is also called the second Fermi golden rule. In the next section, we

shall see its application for a particular potential, which is of great importance for

both EM and weak interactions (Chap. 8).

4.4 The Bosonic Propagator

Let us consider a free particle with a deﬁned energy which is scattered by a potential

of the form (4.15). Note that if the parameter g=4 coincides with the electric charge

Ze and if m D 0 (i.e., R D„=mc D1), the potential (4.15) is exactly the

Coulomb potential of a point-like electric charge. The transition occurs between

the deﬁned initial state to a stationary ﬁnal state. The spatial part of the initial free

particle wave function is 

D e

r=„

and 

D e

r=„

for the ﬁnal state. The

matrix element (4.29) becomes





V.r/

d D g

U.r/e

ip

r=„

d D

D g

U.r/e

i.p

p

/r=„

d D g

U.r/e

iqr=„

d (4.30)

where q D p

 p

, V.r/ D g

U.r/,andg

is a constant describing the coupling

of the particle with the potential U.r/ (in the case of the Coulomb potential, it

coincides with the electric charge of the incident particle). For the considered

potential U.r/, the matrix element (4.30) is called the bosonic propagator.The

name sounds strange, but it actually (as we shall see in the following paragraphs

with the Feynman diagrams) simply expresses the idea that the interaction (i.e., a

momentum exchange) takes place through the exchange of a bosonic particle, which

propagates between the two fermions. The probability that this exchange occurs is

described by the bosonic propagator and depends on the transferred momentum.

The boson propagator is indicated as

f.q/ D g

U.r/e

iqr

d: (4.31)

For the central potential (4.15), one has

• U.r/ D U.r/ D

4r

r=R

• q r D qr cos 

To be rigorous, we should also consider the spinor part of the fermion wave functions. As we shall

see later, this changes the ﬁnal result by a multiplicative factor, depending on the fermion spin.

86 4 The Paradigm of Interactions: The Electromagnetic Case

• d D r

d' sin ddr

•



sin e

iqr cos 

d D .2 sin qr/=qr

For a complex number z, one has sin z D .e

 e

iz

/=2i and Eq. 4.31 can be

written as

f.q/ D f.q/ D 4g

U.r/

sin qr

dr D g

r=R

iqr

 e

iqr

2iq

dr:

Using the relation R D„=mc, integrating the above equation and using „Dc D 1,

one obtains

f.q/ D

C m

: (4.32)

This formula describes in the momentum space the same law as that expressed by

the potential (4.15)inthecoordinate space. Equation 4.32 can be interpreted as

the term describing the probability that a boson be exchanged between the two

interacting particles (fermions). The point where the exchanged boson is emitted or

absorbed is called the vertex. The vertices are characterized by the corresponding

vertex factors, i.e., the coupling constants g

;g; the vertex factors weigh the

probability that the boson couples to each fermion. The propagator is the quantity

C m

1

In Chap. 10, we shall extend the validity of (4.32) to the case where both energy

and momentum are transferred; the variable q

will have a slightly different meaning

(it will represent a relativistic invariant).

4.5 Cross-Sections and Lifetime: Theory and Experiment

In this section, we enter into the heart of the problem of how to compare theoretical

predictions with experimental data. Amongst the experimentally accessible quanti-

ties, the particle lifetime and the cross-section are of fundamental importance.

4.5.1 The Cross-Section

Consider a reaction of the type

a C b ! c Cd

with two particles (a; b) in the initial state and two (c; d) in the ﬁnal state. In an

elastic collision, (c;d) are identical to (a; b). If n

is the density (cm

3

)ofincident