Blanchet L., Spallicci A., Whiting B. (Eds.) Mass and Motion in General Relativity
Подождите немного. Документ загружается.

Computational Methods for the Self-Force in Black Hole Spacetimes 331
the singular fields
N
h
S;dir
˛ˇ
are guaranteed to have the form (5) only in the Lorenz
gauge; other gauge choices may “distort” the local isotropy of the singularity (as
demonstrated in Ref. [18] with a few examples).
Yet, it is important to understand the gauge dependence of the SF. A thorough
analysis of this issue was presented in Ref. [18]. It was explained that the very
definition of the gravitational SF through a mapping of the physical trajectory from
a “perturbed” geometry onto a “background” spacetime automatically gives rise to
a gauge ambiguity in the SF. From this recognition there readily follows a gauge
transformation law for the SF: For a given physical metric perturbation h
in a
given gauge, consider an infinitesimal gauge displacement x
˛
! x
˛
˛
./ /,
under which the perturbation transforms according to
h
! h
C
I
C
I
: (6)
The corresponding gravitational SF then transforms as [18]
F
˛
self
! F
˛
self
h
.g
˛
C u
˛
u
/
R
C R
˛
u
u
i
; (7)
where an overdot denotes covariant differentiation with respect to proper time,
R
˛
is the Riemann tensor associated with the background geometry, and the
terms in square brackets are, of course, evaluated at the particle.
Strictly speaking, the notion of a gravitational SF in a non-Lorenz gauge only
makes sense if, for
˛
relating that gauge to the Lorenz gauge, the expression on
the right-hand side of Eq. 7 is well defined. This is not at all an obvious condition;
some simple counterexamples are analyzed in [18]. In gauges where the expres-
sion in square brackets in Eq. 7 does not have a well-defined (finite and direction
independent) particle limit, one might still devise a useful notion of the SF by aver-
aging over angular directions, or by taking a directional limit in a consistent fashion
[18,57].
1.3 Implementation Strategies
One faces several practical challenges in trying to implement the MiSaTaQuWa
formula. Some analytic approximations (see under “quasi-local” and “weak field”
below) can tackle the tail calculation directly, but an accurate treatment of the
strong-field SF must involve a numerical computation, bringing about several tech-
nical difficulties. Foremostly, how does one go about extracting the tail (or R) piece
from the full retarded perturbation in practice? Equations 2 and 3 suggest using the
subtraction
N
h
˛ˇ
N
h
dir
˛ˇ
(or
N
h
˛ˇ
N
h
S
˛ˇ
). This, however, involves the removal of one
divergent quantity from another, which is not easily tractable in actual numerical
calculations.
Several strategies have been proposed for dealing with this “subtraction” prob-
lem, and in the main part of this review we shall describe a few of them in
some detail. Here we proceed with a brief overview of the main implementation
frameworks, including those based on analytic approximations.
332 L. Barack
1.3.1 Quasi-Local Calculations
This approach tackles the calculation of the tail contribution directly, by analyti-
cally evaluating the Hadamard expansion of the Green’s function [2–5,93,94]. Such
calculations capture the “near” part of the tail, which, one might hope, represents
the dominant contribution in problems of interest. Quasi-local calculations could be
supplemented by a numerical computation of the “far” part of the tail, a strategy
referred to as “matched expansions” (not to be confused with “matched asymptotic
expansions”). The applicability of this idea was demonstrated very recently [37]
with a full calculation in Nariai spacetime (a simple toy spacetime featuring many
of the characteristics of Schwarzschild).
1.3.2 Weak-Field Analysis
The tail formula can be evaluated analytically for certain weak-field configurations
[47, 96, 98–100], within a Newtonian or a post-Newtonian (PN) framework. Such
work has provided important insight into the nature and properties of the SF. PN
techniques have also been implemented in combination with the mode-sum method
discussed below [63,64,89–91].
1.3.3 Radiation-Gauge Regularization
This approach, introduced in 2006 [70], proposes a reformulation of the MiSa-
TaQuWa regularization in the radiation gauge, which enables a reconstruction of the
R-part of the metric perturbation from a regularized Newman–Penrose scalar (
0
or
4
). The main advantage of this method is that it reduces the numerical compo-
nent of the calculation to a solution of a single scalar-like (Teukolsky’s) equation.
However, some of the technical complexity is relegated to the metric reconstruction
stage. This technique has been implemented so far only for a particle held static in
Schwarzschild, but more interesting cases are currently being studied [69,101]. See
also the discussion in Section 3.1.
1.3.4 Mode-Sum Method
An approach whereby one evaluates the tail contribution mode by mode in a multi-
pole expansion [7,16,17,19,21,22,45,62–64,75,87]. The subtraction “fulldirect”
(or “fullS-field”) is performed mode by mode, avoiding the need to deal with di-
vergent quantities. The method exploits the separability of the field equations in
Kerr into multipole harmonics. The mode-sum method has provided the framework
for the bulk of work on SF calculations over the last decade. We will discuss it in
detail in Section 2.
Computational Methods for the Self-Force in Black Hole Spacetimes 333
1.3.5 “Puncture” Methods
A set of recently proposed methods custom-built for time-domain numerical im-
plementation in 2 C1 or 3 C1 dimensions [11, 12, 69, 77, 116]. Common to these
methods is the idea to utilize as a variable for the numerical time evolution a “punc-
tured” field, constructed from the full field by removing a suitable singular piece,
given analytically. The piece removed approximates the correct S-field sufficiently
well that the resulting “residual” field is guaranteed to yield the correct MiSa-
TaQuWa SF. In the 2 C1D version of this approach the regularization is done mode
by mode in the azimuthal (m-mode) expansion of the full field. This procedure of-
fers significant simplification; we shall review it in detail in Section 5.
SF Calculations to Date As we have mentioned already, the program to calculate
the SF in black hole orbits has been progressing gradually, through the study of a
set of simplified model problems. Some of the necessary computational techniques
were first tested within the simpler framework of a scalar-field toy model before
being applied to the electromagnetic (EM) and gravitational problems. Authors have
considered special classes of orbits (static, radial, circular) before attempting more
generic cases, and much of the work so far has focused on Schwarzschild orbits.
The state of the art is that there now exist numerical codes for calculating the scalar,
EM, and gravitational SFs for all (bound) geodesics in Schwarzschild spacetime. It
is reasonable to predict that workers in the field will now increasingly be turning
their attention to the Kerr problem.
The information in Tables 1–3 is meant to provide a quick reference to work done
so far. It covers actual evaluations of the local SF that are based on the MiSaTaQuWa
formulation (or the analogous scalar-field and EM formulations of Refs. [102]and
[46,66,103], respectively), either directly or through one of the aforementioned im-
plementation methods. We have included weak-field and PN implementations, but
have not included quasi-local calculations and work based on the radiative Green’s
function approach. The three tables list separately works on the scalar, EM, and
gravitational SFs. In each table, works are listed roughly in chronological order.
Some of the numerical techniques indicated under “calculation method” are dis-
cussedinSections4 and 5 of this review.
The rest of this review is structured as follows. Section 2 is a self-contained intro-
duction to the mode-sum method. The basic idea is presented through an elementary
example, followed by a formulation of the method as applied to generic orbits in
Kerr. In Section 3, we discuss the practicalities of numerical calculations with point-
like sources, and review some of the methods proposed to facilitate such calculations
in both the frequency and time domains. Section 4 focuses on a particular imple-
mentation method, namely the direct time-domain integration of the Lorenz-gauge
metric perturbation equations (in Schwarzschild). This method enabled the recent
milestone calculation of the gravitational SF for eccentric orbits in Schwarzschild,
and we present results from this calculation. In Section 5 we discuss puncture-type
methods, proposed (with the Kerr problem in mind) as alternative to the standard
mode-sum scheme. We focus on one particular variant: the m-mode regularization
method. Section 6 reflects on recent advances and speculates on future directions.

334 L. Barack
Table 1 Calculations of the scalar-field SF within the MiSaTaQuWa framework. In this table, as
well as in Tables 2 and 3, “direct” implies explicit evaluation of the MiSaTaQuWa tail term; “spec-
tral” indicates numerical calculation through frequency-domain analysis; and “evolution” refers to
numerical calculation via integration in the time domain
Case Author(s)
Implementation
strategy
Computation
method
Schwarzschild: Burko [29] Mode sum Analytic
Static particle
a
Newtonian potential: Pfenning and Poisson [96] Direct Analytic
Generic motion
Schwarzschild: Burko [30] Mode sum Spectral
Circular geodesics Detweiler et al. [45, 48]
Schwarzschild: Barack and Burko [8] Mode sum Evolution in 1C1D
Radial geodesics
Spherical mass shell: Burko et al. [33] Mode sum Analytic
Static particle
Schwarzschild: Nakano et al. [90] Post-Newtonian Analytic
Circular geodesics Hikida et al. [64]
Kerr–Newman: Burko and Liu [32] Mode sum Semi-analytic
Static particle
Isotropic cosmology: Burko et al. [34] Direct Analytic
Static particle
Isotropic cosmology: Haas and Poisson [61] Direct Analytic
Slow motion
Schwarzschild: Haas [59] Mode sum Evolution in 1C1D
Eccentric geodesics
Schwarzschild: Vega and Detweiler [116] Puncture Evolution in 1C1D
Circular geodesics
Kerr: circular Barack and Warburton [119] Mode sum Spectral
Equatorial geodesics
a
This case was analyzed independently by Wiseman [120] using a different method.
Table 2 Calculations of the electromagnetic self-force based on MiSaTaQuWa formula
Case Author(s)
Implementation
strategy
Computation
method
Schwarzschild: Burko [29] Mode sum Analytic
Static particle
a
Newtonian potential: Pfenning and Poisson [96] Direct Analytic
Generic motion
Isotropic cosmology: Haas and Poisson [61] Direct Analytic
Slow motion
Schwarzschild: Keidl et al. [70] Radiation gauge Analytic
Static particle Regularization
Schwarzschild: Haas [60] Mode sum Evolution in 1C1D
Eccentric geodesics
a
This calculation recovers results by Will and Smith [105] using a different method.

Computational Methods for the Self-Force in Black Hole Spacetimes 335
Table 3 Calculations of the gravitational self-force based on MiSaTaQuWa formula
Case Author(s)
Implementation
strategy
Computation method
Newtonian potential: Pfenning and Poisson [96] Direct Analytic
Generic motion
Schwarzschild: Barack and Lousto [14] Mode sum 1C1D evolution in
Radial geodesics Regge–Wheeler gauge
Schwarzschild: Keidl et al. [70] Radiation gauge Analytic
Static particle Regularization
Schwarzschild: Barack and Sago [24] Mode sum 1C1D evolution in
Circular geodesics Lorenz gauge
Schwarzschild: Detweiler [42] Mode sum Spectral numerics in
Circular geodesics Regge–Wheeler gauge
Schwarzschild: Barack and Sago [25, 26] Mode sum 1C1D evolution in
Eccentric geodesics Lorenz gauge
2 Mode-Sum Method
Let us write the MiSaTaQuWa formula (4) in the more compact form
F
˛
self
.z/ D lim
x!z
F
˛
.x/ F
˛
S
.x/
; (8)
where F
˛
and F
˛
S
are fields ( the “full force” and “S force,”respectively) constructed
through
F
˛
.x/ D k
˛ˇ ı
N
h
ˇIı
;F
˛
S
.x/ D k
˛ˇ ı
N
h
S
ˇIı
: (9)
Recall that k
˛ˇ ı
.x/ are tensor fields defined through a (smooth) extension of
k
˛ˇ ı
0
(given in Eq. 3) off the worldline. The fields F
˛
.x/ and F
˛
S
.x/ may be
defined through any such extension, as long as the same extension is applied in
both. Both F
˛
and F
˛
S
, of course, diverge at the particle, but their difference,
F
˛
R
k
˛ˇ ı
N
h
R
ˇIı
,isasmooth (analytic) function of x even at the particle.
In the mode-sum method we formally decompose the fields F
˛
and F
˛
S
into mul-
tipole (l;m) harmonics in the black hole spacetime. These harmonics are defined in
the Kerr/Schwarzschild background based on the Boyer–Lindquist/Schwarzschild
coordinates .t;r;;'/in the standard way, that is, through a projection onto an or-
thogonal basis of angular functions defined on surfaces of constant t and r.Letus
denote by F
˛l
.x/ and F
˛l
S
.x/ the l-mode contribution to F
˛
and F
˛
S
, respectively
(summed over m). A key observation is that each of these l-mode fields is finite even
at the particle. This suggests a natural regularization procedure, which, essentially,
amounts to performing the subtraction F
˛
F
˛
S
mode by mode. The idea is best
developed through an elementary example, as follows.

336 L. Barack
2.1 An Elementary Example
Consider a pointlike particle of mass at rest in flat space. The location of the
particle is x D x
p
in a given Cartesian system. In this simple static configuration,
the perturbed Einstein equations read
r
2
N
h
tt
D16 ı
3
.x x
p
/ (10)
(with all other components vanishing), where
N
h
˛ˇ
.x/, recall, is the trace-reversed
metric perturbation (Eq. 1), r
2
is the Laplacian operator, and we have represented
the particle with a delta-function distribution. The static perturbation
N
h
˛ˇ
automati-
cally satisfies the Lorenz-gauge condition (1). Of course, in this simple case we can
immediately write down the exact physical solution (it is the Coulomb-like solution
N
h
tt
D 4=jx x
p
j), and we simply have
N
h
S
tt
D
N
h
tt
and F
˛
S
D F
˛
, so that Eq. 8
trivially gives F
˛
self
D 0 as expected. However, for the sake of our discussion, let us
instead proceed (indeed, rather artificially in our case) by considering the multipole
expansion of the perturbation.
To this end, introduce polar coordinates .r;;'/, such that our particle is located
at .r
0
¤ 0;
0
;'
0
/, and expand h
tt
in spherical harmonics on the spheres r Dconst,
in the form
N
h
tt
D
1
X
lD0
N
h
l
tt
.r;;'/; where
N
h
l
tt
.r;;'/D
l
X
mDl
Q
h
lm
tt
.r/ Y
lm
.; '/: (11)
This expansion separates Eq. 10 into radial an angular parts, the former reading (for
each l;m)
Q
h
lm
tt;rr
C
2
r
Q
h
lm
tt;r
l.l C 1/
r
2
Q
h
lm
tt
D
16
r
2
0
Y
lm
.
0
;'
0
/ı.r r
0
/; (12)
where an asterisk denotes complex conjugation. The unique physical l;m-mode so-
lution, continuous everywhere and regular at both r D 0 and r !1, reads
Q
h
lm
tt
.r/ D
16
.2l C 1/r
0
Y
lm
.
0
;'
0
/
.r=r
0
/
l1
;r r
0
;
.r=r
0
/
l
;r r
0
;
(13)
giving
N
h
l
tt
.r; / D
4
r
0
P
l
.cos /
.r=r
0
/
l1
;r r
0
;
.r=r
0
/
l
;r r
0
;
(14)
where P
l
is the Legendre polynomial and is the angle subtended by the two radius
vectors to x and x
p
(see Fig. 2).
Now consider the multipole decomposition of the “full-force” field defined on the
left-hand side of Eq. 9. This decomposition will, of course, depend on how the tensor
k
˛ˇ ı
is extended off the particle. We choose here a simple “fixed k” extension,
defined through k
˛ˇ ı
.x/ k
˛ˇ ı
0
(in our Minkowskian coordinates .t; x/). Then
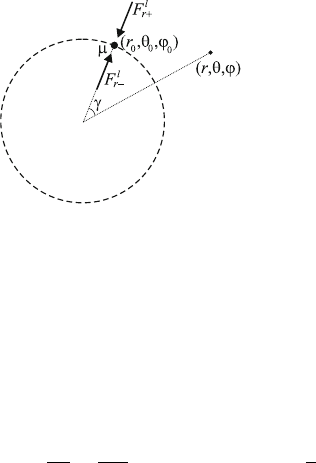
Computational Methods for the Self-Force in Black Hole Spacetimes 337
Fig. 2 An illustration of the simple setup described in the text: A particle of mass in flat space is
at rest at .r
0
;
0
;'
0
/. The gravitational field of the particle is decomposed into spherical harmonics,
each contributing a finite amount F
l
r˙
to the “one-sided” radial full force acting on the particle
F
t
D0 and F
i
Dk
ttj
i
N
h
tt;j
D.=4/
N
h
tt;i
. Focus now on the r component. The l
mode of F
r
is given in terms of the l mode of
N
h
tt;r
simply as F
l
r
D.=4/
N
h
l
tt;r
.
Using Eq. 14 and evaluating F
r
at the particle (taking ! 0 followed by r ! r
˙
0
),
we obtain
F
l
r˙
.x
p
/ DL
2
r
2
0
2
2r
2
0
; where L l C
1
2
: (15)
Here, the subscripts ˙ indicate the two (different) values obtained by taking the
particle limit from “outside” (r ! r
C
) and “inside” (r ! r
).
Let us note the following features manifest in the above simple analysis:
The individual l modes of the metric perturbation,
N
h
l
˛ˇ
, are each continuous at
the particle’s location, although their derivatives are discontinuous there.
The individual l modes of the full force, F
l
˛
,havefinite one-sided values at the
particle.
At large l, each of the one-sided values of the full force at the particle is domi-
nated by a term / l. (The mode sum obviously diverges at the particle, reflecting
the divergence of the full force F
˛
there.)
It turns out (as we shall see later) that all the above features are quite generic,
and they carry over intact to the much more general problem of a particle moving in
Kerr spacetime. Specifically, we find that, at any point along the particle’s trajectory,
the (one-sided values of the) full-force l modes always have the large-l form
F
l
˛˙
D˙LA
˛
C B
˛
C C
˛
=L C O.L
2
/: (16)
In our elementary problem the power series in 1=L truncates at the L
0
term, but in
general the series can be infinite. The l-independent coefficients A
˛
, B
˛
,andC
˛
,
whose values depend on the geometry as well on the particle’s location and four-
velocity, reflect the asymptotic structure of the particle singularity at large l.These
coefficients, called regularization parameters, play a crucial role in the mode-sum
regularization procedure, as we describe next.

338 L. Barack
2.2 The Mode-Sum Formula
Consider a mass particle moving on a geodesic trajectory in Kerr, and suppose we
are interested in the value of the SF at a point z with Boyer–Lindquist coordinates
(t
0
;r
0
;
0
;'
0
) along the trajectory. Starting with Eq. 8, let us formally expand F
˛
.x/
and F
˛
S
.x/ in spherical harmonics
3
on the surfaces t;r D const. Denoting the corre-
sponding l-mode contributions (summed over m)byF
˛l
.x/ and F
˛l
S
.x/, we write
4
F
˛
self
.z/ D lim
x!z
1
X
lD0
h
F
˛l
.x/ F
˛l
S
.x/
i
: (17)
Since F
˛
.x/F
˛
S
.x/ is a smooth function for all x, the mode sum in Eq. 17 is guar-
anteed to converge exponentially for all x (this is a general mathematical property
of the multipole expansion). In particular, the sum converges uniformly at x D z,
and we are allowed to change the order of limit and summation. We expect, how-
ever, that the particle limit of the individual terms F
˛l
and F
˛l
S
is only defined in a
one-sided sense. Hence, we write
F
˛
self
.z/ D
1
X
lD0
h
F
˛l
˙
.z/ F
˛l
S˙
.z/
i
; (18)
where ˙ indicates the values obtained by first taking the limits t ! t
0
, !
0
and
' ! '
0
, and then taking r ! r
˙
0
. Of course, the difference F
˛l
˙
.z/ F
˛l
S;˙
.z/ does
not depend on the direction from which the radial limit is taken: As the difference
F
˛l
.x/ F
˛l
S
.x/ is the l mode of a smooth function, it is itself smooth for all x.
Furthermore, since the mode sum in Eq. 18 converges exponentially, we expect
F
˛l
and F
˛l
S
to share the same large-l power expansion (16), with the same expan-
sion coefficients. This motivates us to reexpress Eq. 18 in the form
F
˛
self
.z/ D
1
X
lD0
h
F
˛l
˙
.z/ LA
˛
B
˛
C
˛
=L
i
1
X
lD0
h
F
˛l
S˙
.z/ LA
˛
B
˛
C
˛
=L
i
: (19)
Here, each of the terms in square brackets vanishes at least as 1
2
as l !1,
and so each of the two sums converges at least as 1=l. We hence arrive at the
3
Here we ignore the vectorial nature of F
˛
and F
˛
S
and treat each of their Boyer–Lindquist com-
ponents as a scalar function. We do this for a mere mathematical convenience. See [62] for a more
sophisticated, covariant treatment.
4
Note that the form of F
˛l
and F
˛l
S
will depend on the specific off-worldline extension chosen for
the tensor k. However, this ambiguity disappears upon taking the limit x ! z, and the final SF is
of course insensitive to the choice of extension.
Computational Methods for the Self-Force in Black Hole Spacetimes 339
following mode-sum reformulation of the MiSaTaQuWa equation:
F
˛
self
.z/ D
1
X
lD0
h
F
˛l
˙
.z/ LA
˛
B
˛
C
˛
i
D
˛
(20)
with
D
˛
1
X
lD0
h
F
˛l
S˙
.z/ LA
˛
B
˛
C
˛
i
: (21)
The mode-sum formula (20), first proposed in Refs. [17] (scalar-field case) and
[7] (gravitational case) provides a practical way of calculating the SF, once the
regularization parameters A
˛
, B
˛
, C
˛
,andD
˛
are known. The values of these
parameters are obtained analytically via a local analysis of the singular (or direct)
field near the particle. Early calculations of the regularization parameters [6,7, 17],
which were restricted to specific orbits, were based on a local analysis of the Green’s
function near coincidence (x ! z)atlargel. Later, after the local form of the direct
field had been derived explicitly to sufficient accuracy [87], workers have been able
to derive general expressions for the regularization parameters, valid for generic
(geodesic) orbits – first in Schwarzschild [16, 19, 21], then in Kerr [20, 22]. Later
derivations (in the scalar case) are presented in Ref. [45], where higher-order terms
in the 1=L expansion were derived analytically in order to accelerate the conver-
gence of the mode sum; and in Ref. [62], where the regularization parameters were
redefined as scalar quantities using a covariant projection of the singular field onto
a null tetrad based on the worldline.
In what follows, we sketch the derivation of the parameters (using the method of
Refs. [16, 22]) for generic geodesics in Kerr.
In passing, we remind that the mode-sum formula (in the gravitational case) is
formulated in the Lorenz gauge, just like the MiSaTaQuWa formula on which it
relies. The values of the regularization parameters; the form of the large-l expansion
of the singular force; or even the very definiteness of the SF – none of these is gauge
independent. It has been shown, however, that the regularization parameters remain
invariant under gauge transformations (from the Lorenz gauge) that are sufficiently
regular [18].
2.3 Derivation of the Regularization Parameters
Consider a geodesic worldline in Kerr, and refer back to Fig. 1. Let point z
along have Boyer–Lindquist coordinates .t
0
;r
0
;
0
;'
0
/. The singular force at
x (a point in the immediate vicinity of z)isF
˛
S
.x/ D k
˛ˇ ı
N
h
S
ˇIı
(Eq. 9), where
N
h
S
ˇ
.x/ is given in Eq. 5. We hereafter use the “fixed k” extension, defined through
k
˛ˇ ı
.x/ k
˛ˇ ı
0
.z/ (note this definition is coordinate dependent; here we re-
fer specifically to contravariant Boyer–Lindquist components). We assume x
˛
are
340 L. Barack
smooth coordinates, and denote the coordinate difference between points x and z by
ıx
˛
x
˛
z
˛
.InEq.5 the quantities
2
.x/ and Ou
˛
.x/ are smooth functions of ıx,
and we may expand them in the form
2
D S
0
C S
1
C O.ıx
4
/; Ou
˛
D u
˛
C ıu
˛
C O.ıx
2
/; (22)
where S
0
and S
1
are, respectively, quadratic and cubic in ıx,andıu
˛
is linear in
ıx. Explicitly, these expansion coefficients read
S
0
D .g
˛ˇ
C u
˛
u
ˇ
/ıx
˛
ıx
ˇ
; (23)
S
1
D
u
u
˛ˇ
C g
˛ˇ;
=2
ıx
˛
ıx
ˇ
ıx
; (24)
ıu
˛
D
˛ˇ
u
ıx
ˇ
; (25)
where the metric and its derivatives, as well as the background connections
˛ˇ
,are
all evaluated at z (for a derivation of S
1
, see Appendix A of [19]).
We now substitute the above expansions in Eq. 5 and construct the field F
˛
S
.x/.
We may write down the resulting expression as a sum of terms sorted according to
how they scale with ıx:
F
˛
S
.x/ D
P
˛
.1/
.ıx/
3
0
C
P
˛
.4/
.ıx/
5
0
C
P
˛
.7/
.ıx/
7
0
C O.ıx/: (26)
Here
0
S
1=2
0
,andP
˛
.n/
denotes a certain multilinear function of the coordinate
differences ıx
˛
, of homogeneous order n in ıx. Note that the first term on the right-
hand side scales as ıx
2
, the second as ıx
1
, and the third as ıx
0
.TheO.ıx/
remainder disappears at the limit x ! z and cannot affect the value of the final SF;
it is therefore safe to ignore it. The explicit form of P
˛
.7/
will not be needed in our
analysis. The two other coefficients read
P
˛
.1/
D
1
2
K
˛ı
0
S
0;ı
; (27)
P
˛
.4/
D
1
2
K
˛ı
0
S
0
S
1;ı
C
3
4
K
˛ı
0
S
1
S
0;ı
1
2
K
˛ı
1
S
0
S
0;ı
; (28)
where
K
˛ı
0
D 4k
˛ˇ ı
u
ˇ
u
;K
˛ı
1
D 4k
˛ˇ ı
.ıu
ˇ
u
C u
ˇ
ıu
/: (29)
The l modes of the F
˛
S
.z/ are constructed, by definition, through
F
˛l
S˙
D lim
x!z˙
l
X
mDl
Y
lm
.; '/
Z
d˝
0
Y
lm
.
0
;'
0
/F
˛
S
.r;t;
0
;'
0
Iz/
D lim
ır!0
˙
L
2
Z
d˝
0
P
l
.cos
Q
/F
˛
S
.r; t
0
;
0
;'
0
Iz/; (30)
