Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena

Подождите немного. Документ загружается.

Chapter

Concentration Distributions

in Turbulent

Flow

521.1

Concentration fluctuations and the time-smoothed concentration

521.2

Time-smoothing of the equation of continuity of

521.3

Semi-empirical expressions for the turbulent mass flux

521.4'

Enhancement of mass transfer by a first-order reaction in turbulent

flow

521.5.

Turbulent mixing and turbulent flow with second-order reaction

In preceding chapters we have derived the equations for diffusion

a fluid or solid, and

we have shown how one can obtain expressions for the concentration distribution, pro-

vided no fluid turbulence is involved. Next we turn our attention to mass transport in

turbulent flow.

The discussion here is quite similar to that in Chapter 13, and much of that material

can be taken over by analogy. Specifically, 5513.4, 13.5, and 13.6 can be taken over di-

rectly by replacing heat transfer quantities by mass transfer quantities. In fact, the prob-

lems discussed in those sections have been tested more meaningfully in mass transfer,

since the range of experimentally accessible Schmidt numbers is considerably greater

than that for Prandtl numbers.

We restrict ourselves here to isothermal binary systems, and make the assumption

of constant mass density and diffusivity. Therefore the partial differential equation de-

scribing diffusion

a flowing fluid (Eq. 19.1-16) is of the same form as that for heat con-

duction in a flowing fluid (Eq. 11.2-9), except for the inclusion of the chemical reaction

term in the former.

CONCENTRATION FLUCTUATIONS AND

THE TIME-SMOOTHED CONCENTRATION

The discussion in 513.1 about temperature fluctuations and time-smoothing can be taken

over by analogy for the molar concentration c,. In a turbulent stream, c, will be a rapidly

oscillating function that can be written as the sum of a time-smoothed value

and a tur-

bulent concentration fluctuation c:

which is analogous to Eq. 13.1-1 for the temperature.

virtue of the definition of c; we

see that

However, quantities such as vick, v$;, and

are not zero, because the

local fluctuations in concentration and velocity are not independent of one another.

The time-smoothed concentration profiles

G(x,

are those measured, for exam-

ple, by the withdrawal of samples from the fluid stream at various points and various

658

Chapter 21 Concentration Distributions in Turbulent Flow

times. In tube flow with mass transfer at the wall, one expects that the time-smoothed

concentration

will vary only slightly with position in the turbulent core, where the

transport by turbulent eddies predominates. In the slowly moving region near the bound-

ary surface, on the other hand, the concentration

will be expected to change within a

small distance from its turbulent-core value to the wall value. The steep concentration

gradient is then associated with the slow molecular diffusion process in the viscous sub-

layer in contrast to the rapid eddy transport in the turbulent core.

521.2

TIME-SMOOTHING OF THE EQUATION

OF CONTINUITY OF

We begin with the equation of continuity for species

which we presume is disappearing

by an nth-order chemical reaction.' Equation 19.1-16 then gives, in rectangular coordinates,

Here

is the reaction rate coefficient for the nth-order chemical reaction, and is pre-

sumed to be independent of position. In subsequent equations we shall consider n

and n

2 to emphasize the difference between reactions of first and higher order.

When cA is replaced by

c;, and

ul!, we obtain after time-averaging

Comparison of this equation with Eq. 21.2-1 indicates that the time-smoothed equation

differs in the appearance

of some extra terms, marked here with dashed underlines.

The

terms containing vlc; describe the turbulent mass transport and we designate them by

FA!,

the ith component of the turbulent molar flux vector. We have now met the third of

the turbulent fluxes, and we may summarize their components thus:

turbulent molar flux (vector)

Ti;

ui c; (21.2-3)

turbulent momentum flux (tensor)

.$)

Pv;v;

(21.2-4)

turbulent heat flux (vector)

ct)

-P

v!~t

(21.2-5)

All of these are defined as fluxes with respect to the mass average velocity.

It is interesting to note that there is an essential difference between the behaviors of

chemical reactions of different orders. The first-order reaction has the same form

the

time-smoothed equation as in the original equation. The second-order reaction, on the

other hand, contributes on time-smoothing an extra term

-k;"c,

this being the manifes-

tation of the interaction between the chemical kinetics and the turbulent fluctuations.

We now summarize all three of the time-smoothed equations of change for turbu-

lent flow of an isothermal, binary fluid mixture with constant p,

gA,,

and

continuity

-5)

(21.2-6)

motion

continuity of

Here

J$)

and it is understood that the op'erator

DIE

is to be written with the

time-smoothed velocity

in it.

Corrsin,

Physics

Fluids,

1,4247

(1958).

521.4

Enhancement of Mass Transfer

a First-Order Reaction in Turbulent Flow

659

521.3

SEMI-EMPIRICAL EXPRESSIONS FOR

THE TURBULENT MASS FLUX

In the preceding section we showed that the time-smoothing of the equation of conti-

I I

nuity of A gives rise to a turbulent mass flux, with components

7;;

To solve

mass transport problems in turbulent flow, it may be useful to postulate a relation be-

tween

and the time-smoothed concentration gradient.

number of empirical expres-

sions can be found in the literature, but we present here only the two most popular ones.

Eddy Diffusivity

By analogy with Fick's first law of diffusion, we may write

as the defining equation for the

turbulent diffusivity

@&,

also called the

eddy diffusivity.

is the case with the eddy viscosity and the eddy thermal conductivity, the eddy diffusiv-

ity is not a physical property characteristic of the fluid, but depends on position, direc-

tion, and the nature of the flow field.

The eddy diffusivity

92)B

and the eddy kinematic viscosity

v"'

p't'/p

have the same

dimensions-namely, length squared divided by time. Their ratio

is a dimensionless quantity, known as the

turbulent Schmidt

number.

As is the case with

the turbulent Prandtl number, the turbulent Schmidt number is of the order of unity

(see the discussion in 513.3). Thus the eddy diffusivity may be estimated by replacing it

by the turbulent kinematic viscosity, about which a fair amount is known. This is done

in 921.4, which follows.

The

Mixing-Length Expression of Prandtl

and

Taylor

According to the mixing-length theory of Prandtl, momentum, energy, and mass are all

transported by the same mechanism. Hence by analogy with Eqs. 5.4-4 and 13.3-3 we

may write

where

is the Prandtl mixing length introduced in Chapter

The quantity

121

dE,/dyl

ap-

pearing here corresponds to

9zL

Eq.

21.3-1, and to the expressions for

v'"

and

a'"

im-

plied by Eqs. 5.4-4 and 13.3-3. Thus, the mixing-length theory satisfies the

Reynolds

annIogy

v(f)

9")

AB,

or ~r'~)

SC")

521.4

ENHANCEMENT OF MASS TRANSFER

A FIRST-ORDER

REACTION IN TURBULENT FLOW'

We now examine the effect of the chemical reaction term in the turbulent diffusion equa-

tion. Specifically we study the effect of the reaction on the rate of mass transfer at the

wall for steadily driven turbulent flow in a tube, where the wall (of material A) is slightly

Hanna,

Sandall, and

Wilson,

Ind.

Eng.

Chem. Research,

26,2286-2290 (1987).

analogous problem dealing

with

falling films is given

Sandall,

Hanna, and

Valeri,

Chem. Eng. Communications,

16,135-147 (1982).

660

Chapter 21 Concentration Distributions in Turbulent Flow

soluble in the fluid (a liquid

flowing through the tube. Material

dissolves in liquid

and then disappears by a first-order reaction. We shall be particularly interested in the

behavior with high Schmidt numbers and rapid reaction rates.

For tube flow with axial symmetry and with

independent of time,

Eq.

21.2-8

becomes

Here we have made the customary assumption that the axial transport by both molecu-

lar and turbulent diffusion can be neglected. We want to find the mass transfer rate at

the wall

where

CA~

and are the concentrations of

at the wall and at the tube axis.

pointed out

the preceding section, the turbulent diffusivity is zero at the wall, and

consequently does not appear in Eq. 21.4-2. The quantity

is a

mass transfer

coefficient,

analogous to the heat transfer coefficient

The coefficient

was discussed in Chapter

and mentioned in Chapter

in connection with "Newton's law of cooling." As

first ap-

proximation' we take to be zero, assuming that the reaction is sufficiently rapid

that the diffusing species never reaches the tube axis; then dZA/dr must also be zero at

the tube axis. After analyzing the system under this assumption, we will relax the as-

sumption and give computations for a wider range of reaction rates.

We now define the dimensionless reactant concentration C

ZA/cA0. Then under

the further assumption' that, for large

the concentration will be independent of

Eq. 21.4-1 becomes

This equation may now be multiplied by r and integrated from an arbitrary position to

the tube wall to give

Here the boundary conditions at r

have been used, as well as the definition of the

mass transfer coefficient. Then a second integration from

R gives

Here we have used the boundary conditions C

at r

and C

Next we introduce the variable

r, since the region of interest

right next to

the wall. Then we get

in which C(y) is not the same function of

iJi

as C(7) is of

For large Sc the integrands are

important only in the region where

R, so that

may be safely approximated

by R. Furthermore, we can use the fact that the turbulent diffusivity in the neighborhood

g21.4

Enhancement of Mass Transfer by a First-Order Reaction in Turbulent Flow

661

of the wall is proportional to the third power of the distance from the wall. When the in-

tegrals are rewritten in terms of

y/R, we get the dimensionless equation

This equation contains several dimensionless groupings: the Schmidt number Sc

v/9,,,

a dimensionless reaction-rate parameter

k;"R2/v, and a dimensionless mass transfer

coefficient Sh

k,D/gAB known as the Sherwood number

being the tube diameter).

In the limit that Rx

the solution to Eq. 21.4-3 under the given boundary condi-

tions is

exp(-Shu/2). Substitution of this solution into Eq. 21.4-7 then gives after

straightforward integration

in which

This can

solved' to give Sh as a function of Sc, Rx, and

The foregoing solution of Eq. 21.4-3 is reasonable when Sc,

Rx,

and

are sufficiently

large, and is an improvement over the result given by Vieth, Porter and Sherwo~d.~

However, in the absence of chemical reaction,

Eq.

21.4-3 fails to describe the downstream

increase of

caused by the transfer of species

into the fluid. Thus, the mass-transfer

enhancement by the chemical reaction cannot be assessed realistically from the results of

either Ref.

or Ref. 2.

For a better analysis of the enhancement problem, we use Eq. 21.4-1 to get a more

complete differential equation for

The assumption that C

at r

is then replaced by the zero-flux condition dC/dr

there. We represent

'3:b

in this geometry as

IdEJdrl for fully developed flow, by use of

a position-dependent mixing length

as in Eq. 21.3-3. Introducing dimensionless nota-

tions

EJv,,

zv,/v, r+

rv,/v, and

It'

lv,/v based on the friction velocity

of 95.3, we can then express Eq. 21.4-11 in the dimensionless form

in which a Damkohler number Da

kyv/v$ has been introduced.

An excellent model for the mixing length

is available in Eq. 5.4-7, developed by

Hanna, Sandall, and Mazet3 by

modifying

the model given by van Drie~t.~ This model

Vieth,

Porter, and T.

Sherwood,

Ind.

Eng.

Chem.

Fundam.,

2,l-3 (1963).

Hanna,

Sandall, and

Mazet,

AKhE

Journal,

27,693-697 (1981).

van

Driest,

Aero.

Sci.,

23,1007-1011,1036 (1956).

662

Chapter 21 Concentration Distributions in Turbulent Flow

will give smooth concentration profiles, provided that we use a velocity function with

continuous radial derivative, rather than the piecewise continuous expressions given in

Fig. 5.5-3. Such a function is obtainable by integrating the differential equation

in the dimensionless variables

&/u,

and

yvJv

of Fig. 5.5-3, with the bound-

ary conditions

0 at

0 (the wall) and

du+/dy+

R+

(the centerline).

Equation 21.4-13 is obtained (see Problem 21B.5) by combining the cylindrical-coordinate

versions of Eqs. 5.5-3 and 5.4-4 with the dimensionless form

of the mixing-length model shown in Eq. 5.4-7. Equation 21.4-13 is solvable via the qua-

dratic formula to give

and

is then computable by quadrature using, for example, the subroutines trapzd and

qtrap of Press et aL5 The resulting

function closely resembles the plotted line in Fig.

5.5-3, with small changes near

30 where the plotted line has a slope discontinuity,

and near the centerline where the calculated

function attains a maximum value de-

pendent on the dimensionless wall radius

R+

whereas the line in Fig. 5.5-3 improperly

does not.

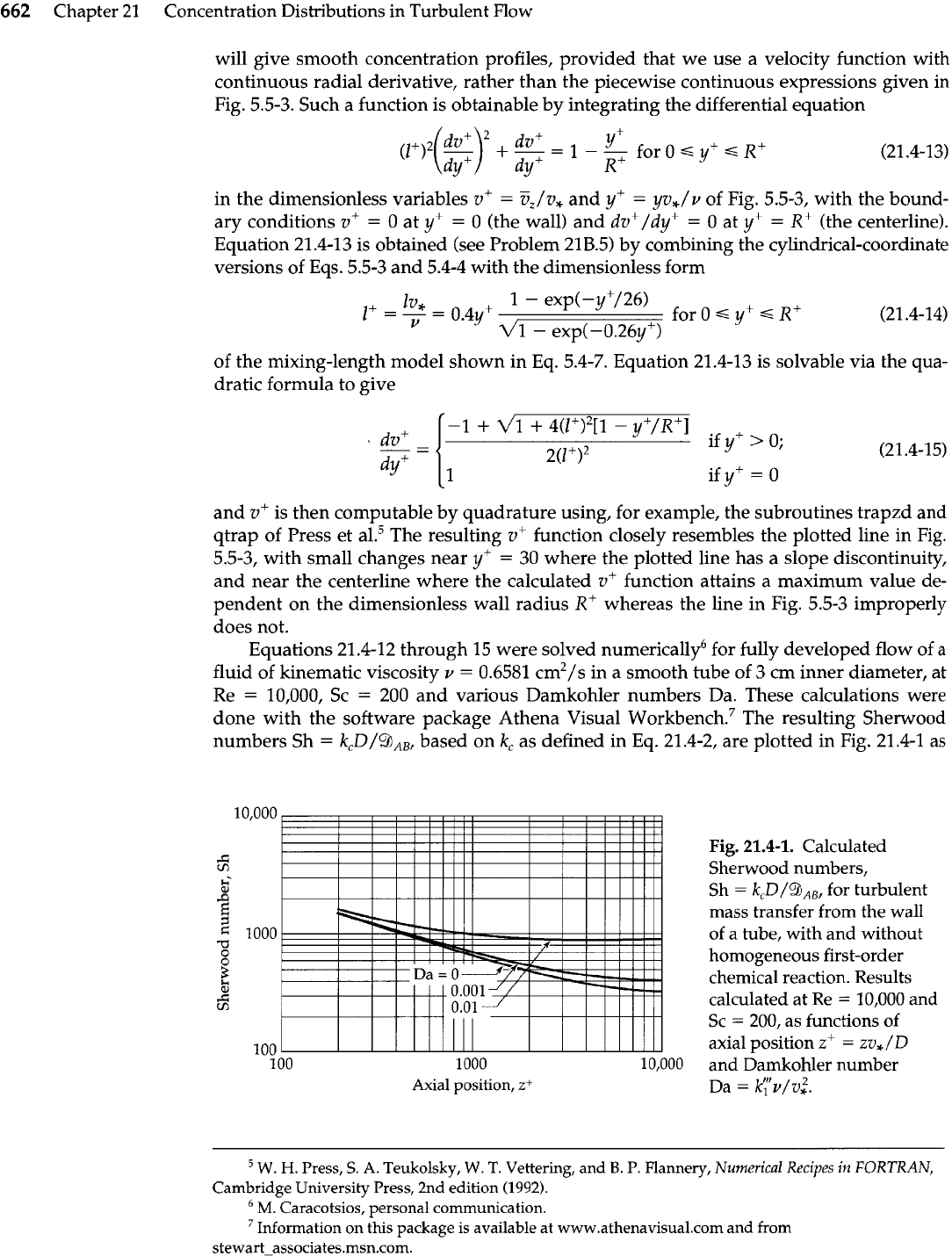
Equations 21.4-12 through 15 were solved numerically6 for fully developed flow of

fluid of kinematic viscosity

0.6581 cm2/s in a smooth tube of 3 cm inner diameter, at

10,000, Sc

200 and various Damkohler numbers

Da.

These calculations were

done with the software package Athena Visual W~rkbench.~ The resulting Sherwood

numbers Sh

kcD/9,,, based on

as defined in Eq. 21.4-2, are plotted in Fig. 21.4-1 as

Axial position,

Fig.

21.4-1.

Calculated

Sherwood numbers,

k,D/QAB,

for turbulent

mass transfer from the wall

of a tube, with and without

homogeneous first-order

chemical reaction. Results

calculated at Re

10,000 and

200, as functions of

axial position

zv,/D

and Damkohler number

kyv/v:.

Press,

Teukolsky,

Vettering, and

P. flannery,

Numerical

Recipes

FORTRAN,

Cambridge University Press, 2nd edition

(1992).

Caracotsios, personal communication.

Information on this package is available at www.athenavisual.com and from

stewart~associates.msn.com.

521.5

Turbulent Mixing and Turbulent Flow with Second-Order Reaction

663

functions of

for various values of the Damkohler number Da. These results lead to the

following conclusions:

In the absence of reaction (that is, when Da

O),

the Sherwood number falls off

rapidly with increasing distance into the mass-transfer region. This behavior is

consistent with the results of Sleicher and

ribu us'

for a corresponding heat trans-

fer problem, and confirms that the convection term of

Eq.

21.4-11 is essential for

this system. This term was neglected in References

and 3 by regarding the con-

centration profiles as "fully developed."

In the presence of a pseudo-first-order homogeneous reaction of the solute (that is,

when Da

O),

the Sherwood number falls off downstream less rapidly, and ulti-

mately attains a constant asymptote that depends on the Damkohler number.

Thus, the enhancement factor, defined as Sh (with reaction)/Sh (without reaction),

can increase considerably with increasing distance into the mass-transfer region.

g21.5

TURBULENT MIXING AND TURBULENT

FLOW WITH SECOND-ORDER REACTION

We now consider processes occurring within turbulent fluid systems, with particular ref-

erence to the two mixers shown in Fig. 12.5-1. In Fig. 12.5-l(a) is shown a steady state sys-

fem,

in which two input streams enter a system of fixed geometry at constant rates, and

in Fig. 12.5-l(b) an unsteady state system, in which two initially stationary, segregated,

miscible fluids are mixed by turning an impeller at a constant angular velocity, starting at

time t

One stream [in (a)] or one initial region [in (b)] contains solute

in solvent

and the other contains solute

in solvent

All solutions are sufficiently dilute that the

solutes do not appreciably affect the viscosity, density, or species diffusivities. Then the

behavior of the solute (A or

in either system [(a) or (b)] is described by the non-time-

smoothed diffusion equations

with suitable initial and boundary conditions.

For these systems, we may write that at

[in (a)] or t

0 [in

(b)]

C,O

and

0 (21.5-3,4)

over the A inlet port [in (a)] or the initial region [in (b)], and

cBO

and

0 (21.5-5,6)

over the

inlet port [in (a)] or the initial region [in

(b)].

In addition, we consider all con-

fining surfaces to be inert and impenetrable.'

No Reaction Occurring

For this situation, the terms

and

are identically zero. We now define a single new

independent variable

Sleicher and

Tribus,

Trans.

ASME,

79,789-797

(1957).

In system

(a),

these boundary conditions are only approximations.

The

indicated values of

and

are regarded as asymptotic values for

664

Chapter

Concentration Distributions

Turbulent Flow

Then both Eqs. 21.5-1 and 2 take the following form over the whole system:

Here the subscript i can represent either solute A or solute

and

for (a) the entering A-rich stream, or

(b)

initially A-rich region (21.5-9)

for (a) the entering B-rich stream, or

(b)

initially B-rich region (21.5-10)

It follows that, for equal diffusivities, the time-smoothed concentration profiles,

T(x,

z, t) are identical for both solutes, where

However, the fluctuating quantities

are also of interest, as they are measures of "un-

mixedness." These can be equal only in a statistical sense. To show this, we subtract

Eq.

21.5-11 from Eq. 21.5-7, and then square the result and time-smooth it to give

Here

d(x,

z, t) is a dimensionless decay function, which decreases toward zero at large

[for the motionless mixer in Fig. 21.5-l(a)I, or at large t [for the mixing tank of Fig. 21.5-

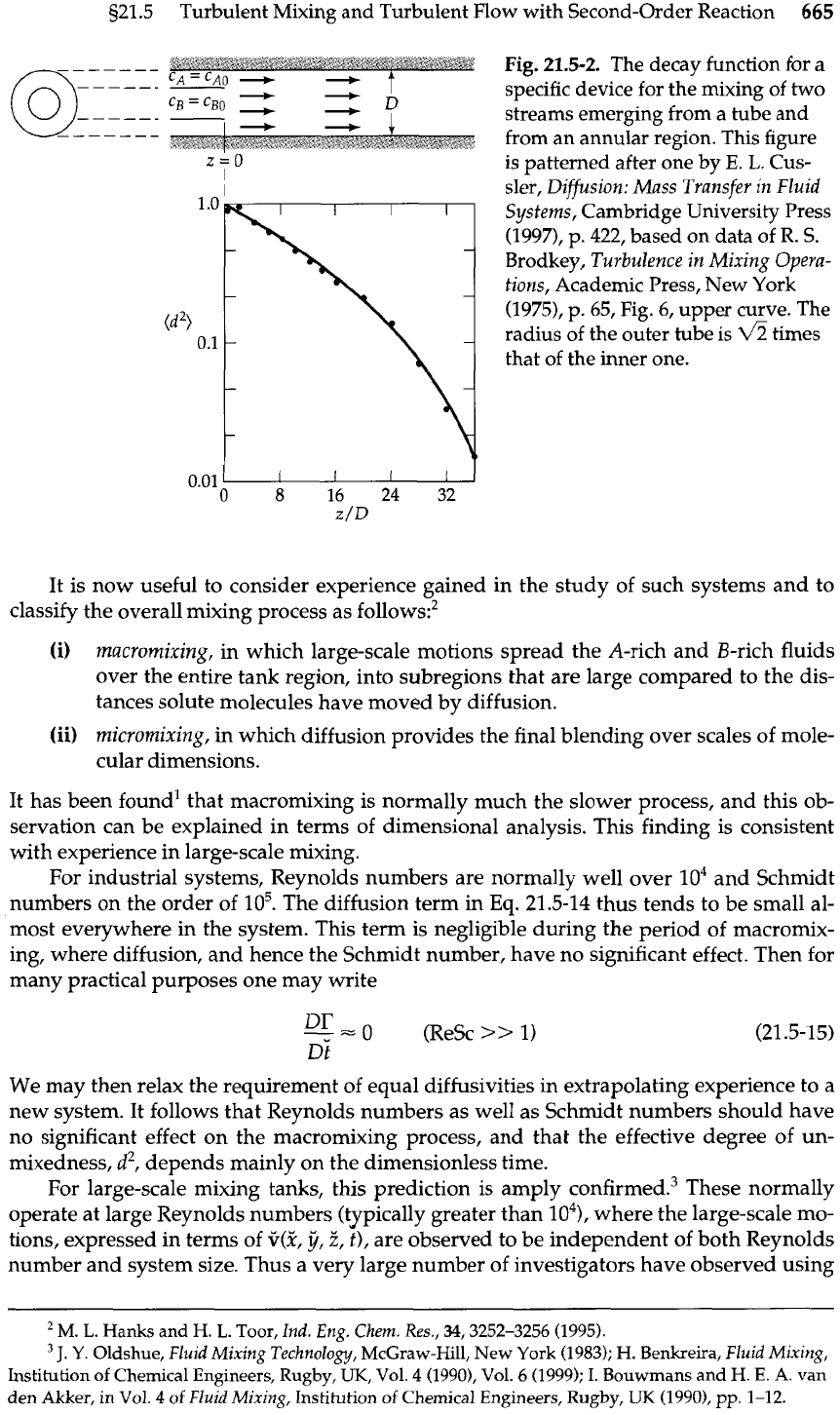
I@)]. Cross-sectional averages of this quantity can be measured, and are shown in Fig.

21.5-2.

It remains to determine the functional dependence of the decay function, and to do

this we introduce the dimensionless variables:

Then

Eq.

21.5-8 becomes

+zr

ReSc

in which Re

I,u,p/p.

In order to be able to draw specific conclusions, we now focus our attention on mix-

ing tanks [see Fig. 21.5(b)], and further assume low-viscosity liquids and low-molecular-

weight solutes. For these systems

is normally chosen to be the diameter of the

impeller, and

to be

I&,

where

is the rate of impeller rotation in revolutions per unit

time.

B+

Fig.

21.5-1.

Two types

mixers:

(a)

a baffled

(a)

mixer with no moving

parts;

(b)

batch mixer

(b)

with a stirrer.

521.5

Turbulent Mixing and Turbulent Flow with Second-Order Reaction

665

Fig.

21.5-2.

The decay function for a

specific device for the mixing of two

streams emerging from a tube and

from an annular region. This figure

is patterned after one by

E. L.

Cus-

sler, Diffusion: Mass Transfer

Fluid

Systems, Cambridge University Press

(1997),

422,

based on data of

Brodkey, Turbulence in Mixing Opera-

tions, Academic Press, New York

(1975),

65,

Fig.

upper curve. The

radius of the outer tube is

times

that of the inner one.

It is now useful to consider experience gained in the study of such systems and to

classify the overall mixing process as

follow^:^

(i)

macromixing, in which large-scale motions spread the A-rich and B-rich fluids

over the entire tank region, into subregions that are large compared to the dis-

tances solute molecules have moved by diffusion.

(ii)

micromixing,

which diffusion provides the final blending over scales of mole-

cular dimensions.

It has been found1 that macromixing is normally much the slower process, and this ob-

servation can be explained in terms of dimensional analysis. This finding is consistent

with experience in large-scale mixing.

For industrial systems, Reynolds numbers are normally well over

lo4

and Schmidt

numbers on the order of lo5. The diffusion term in

Eq.

21.5-14 thus tends to be small al-

most everywhere in the system. This term is negligible during the period of macromix-

ing, where diffusion, and hence the Schmidt number, have no significant effect. Then for

many practical purposes one may write

We may then relax the requirement of equal diffusivities in extrapolating experience to a

new system. It follows that Reynolds numbers as well as Schmidt numbers should have

no significant effect on the macromixing process, and that the effective degree of un-

mixedness, d2, depends mainly on the dimensionless time.

For large-scale mixing tanks, this prediction is amply ~onfirmed.~ These normally

operate at large Reynolds numbers (typically greater than

lo4),

where the large-scale mo-

tions, expressed in terms of

+(?,

jl,

t),

are observed to be independent of both Reynolds

number and system size. Thus a very large number of investigators have observed using

Hanks and

Toor,

Ind.

Eng.

Chem.

Res.,

34,3252-3256 (1995).

Oldshue,

Fluid Mixing

Technology,

McGraw-Hill, New York (1983);

Benkreira,

Fluid Mixing,

Institution of Chemical Engineers,

Rugby,

UK,

Vol.

(1990), Vol. 6 (1999); I. Bouwmans and

van

den Akker, in Vol.

Fluid Mixing,

Institution of Chemical Engineers, Rugby,

(1990),

pp.

1-12.

666

Chapter

Concentration Distributions in Turbulent Flow

many different mixer geometries, that the product of the required mixing time

t,,

and

rotation rate N is a constant independent of mixer size and Reynolds number:

That is, the required mixing time

t,,

corresponds, for a given tank geometry, essen-

tially to the required number of turns of the impeller. This expectation is confirmed by

experience.

This finding is consistent with observations2 that both the dimensionless volume

flow rate through the impeller, Q/ND~, and the tank friction factor, plpIV3D5, are con-

stants, depending only on the tank and impeller geometries (see Problem

6C.3).

Here

is the volumetric flow in the jet produced by the impeller, and

is the power required to

turn it.

Similar remarks usually apply to motionless mixers, where increasing the flow ve-

locities typically has little effect on the degree of mixing. However, approximations like

this must be tested, and such tests should be considered as first steps in an experimental

program. As a practical matter, these approximations are almost always reliable on

scale-up, since Reynolds numbers normally increase with equipment size.

Reaction Occurring

We next consider the effects of a homogeneous, irreversible chemical reaction, and for

simplicity we write this as

products. Again we assume dilute solutions, so that

the heat of reaction and the presence of reaction products have no significant effect.

addition, we assume equal diffusivities for the two solutes.

We next define

Then when we subtract Eq. 21.5-2 from Eq. 21.5-1, we find that the description of

~,e,cti,,

is identical to that for its nonreactive counterpart. Hence

By subtracting from this its time-smoothed counterpart, we find that an equation like

Eq.

21.5-18 must hold for the fluctuations:

(4-4)

=(")

CAO

CBO

reactive nonreactive

The time-smoothed mean square of the quantity on the right side is equal to

d2,

which

measurable as illustrated in Fig. 21.5-2, and therefore we have a way of predicting the

corresponding quantity for reacting systems.

Equation 21.5-19 suggests that the fluctuations in

and

in reactive problems

occur on the same time and distance scales as for nonreactive problems. Note that this is

true for arbitrary geometry, flow conditions, and reaction kinetics. We are now ready to

consider special cases.

We begin with a fast reaction, for which the two solutes cannot coexist, and the rate

of the reaction is controlled by the diffusion of the species toward each other. Then, for

the first (macromixing) stage of the blending process, where diffusion is very slow com-

pared to the larger-scale convective processes, there is no significant reaction. In this,

typically dominant, stage of the blending process

(")

=(")

reactive nonreactive