Bhushan B. Nanotribology and Nanomechanics: An Introduction

Подождите немного. Документ загружается.

1102 B. Bhushan

2.4 times larger than that for the one-level model, respectively, but adhesion energy

decreases rapidly at surfaces with σ greater than 0.05µm; and in every model it ﬁ-

nally decreases to zero at surfaces with σ greater than 10µm. The adhesion energy

for the three-level model with 0.1 k

III

is 2–3 times higher than that for three-level

model.

In order to demonstrate the eﬀect of the hierarchical structure on adhesion en-

hancement, Kim and Bhushan [55] calculated the increases in the adhesion coeﬃ-

cient, the number of contacts, and the adhesion energy of the two-, three- and three-

level (with 0.1 k

III

) models relative to one-level model. These results are shown in

the right side of Fig. 20.15b. It was found for the two- and three-level models, rel-

ative increase of the adhesion coeﬃcient increases slowly with an increase of σnd

has the maximum values of about 70% and 80% at σ1 µm, respectively, and then

decreases for surfaces with σgreater than 3µm. On the whole, at the applied load

of 1.6µN, the eﬀect of the variation of σn the adhesion enhancement for both two-

and three-levelmodels is not so large. However, the relative increase of the adhesion

coeﬃcient for the three-level model with 0.1 k

III

has the maximum value of about

170% at σ1µm, which shows signiﬁcant adhesion enhancement. Due to the relative

increase of adhesion energy, the three-level model with 0.1 k

III

shows signiﬁcant

adhesion enhancement.

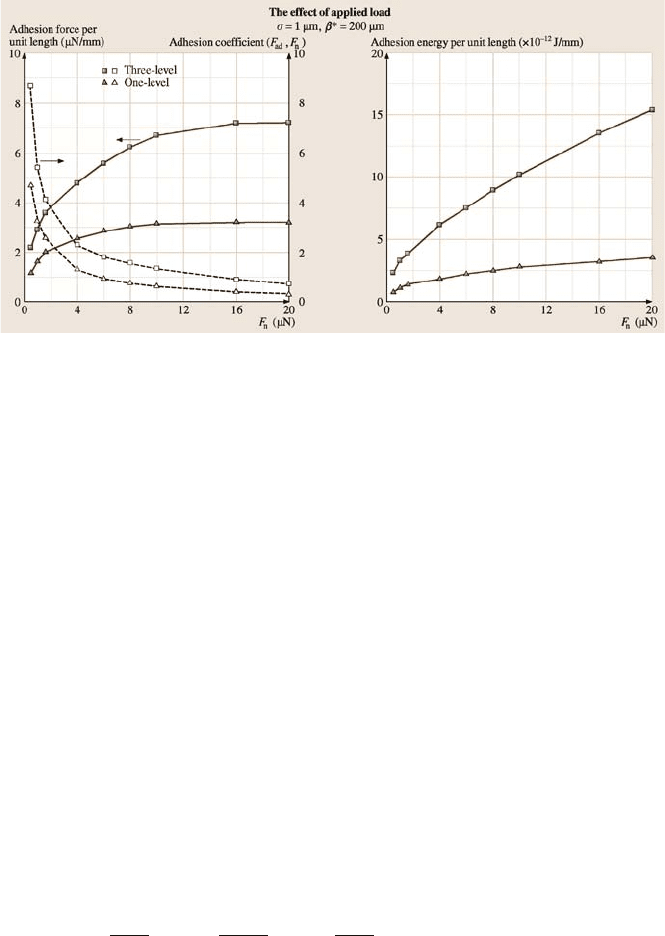
Figure 20.16 shows the variation of adhesion force and adhesion energy as

a function of applied load for both one- and three-level models contacting with sur-

face with σ = 1 µm. It is shown that as the applied load increases, the adhesion force

increases up to a certain applied load and then has a constant value, whereas, adhe-

sion energy continuesto increase with an increase in the applied load. The one-level

model has a maximum value of adhesion force per unit length of about 3µN/mm at

the applied load of 10µN, and the three-level model has a maximum value of about

7 µN/mm at the applied load of 16 µN. However, the adhesion coeﬃcient continues

to decrease at higher applied loads because adhesion force is constant even if the

applied load increases.

The simulation results for the three-level model, which is close to gecko se-

tae, presented in Fig. 20.15 show that roughness reduces the adhesion force. At the

surface with σ greater than 10µm, adhesion force by gecko weight cannot support

itself. However, in practice, a gecko can cling or crawl on the surface of ceiling

with higher roughness. Kim and Bhushan [55] did not consider the eﬀect of lamel-

lae in their study. The authors state that the lamellae can adapt to the waviness of

surface while the setae allow for the adaptation to micro- or nano-roughness and

expect that adding the lamellae of gecko skin to the model would lead to higher

adhesion over a wider range of roughness. In addition, their hierarchicalmodel con-

siders onlynormal to surface deformationand motion of seta. It shouldbe noted that

measurements of adhesion force of a single gecko seta made by Autumn et al. [6]

demonstrated that a load applied normal to the surface was insuﬃcient for an eﬀec-

tive attachment of seta. The lateral force required to pull parallel to the surface was

observed by sliding the seta approximately 5µm laterally along the surface under

apreload.

20 Gecko Feet: Natural Hairy Attachment Systems for Smart Adhesion 1103

Fig. 20.16. The variation of adhesion force, adhesion coeﬃcient, and adhesion energy as

a function of applied loads for both one- and three-level models contacting with surface with

σ = 1 µm. The value of k

III

in the analysis is 2.908 N/m [55]

20.6.5 Capillary Eﬀects

Kim and Bhushan [58] investigated the eﬀects of capillarity on gecko adhesion by

considering capillary force as well as the solid-to-solid interaction. The Laplace

and surface tension components of the capillary force are treated according to

Sect. 20.4.2. The solid to solid adhesive force was calculated by DMT theory ac-

cording to (20.19) and will be denoted as F

DMT

The work of adhesion was then calculated by (20.20). Kim and Bhushan [58]

assumed typical values of the Hamaker constant to be H

air

= 10

−19

J in the air and

water

= 6.7×10

−19

J in the water [50]. The work of adhesions of two surfaces in

contact separated by an atomic distance D ≈ 0.2nm [50] are approximately equal to

66mJ/m

in the air and 44 mJ/m

in the water. Assuming tip radius R is 50 nm, the

DMT adhesion forces F

DMT

of a single contact in the air and the water are F

air

DMT

11nN and F

water

DMT

= 7.3nN, respectively. As the humidity increases from zero to

100%, the DMT adhesion force will take a value between F

air

DMT

and F

water

DMT

.To

calculate the DMT adhesion force for the intermediate humidity, an approximation

method by Wan et al. [91] was used. The work of adhesion W

for the intermediate

humidity can be expressed as

∞



6πh

dh=



water

6πh

dh+

∞



air

6πh

dh (20.26)

where h is the separation along the plane. h

is the water ﬁlm thickness at a ﬁlling

angle φ, which can be calculated as

= D+ R(1−cosφ) (20.27)

1104 B. Bhushan

Therefore, using (20.19), (20.26) and (20.27), the DMT adhesion force for the in-

termediate humidity is given as

DMT

= F

water

DMT



1−

(1+ R(1−cosφ)/D)



+ F

air

DMT



(1+ R(1−cosφ)/D)



(20.28)

Finally, Kim and Bhushan [58] calculated the total adhesion force F

as the sum

of (20.15) and (20.28)

= F

+ F

DMT

(20.29)

Kim and Bhushan [58] then used the total adhesion force as a critical force in the

three-level hierarchical spring model discussed previously. In the spring model for

gecko seta, if the force applied upon spring deformationis greater than the adhesion

force, the spring is regarded as having been detached.

20.6.6 Adhesion Results that Account for Capillary Eﬀects

To simulate the capillary contribution to adhesion force for a gecko spatula, Kim

and Bhushan [58] set the contact angle on gecko spatula tip θ

equal to 128 [47].

It was assumed that the spatula tip radius R = 50nm, the ambient temperature T =

◦

C, the surface tension of water γ = 73 mJ/m

and molecular volume of water

V = 0.03nm

[50].

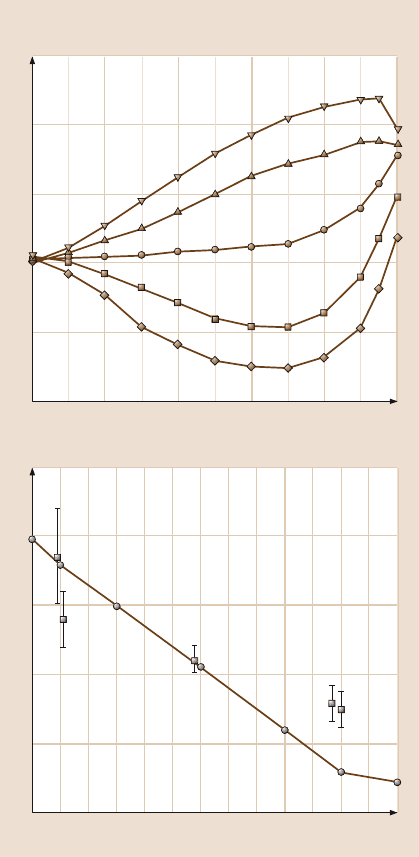
Figure 20.17a shows total adhesion force as a function of relative humidity for

a single spatula in contact with surfaces with diﬀerent contact angles. Total adhe-

sion force decreases with an increase in the contact angle on the substrate, and the

diﬀerence of total adhesion force among diﬀerent contact angles is larger in the in-

termediate humidity regime. As the relative humidity increases, total adhesion force

for the surfaces with contact angle less than 60° has higher value than the DMT

adhesion force not considering wet contact, whereas with the value above 60°, total

adhesion force has lower values at most relative humidity.

The simulation results of Kim and Bhushan [58] are compared with the experi-

mental data by Huber et al. [47] in Fig. 20.17b. Huber et al. [47] measured the

pull oﬀ force of a single spatula in contact with four diﬀerent types of Si wafer

and glass at the ambient temperature 25°C and the relative humidity 52%. Accord-

ing to their description, wafer families “N” and “T” in Fig. 20.16b diﬀer by the

thickness of the top amorphous Si oxide layer. The “Phil” type is the cleaned Si

oxide surface which is hydrophilic with a water contact angle ≈10˚, whereas the

“Phob” type is Si wafer covered hydrophobic monolayer causing water contact an-

gle > 100°. The glass has water contact angle of 58°. Huber et al. [47] showed that

the adhesion force of a gecko spatula rises signiﬁcantly for substrates with increas-

ing hydrophilicity (adhesiveforce increases by a factor of two as mating surfaces go

from hydrophobic to hydrophilic). As shown in Fig. 20.17b, the simulation results

of Kim and Bhushan [58] closely match the experimental data of Huber et al. [47].

20 Gecko Feet: Natural Hairy Attachment Systems for Smart Adhesion 1105

Contact angle (deg)θ

RV= 50 nm, = 73 mJ/m , = 0.03 nm = 128°γθ

Relative humidity (%)

a) Total adhesion force (nN)

0 100

= 10°

= 30°

= 60°

= 90°

= 110°

b) Total adhesion force (nN)

T-phil

N-phil

T-phob

N-phob

Glass

40 60 80 100 120

40 60 80

RH=52%

Fig. 20.17. (a) Total adhe-

sion force as a function of

relative humidity for a single

spatula in contact with sur-

faces with diﬀerent contact

angles. (b) Comparison of

the simulation results of Kim

and Bhushan [58] with the

measured data obtained by

Huber et al. [47] for a sin-

gle spatula in contact with

the hydrophilic and the hy-

drophobic surfaces [58]

Kim and Bhushan [58] carried out adhesion analysis for three-level hierarchical

model for gecko seta. Figure 20.18 shows the adhesion coeﬃcient and number of

contacts per unit length for the three-level hierarchical model in contact with rough

surfaces with diﬀerent values of the root mean square (RMS) amplitude σ ranging

from 0.01µmto30µm for diﬀerent relative humidities and contact angles of the

surface. It can be seen that for the surface with contact angle θ

= 10

◦

the adhesion

coeﬃcient is greatly inﬂuenced by relative humidity. At 0% relative humidity the

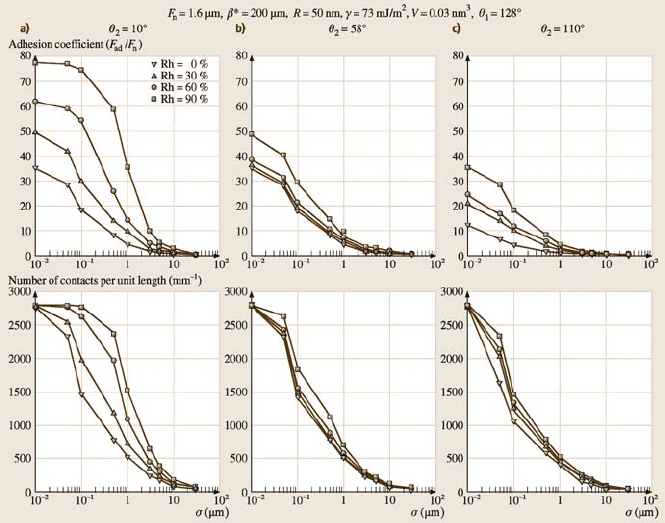
1106 B. Bhushan

Fig. 20.18. The adhesion coeﬃcient and number of contacts per unit length for three-level

hierarchical model in contact with rough surfaces with diﬀerent values of root mean square

amplitudes σ and contact angles for diﬀerent relative humidities [58]

maximum adhesion coeﬃcient is about 36 at value of σ smaller than 0.01µmcom-

pared to 78 for 90% relative humidity with the same surface roughness.As expected

the eﬀect of relativehumidity on increasing the adhesioncoeﬃcient decreases as the

contact anglebecomeslarger. For hydrophobicsurfaces, relative humiditydecreases

the adhesion coeﬃcient. Similar trends can be noticed in the number of contacts.

Thus, the conclusion can be drawn that hydrophilic surfaces are beneﬁcial to gecko

adhesion enhancement.

20.7 Modeling of Biomimetic Fibrillar Structures

The mechanics of adhesion between a ﬁbrillar structure and a rough surface as it

relates to the design of biomimetic structures has been a topic of investigation by

many researchers [31,34,35,52,56,57,69,82,94].In order to better understand the

mechanics of adhesionas it relates to design, the approach of Kim and Bhushan[57]

will be described.

Kim and Bhushan [57] developed a convenient, general and useful guideline

for understanding biological systems and for improvingthe biomimetic attachment.

20 Gecko Feet: Natural Hairy Attachment Systems for Smart Adhesion 1107

This adhesion database was constructed by modeling the ﬁbers as oriented cylin-

drical cantilever beams with spherical tips. The authors then carried out numerical

simulation of the attachment system in contact with random rough surfaces consid-

ering three constraint conditions-buckling, fracture and sticking of ﬁber structure.

For a given applied load and roughnesses of contacting surface and ﬁber material,

a procedure to ﬁnd optimal ﬁber radius and aspect ratio for the desired adhesion

coeﬃcient was developed.

The model of Kim and Bhushan [57] is used to ﬁnd the design parameters for

ﬁbers of a single-level attachment system capable of achieving desired properties –

high adhesioncoeﬃcient and durability. The design variables for an attachment sys-

tem are as follows: ﬁber geometry (radius and aspect ratio of ﬁbers, tip radius),

ﬁber material, ﬁber density and ﬁber orientation. The optimal values for the de-

sign variables to achieve the desired properties should be selected for fabrication of

a biomimetic attachment system.

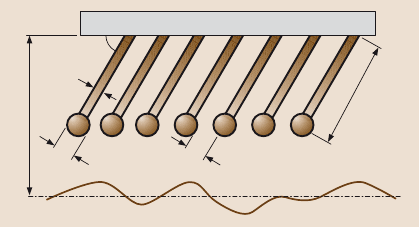
20.7.1 Fiber Model

The ﬁber model of Kim and Bhushan [57] consists of a simple idealized ﬁbrillar

structure consisting of a single-level array of micro/nano beams protruding from

a backing as shown in Fig. 20.19. The ﬁbers are modeled as oriented cylindrical

cantilever beams with spherical tips. In Fig. 20.18, l is the length of ﬁbers; θ is the

ﬁber orientation; R is the ﬁber radius; R

is the tip radius; S is the spacing between

ﬁbers; and h is distance between upper spring base of each model and mean line of

the rough proﬁle. The end terminal of the ﬁbers is assumed to be a spherical tip with

a constant radius and a constant adhesion force.

Fig. 20.19. Single-level attachment system with oriented cylindrical cantilever beams with

spherical tip. In this ﬁgure, l is the length of ﬁbers; θ is the ﬁber orientation, R is the ﬁber

radius; R

is the tip radius; S is the spacing between ﬁbers; and h is distance between base of

model and mean line of the rough proﬁle [57]

1108 B. Bhushan

20.7.2 Single Fiber Contact Analysis

Kim and Bhushan [57] modeled an individual ﬁber as a beam oriented at an angle θ

to the substrate and the contact load F is aligned normal to the substrate. The net

displacement normal to the substrate can be calculated according to (20.16) and

(20.17). The ﬁber stiﬀness (k = F/δ

⊥

) is given by [34]

k =

πR

lsin



cot



πRE

2λ sin



16λ

cot



(20.30)

where λ = l/2R is the aspect ratio of the ﬁber and θ is ﬁxed at 30°.

Two alternativemodels dominatethe worldof contactmechanics – the Johnson–

Kendall–Roberts (JKR) theory [53] for compliant solids and the Derjaguin-Muller-

Toporov (DMT) theory [26] for stiﬀ solids. Although gecko setae are composed of

β-keratin with a high elastic modulus [12, 75] which is close to the DMT model,

in general the JKR theory prevails for biological or artiﬁcial attachment systems.

Therefore the JKR theory was applied in the subsequent analysis of Kim and

Bhushan [57] to compare the materials with wide ranges of elastic modulus. The

adhesion force between a spherical tip and a rigid ﬂat surface is thus calculated

using the JKR theory as [53]

πR

(20.31)

where R

is the radius of spherical tip and W

is the work of adhesion (calculated

according to (20.24)). Kim and Bhushan [57] used this adhesion force as a critical

force. If the elastic force of a single spring is less than the adhesion force, they

regarded the spring as having been detached.

20.7.3 Constraints

In the design of ﬁbrillar structures a trade-oﬀ exists between the aspect ratio of the

ﬁbers and their adaptability to a rough surface. If the aspect ratio of the ﬁbers is

too large, they can adhere to each other or even collapse under their own weight as

shown in Fig. 20.20a. If the aspect ratio is too small (Fig. 20.20b), the structures

will lack the necessary compliance to conform to a rough surface. Spacing between

the individual ﬁbers is also important. If the spacing is too small, adjacent ﬁbers can

attract each other through intermolecularforces which will lead to bunching. There-

fore, Kim and Bhushan [57] considered three necessary conditions in their analysis;

buckling, fracture and sticking of ﬁber structure, which constrain the allowed geo-

metry.

Non-Buckling Condition

A ﬁbrillar interface can deliver a compliant response while still employing stiﬀ

materials because of bending and micro-bucklingof ﬁbers. Based on classical Euler

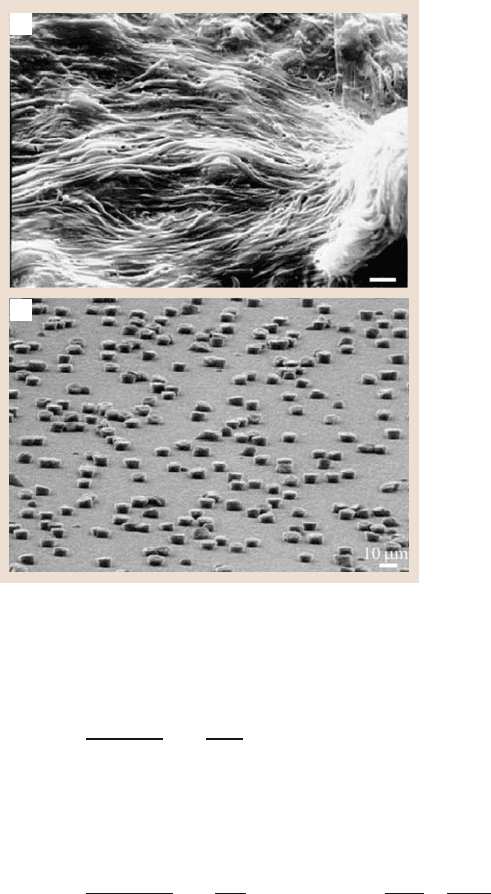
20 Gecko Feet: Natural Hairy Attachment Systems for Smart Adhesion 1109

1 μm

10 μm

Fig. 20.20. SEM micrographs

of (a) high aspect ratio poly-

mer ﬁbrils that have collapsed

under their own weight and

(b) low aspect ratio poly-

mer ﬁbrils that are incapable

of adapting to rough sur-

faces [82]

buckling, Glassmaker et al. [34] established a stress-strain relationship and a criti-

cal compressive strain for buckling, ε

, for the ﬁber oriented at an angle, θ,tothe

substrate,

= −

3(Al

/3I)



cot



(20.32)

where A

is the cross-sectional area of the ﬁbril and b

is a factor that depends

on boundary conditions. The factor b

has a value of 2 for pinned-clamped micro-

beams. For ﬁbers having a circular cross section, ε

is calculated as

= −

3(4l

/3R

)



cot



= −b



16λ

cot



(20.33)

In (20.33), ε

depends on both the aspect ratio, λ, and the orientation, θ,ofﬁbers.

If ε

= 1, which means ﬁber deforms up to the backing, buckling does not occur.

Figure 20.21 plots the critical orientation, θ, as a function of aspect ratio for the case

of ε

= 1. The critical ﬁber orientation for buckling is 90° at λ less than 1.1. This

means that the buckling does not occur regardlessof the orientation of ﬁber at λ less

than 1.1. For λ greater than 1.1, the critical ﬁber orientation for buckling decreases

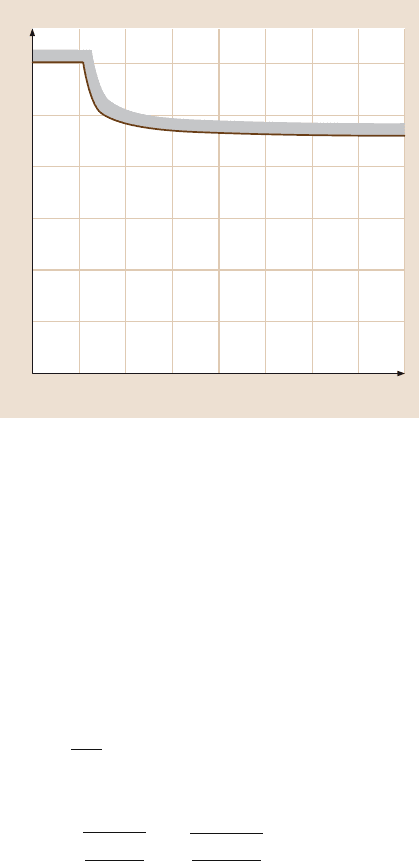
1110 B. Bhushan

θ (deg)

Non-buckling region

1234567

Fig. 20.21. Critical ﬁber ori-

entation as a function of as-

pect ratio λ for non-buckling

condition for pinned-clamped

micro-beams (b

= 2) [57]

with an increase in λ, and has a constant value of 69° at λ greater than 3. Kim and

Bhushan [57] used a ﬁxed value at 30° for θ, because as stated earlier the maximum

adhesive force is achieved at this orientation and buckling is not expected to occur.

Non-Fiber Fracture Condition

For small contacts, the strength of the system will eventually be determined by frac-

ture of the ﬁbers. Spolenak et al. [84] suggested the limit of ﬁber fracture as a func-

tion of the adhesion force. The axial stress σ

in a ﬁber is limited by its theoretical

fracture strength σ

≤ σ

(20.34)

Using (20.31), a lower limit for the useful ﬁber radius R is calculated as

R ≥



2σ

≈



15R

(20.35)

where the theoretical fracture strength is approximated by E/10 [27]. The lower

limit of ﬁber radiusfor ﬁber fractureby the adhesion forcedepends on elastic modu-

lus. By assuming W

= 66mJ/m

stated earlier, Kim and Bhushan [57] calculated

the lower limits of ﬁber radius for E = 1MPa,0.1GPaand10GPatobe0.32µm,

0.032µmand0.0032µm, respectively.

The contact stress cannot exceed the ideal contact strength transmitted through

the actual contact area at the instant of tensile instability [84].Kim and Bhushan[57]

20 Gecko Feet: Natural Hairy Attachment Systems for Smart Adhesion 1111

used this condition (20.34) to extract the limit of tip radius, R

≤ σ

(20.36)

where σ

is the contact stress and σ

is the ideal strength of van der Waals bonds

which equals approximately W

/b, b is the characteristic length of surface interac-

tion and a

is the contact radius. Based on the JKR theory, for the rigid contacting

surface, a

at the instant of pull-oﬀ is calculated as



9πW

(1−ν

)



1/3

(20.37)

where ν is Poisson ratio. The tip radius can then be calculated by combining (20.36)

and (20.37) as

≥

3π

(1−ν

)

(20.38)

The lower limit of tip radius also depends on elastic modulus. Assuming W

66mJ/m

and b = 2×10

−10

m [27], the lower limits of tip radius for E = 1MPa,

0.1GPa and 10GPa are calculated as 6×10

−7

nm, 6×10

−3

nm and 60 nm, respec-

tively. In this study, Kim and Bhushan [57] ﬁxed the tip radius at 100nm, which

satisﬁes the tip radius condition throughout a wide range of elastic modulus up to

10GPa.

Non-Sticking Condition

A high density of ﬁbers is also important for high adhesion. However, if the space S

between neighboring ﬁbers is too small, the adhesion forces between them become

stronger than the forces required to bend the ﬁbers. Then, ﬁbers might stick to each

otherand get entangled.Therefore,to preventﬁbers from stickingto each other,they

must be spaced apart and be stiﬀ enough to prevent sticking or bunching. Several

authors (e.g., [82]) have formulated a non-sticking criterion. Kim and Bhushan [57]

adopted the approach of Sitti and Fearing [82]. Both adhesion and elastic forces

will act on bent structures. The adhesion force between neighboring two round tips

is calculated as

πR



(20.39)

where R



is the reduced radius of contact, which is calculated as R



(

1/R

+ 1/R

)

−1

; R

, R

– radii of contacting tips; for the case of similar tips,

= R

, R



= 2/R

The elastic forceof a bent structure can be calculated by multiplying the bending

stiﬀness (k

= 3πR

E/4l

) by a given bending displacement δ as

πR

Eδ

(20.40)