Bennemann K.H., Ketterson J.B. Superconductivity: Volume 1: Conventional and Unconventional Superconductors; Volume 2: Novel Superconductors
Подождите немного. Документ загружается.


868 J.Zasadzinski
100. A. Nowack, U. Poppe, M. Weger, D. Schweitzer and H.Schwenk, Z. Phys. B - Condensed Matter 68, 41 (1987)
101. M.E.Hawley, K.E. Gray, B.D. Terris, H.H. Wang, K.D. Carlson and Jack M.Williams, Phys. Rev. Lett.57, 629 (1986)
102. M. Dresselhaus, G. Dresselhaus, P. Eklund, Science of Fullerenes and Carbon Nanotubes (Academic Press, San Diego,
CA,1996)
103. Laszlo Forro and Laszlo Mihaly, Rep .Prog. Phys. 64, 649 (2001)
104. S. Nolen, S.T. Ruggiero,Chem. Phys.Lett. 300, 656 (1999)
105. Y.DeWilde, M. Iavarone, U.Welp,V. Metlushko,A.E. Koshelev,I.Aranson, G.W. Crabtree and P.C. Canfield, Phys.Rev.
Lett. 78, 4273 (1997)
106. Toshikazu Ekino,H. Fuj¨u, M. Kosugi,Y. Zenitani and J.Akimitsu,Phys. Rev. B 53, 5640 (1996)
107. H. Suderow, P.Martinez-Samper,N. Luchier, J.P. Brison,S.Vieira and P.C. Canfield, Phys. Rev. B 64, 020503 (2001)
108. J.Nagamatsu, N. Nakagawa, T. Muranaka,Y. Zenitani, J.Akimitsu, Nature 410, 63 (2001)
109. H. Schmidt, J.F. Zasadzinski, K.E. Gray and D.G. Hinks, Phys. Rev. B 63, 220504 (2001)
110. G. Rubio-Bollinger, H. Suderow and S.Vieira, Phys.Rev. Lett. 86, 5582 (2001)
111. A.Y. Liu, I.I. Mazin and J.Kortus, cond-mat/0105146

16 Phase-Sensitive Tests of Pairing Symmetry in Cuprate Superconductors
C. C. Tsuei IBM Thomas J. Watson Research Center, New York, USA
J. R. Kirtley IBM Thomas J. Watson Research Center, New York, USA
16.1 Introduction .............................................................................869
16.1.1SuperconductingOrderParameterandSymmetryBreaking .............................870
16.1.2SymmetryClassificationsofthePairStates.............................................871
16.2 Phase-Sensitive Tests: Theoretical Background ...............................................874
16.2.1JosephsonSupercurrentsbetweenUnconventionalSuperconductors......................874
16.2.2PhysicsofFrustratedSuperconductingLoops..........................................876
16.3 Phase-Sensitive Tests: Experiments .........................................................880
16.3.1SQUIDInterferometry...............................................................880
16.3.2SingleJosephsonJunctionModulation.................................................882
16.3.3TricrystalMagnetometry.............................................................883
16.3.4Electron-DopedCuprateSuperconductors.............................................893
16.3.5Thin-FilmSQUIDMagnetometry.....................................................896
16.3.6SpontaneousMagnetizationinFacettedGrainBoundaries...............................897
16.3.7 c-AxisJosephsonTunneling..........................................................897
16.4 Angle-Resolved Determination of Gap Anisotropy in YBCO ...................................901
16.5 Universality of the d-Wave Pair State ........................................................902
16.5.1Optimally-DopedCuprates...........................................................903
16.5.2 Extended s-WavePairState...........................................................904
16.5.3TheEffectofDoping.................................................................905
16.5.4TemperatureDependence............................................................905
16.5.5Time-ReversalSymmetryBreaking....................................................906
16.6 Implications of d-Wave Pairing Symmetry ..................................................907
16.6.1 Universal d-WavePairStateinCuprates ...............................................907
16.6.2ConstraintsonPairingInteraction....................................................908
16.6.3NodalExcitations...................................................................910
16.6.4 Applications of d-WaveSuperconductivity.............................................911
16.7 Conclusions ..............................................................................912
References...............................................................................913
16.1 Introduction
The discovery of high-temperature superconduc-
tivity (HTS) in the cuprates [1] has stimulated a
great deal of re-thinking of some of the most ba-
sic concepts in condensed matter physics. Despite
the progress that has been made in the studies of
the superconducting and normal-state properties
of cuprate superconductors, there is still no con-
sensus on the extent to which such basic conven-
tional paradigms as the concept of the Fermi liq-
uid or the Bardeen–Cooper–Schrieffer (BCS) [2] the-
ory can be used to understand the mechanism of
high-temperature superconductivity [3]. An impor-

870 C. C. Tsuei and J.R. Kirtley
tant part of the HTS problem, the symmetry of
the pair wavefunction in cuprates, was a controver-
sial topic for more than a decade [4, 5]. Recently, a
new class of experiments have emerged that provide
phase-sensitive tests of this symmetry, producing
compelling evidence for a d
x
2
−y
2
-wave pair state in
several optimally hole– and electron-doped cuprate
superconductors [6, 7]. In this chapter, we will de-
scribe the fundamental aspects and present the main
results of these experiments. The implications of the
universally observed d-wave pairing for understand-
ing various anomalous properties of the high-T
c
su-
perconductors will be discussed. We will conclude
with potential applications of d-wave superconduc-
tivity in areas such as SQUIDs and quantum com-
puters.
16.1.1 Superconducting Order Parameter and
Symmetry Breaking
The order parameter is a fundamental concept for
characterizing the ordered state of various phase
transitions [8,9]. Ginzburg and Landau [10] intro-
duced the idea of a superconducting order param-
eter, based on the Landau theory of second-order
phase transitions, to represent the extent of macro-
scopic phase coherence in a superfluid condensate.
In their phenomenological description of the su-
perconducting state, the thermodynamic and mag-
netic properties of a superconductor are described
by a complex position-dependent order parameter
¦ (r)=|¦ (r)|e
i' (r)
, characterized by a phase '(r)
and a modulus |¦ (r)|. The local superfluid density
n
s
(r)isequalto|¦ (r)|
2
, suggesting that ¦ (r)isa
wavefunction of some sort. In the Ginzburg–Landau
formalism, the total free energy of a superconductor
is expressed in terms of the order parameter ¦ (r)
and the vector potential A(r). Minimization of the
free energy with respect to variations in ¦ (r)and
A(r) leads to the two celebrated Ginzburg–Landau
differential equations.Theseequations can be solved,
with appropriate boundary conditions,to determine
the order parameter for describing the macroscopic
properties such as critical field, critical current, and
flux lattice dynamics. Thus the superconducting or-
der parameter is, in principle, defined phenomeno-
logically in the context of the Ginzburg–Landau the-
ory. There is a huge literature on the applications of
Ginzburg–Landau theory and its extensions to a va-
riety of superconducting systems [11,12].
The microscopic significance of the phenomeno-
logical Ginzburg–Landau order parameter was es-
tablished by Gor’kov [13] shortly after the publi-
cation of the BCS theory of superconductivity. He
showed that, near T
c
, the Ginzburg–Landau equa-
tions can be derived from the BCS theory. The
Ginzburg–Landau order parameter ¦ (r)isthen
identified with the pair wavefunction, and is propor-
tional to the energy gap (r). In principle,Ginzburg–
Landau theory is only valid in a temperature range
near T
c
, due to the inherent assumption that the or-
derparameter is small and slowly varying close to the
phase transition. In practice, the Ginzburg–Landau
theory is often successfully applied well beyond its
range of validity. The equivalence between the en-
ergy gap (r) and the Ginzburg–Landau order pa-
rameter is expected to be valid for all temperatures
belowT
c
,since the superconducting order parameter
represents the degree of long–range phase coherence
in the pair state,regardless of whether it is defined at
a phenomenological or a microscopic level.
The superconducting transition temperature, T
c
,
signals the onset of a macroscopic phase-coherent
pair state [2]. An essential condition of macro-
scopic quantum phenomena such as superfluid-
ity and superconductivity is the occurrence of off-
diagonal long-range order (ODLRO) [14]. In BCS
superconductors, the ODLRO stems from a non-
vanishing anomalous expectation value of the local
pair amplitude < ¦
†
↓
(r)¦
†
↑
(r) >, which is basically
the Ginzburg–Landau order parameter. In the mo-
mentum space representation, ODLRO corresponds
to a non-zero expectation value of < c
k↑
c
−k ↓
>,
which is proportional to the gap potential (k),
the microscopic order parameter. The normal-to-
superconducting phase transition, since it involves
the onset of long-range order, is accompanied by
a lowering in symmetry. As in any second-order
phase transition, the symmetries above and below
the normal-to-superconducting state phase transi-
tion are related, since the symmetry breaking across
the transitionis continuous.Inthis context, the order

16 Phase-Sensitive Tests of Pairing Symmetry in Cuprate Superconductors 871
parameter is just a measure of the amount of sym-
metry breaking in the ordered state. The symmetry
group H describing the superconducting state must
be a subgroup of the full symmetry group G describ-
ing the normal state:
G = X × R × U(1) × T for T > T
c
(16.1)
and H ⊂ G for T ≤ T
c
, (16.2)
where X is the symmetry group of the crystal lat-
tice,R the symmetry group of spin rotation, U(1) the
one-dimensional global gauge symmetry, and T the
time-reversal symmetry operation.
The establishment of ODLRO in superconductors
below T
c
leads to a phase-coherent pair condensate
which is always characterized by a spontaneously
broken global gauge symmetry, U(1). Macroscopic
phase-coherent quantum phenomena, such as the
Meissner effect, flux quantization, and the Joseph-
son effects, are all manifestations of the global gauge
symmetry violation in the superconducting state.
Only U(1) is broken in a conventional supercon-
ductor as a result of the normal-to-superconducting
phase transition.In an unconventional superconduc-
tor,one or more symmetries in addition to U(1) can
be spontaneously broken at and below T
c
.Thedegree
of symmetry breaking occurring in the pair state is
reflected in the symmetry properties of the order pa-
rameter.
16.1.2 Symmetry Classifications of the Pair States
The pair wavefunction is antisymmetric under par-
ticle exchange as a consequence of the Pauli exclu-
sion principle. Therefore, spin singlet pairs, with to-
tal spin S = 0, can only have angular momentum
L =0, 2, 4,...quantum numbers, while spin triplet
pair states can only have odd–number angular mo-
mentum L =1, 3,...quantum numbers. All known
bulk superconductors, including the heavy-fermion
and cuprate systems, have crystal structures with a
center of inversion. Therefore superconductors can
be classified by the parity of the pair state: The spin
triplet state (total spin S = 1) has a superconduct-
ing order parameter (gap function) with odd par-
ity; the spin–singlet pair state (S = 0) has an orbital
pair wavefunction ¦ (k) ∝ (k) with even parity: i.e.
(k)=(−k). The mixing of singlet and triplet pair
states induced by spin-orbit interaction, which may
be important in heavy-fermion superconductors,can
result in an observable non-linear magneto-optical
effect due to theabsence of inversion symmetry close
to a surface [15]. In cuprate superconductors, the
spin-orbit coupling is expected to be relatively small.
Thus,the spin singlet and triplet pair states are well-
defined. It is now well-established that spin-singlet
Cooper pairing prevails in cuprate superconductors,
based on evidence from Andreev reflection and spin
susceptibility measurements [5,7].
Further classification of superconductors requires
a knowledge of possible lattice symmetries, X in
(16.1), that might be broken in the superconduct-
ing state. This assumes that the symmetry of the su-
perconducting pair wavefunction reflects that of the
underlying crystal lattice.
The Landau theory of second order phase transi-
tions states that the order parameter describing the
transition must transform according to one of the
irreducible representations of the symmetry group
of the high-temperature phase [16]. Therefore the
possible forms of the order parameter can be cat-
egorized by decomposing the representation of the
normal-state symmetry group into irreducible rep-
resentations [17].
Point-groupsymmetry classification of pair states
has been extensively studied in superfluid He [18],
heavy-fermion superconductors [19–27], and also
in cuprate superconductors [5,17,28–32]. Nearly all
group theoretic classifications of superconducting
states are based on point group symmetry. There are
exceptions when translational invariance is broken.
For example, the presence of spin density waves in
certain heavy-fermion superconductors reduces the
number of possible pair states [17,33].
Thegapfunctioncanbeexpressedasalinearcom-
bination of the basis functions(
j
) of the irreducible
representation (
j
):
(k)=
l
j
=1
j
(k) , (16.3)
where l
j
is the dimensionality of
j
,andthecomplex
number
is invariant under all symmetry opera-
tions of the normal-state group G in (16.1).

872 C. C. Tsuei and J.R. Kirtley
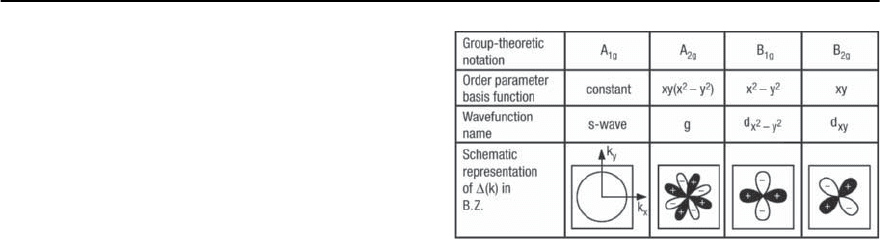
Table 16.1.Spin-singlet even-parity pair states in a tetragonal crystal with point group D
4h
Wavefunction Group Theoretic Residual Symmetry Basis Function Nodes
Name Notation, T
j
s–wave A
1g
D
4h
×T1,(x
2
+y
2
),z
2
none
g A
2g
D
4
[C
4
] ×C
i
×Txy(x
2
-y
2
)line
d
x
2
−y
2 B
1g
D
4
[D
2
] × C
i
×Tx
2
-y
2
line
d
xy
B
2g
D
4
[D
2
] × C
i
×Txyline
e
(1,0)
E
g
(1, 0) D
4
[C
2
] ×C
i
×Txzline
e
(1,1)
E
g
(1, 1) D
2
[C
"
2
] ×C
i
×T (x+y)z line
e
(1,i)
E
g
(1, i)D
4
[E] × C
i
(x+iy)z line
Table 16.2. Spin-singlet even-parity pair states in an or-
thorhombic crystal (point group D
2h
)
Group Residual Basis Nodes
Theoretic Symmetry Function
Notations
A
1g
D
2h
×T1—
B
1g
D
2
[C
z
2
] × C
i
×Txy line
B
2g
D
2
[C
y
2
] × C
i
×Txz line
B
3g
D
2
[C
x
2
] ×C
i
×Tyz line
The basis functions
j
can always be selected to
be real, so that
∗
will contain information about
the time–reversal symmetry [26]. Furthermore, as
shown by Yip and Garg [26], time-reversal symme-
try (T in (16.1)) can be broken only when the repre-
sentation is multidimensional.The basisfunctions of
the irreducible representations for various symmetry
groups are well–tabulated in the literature [17,22,25]
for various crystal structures.
The crystal structures of cuprates have been
intensively studied and well-documented [34, 35].
All cuprate superconductors have a generic lay-
ered structure with a Cu-O square lattice (the CuO
2
planes) sandwiched between charge reservoir blocks.
The crystal structure of the cuprates can be gener-
ally divided into two categories based on their atomic
arrangements: a tetragonal lattice with point group
symmetry D
4h
,and an orthorhombiclatticewith D
2h
.
Examples of the tetragonal superconductors include:
La
2−x
Sr
x
CuO
4
(LSCO), Tl
2
Ba
2
CaCu
2
O
8
(Tl-2212),
HgBa
2
CaCu
2
O
6
(Hg-1212), and Nd
1.85
Ce
0.15
CuO
4−ı
(NCCO). Structural distortions arising from Cu-O
chains, as in YBa
2
Cu
3
O
7
(YBCO), or the incommen-
surate superlattice modulation in the BiO layers,as in
Bi
2
Sr
2
CaCu
2
O
8
(Bi-2212),results in an orthorhombic
variant of the basic tetragonal crystal structure.
Allowed pair states for a tetragonal superconduc-
tor are listed in Table 16.1, for spin singlet even-
parity pairing under the standard group theoretic
constraints [29].The notation in Tables 16.1 and 16.2
is adopted from [12].The superconducting order pa-
rameter should transform like the basis function of
an irreducible representation of the relevant point
group. However, the basis function is not necessar-
ily unique [26]. An example is the case of the A
1g
(s-wave) pair state in Table 16.1. Each of the four
one–dimensional irreducible representations corre-
sponds to a single, scalar gap function of complex
numbers. Therefore, these pair states should exhibit
only one superconducting transition. For the two-
dimensional representation (E
g
),there are three pos-
sible states characterized by different residual sym-
metries (see Table 16.1).Of the three states of E
g
,only
E
g
(1, i) has broken time reversal symmetry. The gap
function(k) for each pair statecan beexpanded as a
function ofk
x
,k
y
,andk
z
,the wavevector components
along the principal axes in the Brillouin zone [30] us-
ing the basis functions listed in Table 16.1.With the
exception of the s-wave pair state, the order param-
eters have basis functions with node lines. However,
thenumber and thelocationof thenodesat the Fermi
surface depends on the Fermi surface topology, as
well as the band filling of a given bandstructure[36].
In addition to the pure states listed in Table 16.1,
the order parameter of various mixed pair states can
16 Phase-Sensitive Tests of Pairing Symmetry in Cuprate Superconductors 873
be formed by combining a real subcomponent from
one 1D-representation with an imaginary subcom-
ponent from another 1D-representation [30]. Time-
reversal symmetry is broken in all these mixed states.
We reiterate that such states can only occur if the su-
perconductivity is first order, or as a result of two
successive phase transitions. This follows from the
ideas of the Landau theory of second order phase
transitions stated earlier.
The symmetry properties of the allowed spin-
singlet even-parity pair states for a standard or-
thorhombic superconductor are tabulated in Table
16.2 [29]. Note that both the s and d
x
2
−y
2
-wave pair
statesintheorthorhombiccasebelongtothesameir-
reducible representation (A
1g
). Hence, an admixture
of these two states is allowed and only one single su-
perconducting transition should be observed.This is
apparently the case for YBCO.
Regardless of the crystal structure, tetragonal or
orthorhombic, all cuprates share one common struc-
tural feature: the CuO
2
planes. These planar Cu-O
lattices give rise to the strong anisotropy and elec-
tron correlation effects which dictate many of the
normal–state and the superconductivity properties
of this class of materials. In particular, there is
a general consensus, based on over a decade of
extensive research on HTS, that superconductivity
in all cuprates basically originates from the CuO
2
layers. Recent studies of interplane dc and ac in-
trinsic Josephson effects have convincingly demon-
strated that highly-anisotropic high-T
c
supercon-
ductors such as Bi-2212 can be modeled as stacks of
two-dimensional superconducting CuO
2
–based lay-
ers coupled by Josephson interactions [37,38]. Also,
the vortex state can be understood in terms of stacks
of two–dimensional pancake vortices with cores lo-
calized in the CuO
2
layers, which are connected by
Josephson vortices with cores confined in the non-
superconducting charge reservoir layers [39,40]. In-
vestigations of c–axis charge dynamics and trans-
port have also produced strong evidence for charge
confinement in the CuO
2
layers [41–44].Similar sup-
porting evidence is abundant in the literature.
In view of the importance of CuO
2
planes in the
HTS problem, one expects that pairing symmetry
Fig. 16.1. k-space representation of allowed symmetry basis
functions for the C
4
symmetry appropriate for the CuO
2
planes in the high-T
c
superconductors
reflects the point-group symmetry of the underly-
ing Cu-O lattices. In the tetragonal cuprates such
as LSCO, NCCO, Hg-1201, Hg-1212,Tl-2201, ...,etc.,
the Cu and O atoms arrange themselves in a simple
square lattice with point group symmetry C
4v
.The
point group C
4v
consists of the following symme-
try operations: mirror reflections with respect to the
principle crystallographic axes (x =0,y =0)and
the diagonals (x = ±y); and a fourfold (C
4
)anda
twofold (C
2
)rotationaboutthec -axis.In orthorhom-
bic cuprates such as YBCO, the CuO
2
plane takes the
form of a CuO rectangular lattice with point group
symmetry C
2v
. The symmetry elements of C
2v
differ
from those of C
4v
by the absence of C
4
and reflections
with respect to the diagonals of the rectangular unit
cell.A schematic representation in k-space for possi-
ble spin-singlet pair states in a simple square lattice
with point group symmetry C
4v
is shown in Fig. 16.1.
Each of these candidates corresponds to a distinctly
differentirreducible representation (see(16.3)).Thus
pure d-wave and s-wave pair states are not allowed to
mix in the tetragonal cuprates.In the case of theCu-O
rectangular lattice both the s and d-wave pair states
belong to the same irreducible representation (A
1g
)
because of the lower crystal symmetry. Therefore an
s + d mixed pair state is allowed.
In short, we have classified and enumerated pos-
sible spin–singlet pair states in the cuprates based
on crystal symmetry. Thus the stage is set for ex-
perimentally probing pairing symmetry in various
cuprate superconductors.

874 C. C. Tsuei and J.R. Kirtley
16.2 Phase Sensitive Tests:
Theoretical Background
Symmetry tests using a variety of non-phase sen-
sitive techniques, including nuclear magnetic reso-
nance [4,45–49], penetration depth [50–52], angle–
resolved photoemission [53–56], Josephson tun-
neling spectroscopy [57, 58], specific heat [59–62],
Raman scattering [63–65], and thermal conductiv-
ity [66–68], provide strong evidence that the gap
in cuprates such as YBCO and Bi-2212 is highly
anisotropic with nodes, consistent with d-wave pair-
ing symmetry. However, these tests are not sensitive
to the phase of the order parameter, and often de-
pend on modeling details, making for much contro-
versy. Nevertheless, these studies have produced a
large body of indirect evidence for d-wave pairing.
In recent years, there has arisen a new class of
phase–sensitive pairing symmetry tests. These sym-
metry probing techniques are based on two macro-
scopic quantum coherence phenomena: Josephson
tunneling and flux quantization.
16.2.1 Josephson Supercurrents Betw een
Unconventional Superconductors
The symmetry of a pair wavefunction can best be
probed at the junction interface as the Cooper pairs
tunnel across a Josephson junction or weak link. A
schematic drawing of a Josephson tunnel junction
is depicted in Fig. 16.2, showing the tunnel barrier
sandwiched between two junction electrodes, with
order parameters
i
(k
i
)=|
i
| e
i'
i
,wherethesub-
script i = L, R. The concept of Josephson tunnel-
Fig. 16.2. Schematic diagram of a Josephson junction be-
tween a pure d
x
2
−y
2 superconductor on the left,andasu-
perconductor with some admixture of s in a predominantly
d
x
2
−y
2 state onthe right.The gap states are assumed to align
with the crystalline axes,which are rotated by angles
L
and
R
with respect to the junction normals n
L
and n
R
on the
left and right hand sides, respectively
ing is not just limited to superconductor–insulator–
superconductor (SIS) junctions, but has been gen-
eralized to all weak-link structures consisting of
two superconductors (not necessarily identical) cou-
pled by a small region of depressed order parame-
ter [12,69,70]. The supercurrent I
s
, proportional to
the tunneling rate of Cooper pairs through the bar-
rier, as given by Josephson, can be expressed by:
I
s
= I
c
sin , (16.4)
where I
c
is the Josephson criticalcurrent,and is the
gauge-invariant phase difference [12] at the junction:
= '
L
− '
R
+
2
¥
o
R
L
A · d, (16.5)
where A is the vector potential, and d is the ele-
ment of line integration from the left electrode (L)to
the right electrode (R) across the tunneling barrier.
There have been numerous studies on the Joseph-
son effects and their applications [70]. We wish here
only to concentrate on the fact that pair tunneling,as
measured by I
s
, is sensitive to the gap symmetry and
the relative orientation of the junction electrodes.To
illustrate this point, we use the tunneling Hamilto-
nian approach first introduced by Cohen et al. [71].
The supercurrent I
s
at zero temperature is given by:
I
s
∝
k,l
| T
k,l
|
2
L
(k)
R
(l)
E
L
(k)E
R
(l)
1
(E
L
(k)+E
R
(l))
× sin(
L
−
R
) , (16.6)
where T
k,l
is the time–reversal symmetry invariant
tunneling matrix element, E
i
(k)=
2
i
(k)+
2
i
(k),
and (k) is the one-electron energy.
The tunneling matrix element T
k,l
is in general
dependent on the characteristics of the junction
electrodes and the tunnel barrier in between them.
Therefore, it is not always straightforward to de-
cipher information about pairing symmetry from
measurements of supercurrent alone, but it is pos-
sibletogainsomeinsightsintothenatureofthe
pair state. For tunnel junctionsbetween spin–singlet
(even parity) and spin-triplet (odd-parity) super-
conductors, Pals et al. [72] proved that the supercur-
rent I
s
always vanishes, up to second order in T
k,l
,

16 Phase-Sensitive Tests of Pairing Symmetry in Cuprate Superconductors 875
Table 16.3. Basis functions (n) for a Josephson junction
electrode with tetragonal crystal symmetry
Irreducible A
1g
A
2g
B
1g
B
2g
Representation s g d
x
2
−y
2 d
xy
Basis Function (n)1n
x
n
y
(n
2
x
− n
2
y
) n
2
x
− n
2
y
n
x
n
y
if the spin-orbit coupling is not large (which is ap-
parently the case for the cuprate superconductors).
The fact that pair tunneling has been observed in
many Josephson junctions made between a cuprate
superconductor anda low-T
c
conventional supercon-
ductor such as Pb or Nb constitutes an experimental
proof that the cuprates have even parity in the super-
conducting state.As can be concluded from (16.6), a
k-independent tunneling matrix element would im-
ply I
s
≡ 0 for Josephson junctions made with d-wave
superconductors.There havebeen several theoretical
studies on the Josephson effect in d-wave junctions
with emphasis on the consequences of direction–
dependent tunneling matrix elements [73–75]. In a
systematic experimental study of the Josephson ef-
fect in YBCO bicrystalgrain boundary junctions, the
observed highly anisotropic supercurrent transport
was indeed found to be consistent with a combined
effect of unconventional pairing symmetry such as
that of the d
x
2
−y
2
-wave pair state and anisotropy of
the tunneling matrix elements [76].
Although various theoretical studies [73–75] on
Josephson junctions of unconventional supercon-
ductorshave predictedwell-defined Cooper pairand
quasiparticle tunneling characteristics that, in prin-
ciple,can be used for discriminating between d-wave
and s-wave pairing symmetries,it is difficult in prac-
tice to make a quantitative comparison between the-
ory and experiment because nearly all theoretical
treatments on this topicare based on the implicit as-
sumption that the junction interface is smooth and
uniform. However, there are suggestions that super-
current transport across a junction between uncon-
ventional superconductors can be significantly al-
tered by the presence of roughness, pair breaking
states or other microstructural defects at the junc-
tion interface [57,77]. This problem is also present
even for isotropic s-wave Josephson junctions, but is
more acute for the d-wavecaseduetothedirectional
dependence of the tunneling. Therefore, the use of
the magnitude of I
s
as a probe of pairing symmetry
is sample-dependent and is not reliablefor decipher-
ing information about pairing symmetry.
To circumvent this difficulty,phase-sensitive sym-
metry tests probe instead the sign change in I
s
as
a consequence of directional tunneling processes in
junctions made of at least one unconventional super-
conductor. I
s
can be related to the group symmetry
properties of the gap functions
R,L
(k)intermsof
the irreducible representations
R,L
[17, 25, 78–81].
By minimizing the total free energy with respect to
L
and
R
, the Josephson current density J
s
,flowing
perpendicular to the junction interface from super-
conductor L to R, is given by [81]:
J
s
= t
L,R
L
(n)
R
(n) |
L
||
R
| sin = J
c
sin ,
(16.7)
where J
c
is the critical current density and
L,R
,
the basis function, is related to the gap function
(k) through (16.3),
L,R
(n)=
L,R
(n)
L,R
(n), n is
the unit vector normal to the junction interface,
L,R
(n)=|
L,R
| e
i'
L,R
,and is the gauge–invariant
phase difference as defined in (16.5). The quantity
t
L,R
is a constant characteristic of a given junction
configuration and is closely related to the tunneling
matrix element in (16.6). The basis functions (n)
for a Josephson junction electrode with tetragonal
crystal symmetry (point group C
4
), are listed in Ta-
ble 16.3, where n
x
, n
y
are the projections of the unit
vector n onto the crystallographic axes x and y re-
spectively.
For Josephson junctions between two d-wave su-
perconductors ( ˆn)=n
2
x
− n
2
y
, (16.7) reduces to the
well-known Sigrist and Rice [82] formula:
J
s
= A
s
(n
2
x
− n
2
y
)
L
(n
2
x
− n
2
y
)
R
sin , (16.8)
or in terms of
L
,
R
, the angles of the crystallo-
graphic axes with respect to the interface:
J
s
= A
s
cos(2
L
)cos(2
R
)sin, (clean) , (16.9)
where A
s
is a constant characteristic of the junc-
tion. It is implicitly assumed in the derivation of
the Sigrist–Riceformula that the junctioninterfaceis
uniform andsmooth,andthat only thepair tunneling

876 C. C. Tsuei and J.R. Kirtley
process perpendicular to thejunction interface needs
to be considered. In real Josephson junctions, espe-
cially those made with cuprates, the electron wave
vector normal to the junction interface can be sig-
nificantly distorted by interface roughness, oxygen
deficiency, faceting, strain, etc. In particular, cuprate
grain boundary junctions have inhomogeneous and
meandering junctioninterfaces which depend on the
fabrication conditions [77,83–86]. A maximum dis-
order formula for the Josephson current can be de-
rived [87], by allowing a broad distributionof angu-
lar deviations at the tunnel barrier,and by taking into
account the fact that,due to the fourfold symmetry of
CuO
2
planes,the maximum angleof deviation is /4:
J
s
= A
s
cos 2(
L
+
R
)sin (dirty) . (16.10)
The difference between (16.10) and (16.9) shows
that it is important to consider the effectsof disorder
at the junction interface in using Josephson tunnel-
ing to determine pairing symmetry. A series expan-
sion of trigonometric functions of
L,R
is needed for
a general description of the angular dependence of
the Josephson current. Such an expression was ob-
tained by Walker and Luettmer–Strathmann [80] by
writing the Ginzburg–Landau free energy of Joseph-
son coupling in the form:
F
J
= C(
L
,
R
)cos , (16.11)
and by imposing the symmetry requirements for
the tetragonal lattice: C(
L
,
R
)=C(
R
,
L
)=
C(−
L
, −
R
)=C(
L
+ ,
R
); and C(
L
+
2
,
R
)=
C(
L
,
R
) for s-wave pairing symmetry, C(
L
+
2
,
R
)=−C(
L
,
R
) for d-wave superconductivity.
With these symmetry constraints, the supercurrent
for a junction between two generalized s-wave su-
perconductors can be given as:
J
s
s
=
n,n
[C
4n,4n
cos(4n
L
)cos(4n
R
) (16.12)
+ S
4n,4n
sin(4n
L
) sin(4n
R
)] sin ,
where n, n
are positive integers including zero. Al-
ternately, (16.13) can be re-written as:
J
s
s
= {C
0,0
+ C
4,0
[cos(4
L
) + cos(4
R
)] + ···}sin .
(16.13)
For a Josephson junction between d-wave supercon-
ductors,
J
d
s
= {C
4n+2,4n
+2
(16.14)
× cos[(4n +2)
L
] cos[(4n
+2)
R
]
+ S
4n+2,4n
+2
× sin[(4n +2)
L
] sin[(4n
+2)
R
]}sin ,
which can be re-written as:
J
d
s
= {C
2,2
cos(2
L
)cos(2
R
) (16.15)
+ S
2,2
sin(2
L
) sin(2
R
)+···}sin .
It is interesting to note that, in (16.16),the first term
is just the Sigrist–Rice clean limit formula (16.9). If
S
2,2
=−C
2,2
, the sum of the first two terms leads to
the dirty limit formula (16.10).
16.2.2 Physics of Frustrated Superconducting Loops
For the purposes of this chapter, a frustrated super-
conducting loop is one that, in the absence of an
externally applied field, has a local maximum in its
free energy with zero circulating supercurrent (see,
e.g.(16.17)). Sign changes in I
c
resulting from the di-
rection dependence of the pair wavefunction can be
systematically examined by looking for the experi-
mental signatures of such frustrated loops,providing
apowerfultoolforstudyingtheinternalsymmetryof
Cooper pairs. Sign changes in I
c
due to pairing sym-
metry are arbitrary for a particular junction, since
an arbitrary phase can always be added to both sides
of the junction.However,the signs of the critical cur-
rents in a closed ring of superconductorsinterrupted
by Josephson weak links can always be assigned self-
consistently. Counting these sign changes provides a
convenient way to determine if a particular geome-
try is frustrated.A geometry with an odd number of
sign changes in the normal component of the order
parameter across the Josephson weak links around a
closed loop is a frustrated or geometry. A geome-
try with an even number of such sign changes is an
unfrustrated or 0 geometry. A negative pair tunnel-
ing critical current I
c
can be thought of (for count-
ing purposes) as a phase shift of at the junction
interface (I
s
=−| I
c
| sin =| I
c
| sin( + )).
Such phase-shifts were theoretically predicted be-
tween Josephson junctions involving unconventional

16 Phase-Sensitive Tests of Pairing Symmetry in Cuprate Superconductors 877
superconductors such as heavy fermion supercon-
ducting systems [78,88] and cuprate superconduc-
tors [82]. In addition, it has been suggested that a
-phase shift can be realized within a junction by
mechanisms unrelated to pairing symmetry such as
spin–flip scattering by magnetic impurities [89] or
indirect electron tunneling [90]. There is now ex-
perimental evidence for this second mechanism for
phase shifts [91–93]. However, as we will describe
below, there is ample evidence that the experiments
discussed in this chapter depend on pairing sym-
metry dependent phase shifts. Historically,the term
-junction has been used to describe both the situa-
tions in which phase shifts within the superconduc-
tor are caused by pairing symmetry, and in which
phase shifts within a single Josephson junction are
caused by the tunneling mechanism.We will restrict
our use of the term -junction to the latter. How-
ever, a superconducting ring with an odd number of
-shifts is frustrated [87], and will show the special
effects described below, independent of whether the
-shifts result from symmetry or tunneling mech-
anism effects. Such frustrated rings will be referred
to as -rings, independent of the mechanism for the
-phase shifts.
It is useful to distinguish two classes of geom-
etry for phase-sensitive tests of pairing symmetry
in the cuprate superconductors: “rings” and “junc-
tions”. For the present purposes a “ring” is a closed
superconducting loop interrupted by one or more
Josephson weak links; a “junction” is two slabs of
superconductor separated by a Josephson weak link.
The most striking property of frustrated super-
conducting rings and junctionsis thatthey will spon-
taneously generate circulating supercurrents and
magnetic flux when cooled through the supercon-
ducting transition temperature in zero applied ex-
ternal field. Consider first a ring with inductance L
interrupted by a single Josephson junction with crit-
ical currentI
c
,in an externally applied field sufficient
to produce ¥
a
of total flux throughthering (in theab-
sence of screening from a supercurrent I
s
circulating
around the ring).Writing the total flux ¥ through the
ring as ¥ = ¥
a
+ I
s
L, the free energy of the ring has
two contributions, from the inductive energy in the
supercurrents, and the Josephson coupling energy:
U(¥ , ¥
a
)=
¥
2
0
2L
1
¥ − ¥
a
¥
0
2
(16.16)
−
L | I
c
|
¥
0
cos[
2¥
¥
0
+ Ÿ]
,
where ¥
0
= hc/2e is the superconducting flux quan-
tum, and Ÿ=0, for a 0 and a -ring, respectively.
The ground state of the single-junction ring can
be obtained by minimizing U(¥ , ¥
a
)toobtain¥ as
afunctionof¥
a
[82] (Fig. 16.3). For a small applied
flux ¥
a
,a0-ringhas¥ < ¥
a
(diamagnetic shielding,
Fig. 16.3(a)), while a -ring has ¥ > ¥
a
(param-
agnetic shielding, Fig.16.3(b)).Paramagnetic shield-
ing in granular samples [94–99] was one of the early
hints that the cuprates had unconventional pairing
symmetry [81,82].
Plots of the free-energy vs ¥ at ¥
a
= 0 (Fig. 16.4)
showsthat the 0-ring hasa ladder of metastablestates
centered at the ground state ¥ =0,whilethe-ring
has a ladder of states shifted by ¥
0
/2fromthoseof
the 0-state, centered on a doubly degenerate ground
state. In the limit ˇ
e
=2LI
c
/¥
0
1, the 0-ring has
allowed flux states that are integer multiples of the
superconducting flux quantum
¥ = n¥
0
n =0, ±1, ±2,..., (16.17)
while the -ring has allowed flux states that are half-
integer multiples
¥ =(n +1/2)¥
0
n =0, ±1, ±2,... (16.18)
The total ground state spontaneous flux ¥ at zero
applied flux ¥
a
is plotted as a function of 2LI
c
/¥
0
in Fig. 16.5.
A frustrated junction can be modeled as having
two sections, one with no intrinsic phase shift (a 0-
section), and one with an intrinsic -phase shift (a
-section). The phase shift (r
i
)acrossthei
th
sec-
tion of the junction is a solution of the sine-Gordon
equation [100,101]
∇
2
(r
i
)=sin((r
i
)+Ÿ(r
i
))/
2
Ji
, (16.19)
where r
i
is the position along the i-th section of the
junction,
Ji
=(c
2
/8edJ
1
)
1/2
is the Josephson pen-
etration depth of the i-th section of the junction,
