Bennemann K.H., Ketterson J.B. Superconductivity: Volume 1: Conventional and Unconventional Superconductors; Volume 2: Novel Superconductors
Подождите немного. Документ загружается.


66 L.Pitaevskii
When this operator acts on the ground state
wave function of a system of N electrons,
ˆ
¦
†
˛
(
X
1
)
ˆ
¦
†
ˇ
(
X
2
)
|N it creates, generally speaking, a
superposition of excited states of the system. How-
ever, in the presence of the condensate of the Cooper
pairs, a pair can be added to the condensate. Be-
cause of the large number of pairs, such an addi-
tion does not excite the system; i.e. in the presence of
theBosecondensatetheoperator
(
2.310
)
has a non-
vanishing matrix element between ground states of
system, which differ only by the numbers of elec-
trons:
N +2|
ˆ
¦
†
˛
(
X
1
)
ˆ
¦
†
ˇ
(
X
2
)
|N. (2.311)
In the thermodynamic limit the difference between
these two states is negligible and this quantity can be
considered as an average of the operator
(
2.310
)
.The
appearance of such“anomalous averages”is a special
feature of a superconducting system.
Let us consider the two-particle Green’s function
K defined according to
(
2.309
)
. The average of the
products of the ¦ -operators can be written accord-
ing to the matrix multiplication rule. However, for
the reconstruction of the ground state of the system
in lowest approximation only the “anomalous” ma-
trix elements
(
2.311
)
are important. The BCS theory
corresponds to the following approximation of the
function K:
K
ı;˛ˇ
(
X
3
, X
4
; X
1
, X
2
)
≈
N|
ˆ
T
ˆ
¦
(
X
3
)
ˆ
¦
ı
(
X
4
)
|N +2
×
N +2|
ˆ
T
ˆ
¦
†
˛
(
X
1
)
ˆ
¦
†
ˇ
(
X
2
)
|N
. (2.312)
Thus, in a natural way, so-called anomalous Green’s
functions appear:
F
˛ˇ
(
X
1
, X
2
)
=
N|T
ˆ
¦
˛
(
X
1
)
ˆ
¦
ˇ
(
X
2
)
|N +2
, (2.313)
and
F
+
˛ˇ
(
X
1
, X
2
)
=
N +2|T
ˆ
¦
†
˛
(
X
1
)
ˆ
¦
†
ˇ
(
X
2
)
|N
. (2.314)
We must make an important remark here. In ad-
dition to the anomalous matrix elements
(
2.311
)
,the
function K also contains “normal” terms, for exam-
ple matrix elements of the operator
ˆ
¦
˛
(
X
1
)
ˆ
¦
†
ˇ
(
X
2
)
.
They are not at all small compared with the anoma-
lous terms. (On the contrary, the anomalous func-
tions F and F
+
are proportional to the gap and
consequently are exponentially small with respect to
the coupling constant g.) However, we will nonethe-
less ignore these non-anomalous matrix elements in
lowest approximation, because they do not cause the
qualitative change in the nature of the ground state
of the system (which is not the case for the anoma-
lous terms). They only give “regular" corrections to
the chemical potential and the effective mass.
Note that the wave function of the superconduct-
ing pair
˛ˇ
(
r, t
)
, which we introduced in Sect. 2.2,
is expressed in terms of F
˛ˇ
as
˛ˇ
(
r, t
)
= F
˛ˇ
(
X, X
)
, X =
(
r, t
)
. (2.315)
Like
˛ˇ
,thefunctionF
˛ˇ
is proportional to the an-
tisymmetric unit spinor of rank two I
˛ˇ
:
F
˛ˇ
(
X
1
, X
2
)
= I
˛ˇ
F
(
X
1
, X
2
)
,
F
+
˛ˇ
(
X
1
, X
2
)
= I
˛ˇ
F
+
(
X
1
, X
2
)
. (2.316)
The difference in the spinor structures of G
˛ˇ
and
F
˛ˇ
is related to the fact that G
˛ˇ
is a mixed contraco-
variant spinor while F
˛ˇ
is a contravariant one. Note
also that due to the commutation relations
(
2.294
)
the functions
(
2.316
)
are symmetrical in X
1
and X
2
:
F
(
X
1
, X
2
)
= F
(
X
2
, X
1
)
,
F
+
(
X
1
, X
2
)
= F
+
(
X
2
, X
1
)
. (2.317)
In the absence of an external field the functions G,
F and F
+
depend only on the coordinate difference
X = X
1
− X
2
. Then the function
F
(
0
)
= (2.318)
is a constant. Note also that F
+
(
0
)
=
∗
.
Our notation here is different from the one that
we used previously. Here, we use the grand canonical
Hamiltonian H
.Becauseofthis,
(
2.315
)
for
˛ˇ
does
not contain the factor e
−2it
, which we had in (2.33).
Let us now derive equations for the BCS model of
a superconductor. Using the decomposition
(
2.312
)
2 Theoretical Foundations 67
and taking into account that I
2
=−1,wecanrewrite
(
2.308
)
in the form
i
@
@t
+
∇
2
2m
+
G
(
X
)
+igF
+
(
X
)
=ı
(
X
)
. (2.319)
This equation contains the second unknown func-
tion F
+
. To derive the equation of motion for this
function,werequiretheequationofmotionfor
ˆ
¦
†
˛
,
which is the Hermitian conjugation of
(
2.307
)
:
i
@
@t
ˆ
¦
†
˛
(
r, t
)
=
∇
2
2m
+ + g
ˆ
¦
†
˛
(
r, t
)
ˆ
¦
†
ˇ
(
r, t
)
ˆ
¦
ˇ
(
r, t
)
. (2.320)
Differentiating
(
2.314
)
with respect to t
1
gives an
equation with the a nomalous two-particle Green’s
function
ˆ
T
ˆ
¦
†
a
(
X
1
)
ˆ
¦
†
(
X
1
)
ˆ
¦
(
X
1
)
ˆ
¦
†
ˇ
(
X
2
)
=
iI
˛ˇ
∗
G
(
X
1
− X
2
)
. (2.321)
As a result, we obtain the following equation for
F
+
(
X
)
:
i
@
@t
−
∇
2
2m
−
F
+
(
X
)
− ig
∗
G
(
X
)
=0. (2.322)
A delta-function term similar to the right-hand side
of
(
2.319
)
does not arise here, since the function
F
+
(
X
1
, X
2
)
,unlikeG
(
X
1
, X
2
)
,is continuous at t
1
= t
2
.
Equations
(
2.319
)
and
(
2.322
)
can be easily solved in
the momentum representation. Taking the Fourier
components of these equations, we obtain
! −
p
G
(
P
)
+ igF
+
(
P
)
=1,
! +
p
F
+
(
P
)
− ig
∗
G
(
P
)
=0, (2.323)
where P =
(
p, !
)
and
p
= p
2
/2m − . Eliminating
F
+
(
P
)
from
(
2.323
)
gives the formal solution
G
(
P
)
=
! +
p
!
2
− "
2
p
=
u
p
! − "
p
+
v
p
! + "
p
, (2.324)
where
"
p
=
2
+
2
p
. (2.325)
Here, we used the notation
= g || , (2.326)
and u
p
and v
p
are given by
(
2.217
)
.Thesolution
(
2.324
)
is only a formal one, since it does not define
the way to pass around the poles. In this section we
consider only the case of zero temperature. The case
of finite temperatures will be considered in the next
section by a different method. At T = 0 the way to
pass around the poles is given by the general equa-
tion
(
2.292
)
. Adding infinitesimal imaginary parts
according to this equation we obtain
G
(
P
)
=
u
p
! − "
p
+ i0
+
v
p
! + "
p
− i0
=
! +
p
! − "
p
+ i0
! + "
p
− i0
. (2.327)
Comparing this with
(
2.292
)
also shows that "
p
is
the energy of elementary excitations. Thus we have
recovered equation
(
2.220
)
for the spectrum. Equa-
tion
(
2.326
)
establishes a relation between the gap in
the spectrum and the anomalous Green’s function of
a superconductor. Substituting
(
2.327
)
gives
F
+
(
P
)
=
ig
! − "
p
+ i0
! + "
p
− i0
. (2.328)
Then, rewriting
(
2.318
)
in momentum space, we find
∗
=
F
+
(
P
)
d!d
3
p
(
2
)
4
. (2.329)
Substituting
(
2.328
)
,we can integrate with respect to
! by closing the contour with an infinite semicir-
cle in the upper or lower half plane. The integral is
expressed in terms of the residue at the pole:
∗
=
∗
g
2
1
"
p
d
3
p
(
2
)
3
. (2.330)
Thus we recapture
(
2.224
)
for the gap
0
at T =0.
The function
F
+
(
r
)
=
F
+
(
P
)
e
−ip·r
d!d
3
p
(
2
)
4
=
∗
g
2
e
−ip·r
"
p
d
3
p
(
2
)
3
(2.331)
68 L.Pitaevskii
describes Cooper pairs and has the physical meaning
of a pair wave function. It follows from
(
2.331
)
that
this function decreases for large r as
sin
p
F
r/
e
−r/
0
,with
0
=
p
F
m
0
. (2.332)
Thus, the coherence length
0
introduced in (2.55)
has the physical meaning of the size of a Cooper
pair. Since
0
is exponentially small, the coherence
length is very large in comparison to the interelec-
tronic spacings /p
F
.
So far we have considered a superconductor in the
absence of a magnetic field. The introduction of the
magnetic field is achieved by replacing the operator
∇ in
(
2.307
)
by
(
∇ − ieA/c
)
and correspondingly by
(
∇ + ieA/c
)
in
(
2.320
)
.In the presence of a stationary
magnetic field Green’s functions depend on r
1
and r
2
separately, but continue to depend on t
1
and t
1
only
through the differencet = t
1
−t
2
.Wecankeeptheold
spin structure of the Green’s functions, since direct
interaction of the magnetic field with spin magnetic
momenta of electrons is small and can be neglected.
Finally, the equations for the Green’s functions in the
field take the form:
i
@
@t
+
1
2m
(
∇
1
− ieA
(
r
1
)
/c
)
2
+
× G
(
X
1
, X
2
)
+ ig
(
r
1
)
F
+
(
X
1
, X
2
)
= ı
(
X
1
− X
2
)
(2.333)
and
i
@
@t
+
1
2m
(
∇
1
+ ieA
(
r
1
)
/c
)
2
+
F
+
×
(
X
1
, X
2
)
− ig
∗
(
r
1
)
G
(
X
1
, X
2
)
=0. (2.334)
It is not difficult to show that these equations are
invariant with respect to the gauge transformation
A
(
r
)
→ A
(
r
)
+ ∇
(
r
)
, (2.335)
G
(
X
1
, X
2
)
→ G
(
X
1
, X
2
)
× exp
ie
c
(
r
1
)
−
(
r
2
)
, (2.336)
F
+
(
X
1
, X
2
)
→ F
+
(
X
1
, X
2
)
× exp
−
ie
c
(
r
1
)
+
(
r
2
)
. (2.337)
Accordingly, one has
∗
(
r
)
→
∗
(
r
)
exp
−
2ie
c
(
r
)
. (2.338)
However, the momentum cut-off actually breaks the
gauge invariance. A consistent gauge-invariant the-
ory can be formulated only by using a realistic
electron–phonon interaction.
2.16 Temperature Green’s Functions
There are several approaches to using Green’s func-
tion methods to solve finite-temperature problems.
We will present here the general and elegant method
of so-called imaginary-time temperature Green’s
functions.This methodisbasedonintroducinganew
imaginary-time representation for the ¦ -operators
of the electrons.So far we used these operators in the
ordinary time-dependent Heisenberg representation
( 2.274). The imaginary-time operators
ˆ
(
r,
)
and
ˆ
+
(
r,
)
can be obtained from the Heisenberg oper-
ators
ˆ
¦
˛
(
r, t
)
and
ˆ
¦
†
˛
(
r, t
)
by replacing the variable
t by the imaginary variable (−i ):
ˆ
˛
(
r,
)
=exp
ˆ
H
ˆ
¦
˛
(
r
)
exp
−
ˆ
H
,
ˆ
+
˛
(
r,
)
=exp
ˆ
H
ˆ
¦
†
˛
(
r
)
exp
−
ˆ
H
. (2.339)
We will denote the imaginary-time operators by
oblique(“Italic”) letters.We emphasize that the oper-
ator
ˆ
+
˛
(
r,
)
is not the Hermitian-conjugate of the
operator
ˆ
˛
(
r,
)
; actually
ˆ
˛
(
r,
)
†
=
ˆ
+
˛
(
r, −
)
.
The temperature Green’s function G is defined in
terms of these operators.
8
The definitionincludesav-
eraging with respect to the statistical distribution:
G
˛ˇ
(
r
1
,
1
; r
2
,
2
)
=
−
ˆ
T
ˆ
˛
(
r
1
,
1
)
ˆ
+
ˇ
(
r
2
,
2
)
, (2.340)
8
The mathematical technique based on using these Green’s functions was introduced by T. Matsubara in 1955 [35], see
also Abrikosov et al.[36].
2 Theoretical Foundations 69
where
ˆ
T
is the symbol for “-chronological order-
ing”. It arranged the operators from right to left in
order of increasing . The sign is changed for every
interchange of Fermi operators. The brackets ...
mean averaging over the grand canonical distribu-
tion:
... =tr
1
exp
§ −
ˆ
H
T
%
...
2
, (2.341)
where tr denotes the sum of the diagonal matrix ele-
ments,and § is the grand canonical thermodynamic
potential,whichwe already used in Sect.3.3.LikeG
˛ˇ
the function G
˛ˇ
in the absence of a magnetic field is
proportional to a unit spinor: G
˛ˇ
= ı
˛ˇ
G.Thus,
G
(
r
1
,
1
; r
2
,
2
)
=
−
e
ˇ§
2
tr
e
−ˇ
ˆ
H
ˆ
T
ˆ
¦
˛
(
r
1
,
1
)
ˆ
¦
+
˛
(
r
2
,
2
)
. (2.342)
Here and below ˇ means the inverse temperature:
ˇ =1/T . (2.343)
Next,we will prove an important property of the tem-
perature Green’s functions.The function G depends
only on the“time”difference =
1
−
2
.Considerthe
case
1
2
. Then, according to
(
2.339
)
and
(
2.342
)
:
G
(
r
1
,
1
; r
2
,
2
)
=−
e
ˇ§
2
tr (2.344)
×
e
−ˇ
ˆ
H
e
2
ˆ
H
ˆ
¦
†
˛
(
r
2
)
e
−
(
2
−
1
)
ˆ
H
ˆ
¦
˛
(
r
1
)
e
−
2
ˆ
H
.
A cyclic permutationof operators in the trace reduces
this equation to:
G
(
r
1
,
1
; r
2
,
2
)
=
e
ˇ§
2
tr (2.345)
×
e
−
(
ˇ+
)
ˆ
H
ˆ
¦
†
˛
(
r
2
)
e
ˆ
H
ˆ
¦
˛
(
r
1
)
ts, 0 .
Analogous transformations give for 0:
G
(
r
1
,
1
; r
2
,
2
)
=−
e
ˇ§
2
tr (2.346)
e
−
ˆ
H
ˆ
¦
†
˛
(
r
2
)
e
−
(
ˇ−
)
ˆ
H
ˆ
¦
˛
(
r
1
)
, 0 .
These equation show that indeed G is a functiononly
of . Moreover, comparison of
(
2.346
)
and
(
2.346
)
gives an important symmetry relation:
G
(
0
)
=−G
+ ˇ
. (2.347)
Hence, it is sufficientto consider the variable in the
interval
−ˇ ≤ ≤ ˇ . (2.348)
Then
(
2.347
)
expresses G for negative through its
value for 0.
The functionG defines the thermodynamic prop-
erties of the system. For example, one can calculate
the density of the system from the equation
2G
(
r; r; → −0
)
=−
ˆ
¦
†
˛
(
r
)
ˆ
¦
˛
(
r
)
=−
N
V
. (2.349)
The density N/V can be expressed in terms of the
chemical potential ,volumeV and temperature T.
One can calculate the potential § by integration of
the equation N =−
(
@§/@N
)
T,V
.
The periodicitycondition
(
2.348
)
allows us to rep-
resent the temperature Green’s function as a Fourier
series with respect to (as opposed to a Fourier inte-
gral) of the form
G
(
r
1
, r
2
,
)
= T
∞
n=−∞
e
−i
n
G
r
1
, r
2
,
n
; (2.350)
this greatly simplifies the application of the formal-
ism. The symmetry condition
(
2.347
)
will be auto-
matically fulfilled if
n
=(2n +1)T , n =0, ±1, ±2, ±3,... (2.351)
The inverse transformation is
G
r
1
, r
2
,
n
=
ˇ
0
e
i
n
G
(
r
1
, r
2
,
)
d . (2.352)
In a spatially-uniform system G
r
1
, r
2
,
n
depends
only on the coordinate difference r = r
1
− r
2
.Then
G
r,
n
=
e
ip·r
G
p,
n
d
3
p
(
2
)
3
. (2.353)
In concluding this section note that differentiation
of
(
2.339
)
gives the equation of motion for ¦
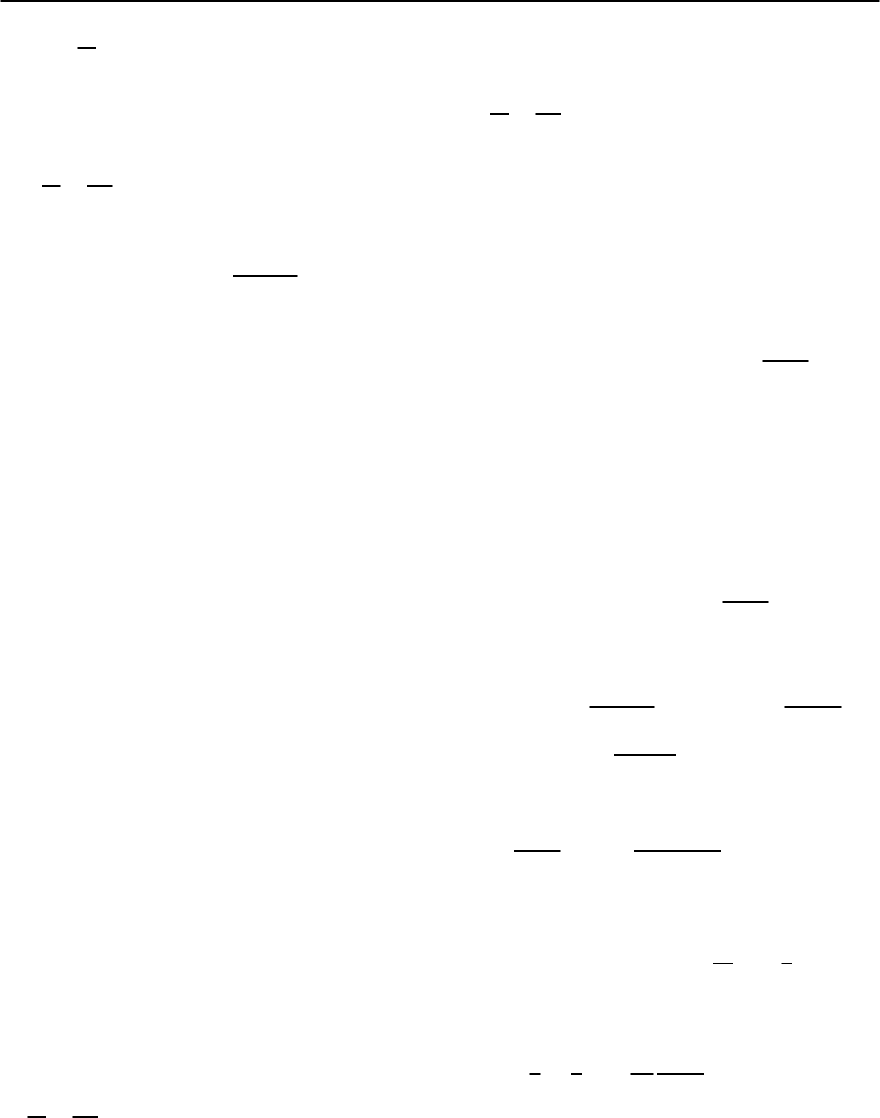
70 L.Pitaevskii
−
@
@
ˆ
¦
˛
=−
ˆ
H
0
ˆ
¦
˛
−
ˆ
¦
˛
ˆ
H
0
, (2.354)
which can be obtained from
(
2.296
)
by t → −i.An
equation of the same form is valid also for ¦
+
.For
an ideal gas we find instead of
(
2.300
)
:
−
@
@
+
∇
2
2m
+
G
(
0
)
(
r,
)
= ı
(
r
)
ı
(
)
. (2.355)
The Fourier transform of this equation gives
G
(
0
)
p,
n
=
1
i
n
−
p
. (2.356)
Since this function has no poles on the real axes of
n
, it is uniquely defined by
(
2.355
)
without any ad-
ditional conditions.
2.17 Temperature Green’s Functions
for a Superconductor
Equations for the temperature Green’s functions of
a superconductor can be derived in the same way as
was done for T =0.Anequationfortheimaginary-
time ¦ -operator can be obtained from
(
2.307
)
and
(
2.320
)
by replacing t → −i. Differentiating
(
2.340
)
fortheGreen’sfunctionleadsto thetwo-particletem-
perature Green’s function
K
ı;˛ˇ
(
X
3
, X
4
; X
1
, X
2
)
=
ˆ
T
ˆ
¦
(
X
3
)
ˆ
¦
ı
(
X
4
)
ˆ
¦
+
˛
(
X
1
)
ˆ
¦
+
ˇ
(
X
2
)
, (2.357)
where X =
(
r,
)
and ... denotes the statistical
average. In the superconducting state, the system is
characterized by the existence of the anomalous av-
erages
F
+
˛ˇ
(
X
1
, X
2
)
=
ˆ
T
ˆ
¦
+
˛
(
X
1
)
ˆ
¦
+
ˇ
(
X
2
)
= I
˛ˇ
F
+
(
X
1
, X
2
)
, (2.358)
and a similar form for F
˛ˇ
. Instead of the decompo-
sition
(
2.312
)
we then have
K
ı;˛ˇ
(
X
3
, X
4
; X
1
, X
2
)
=
F
ı
(
X
3
, X
4
)
F
+
˛ˇ
(
X
1
, X
2
)
. (2.359)
As a result we obtain for G the equation
−
@
@
+
∇
2
2m
+
G
(
X
)
+F
+
(
X
)
= ı
(
X
)
. (2.360)
The equation for F
+
is derived analogously to
(
2.322
)
.Thus,weobtain
@
@
+
∇
2
2m
+
F
+
(
X
)
−
∗
G
(
X
)
=0, (2.361)
where
∗
= gF
+
(
0
)
. (2.362)
One can easily solve this equation by Fourier trans-
formation. Then,
F
+
(
X
)
= T
∞
n=−∞
e
i
(
p·r−
n
)
× F
+
p,
n
d
3
p
(
2
)
3
,(2.363)
with
n
defined by
(
2.351
)
. The system of equations
(
2.360
)
to
(
2.362
)
takes the form
i
n
−
p
G
p,
n
+ F
+
p,
n
=1,
i
n
+
p
F
+
p,
n
+
∗
G
p,
n
=0, (2.364)
with
∗
= gT
∞
n=−∞
F
+
p,
n
d
3
p
(
2
)
3
. (2.365)
The solutions of
(
2.364
)
are
G
p,
n
=−
i
n
+
p
2
n
+ "
2
, F
+
p,
n
=
∗
2
n
+ "
2
,
(2.366)
where again " =
2
+
2
p
.Equation
(
2.365
)
for
∗
is reduced to
gT
(
2
)
3
∞
n=−∞
d
3
p
2
n
+ "
p
2
=1. (2.367)
The sum with respect to n is given by
∞
n=−∞
(
2n +1
)
2
2
+ x
2
−1
=
1
2x
tanh
x
2
. (2.368)
Finally we obtain the gap equation
g
2
1
"
tanh
"
2T
d
3
p
(
2
)
3
=1, (2.369)
which coincides with
(
2.222
)
.

2 Theoretical Foundations 71
References
1. L.D. Landau and E.M. Lifshitz, Statisticheskaya Fizika (Fizmatgiz, Moscow, 1951); In: Statistical P hysics (Pergamon
Press, Oxford 1958) Sect. 133
2. O. Penrose,Phylos. Mag. 42, 1373 (1951)
3. L. Onsager, Nuove Cimento 6, Suppl. 2, 249 and 281 (1949)
4. R.P. Feynman, In: Progress in Low Temperature Physics, ed. by C.J. Gorter (North-Holland,Amsterdam 1955)
5. L.D. Landau, J. Phys. (USSR) 5, 71 (1941)
6. J.Bardeen,L.N.Cooper,and J.R.Schrieffer,Phys.Rev.106, 162; 108, 1175 (1957)
7. L.P. Gor’kov, Sov. Phys. JETP 7, 505 (1958)
8. B.D. Josephson, Phys. Lett. 1, 251 (1962); Rev. Mod.Phys. 36, 216 (1964)
9. F. London and H London, Proc. Roy. Soc. 149, 71 (1935); Physica 2, 341 (1935)
10. A.B.Pippard,Proc.Roy.Soc.A216, 547 (1953)
11. F. London, In: Superfluids (Wiley, New York 1950) Vol. 1
12. L.D. Landau, Phys. Z. (USSR) 11, 129 (1937)
13. R.E. Peierls, Proc. Roy. Soc. A 155, 613 (1936)
14. F. London, Physica 3, 450 (1936)
15. V.L Ginzburg and L.D. Landau, Zh. Exp. Teor. Fiz. 20 1064 (1950)
16. L.D. Landau and E.M. Lifshitz, In: Statistical Physics, Part 1, 3rd edn.(Pergamon Press, Oxford 1986)
17. L.D. Landau and E.M. Lifshitz, In: Electrodynamics of Continuous Media, 2nd edn. (Pergamon Press, Oxford 1984)
18. A.A.Abrikosov, Zh. Exp. Teor. Fiz. 32, 1442 (1957)
19. D. Sain-James and P.G.De Gennes,Phys.Lett. 7, 306 (1963)
20. A.A. Abrikosov, In: Fundamentals of the Theory of Metals (North-Holland,Amsterdam,1988)
21. A.A.Abrikosov, Dokl. AN SSSR 86, 489 (1952)
22. J.R. Schrieffer, In: Theory of Superconductivity (W.A.Benjamin, New York 1964)
23. P.G. de Gennes, In: Supercpnductivity of Metals and Alloys (W.A. Benjamin, New York 1966)
24. J. Bardeen,Phys. Rev. 79, 167; 80, 567 (1950)
25. H. Fr¨ohlich,Phys. Rev.79, 1189 (1956)
26. L.N.Cooper, Phys. Rev. 104, 845 (1950)
27. N.N. Bogoliubov,Sov. Phys. JETP 7, 41 (1958); Nuovo Cimento 7, 794 (1958)
28. J.G.Valatin,Nuovo Cimento 7, 843 (1958)
29. N.N. Bogoliubov, J. Phys.(USSR)11, 23 (1947)
30. L.P. Gor’kov, T.K. Melik-Barkhudarov, Sov. Phys. JETP 13,
1018 (1961)
31. L.P. Gor’kov, Sov. Phys. JETP 9, 1364 (1959)
32. E.M. Lifshitz, L.P. Pitaevskii, In: Statistical Physics, Part 2 (Pergamon Press, Oxford 1986)
33. A.A. Abrikosov, L.P. Gor’kov and I.E. Dzyaloshinski, In: Methods of Quantum Field Theory in Statistical Physics
(Prentice-Hall, Englewood Cliffs, NJ,1963)
34. Y. Nambu,Phys. Rev. 117 648 (1960)
35. T. Matsubara, Prog. Theor. Phys. 14, 351 (1955)
36. A.A.Abrikosov, L.P. Gor’kov and I.E. Dzyaloshinski, Sov. Phys.JETP 9, 636 (1959)

3 Electron–Phonon Superconductivity
?
F. Marsig lio University of Alberta, Dept. of Physics, Edmonton, Canada
J.P. Carbotte McMaster University, Physics Dept. Hamilton, Canada
3.1 Introduction ...............................................................................74
3.2 The Electron–Phonon Interaction: Overview ...................................................74
3.2.1HistoricalDevelopments................................................................74
3.2.2Electron–IonInteraction................................................................78
3.2.3MigdalTheory.........................................................................81
3.2.4EliashbergTheory......................................................................85
3.3 The Phonons ...............................................................................92
3.3.1NeutronScattering.....................................................................92
3.3.2 The Eliashberg Function, ˛
2
F( ):Calculations.............................................93
3.3.3ExtractionfromExperiment.............................................................96
3.4 The Critical Temperature and the Energy Gap ................................................108
3.4.1ApproximateSolution:TheBCSLimit ...................................................109
3.4.2 Maximum T
c
,AsymptoticLimits,andOptimalPhononSpectra.............................109
3.4.3IsotopeEffect.........................................................................111
3.4.4TheEnergyGap.......................................................................113
3.4.5 The Energy Gap: Dependence on Coupling Strength T
c
/!
n
...............................116
3.4.6OptimalPhononSpectraandAsymptoticLimits..........................................117
3.5 Thermodynamics and Critical Magnetic Fields ...............................................118
3.5.1TheSpecificHeat......................................................................118
3.5.2CriticalMagneticFields................................................................121
3.6 Response Functions ........................................................................122
3.6.1Formalities...........................................................................123
3.6.2BCSResults...........................................................................125
3.6.3EliashbergResults ....................................................................130
3.6.4PhononResponse .....................................................................137
3.7 Anisotropy and MgB
2
......................................................................139
3.8 Summary .................................................................................145
Appendix. Microscopic Developments ...........................................................148
A.1Migdal–EliashbergTheory ..............................................................148
A.2ThePolaronProblem...................................................................150
A.3ManyElectronsonaLattice.............................................................151
References................................................................................153
A Brief Note on This Revision. The original article has been revised in several areas, mainly concerning the older
literature. No attempt has been made to account for all the updates since the first version was written. We have added
material related to structure in the bare electronic density of states (EDOS), and concerning anisotropy. In particular
the anisotropic aspects of MgB
2
have been emphasized.

74 F. Marsiglio and J.P. Carbotte
3.1 Introduction
A fairly sophisticated description of electron–pho-
non superconductivity has existed since the early
1960s, following the work of Eliashberg [1], Nambu
[2], Morel and Anderson [3], and Schrieffer et al.
[4]. All of this work extended the original ideas of
Bardeen, Cooper, and Schrieffer [5] on supercon-
ductivity, to include dynamical phonon exchange as
the root cause of the effective attractive interaction
between electrons in a metal. For certain supercon-
ductingmaterials,Eliashberg theory (as this descrip-
tion is generally called) provides a very accurate de-
scription of the superconducting state. Nonetheless,
as B.T. Matthias was fond of iterating [6], this de-
scription was never considered (by him and others)
particularly helpful for discovering new, high tem-
perature superconductors. Note, however, that Bed-
norz and M¨uller [7,8] were motivated by BCS the-
ory and various ideas that would lead to a stronger
electron–phonon coupling in metallic oxides. Part of
the problem remains that a truly accurate descrip-
tion of the normal state has not been forthcoming.
Part of that problem is the “curse” of Fermi Liquid
Theory. To the extent that the electron–phonon cou-
pling causes relatively innocuous corrections to most
normal state properties, its underlying characteris-
tics remain undetectable (indeed,as will be reviewed
here, the characteristics of the electron–phonon in-
teraction are made more apparent in the supercon-
ducting state). An exception may be the A15 com-
pounds, whose anomalous normal state properties
might help us achieve further understanding of the
electron–phonon interaction in these materials [9].
This review will barely touch upon normal state
properties influenced by the electron–phonon inter-
action.A considerable literature continues to develop
on this topic, including a more microscopic treat-
ment of model systems with simple electron–ion in-
teractions. There have been many theoretical devel-
opments in the last two decades, many of which have
been directed towards understanding the high tem-
perature oxides. Some references will be provided in
the Appendix, but, for the bulk of the chapter, we
will focus primarily on the superconducting state in
“conventional” superconductors. In the past, many
reviews have been written on the role of theelectron–
phonon interaction in superconductors.The reader is
directed in particular to the reviews by Carbotte [10],
Rainer [11], Allen and Mitrovi´c [12], and Scalapino
[13] (they are listed here in inverse chronological or-
der).Whilewehaverepeatedmuchofwhatalready
exists in these reviews, we felt it was important for
completeness in the present volume, and because the
material ispresented with a slightlydifferent outlook
than has been done in the past.
The first section provides an overview of the sub-
ject as we see it,with some details relegated to theAp-
pendix.This is followedby a discussion of our knowl-
edge of the electron–phonon interaction in metals,
including an update on old ideas to use the optical
conductivity to extract this information. The next
two sections provide a very brief review of the impact
of the electron phonon interaction on the supercon-
ducting critical temperature,the energy gap,the spe-
cific heat, and critical magnetic fields. The next sec-
tion examines dynamical response functions. Again,
largely because of the discovery of the high tem-
perature superconductors, workers were prompted
to re-examine in more detail the effect of stronger
electron–phononcoupling on various response func-
tions. For example, as will be discussed in the per-
tinent subsection, the lack of a coherence peak in
the NMR relaxation time was observed. Does this
(on its own) indicate an exotic mechanism, or can it
be explained by damping effects due to substantial
electron–phonon coupling? Answers to such ques-
tions are reviewed in this section. Finally, we end
with a summary, including some remarks on various
non-cuprate but non-conventional superconductors.
The Appendix will sketch some derivations and pro-
vide references to more recent literature.
3.2 The Electron–Phonon Interaction:
Overview
3.2.1 Historical Developments
The history of superconductivity is an immense and
fascinating subject [14]. While the discovery of su-
perconductivity occurred in 1911 [15], from a theo-
retical point of view, a first breakthrough occurred

3 Electron–Phonon Superconductivity 75
with the discovery of the Meissner–Ochsenfeld ef-
fect [16], and the understanding that this implied
that the superconducting state was a thermodynamic
phase [17]. During this time a few attempts were
made at proposing a mechanism for superconduc-
tivity (see, for example, [18]), but, by 1950, when
London’s book [19] appeared, nothing concerning
mechanism was really known [20].
In 1950 several important developments took
place [21]; first, two independent isotope effect mea-
surements were performed on Hg [22, 23], which
indicated that the superconducting transition was
intimately related to the lattice, probably through
the electron–phonon interaction.These experiments
were allthemoreremarkablebecause in 1922 Kamer-
ling Onnes and Tuyn had lookedfor an isotope effect
in superconducting Pb, and, within the experimental
accuracy of the time, had found no effect [24].
Secondly, Fr¨ohlich [25] adopted, for the first
time, a field-theoretical approach to problems in
condensed matter. In particular, he studied the
electron–phonon interaction in metals, and demon-
strated, through second order perturbation theory,
that electrons exhibit an effective attractive interac-
tion through the phonons. Although the theory as
formulated was incomplete,it did lay the foundations
for subsequent work. In fact one of the essential fea-
turesofthismechanismwassummarizedinhisin-
troduction [25]: “Nor is it accidental that very good
conductors do not become superconductors, for the
required relatively strong interaction between elec-
trons and lattice vibrationsgives rise to large normal
resistivity”. His theory correctly produced an iso-
tope effect (recognized in a Note Added in Proof ),
and, moreover, foreshadowed the discovery of the
perovskite superconductors, by suggesting that the
number of free electrons per atom shouldbereduced.
After hearing about the isotope effect measure-
ments, Bardeen also formulated a theory of su-
perconductivity based on the electron–phonon in-
teraction, wherein he determined the ground state
energy variationally [26]. Both of these theories
failed to properly explain superconductivity, essen-
tially because they focussed on the single-electron
self-energies, rather than the two-electron instabil-
ity [21]. Another breakthrough occurred a little later
when Fr¨ohlich [27] used a self-consistent method
to determine an energy lowering proportional to
exp (−1/), where is the dimensionless electron-
phonon coupling constant. This showed how essen-
tial singularities could enter the problem, and why
no perturbation expansion in would succeed in
this problem (although in fact the energy lowering is
due to a Peierls instability, notsuperconductivity).
A parallel development meanwhile had been tak-
ing place in the problem of electron propagation in
polar crystals, i.e. the study of polarons. In fact, this
problemdatesbacktoatleast1933 [28],whenLandau
first introduced the idea of a“polarization”cloud due
to the ions surrounding an electron, which, among
other things, renormalized its properties. Fr¨ohlich
also addressed this problem, first in 1937 [29], and
then again in 1950 [30]. Lee, Low and Pines [31]
subsequently took up the problem, also using field-
theoretic techniques, to provide a solution to the in-
termediate coupling polaron problem. This problem
was taken on later by Feynman [32], then by Hol-
stein and others [33], along with many others to the
present day. In fact, as described in the Appendix,
a small group of physicists continues to emphasize
polaron physics as being critical to high temperature
superconductivity in the perovskites.
Pines, having worked with Bohm on electron-
electron interactions, and having just used field-
theoretic techniques in the polaron problem, now
combined with Bardeen to derive an effective
electron–electron interaction, taking into account
both electron–electron interactions and lattice de-
grees of freedom [34]. The result was the effective
interaction Hamiltonian between two electrons with
wave vectors k and k
and energies
k
and
k
[35]:
V
eff
k,k
=
4e
2
(k − k
)
2
+ k
2
◦
×
1+
2
!
2
(k − k
)
(
k
−
k
)
2
−
2
!
2
(k − k
)
,
(3.1)
where k
◦
is the Thomas-Fermi wave vector, and !(q)
is the dressed phonon frequency.Equation(3.1) is an
effective interaction; a more formal and general ap-
proach, utilizing Green functions, will be given later.
Nonetheless, it is clear that this effective interaction

76 F. Marsiglio and J.P. Carbotte
Fig. 3.1. In (a) one electron polarizes the lattice (indicated
by dashed circles displaced towards uppermost electron);
in (b)thatelectronhasmovedaway.Inthemeantimea
second electron (seen below in (a)) is attracted to the po-
larized region,which has remained polarized long after the
first electro n has left the region. The figure is schematic
only, and does not, for example, properly convey the oppo-
site momenta such a pair should possess
captures the essence of “overscreening”, i.e. for elec-
tronic energy differences less than the phonon en-
ergy, the phonon contribution to the screened in-
teraction has the opposite sign from the electron-
ically screened interaction, and exceeds it in mag-
nitude. Physically [36], one electron makes a tran-
sition, which excites a phonon, accompanied by an
ionic charge density fluctuation. A second electron
undergoes a transition causedby this induced charge
density fluctuation. If the differences in the electron
energies is small compared to the phonon excitation
energy, the second electron is actually attracted to
thefirst.ThisisshowninFig.3.1.
Equation (3.1) represents the starting point for
the two-electron interaction in metals.It was further
simplified for both the Cooper pair calculation [37]
and the Bardeen–Cooper–Schrieffer (BCS) [5] calcu-
lation. The progression of events that ultimately led
to a successful theory for BCS has been well docu-
mented[21].Mostofthispartofthestoryhadlittleto
do with the details of the attractive mechanism, but
rather with the pairing theory itself. Thus, one can
divide the theory of superconductivity into two sep-
arate conquests: first the establishment of a pairing
formalism, which leads to a superconducting con-
densate, given some attractive particle–particle in-
teraction, and secondly, a mechanism by which two
electrons might attract one another. BCS, by simpli-
fying the interaction, succeeded in establishing the
pairing formalism. They were able to explain quite
a number of experiments, previously performed, in
progress at the time of the formulation of the theory,
and many that were to follow. However, one might
well ask to what extent the experiments support the
electron–phonon mechanism as being responsible
for superconductivity. The electron–phonon mecha-
nism has been questioned many times over the years
since BCS, not just on new superconductors, but on
conventional ones as well. Indeed, one of the elegant
outcomesof the BCS pairing formalismisthe univer-
salityofvariousproperties;atthesametimethisuni-
versality means that the theory really doesn’t distin-
guish one superconductor from another, and, more
seriously, one mechanism from another. Fortunately,
while many superconductors do display universal-
ity, some do not, and these, as it turns out, provided
very strong support for the electron–phonon mech-
anism, as initially motivated by Fr¨ohlich [25] and by
Bardeen and Pines [34]. Much of this chapter will be
concerned with these deviations from universality.
After the BCS paper appeared, several workers re-
derived their results using alternative formalisms.
For example,Anderson used an RPA treatment of the
reduced BCS Hamiltonian in terms of pseudospin
operators [38], and Bogoliubov and others [39,40]
