Bennemann K.H., Ketterson J.B. Superconductivity: Volume 1: Conventional and Unconventional Superconductors; Volume 2: Novel Superconductors

Подождите немного. Документ загружается.

13 Unconventional Superconductivity in Novel Materials 711

FS, may lead to nonequilibrium persistent currents

[498]. This effect might explain the hysteresis loops

observed in ˛-(ET)

KHg(SCN)

.Inanycase,thenew

and interesting physics of this field-induced phase

deserves further research.

Finally, magnetic field induced superconductiv-

ity (MFIS) has been found between 18 and 41 T in

-(BETS)

FeCl

[499,500]. At first glance, the evolu-

tion of a superconducting state in a magnetic field is

surprising since the magnetic field usually destroys

superconductivity. In the first experiment in mag-

netic fields up to 20 T [499], the increase of T

with

field led to speculations about unconventional pair-

ing interactions caused by magnetic ﬂuctuations via

the Fe moments. In another experiment in fields up

to 42 T, however, a more complete superconducting

phase diagram evolved as shown in Fig. 13.75 [500].

At the lowest temperature (about 0.8 K), the resis-

tance drops to zero at an in-plane field of about 18 T

and becomes finite again at a field of about 41 T. The

superconducting phase could be resolved up to about

4 K. It was also shown that only the in-plane field

component is relevant for the occurrence of super-

conductivity.

One possible explanation for the MFIS observed

in -(BETS)

FeCl

is the Jaccarino–Peter field-

compensation effect [4], as mentioned in [499]. In

this scenario the applied magnetic field aligns the

S =5/2Fe

moments, which impress an exchange

field J S on the spins s of the conduction electrons

via the exchange interaction J S · s, as described in

Sect. 13.2.4. For J < 0, the applied magnetic field

becomes compensated by the exchange field and

may reach values less than the Pauli paramagnetic

limit, thereby allowing the field-induced supercon-

ducting stateto occur.Oneofthemost striking exam-

ples of the Jaccarino–Peter effect, is provided by the

Chevrel-phase superconductor Eu

1−x

con-

taining small quantities of Br or Se (see Fig. 13.20)

[101].In this case,superconductivitywith T

≈ 3.9K

is first destroyed at about 1T and then restored above

about 4 T but only below 1 K. In -(BETS)

FeCl

,the

antiferromagnetic state has to be suppressed in high

magnetic fields before the superconducting state can

evolve out of a paramagnetic state.

TheNatureoftheSuperconductingState

For the 2D organic superconductors, the controver-

sial experimental situation still prevents a definite

conclusionas to whetherconventional BCSor uncon-

ventional superconductivity exists. It is generally ac-

cepted thatthe Cooper pairsare ina singlet spin state.

Evidence for a superconducting ground state with

singlet spin pairing includes a vanishing spin sus-

ceptibility detected by NMR experiments [501–503]

and the value of the paramagnetic-limited upper

critical field [504,712]. For the latter analysis, how-

ever, the above mentioned weak-coupling estimate

is not appropriate, since many-body effects have to

be taken into account. An elegant determination of

the Pauli limit has been performed byZuo et al.using

known thermodynamic quantitiessuchas the super-

conducting condensation energy from specific-heat

data and the Pauli spin susceptibility and is taken as

further evidence for a singlet ground state [505].

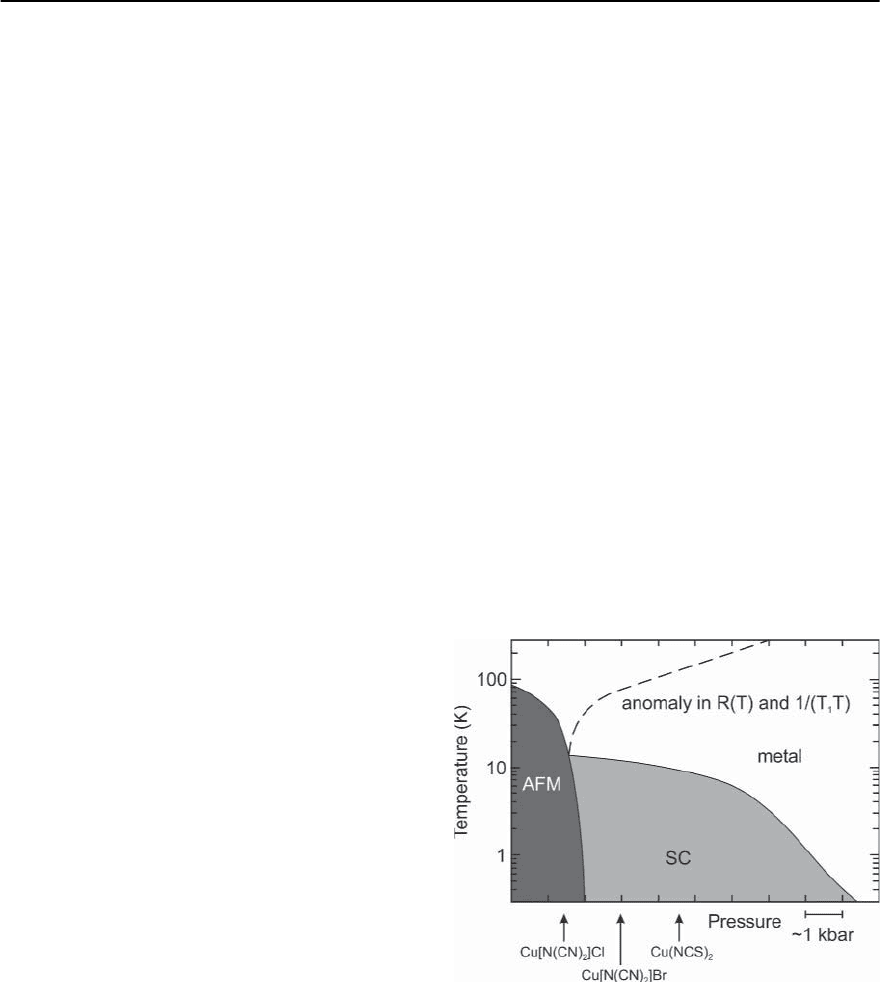
One motivation for suggesting an unconventional

order parameter, i.e., of singlet d-wave symmetry, is

the nature of the P −T phase diagram of the -phase

superconductors first proposed in [506] (Fig. 13.76).

Fig. 13.76. Schematic P −T phase diagram for the -(ET)

salts depicting regions of antiferromagnetic insulator be-

havior (AFM) and superconductivity (SC). The arrows in-

dicate starting points for the different materials at ambient

pressure

712 M.B. Maple et al.

Next to the superconducting state an antiferromag-

netic insulating state exists, similar to that observed

for the 1D Bechgaard salts and reminiscent of the

phase diagram of many high-T

materials. However,

there is only one -phase material known that dis-

plays an antiferromagnetic insulating state at am-

bient pressure that can be suppressed by pressure.

For -(ET)

Cu[N(CN)

]Cl, a pressure of 300 bar is

sufficient to induce superconductivity at a record

of about 12.8 K (Fig. 13.76) [392]. A further in-

crease in the hydrostatic pressure rapidly suppresses

the superconducting ground state. In fact, the com-

pound -(ET)

Cu(NCS)

displays the largest pres-

sure dependence of T

yet observed for any super-

conductor, T

/p ≈ −3 K/kbar [507]. Thermal-

expansion measurements revealed that T

is most

sensitive to uniaxial pressure perpendicular to the

planes [508, 509] indicating that, at least for the 

phase, an enlargement of the anion-layer thickness

might increase T

even further. However, as evi-

dencedby theP−T phase diagram,the -phase mate-

rials containing large anions reside close to an insu-

lating state,which is proposed to be a Mott–Hubbard

insulating state driven by the small bandwidth and a

large Coulomb interaction [397,398,510–512]. This

metal-insulator transition — under intense study

now — reveals an unusual criticality beyond the

known universality classes [513]. Additional stud-

ies are necessary to gain a better understanding on

the nature of this metal-insulator transition in 2D

organic metals.

A key experiment that provided evidence for the

electron–phonon interaction as the pairing interac-

tion in the early superconductors was the detection

of an isotope effect, i.e., the increase of T

propor-

tional to m

−1/2

as predicted by BCS theory. For the

organics, m is some average molecular mass. At first

sight, a somewhat confusing picture exists for the 2D

organic superconductors.Substitutionofalleighthy-

drogens in the ET ethylene end groups by deuterium

causes an enhancement of T

by about 0.3 K for

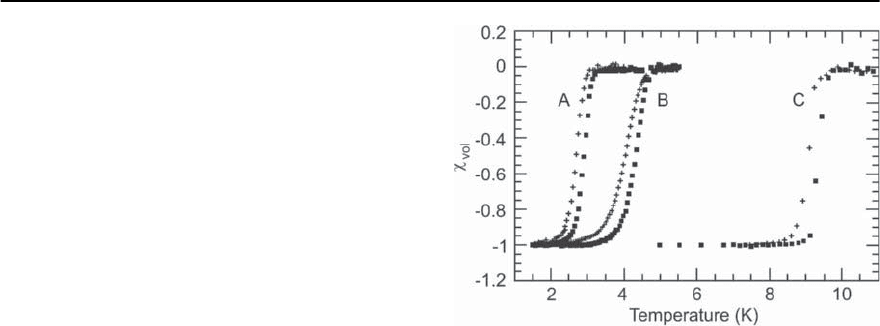
three different organic superconductors (Fig. 13.77)

[514]. This effect is not only inverse to what is ex-

pected but, in addition, the size of the T

shift is

much larger than would be explicable by a simple

molecular-mass change. Apparently, the interaction

Fig. 13.77. Representative superconducting transition

curves measured by ac susceptibility for (A) 

(ET)

Ag(CF

)

, (B) ˇ



-(ET)

,and(C) -

(ET)

Cu(NCS)

. Plus symbols are for hydrogenated, filled

squares for deuterated samples [514]

between the outer parts of the ET molecules and the

anions plays a crucial role in the pairing strength.

This interaction is expected to be rather sensitive

to uniaxial pressure perpendicular to the planes. As

mentioned, this was indeed confirmed by thermal-

expansion measurements which extracted an initial

reduction of T

by 6 K/kbar for ˇ



-(ET)

and -(ET)

Cu(NCS)

[509]. With this re-

sult in mind, the inverse isotope effect can be un-

derstood by the smaller zero-point displacement of

the carbon-deuteriumbonds compared to the lighter

carbon-hydrogen bonds. This results in a reduced

internal lattice pressure and, consequently, in an in-

creased T

for the deuterated samples [514].

In order to avoid any changes of the anion-

hydrogen interaction, experiments were carried out

on samples in which only central atoms of the ET

molecules were exchanged. Substitution of four

atoms by

Candalleight

Satomsby

Sresultedin

areductionofT

of about 1% [515]. This agrees well

with the BCS expectation and provides strong sup-

port for a Cooper-pair coupling which is at least par-

tially mediated by phonons. Additional support for

this scenario comes from Raman data which showed

a clear hardening of the Raman modes below T

for -(ET)

Cu[N(CN)

]Br [516] and from inelastic

neutron-scattering experiments [517]. In the latter

13 Unconventional Superconductivity in Novel Materials 713

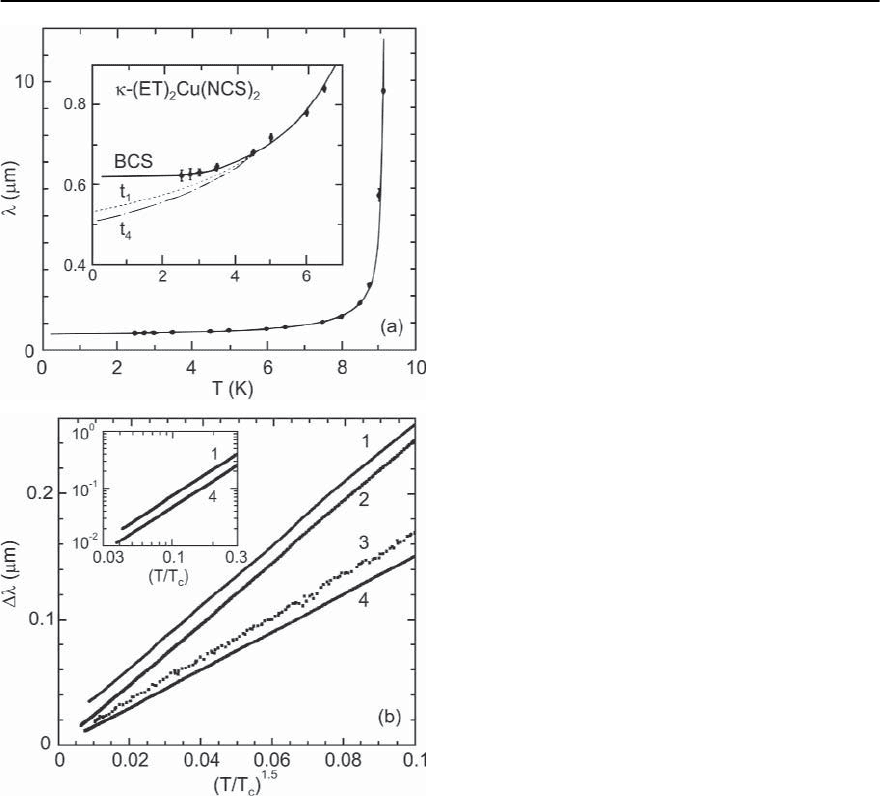
Fig. 13.78. Temperature dependences of the in-plane pen-

etration depths extracted from (a) dc-magnetization mea-

surements [518] and (b) by use of a high-frequency tunnel-

diode-oscillator technique [519]. The inset in (a) shows

the low-T data in an enlarged scale together with model

curves for BCS (solid line) and for two triplet states, t

and t

(broken curves)Thedatain(b) are for two sam-

ples of -(ET)

Cu[N(CN)

]Br (1,2) and two samples of -

(ET)

Cu(NCS)

(3,4)

measurement a significant shift of a phonon branch

was observed below T

, an effect hardly seen this

clearly in any other superconductor. Consequently,

there are a number of experiments indicating an

electron-phonon mechanism for superconductivity

in organic superconductors, whereas the argument

for a pairing mechanism mediated by antiferromag-

netic ﬂuctuations is largely based on the P − T phase

diagram (Fig. 13.76).

Apart from the coupling mechanism in the or-

ganic superconductors, the symmetry of the order

parameter is a highly controversial issue. The exper-

imental situation appears to be rather inconclusive

and only some aspects shall be presented. For fur-

ther discussion of this topic see [395,396,399–402].

One quantity which should reﬂect the symme-

try of the order parameter is the magnetic pene-

tration depth, (T). For a conventional BCS s-wave

gap, should exhibit an exponential temperature de-

pendence, whereas for a superconducting gap with

nodes a power-law T dependence is expected. The

serious experimental problems in determining  re-

liably are illustrated in Fig. 13.78. In Fig. 13.78(a)

the magnetic penetration depth is determined by

dc-magnetization measurements [518]; whereas, in

Fig. 13.78(b) the same quantity is extracted utiliz-

ing a tunnel-diode-oscillator technique [519]. In the

former experiment by Lang et al., the magnetization

data can be very well described by the conventional

weak-coupling BCS theory as shown by the solid line

in Fig. 13.78(a). The broken lines represent model

curves for two earlier suggested triplet states. In the

high-resolution data of Carrington et al. [519],a T

3/2

variation of  is found,in contrast to the BCS results

of Lang et al. as well as other groups, and, in addi-

tion, different from the T

behavior previously re-

ported by others. This inconclusive picture prevails

in a number of other experiments utilizing differ-

ent techniques which report either BCS or uncon-

ventional behavior of  (see [395,396,399–402] for

more details). The temperature dependence of the

spin-lattice relaxation rate, 1/T

,fromNMRmea-

surements is often used to obtain information about

nodes in the superconducting energy gap.Usually,an

increase of 1/T

just below T

is expected, known as

the Hebel–Slichter coherence peak (see Fig. 13.80).

Although the occurrence of this peak is an impor-

tant proof of BCS superconductivity,itsabsence can-

not disprove conventional superconductivity as ev-

714 M.B. Maple et al.

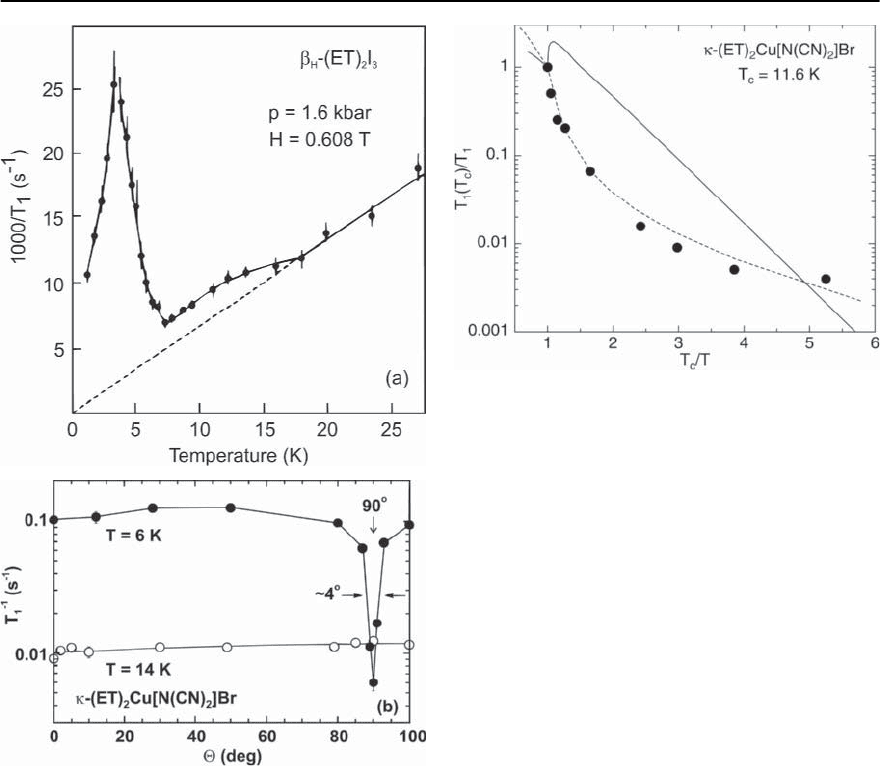
Fig. 13.79. Proton NMR spin-lattice relaxation rates T

−1

(a) Temperature dependence of 1000/T

for ˇ

-(ET)

≈ 8 K) [520, 521]. (b) Angular dependence of 1/T

for -(ET)

Cu[N(CN)

]Br (T

≈ 11.5K)aboveandbelow

[522]

idenced by the missing peak for the BCS supercon-

ductors Nb and V. At low temperatures, 1/T

is pre-

dicted to vanish exponentially for a fully-gapped su-

perconductor,and display a power-law T dependence

when the superconducting energy gap goes to zero at

points or lines on the Fermi surface (see Fig. 13.80).

Early proton-NMR experiments received partic-

ular attention when reporting an unexpected en-

Fig. 13.80. Temperature dependence of the normalized

spin-lattice relaxation rate.The solid line depicts the behav-

ior for the weak-coupling BCS superconductor aluminum

[523]. Data from

CNMRfor-(ET)

Cu[N(CN)

]Br in

fields parallel to the conducting ET planes are shown as

closed circles [501] and dashed line [502]. There is no

Hebel–Slichter peak and the relaxation rate decreases as

rather than exponentially

hancement of 1/T

by about a factor of 10 at ∼ T

as seen in Fig. 13.79(a) [520, 521]. Similar results

were reported for many other organic superconduc-

tors and caused considerable confusion at the time.

This feature was only understood a number of years

later when an NMR measurement of the angular-

dependence of 1/T

showed that the strong enhance-

ment of 1/T

below T

vanishes when the magnetic

field is aligned within the ET planes (Fig. 13.79(b)).

Below T

,1/T

exhibits a sharp dip in a narrow an-

gular region about 4

◦

wide around this field orien-

tation ( =90

◦

). It is therefore clear that this ef-

fect is connected with the ﬂux-line vortex dynam-

ics in the Shubnikov state. In the reversible region,

the ﬂux lines are free to move which results in a

rapidly changing magnetic fieldat the hydrogen sites

and, consequently, causes the strongly enhanced re-

laxation rate. However, when the magnetic field lies

within the ET planes the ﬂux lines prefer to stay

within the poorly conducting anion layers, which

results from a well-known phenomenon called in-

trinsic pinning. For this field orientation, the mag-

netic field at the hydrogen sites remains approxi-

13 Unconventional Superconductivity in Novel Materials 715

mately constant and 1/T

approaches the expected

intrinsic value. In the organic metals, the hydrogen

nucleus has a relatively weak coupling to the conduc-

tion electrons residing in the spatially distant ET lay-

ers. Therefore, the relaxation due to the moving ﬂux

lines easily dominates. This is in contrast to the sit-

uation for the cuprate superconductors, where very

large relaxation rates due to the conduction electrons

occur that mask the ﬂux-line effect.

After realizing this important additional relax-

ation channel, NMR experiments were performed

with the magnetic field aligned within the ET lay-

ersand,inaddition,the

Cnucleiwerechosenasa

local probe since they are located in the center of the

ET molecules,closer to the itinerant-electron system.

Three groups almost simultaneously reported com-

parable results [501–503], two of which are shown

schematically in Fig. 13.80. There are several marked

differences between the experimental results and the

behavior fora BCS superconductor(shown as a solid

line) [523]. First, the coherence peak just below T

is absent (although this is not conclusive evidence

for unconventional superconductivity, as mentioned

above). Second, 1/T

follows a T

dependence that

provides strong evidence for a node-like structure of

the energy gap. The rapid decrease of 1/T

just be-

low T

suggests a very fast gap opening, i.e., much

faster than is realized for a conventional supercon-

ductor. One should bear in mind, however, that all

of the NMR experiments were performed in an ap-

plied magnetic field, which might inﬂuence the gap

structure even if the vortex dynamics can be ne-

glected.

In light of the controversial issue of the symme-

try of the superconducting gap in the 2D organic

superconductors, all of the above measurements as

well as the specific-heat measurements discussed

below provide no information about the detailed

nodetopology.Therefore,the recentattempts to mea-

sure the possible anisotropy of the order parame-

ter directly received particular attention. The first

report of gap nodes in -(ET)

Cu(NCS)

[524] was

argued to be a misinterpretation of the millimeter-

wave magneto-optical data [525,526]. In other work,

scanning-tunneling spectroscopy (STM) was utilized

to measure the in-plane anisotropy of the supercon-

ducting gap [527]. The STM tip was placed on vari-

ous as-grown or in-air prepared surfaces perpendic-

ular to the interlayer direction.Strongly anisotropic

current–voltage spectra were recorded, which were

interpreted as being consistent with d-wave pairing.

In line with this result, thermal-conductivity mea-

surements in applied magnetic fields revealed a four-

fold symmetry of the electronic contribution to the

thermal conductivity [528]. Interestingly, however,

these two experiments disagree on the node direc-

tions in -(ET)

Cu(NCS)

. One should further keep

in mind that both experiments can only manifest an

asymmetry of their signals but cannot verify real zero

points of the energy gap. Furthermore, STM investi-

gations are naturally extremely sensitive to surface

states and should, therefore, be performed on well-

characterized surfaces. Finally, the thermal conduc-

tivity due to quasiparticle excitation had to be mea-

sured in an applied magnetic field leading to similar

difficulties as mentioned for the NMR experiments

above.

The proposed zero-field nodes of the supercon-

ducting gap should result in power-law temperature

dependences of the thermal conductivity and the

specific heat and finite values at T = 0. Indeed, in

one thermal-conductivity study a small residual lin-

ear contribution was reported [529]; however, it is

questionable whether the chosen extrapolation from

above ∼ 0.18 K towards low temperatures, assuming

a linear electronic and cubic phonon contribution,

is justifiedor not. Recent thermal-conductivity mea-

surements showed that the phonon-scattering length

down to lowest temperatures (T =0.25K) is strongly

inﬂuenced by quasiparticle scattering preventing the

evolution of the T

dependence expected for sample-

boundary scattering of phonons [530]. Therefore,

further studies are needed to clarify this issue.

Specific heat, C, is a powerful technique for de-

termining whether the superconducting energy gap

goes to zero at nodes on the Fermi surface. If the

quasiparticle contribution to the specific heat, C

vanishes with an exponential temperature depen-

dence in the superconducting state, nodes in the en-

ergy gapcan be ruled outunequivocally.On the other

hand, a power-law in T dependence of C

indicates

that line or point nodes may be present. However, a

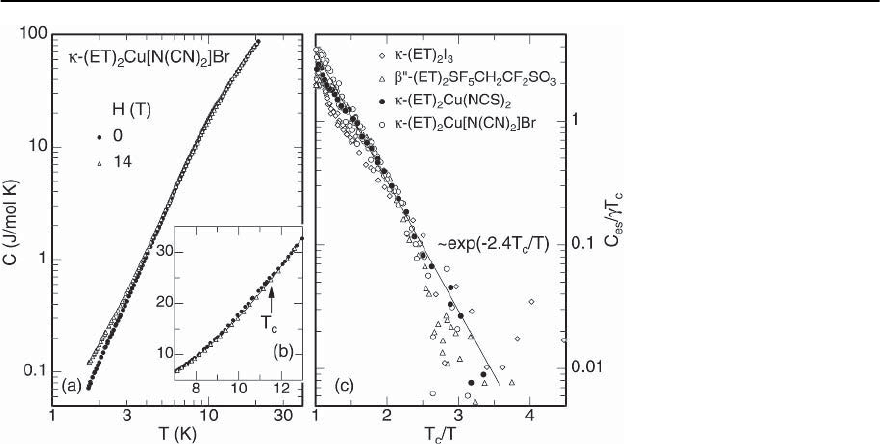
716 M.B. Maple et al.

Fig. 13.81. (a) Specific heat of -

(ET)

Cu[N(CN)

]Br in the su-

perconducting (H =0)and

normal (H = 14 T) state. (b)

The anomaly at T

=11.5K

is weakly visible in the inset.

tronic contribution to the spe-

cific heat C

in the supercon-

ducting state divided by  T

as a function of T

/T.The

solid line shows the exponen-

tial vanishing of C

towards

low T (fit to the data of -

(ET)

Cu(NCS)

)

power-law dependence in the specific heat needs to

be checked carefully since any extrinsic effect such

as a partially normal-conducting sample or an im-

proper subtraction of the nonelectronic contribu-

tions to C can obscure the exponential temperature

dependence and lead to incorrect conclusions.

Measurements of C(H,T)for-(ET)

C u [N (C N)

]Br

[531] are shown in Fig. 13.81(a) on a double-loga-

rithmic scale. The electronic contribution to C is

rather small, only about 1% at T

=11.5K.There-

fore, the specific-heat anomaly at T

can only be re-

solved in a high-resolution experiment (see the en-

largement of the data in Fig.13.81(b)). In a magnetic

field of 14 T applied perpendicular to the planes, the

sample is in the normal state. Therefore, the data at

H = 14 T comprises the phonon contribution and

thenormal-stateelectronicspecificheat T,where

the Sommerfeld coefficient  ≈ 25 mJ/mol K

.Con-

sequently, C

can be extracted by subtracting the

phonon contribution from the C data in H =0.As

evidenced in the normalized plot of Fig. 13.81(c),

vanishes exponentially for -(ET)

Cu[N(CN)

]Br

[531] as well as for three other organic superconduc-

tors [530, 532, 533]. These results are in agreement

with recent reports by other groups [534,535]. How-

ever,the C(H, T) measurements are not sensitiveto a

particular direction in k space and therefore cannot

resolve any possible anisotropy of the superconduct-

ing gap. In a very recent specific-heat experiment a

behavior in C

was resolved at lower tempera-

tures [713].Although this result would be consistent

with line nodes,the increase of  linear with H rather

points to a conventional gap symmetry.

An analysis of the specific-heat jump at T

yields

an interesting trend (Fig. 13.82). For the four super-

conductors shown,with values of T

between 3.4 and

11.5K, the specific-heat jump at T

is always larger

than the weak-couplingvalue C/ T

=1.43 and in-

creases monotonically with increasing T

[400,531].

This provides direct evidence for an increasing cou-

pling strength with T

.The dashed lines in Fig.13.82

represent the weak-coupling C dependence [536],

while the solid lines describe strong-coupling be-

havior; a BCS-like T dependence of the energy gap

(T) scaled by an appropriate parameter has been

assumed [537]. Reasonable descriptions of the ex-

perimental data are achieved for the gap ratios

˛ = (0)/k

given in Fig. 13.82, where ˛ =1.76

in the weak-coupling limit. The coupling strengths

 can be estimated [531] using phenomenological

models, yielding values ranging from ∼ 0.8 for -

(ET)

=3.4 K) to 2.5 for -(ET)

Cu[N(CN)

]Br

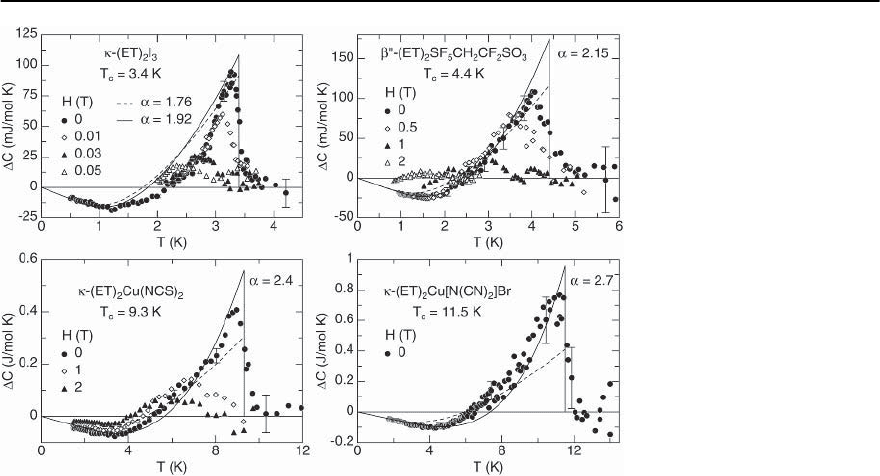
13 Unconventional Superconductivity in Novel Materials 717

Fig. 13.82. Specific heat in the

superconducting state minus

the specific heat in the nor-

mal state for four different

organic superconductors with

values of T

between 3.4 and

11.5K. The lines show the pre-

dicted BCS behavior for weak

coupling (dashed lines, ˛ =

1.76) and for strong coupling

characterized by various values

of the parameter ˛ (solid lines)

=11.5K). The large values of  are in rough

agreement with the mass renormalizations extracted

from magnetic quantum oscillations [410,449].

13.4.4 Concluding Remarks

The organic metals and superconductors show a rich

variety of novel ground statesand cover a broad spec-

trum of tunable fundamental physical phenomena

that are of current interest. One of the most stud-

ied, but still very controversial, issues is the nature

of the superconductivity. Forthe 1D Bechgaard salts,

earlier indications for triplet superconductivity had

to be corrected by recent results. For the 2D organic

superconductors, the experimental situation is un-

settled. NMR and thermal-conductivity experiments

suggest that the superconducting order parameter

is unconventional, whereas the measurements of the

specific heat indicate a nodeless gap. In an attempt

to solve this puzzling situation, it was suggested that

an s wave to d-wave gap-symmetry transition could

be induced by an in-plane magnetic field [538]. In

this theoretical treatment, phonon-mediated super-

conductivity with a small wavevector was assumed,

which could explain such a transition. Although this

proposal would resolve the controversial NMR and

specific-heat results, other predictions of this the-

ory such as the orientation of the d-wave gap nodes

are at odds with recent experimental results [528].

Further studies, especially experiments that directly

probe the phase difference of the superconducting

wave function, are highly desirable.

13.5 Layered Cuprate and Ruthenate

Superconductors

13.5.1 Introduction

The discovery of superconductivity at ∼ 30 K in the

La–Ba–Cu–O system by Bednorz and M¨uller in 1986

ignited a worldwide explosion of research on high

temperature superconductivity that still persists af-

ter more than one and a half decades. The impe-

tus behind this extraordinary episode in the his-

tory of science has been driven by two objectives:

the development of a fundamental understanding

of high temperature superconductivity and the wide

spread use of high temperature superconductors in

technological applications. Although an enormous

amount of progress has been made on these chal-

lenging problems since 1986, many obstacles remain

718 M.B. Maple et al.

to be overcome before these two objectives can be

achieved.

In this section, we give an overview of the cur-

rent knowledgeand understandingof the fundamen-

tal aspects of high T

superconductivity in cuprates.

We begin with a brief introduction to the layered

perovskite-like cuprate materials that have been

studied the most extensively as well as the struc-

turally related ruthenocuprate magnetic supercon-

ductorsand the compound Sr

RuO

,the leading can-

didate for p-wave superconductivity.The rest of this

section is devoted to a brief survey of the extraor-

dinary superconducting and normal state physical

properties of the high T

cuprate superconductors

and a discussion of some of the concepts and notions

that have been advanced to account for these prop-

erties. Specific topics discussed include: materials,

structure and charge carrier doping, superconduct-

ing state properties (critical field and current den-

sities, superconducting pairing mechanism, doping

dependence of T

, symmetry of the superconducting

order parameter), and normal state properties (non-

Fermi liquid behavior, normal ground state, pseu-

dogap, electronic inhomogeneity) and the generic

phase diagram. The enormous scope of this subject

dictated a very selective choice of the examples cited

to illustrate the progress made in this field since

its inception in 1986. For comprehensive accounts

of specific topics in high T

superconductivity, the

reader is referred to various review articles, such as

those that appear in the volumes edited by Ginsberg

[539] and by Gschneidner, Eyring and Maple [540].

The materials that are emphasized in this section in-

clude the hole-dopedsuperconductors La

2−x

CuO

(M = Ba, Sr, Ca, Na), RBa

7−ı

(R = Y or

Ln), Y

1−x

7−ı

,Bi

CaCu

8+ı

, and the

electron-doped superconductors Ln

2−x

CuO

4−y

(Ln=Pr,Nd,Sm,Eu;M=Ce,Th).

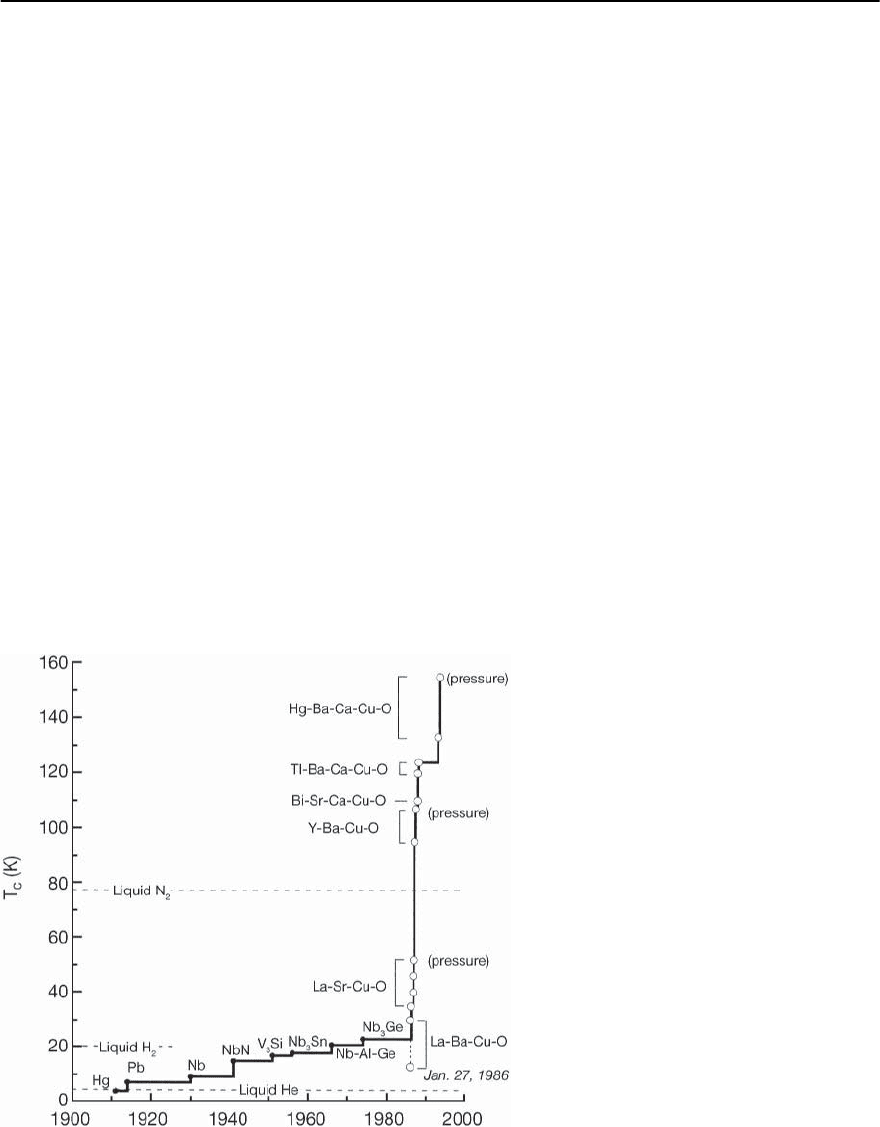
13.5.2 The Materials

Since 1986, dramatic increases of T

have been

achieved in the layered perovskite-like cuprate su-

perconductors, as illustrated in the plot of the maxi-

mum value of T

vs date in Fig. 13.83. Prior to 1986,

therecordforthehighestvalueofT

(∼ 23 K) was

heldbytheA15compoundNb

Ge [541]. Currently,

the maximum value of T

at atmospheric pressure is

∼133 K for the compound HgBa

CuO

[542,543].

When this compound is subjected to high pressures

Fig. 13.83. Maximum superconducting critical

temperature T

vs date ( [546])

13 Unconventional Superconductivity in Novel Materials 719

of ∼ 30 GPa,the T

onset increases to ∼ 164 K (more

than halfway to room temperature!) [544,545].

The first superconducting oxide system found

with T

values exceeding the 23 K record value held

by Nb

Ge was La

2−x

CuO

, which has a maximum

value of T

of ∼ 30 K for x ≈ 0.15 [547,548]. Shortly

thereafter, La

2−x

CuO

systems with M = Sr, Ca,

and Na were synthesized that exhibited supercon-

ductivity with maximum values of T

of ∼ 40 K for

M = Sr [549], ∼ 20 K for M = Ca [550], and ∼ 20 K

for M = Na [551,552].

The first superconductors with values of T

ex-

ceeding the boiling point of liquid nitrogen (77 K)

belong to the RBa

7−ı

family. Superconductiv-

ity was reported to occur for R = Y [553] and all

of the lanthanides except Ce and Tb (which do not

form the phase) and Pr (which forms the phase, but

is insulating and thereby not superconducting) with

values that range from ∼ 92 K for R = Y to

∼ 95 K for R = Nd [554]. Superconductivity at ∼ 90

K in the lanthanide analogues of YBa

7−ı

was

discovered independently in laboratories at several

institutions throughout the world. (See, for exam-

ple, [554] and references cited therein.) Surprisingly,

superconductivity with T

∼ 85 K has been reported

in PrBa

samples prepared by the traveling

ﬂoating zone method [555]. However, these mate-

rials show large inhomogeneity in both lattice pa-

rameters and transport and magnetic properties. In

the parts of the sample that exhibit bulk supercon-

ductivity, the c-axis lattice constant is reported to be

larger than that of the nonsuperconducting ceramic

polycrystalline or single crystal samples prepared by

standard methods. The pressure dependence of T

the PrBa

samples is quite large and compara-

ble to values observed in underdoped cuprates. Fur-

ther research on this fascinating material is clearly

warranted, although to date the superconductivity

reported for PrBa

has not been confirmed.

These results illustrate the complexity of these ma-

terials and how sensitive their properties are to the

conditions of their growth.

Like most high T

cuprates, the La

2−x

CuO

and

RBa

7−ı

compounds are hole-doped supercon-

ductors. The first system of electron-doped cuprate

superconductors discovered was Ln

2−x

CuO

4−y

(Ln=Pr,Nd,Sm,Eu;M=Ce,Th;x ∼ 0.1−0.18; y ≈

0.02) {(Ln = Pr, Nd, Sm; M = Ce) [556]; (Ln = Nd; M

= Th) [557]; (Ln = Pr,Sm; M = Th) [558]; (Ln = Eu; M

= Ce) [559].} The highest values of T

found among

the electron-doped superconductors are ∼ 25 K.

There has been considerable interest in systems

ofthetypeR

1−x

7−ı

, especially for R =

Y [282]. This was initially inspired by the puzzling

observation that PrBa

7−ı

, when prepared by

standard methods, is insulating and nonsupercon-

ducting,whereas the other RBa

7−ı

compounds

that can be formed are metallic and superconduct-

ing with values of T

∼ 92 − 95 K, as noted above.

The Y

1−x

7−ı

system is replete with ex-

traordinary physical phenomena including a metal-

insulator transition at x

≈ 0.55, a monotonic de-

pression of T

with x that vanishes near x

in the

metallic phase, a striking crossover in the pres-

sure dependence of T

from positive to negative

with increasing x,alarge T contribution to the

low temperature specific heat that is reminiscent

of heavy fermion behavior, and Cu

and Pr

an-

tiferromagnetic (AFM) ordering in the insulating

phase with T

(Cu

) > T

(Pr

), where T

is the

N´eel temperature. It has been suggested that these

and other peculiar properties of this system are re-

lated to hybridization between the Pr localized 4f

states and the valence band states associated with

the conducting CuO

planes [282]. A theoretical

model that involves the hybridization between the

4f states of Pr and the 2p states of neighboring

oxygen atoms in the Y

1−x

7−ı

system has,

in fact, been developed by Fehrenbacher and Rice

[560]. Similar phenomena are found in the related

1−x

system which has also been exten-

sively investigated [561].The striking behaviorof the

1−x

7−ı

system is discussed throughout

this section.

In several of the compounds containing R layers,

the R ions with partially-filled 4f electron shells and

magnetic moments have been found to order antifer-

romagnetically at lowtemperature[554,562].Specific

heat data for RBa

7−ı

with R = Nd, Sm, Gd, Er

and Dy that exhibit AFM ordering of the R ions at

low temperatures reveal pronounced peaks near the

N´eel temperature T

as shown in Fig. 13.84 [562].

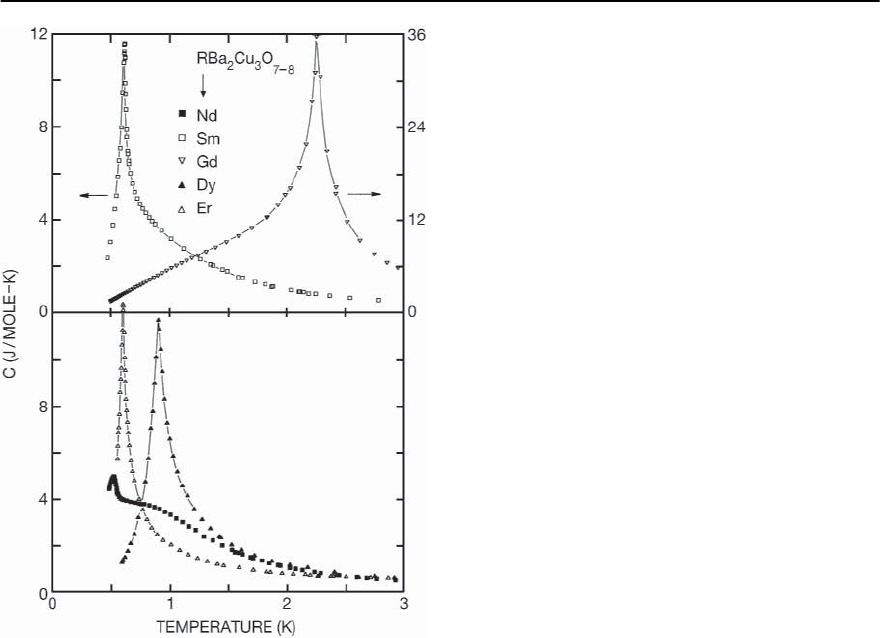
720 M.B. Maple et al.

Fig. 13.84. Specific heat C vs temperature between 0.5 K

and 3 K for RBa

7−ı

compounds with R = Nd, Sm, Gd,

Dy, and Er, after [562]

Several compounds containing CuO

and RuO

planes have been fabricated in which supercon-

ductivity and ferromagnetism, apparently confined

to the CuO

and RuO

planes, respectively, have

been reported to coexist with one another mi-

croscopically. Examples of these materials include

1.4

0.6

RuSr

10−ı

∼ 42 K, Curie temper-

ature 

∼ 180 K for R = Gd; T

∼ 32 K,

∼ 122 K

for R = Eu) [563] and GdRuSr

∼46 K,

∼

132 K) [564,565]. Magnetic order in the compound

GdRuSr

has been studied by means of neu-

tron diffraction measurements by Lynn et al. [566].

The Ru magnetic moments order antiferromagneti-

cally at T

= 136 K,coincidentwith the previously re-

ported onset of ferromagnetism. Neighboring spins

are antiparallel in all three directions,with a low tem-

perature moment of 1.18 

along the c-axis. These

measurements place an upper limit of ∼ 0.1 

any net zero field moment, with fields exceeding 0.4

tesla needed to induce a measurable magnetization.

The Gd ions order independently at T

=2.5Kwith

thesamespinconfiguration.ThevalueT

= 136 K

for AFM ordering of the Ru ions is by far the high-

est known N´eel temperature for antiferromagnetic

ordering that coexists with superconductivity. In ad-

dition to these rare earth andactinide based cuprates,

many other high T

cuprates have been discovered, a

number of which also contain rare earth elements.

The superconducting compound Sr

RuO

has the

same crystal structure as the La

2−x

CuO

(M = Ba,

Sr, Ca, Na) high T

cuprate superconductors [571].

While the T

of Sr

RuO

is only ∼ 1K,thiscom-

pound is of considerable interest because it is the

only layered perovskite-like superconductor without

Cu and it appears to be a leading candidate for p-

wave superconductivity. The anisotropy of the su-

perconducting properties of Sr

RuO

is very large

( = 

/

≈ 26), where  is the superconducting

coherence length. Although this anisotropy is larger

thanthat of La

2−x

CuO

,thein-planeandc-axis re-

sistivities of Sr

RuO

vary as T

at low temperature,

indicative of Fermi liquid behavior. Based on sim-

ilarities to

He and the existence of closely related

oxides such as SrRuO

that are ferromagnetic, it was

suggested [572,573] that Sr

RuO

may exhibit p-wave

(L = 1) spin-triplet superconductivity. In contrast,

the cuprate superconductors are generally derived

by doping the CuO

planes of antiferromagnetic par-

ent compounds by means of chemical substitutions,

apparently resulting in superconductivity with spin-

singlet pairing in a d -wave (L = 2) channel.

There is a considerable amount of evidence for

unconventional superconductivity in Sr

RuO

[569,

570,574].

O NMR Knight shift [321] and spinpolar-

ized neutron scattering [567] measurements provide

the principal evidence for spin-triplet pairing of su-

perconducting Sr

RuO

. These measurements reveal

that there is no reduction of the conduction elec-

tron spin susceptibility 

(T) in the superconduct-

ing state relative to that 

(T)inthenormalstate.

For singlet spin pairing, 

(T)shouldvanishatlow

temperatures T  T

. Although spin orbit scatter-