Bennemann K.H., Ketterson J.B. Superconductivity: Volume 1: Conventional and Unconventional Superconductors; Volume 2: Novel Superconductors

Подождите немного. Документ загружается.

13 Unconventional Superconductivity in Novel Materials 691

-0.5

0.0

0.5

1.0

1.5

2.0

2.5

3.0

012345678910

PrOs

K lom/J( T/C

)

T (K)

7.15 K

C(T) = γT + βT

+ C

Sch

(T)

Pressed pellet

0.5

0.7

0.9

1.1

1.3

1.2 1.6 2 2.4

= 1.77 K

2.0

2.5

3.0

3.5

1.4 1.6 1.8 2

Single crystals

T/C

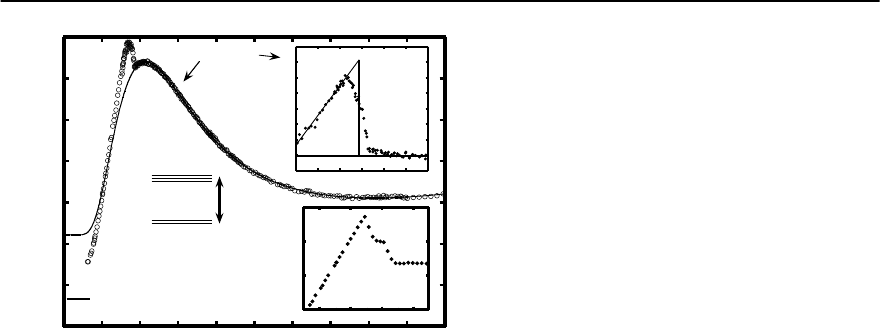
Fig. 13.54. Specific heat C divided by temperature T,

C/T,vsT for a PrOs

pressed pellet.The line repre-

sents a fit of the sum of electronic, lattice,and Schottky

contributions to the data. Upper inset: C

/T vs T near

for a PrOs

pressed pellet (C

is the electronic

contribution to C). Lower inset: C/T vs T near T

for

PrOs

single crystals, showing the structure in C

near T

. Data from [123,135]

cited f

states (i.e., 

) gives rise to a heavy Fermi-

liquid ground state [351, 354]. The Schottky-like

anomaly in specific heat and the peak in magnetic

susceptibility at T ≈ 3 K just above the supercon-

ducting transition are consistent with a 

ground

state and a low-lying 

first excited state separated

by ı ≈ 8 K, followed by 

(134 K) and 

(205 K)

excited states [123, 355, 356]. Low-temperature in-

elastic neutron scattering experiments [357,358] re-

veal peaks in intensity in the excitation spectrum at

0.72 meV (8.2K),11.4 meV (120 K), and 17.2meV

(200 K) corresponding to ground state transitions

that are in excellent agreement with this CEF energy

level scheme [123,355, 356,359]. Based on the near

isotropy of the magnetization along the [100] and

[110] directions and on the occurrence of a high-

field ordered phase (discussed below), Tayama et al.

suggested that such a 

ground state scenario bet-

ter described the properties of PrOs

than the



ground state scenario. Neutron diffraction mea-

surements reveal a small antiferromagnetic moment

(

AFM

=0.025

) parallel to the [010] direction in

magnetic fields above H ∼ 5TatT =0.25 K [360].A

crystalline electric field analysisbased ona 

ground

state suggests that antiferroquadrupolar order arises

from a level crossing of the 

ground state with an

excited state in magnetic fields, consistent with the

diffraction results. In addition, specific heat experi-

ments up to ∼ 30 T are also consistent with the evo-

lution of the 

ground state CEF level scheme in

magnetic field [361].

A number of experimental results suggest un-

conventional superconductivityin PrOs

.Recent

specific heat measurements [158,359]on single crys-

tals of PrOs

show a double superconducting

jump reminiscent of UPt

(Fig. 13.50). The two su-

perconducting transitions are estimated to occur at

=1.75 K and T

=1.85 K using an entropy

conserving construction.This double transition has

been observed for several different combinations of

single crystals as well as for individual single crystals

[158,359].In fact,theonly specific heat measurement

of PrOs

that does not show the double jump fea-

ture is the original measurement of a pressed pow-

der sample, in which a single broad transition is ob-

served, probably due to strain effects [123]. These

two transitions have also been observed in a variety

of other measurements including: thermal expan-

sion [362], magnetization [356, 363, 364], and pen-

etration depth studies [365].The thermal expansion

measurements [362] reveal that, from the Ehrenfest

relation, T

and T

have different pressure depen-

dences suggesting that they are associated with two

distinct superconducting phases. In addition, a third

superconducting phase at T

∼ 0.6 K has been pro-

posed on the basis of a kink in the lower criticalfield

and the critical current I

, possibly with a differ-

ent order parameter symmetry [366].

692 M.B. Maple et al.

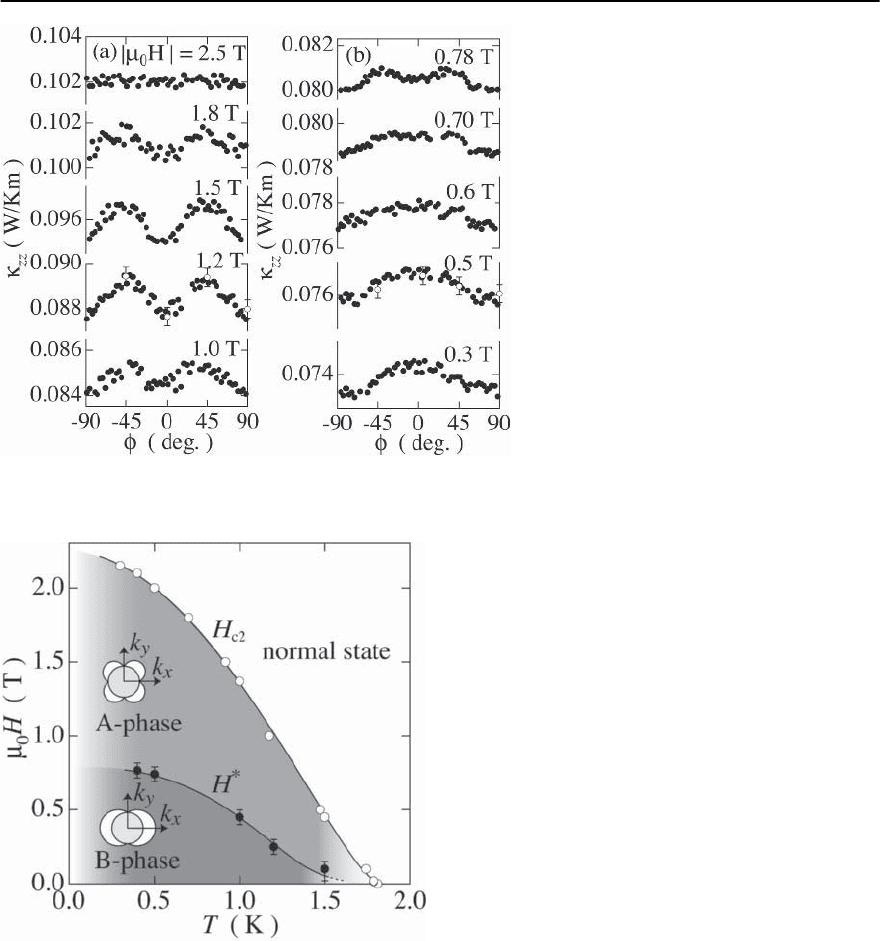
Fig. 13.55. (a) and (b):Angularvariationof



of PrOs

in a magnetic field rotating

within the ab plane at 0.52 K above and below

( 2.0 T), from [367]

Fig. 13.56. Phase diagram of the superconducting gap sym-

metry of PrOs

determined from thermal conductiv-

ity measurements. The filled circles represent the magnetic

field H

∗

at which the transition from fourfold to twofold

symmetry takes place. The open circles represent H

.The

area of the gap function with fourfold symmetry is shown

in pink (A-phase) and the area of the gap function with

twofold symmetry is shown in blue (B-phase),after [367]

The nature of the superconductivity of PrOs

was investigated by means of thermal transport mea-

surements in aligned magnetic fields by Izawa and

coworkers [367]. The thermal transport shows an

anisotropy as the magnetic field is rotated in the a−b

crystallographic plane as shown in Fig. 13.55, which

is coupled to anisotropy in the superconducting or-

der parameter. As the temperature is lowered below

, the thermal transport changes symmetry from

fourfold to twofold in the ab plane. Group theoret-

ical arguments given the cubic crystal structure of

PrOs

suggest that the high-temperature super-

conducting phase between T

and T

has six point

nodes, whereas the low temperature phase below T

has two point nodes.

The compound PrOs

is the first heavy-ferm-

ion superconductor for which there is evidence of

point nodes rather than line nodes in the supercon-

ducting order parameter.Izawa et al. also established

the H − T phase diagram of the two superconduct-

ing phases from thermal transport measurements

as shown in Fig. 13.56, in which T

is suppressed

by 25 kOe, and T

is suppressed by 7 kOe. On the

other hand, recent transverse field ‹SR [368] and

Sb-NQR measurements [369] on PrOs

are con-

sistent with an isotropic energy gap. Along with the

13 Unconventional Superconductivity in Novel Materials 693

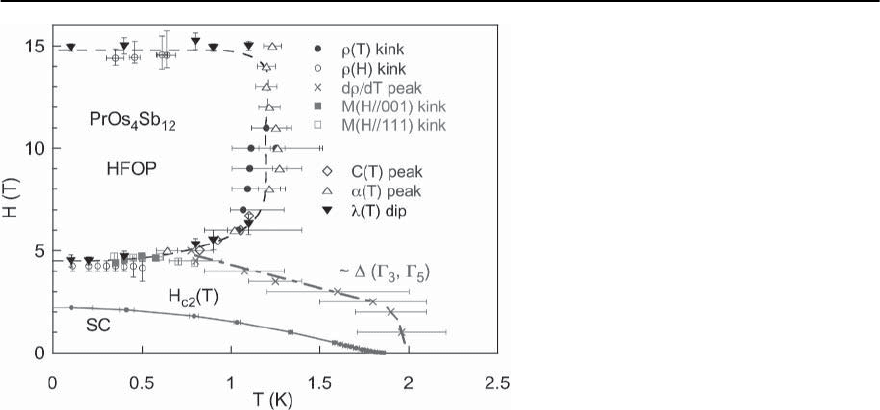
Fig. 13.57. Magnetic field-temperature (H − T)

phase diagram of PrOs

showing the re-

gions exhibiting superconductivity (SC) and

the high field ordered phase (HFOP) The

dashed line is a measure of the splitting be-

tween the Pr



ground state and 

excited

state (see text for further details), data from

[158,359,362–364,375]

specific heat, these measurements indicate strong

coupling superconductivity. These findings suggest

an s-wave, or, perhaps, a Balian–Werthamer p-wave

order parameter. The Balian–Werthamer p-wave or-

der parameter,originally suggested for the“B”phase

He, involves Cooper pairs with aligned spins in

the L = 1 angular moment state, and results in an

isotropic energy gap [370]. It is also interesting to

note that zero-field ‹SR measurements reveal the

presence of internal magnetic fields below T

=1.85

K indicating time-reversal symmetry breaking in the

superconducting state [371] of PrOs

There is also evidence for a high field ordered

phase (HFOP) in PrOs

.TheH − T phase dia-

gram, depicting the superconducting region and the

HFOP, is shown in Fig. 13.57 [364, 372]. The HFOP

was first detected by means of features in (H, T)

data [359,363,364].The line that intersects the high

field ordered state represents the inﬂection point

of the “roll-off” in (T) at low temperatures and

is a measure of splitting between the Pr

ground

state and the first excited state, which decreases with

field. The HFOP has also been observed by means

of large peaks in the specific heat [158,355], thermal

expansion [362,373],andkinks in the magnetostric-

tion [373] and M(H) curves [359,364,374,375].

The heavy-fermion superconductor PrOs

an intriguing material because of the possibility

that the heavy-fermion behavior and the supercon-

ductivity [376,377] arise from quadrupolar ﬂuctua-

tions. It is also only the second superconductor (af-

ter UPt

) that exhibits two distinct superconducting

phases with different order parameter symmetries.

The heavy fermion state and unconventional super-

conductivity observed in this material represent a

challenge for theoretical description [376–380].

PuMGa

Superconductivity has been observed in the Pu-

based material PuCoGa

, which crystallizes in the

same tetragonal structure as the CeMIn

materials,

with a critical temperature T

=18.5 K [133], an

order of magnitude larger than that of other heavy-

fermion superconductors. Shortly after the discov-

ery of superconductivity in PuCoGa

,theisostruc-

tural compound PuRhGa

was also found to be su-

perconducting at T

=8.7 K [134] with nearly iden-

tical normal and superconducting state properties

as PuCoGa

described below. The specific heat of

PuCoGa

, shown in Fig. 13.58, reveals a large spe-

cific heat jump at T

, C/T

= 110 mJ/mol K

,con-

firming bulk superconductivity in this material. If

one assumes the BCS value C/ T

=1.43, the elec-

tronic specific heat coefficient is  =77mJ/molK

indicating a moderate enhancement of the effective

mass. Further evidence for bulk superconductivity is

provided by electrical resistivity and magnetic sus-

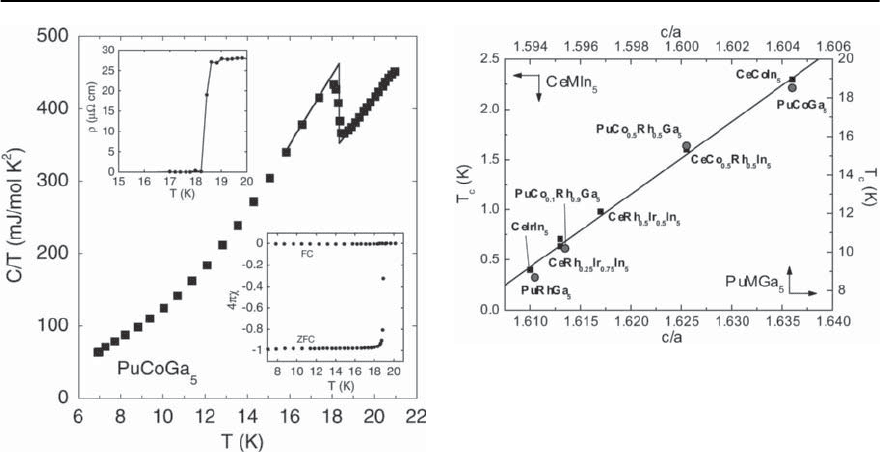
694 M.B. Maple et al.

Fig. 13.58. Specific heat over temperature C/T, electrical

resistivity  and magnetic susceptibility  as a function

of temperature T for PuCoGa

showing a superconducting

transition at 18 K, after [133]

ceptibility measurements (Fig. 13.58).In addition to

the unusually large value of T

for an intermetal-

lic compound, the upper critical field is correspond-

ingly large. An initial slope dH

/dT =−99kOe/K

yieldsanorbitalcriticalfieldH

∗

(0) = 1270 kOe

(Eq.(13.22)). Fromthis value of H

∗

(0),the supercon-

ducting coherence length is estimated to be 

=16

Å,from which an effective mass m

∗

=47m

is deter-

mined.This estimate of m

∗

yields = 161 mJ/mol K

providing further evidence for heavy-fermion behav-

ior in PuCoGa

. The critical current density is also

quite large, J

> 10

A/cm

for T > 0.9T

,dueto

pinning defects caused by the radioactive decay of

Pu.

In the normal state, PuCoGa

exhibits behav-

ior characteristic of both localized and itinerant 5f

electrons, a situation well-known in the various al-

lotropes of Pu and in the early actinides (Th-Am)

[381,382]. The magnetic susceptibility of PuCoGa

follows a modified Curie-Weiss law all the way down

to the superconducting transition temperature with

an effective moment 

eff

=0.68 

,close to the value

expected for localized Pu

(

eff

=0.84 

). On the

Fig. 13.59. Linear variation of the superconducting tran-

sition temperature T

with ratio of the tetragonal lattice

parameters c/a of CeMIn

(M=Co, Rh, Ir) and PuMGa

(M=Co, Rh) heavy-fermion superconductors, after [385]

other hand, the Sommerfeld coefficient  ∼ 100

mJ/molK

suggests some degree of delocalization.

This dual nature of the 5f electrons in PuCoGa

is consistent with photoemission experiments [383]

that reveal peaks at 1.5 eV below E

and at E

char-

acteristic of localized and itinerant behavior, respec-

tively. The electrical resistivity of PuCoGa

has s-

shaped curvature typical of materials with strong

spin ﬂuctuations. A power-law T-dependence is ob-

served below 75 K ((T) ∼ T

4/3

) [134] similar to

the NFL behavior found in the related compound

CeCoIn

(Sect. 13.3.5) [384].

Recent measurements confirm d-wave supercon-

ductivity in the PuMGa

(M=Co, Rh) materials.

The striking similarity of the physical properties of

PuMGa

and CeMIn

(M=Co, Rh, Ir), where heavy-

fermion d-wave superconductivity has been well es-

tablished, indicates magnetically mediated super-

conductivity in PuMGa

. One example of the close

resemblance between the two families of supercon-

ductors is shown in Fig. 13.59, where a linear rela-

tion between T

and the ratio of tetragonal lattice

parameters is found [385]. The power-law behavior

of the specific heat of PuCoGa

[385] and CeCoIn

below T

is also similar and is consistent with line

nodes in the superconducting gap function. Perhaps

the best evidence for unconventional superconduc-

tivity is provided by NQR/NMRmeasurements[386].

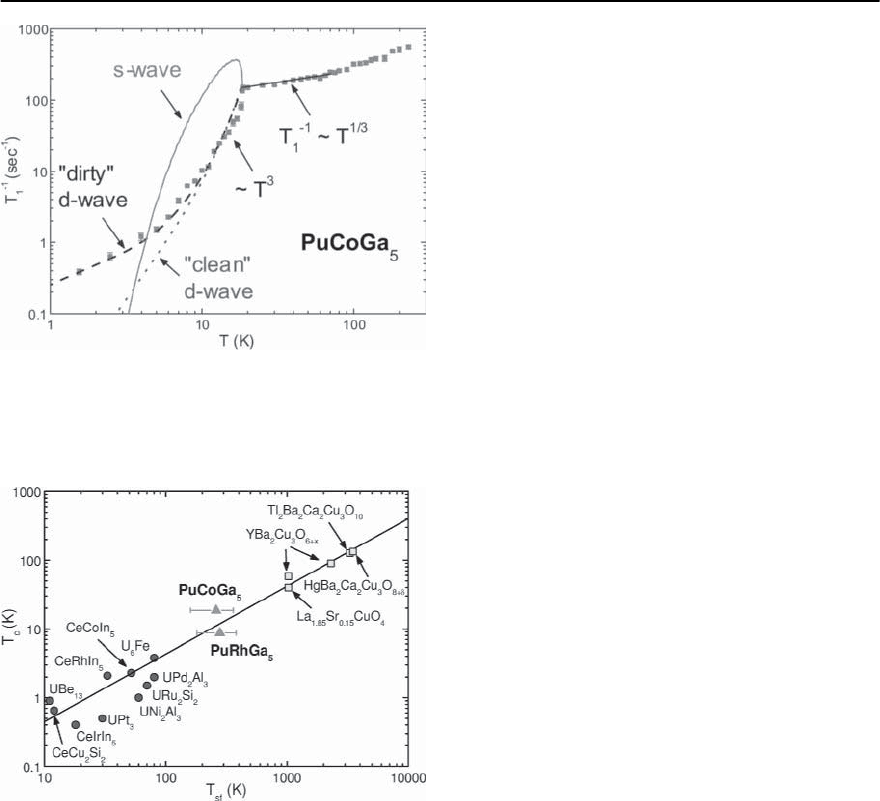
13 Unconventional Superconductivity in Novel Materials 695

Fig. 13.60. Spin-lattice relaxation rate 1/T

of the Ga nu-

clei in PuCoGa

.Thelines are calculations for BCS s-wave

(solid), pure d-wave (dotted)and“dirty” (dashed) d-wave

gap functions, after [386]

Fig. 13.61. Characteristic (spin ﬂuctuation) energy scale

vs superconducting transition temperature T

for the

heavy-fermion (circles), plutonium-based (triangles), and

high-T

(squares) superconductors, after [386]

As displayed in Fig. 13.60, the spin-lattice relaxation

rate 1/T

of the Ga nuclei in PuCoGa

exhibits a T

temperature dependence just below T

followed by a

T-linear dependence at the lowest temperatures due

to the impurities created by the radioactive decay of

Pu in the sample. The power-law behavior of 1/T

clearly in contrast to the expected behavior of a con-

ventional s-wave superconductor; the best fit to the

data is a “dirty d-wave” scenario in the strong cou-

pling regime (/k

∼ 4) [386]. Very similar be-

havior has recently been reported in PuRhGa

[387].

Scaling of the 1/T

data for a number of different d-

wave superconductors including CeCoIn

,PuCoGa

and YBa

7−ı

, with a characteristic spin ﬂuc-

tuation energy scale T

(found to be proportional

to the superconducting transition temperature) in-

dicates that these Pu-based superconductors bridge

the gap between the heavy-fermion superconductors

with T

s generally less than 1 K and the high-T

cuprates with T

s of order 100 K as shown in Fig.

13.61. This linear relation between T

and the spin

ﬂuctuation energy scale suggest that other classes of

materials within this magnetically mediated mech-

anism of superconductivity may yet be found with

even higher transition temperatures.

13.3.9 Concluding Remarks

The past two decades of research into heavy-fermion

superconductors have consistently yielded a rich va-

riety of new compounds, new phenomena, and new

insights into the nature of superconductivity.When

heavy-fermion superconductivity was first discov-

ered in CeCu

in 1979 by Steglich and cowork-

ers, the coexistence of magnetism and supercon-

ductivity had only been established in ternary rare

earth compounds such as the rare earth molybde-

num chalcogenides RMo

(X = S, Se) and the rare

earth rhodium borides RRh

. Since then about

20 different Ce, Pr, U, and Pu-based heavy-fermion

superconductors have been discovered and the list

will continue to grow. The past few years have seen

renewed interest in heavy-fermion superconductors

with the discovery of the coexistence of ferromag-

netism and superconductivity in UGe

,URhSi,and,

possibly, ZrZn

. The exciting discovery of supercon-

ductivity in PrOs

,which contains two supercon-

ducting phases which appear to have different order

parameter symmetries, and may have a quadrupo-

lar origin, suggests a new route to unconventional

superconductivity. Heavy-fermion superconductors

continue to be a fertile area of research and enhance

our understanding of superconductivity.

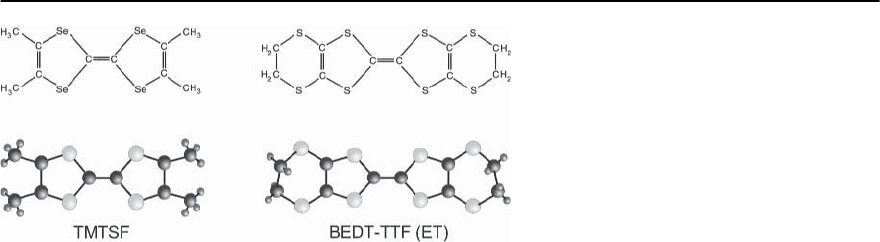
696 M.B. Maple et al.

Fig. 13.62. The molecular structures of TMTSF

(tetramethyltetraselenafulvalene) and BEDT-

TTF (bisethylenedithio-tetrathiafulvalene, or

ET for short), which are the main building

blocks of crystalline molecular conductors. In

contrast to TMTSF, the ET molecule is not com-

pletely planar

13.4 Organic Superconductors

13.4.1 Introduction

The first organic superconductor, (TMTSF)

, was

discovered more than 25 years ago by J´erome et

al. [388]. It belongs to a class of compounds known

as Bechgaard salts, named after the first person to

synthesize them,Klaus Bechgaard.(TMTSF)

un-

dergoes a transition from a quasi-one-dimensional

(1D) metal to a superconductor as the temperature

is lowered below 1 K under an applied pressure of

∼12 kbar. At ambient pressure, however, it experi-

ences a Peierls metal-insulator transition to a spin-

density-wave (SDW) state at low temperatures. With

one exception, SDW phase transitions are observed

in all Bechgaard salts based on TMTSF. The molecule

TMTSF (tetramethyltetraselenafulvalene) is shown

in Fig. 13.62.

Within a few years of this stimulating discov-

ery, a large variety of other superconductors based

on different molecular building blocks were found.

Of the more than 100 different organic supercon-

ductors known to date [389], the largest number

are based on the molecule BEDT-TTF (bisethylene-

dithio-tetrathiafulvalene or ET for short), which is

also shown in Fig. 13.62. The usual stoichiometry

of these quasi-two-dimensional (2D) organic metals

is (ET)

X, where X represents a monovalent anion

such as I

−

or Cu(NCS)

−

[389,390]. Charge transfer

from the neutral ET molecule to the anion leads to

partially filled molecular bands, resulting in metal-

lic conductivity. To date, the highest values of T

are

11.5 K at ambient pressure for -(ET)

Cu[N(CN)

]Br

[391] and about 13 K for the isostructural material

-(ET)

Cu[N(CN)

]Cl under a moderate pressure of

about 0.3 kbar [392].

Beyond the main building blocks, TMTSF and

ET, of crystalline organic superconductors, a num-

ber of additional organic molecules are known

to form superconducting species [389]. A very

promising new class of 2D organic metals is

based on the donor BETS (bisethylenedithio-tetra-

selenafulvalene), a selenium-substituted ET ana-

logue. Molecular crystals of this donor with Fe

containing anions are prone to magnetic interactions

between the BETS -electron system and the iron

d electrons [393,394]. This makes these materials

a fascinating playground for studying the compet-

ing magnetic and superconducting interactions.For

example, reentrant superconductivity upon cooling

(Sect. 13.2.3) and magnetic-field-induced supercon-

ductivity (Sect.13.2.4) can be foundin these systems.

Besides superconductivity, the organic metals

show a wealth of different ground states such as

antiferromagnetism, spin-Peierls, SDW, and charge-

density-wave (CDW) states. These ground states are

accessible by tuning the structure, counter anion,

magnetic field, temperature, and pressure. The study

of these fertile phase diagrams has led to new the-

oretical concepts; however, a solid understanding

of some of these states still remains a challenge.

Even the normal metallic state of these electronically

low-dimensional metals reveals unusual properties

sometimes not in line with conventional Fermi-

liquid theory.

Many superconducting properties of the organic

superconductors, especially of the 2D materials, re-

semble the behavior found in the cuprate supercon-

ductors[395–398].The H–T phase diagrams and the

13 Unconventional Superconductivity in Novel Materials 697

vortex dynamics are very similar in these layered

strongly type-II superconductors. In contrast to the

high-T

cuprates,however,much less is known about

the nature of the superconducting state in organic

metals. Clear-cut experimental proof for unconven-

tional non-BCS-like superconductivity is missing.

Until very recently the 1D Bechgaard salts were be-

lieved to be triplet superconductors. This, however,

is not the case as recent sophisticated experiments

proved singlet pairing [711]. For the 2D organic su-

perconductors,on the other hand,where singlet pair-

ing has been established, an ongoing debate persists

as to the exact symmetry of the order parameter

(see [399–402] for recent reviews).Some experimen-

tal results hint at line nodes in the superconducting

energy gap, i.e., d-wave symmetry, whereas other ex-

periments, in particular, specific-heat data, suggest

the existence of a nodeless energy gap, fully consis-

tent with BCS-like s-wave symmetry.

A further unsolved issue is the mechanism re-

sponsible for the Cooper-pair coupling. While there

is some belief in the existence of a non-phonon-

mediated coupling mechanism, as appears to be

in unconventional superconductors such as the

cuprates, Sr

RuO

, and heavy-fermion compounds,

this issue remains unclear and heavily debated for

the organic superconductors. The proximity of an-

tiferromagnetic or SDW states to the superconduct-

ing state spurred the notion that antiferromagnetic

ﬂuctuations are responsible for the coupling. On the

other hand, a number of experiments indicate that

some interaction between phonons and supercon-

ductivity exists. In any case, it is clear that the exci-

tonic mechanism originally proposed by Little [403]

is not responsible for the pairing of superconduct-

ing electrons.Nevertheless,Little’s prediction of very

high transition temperatures in organic materials

was an important stimulus for the search for organic

superconductors.

13.4.2 Normal-State Properties

Ordinarily,theterm“organic materials”implies plas-

tics, i.e., polymeric carbon-based chains. However,

the 1D and 2D organic metals discussed here are

high-quality single crystals usually grown by electro-

crystallizationfromappropriate solutions,thedetails

of which are reviewed in depth in [390]. During this

process the oxidation of the donor molecules occurs

together with the crystal growth at the electrodes.

The resulting charge-transfer salts weigh up to a few

milligrams and are usually shaped like needles or

plates.

Quasi-One-Dimensional Organic Metals

All 1D organic superconductors based on the donor

molecule TMTSF crystallize in the same triclinic

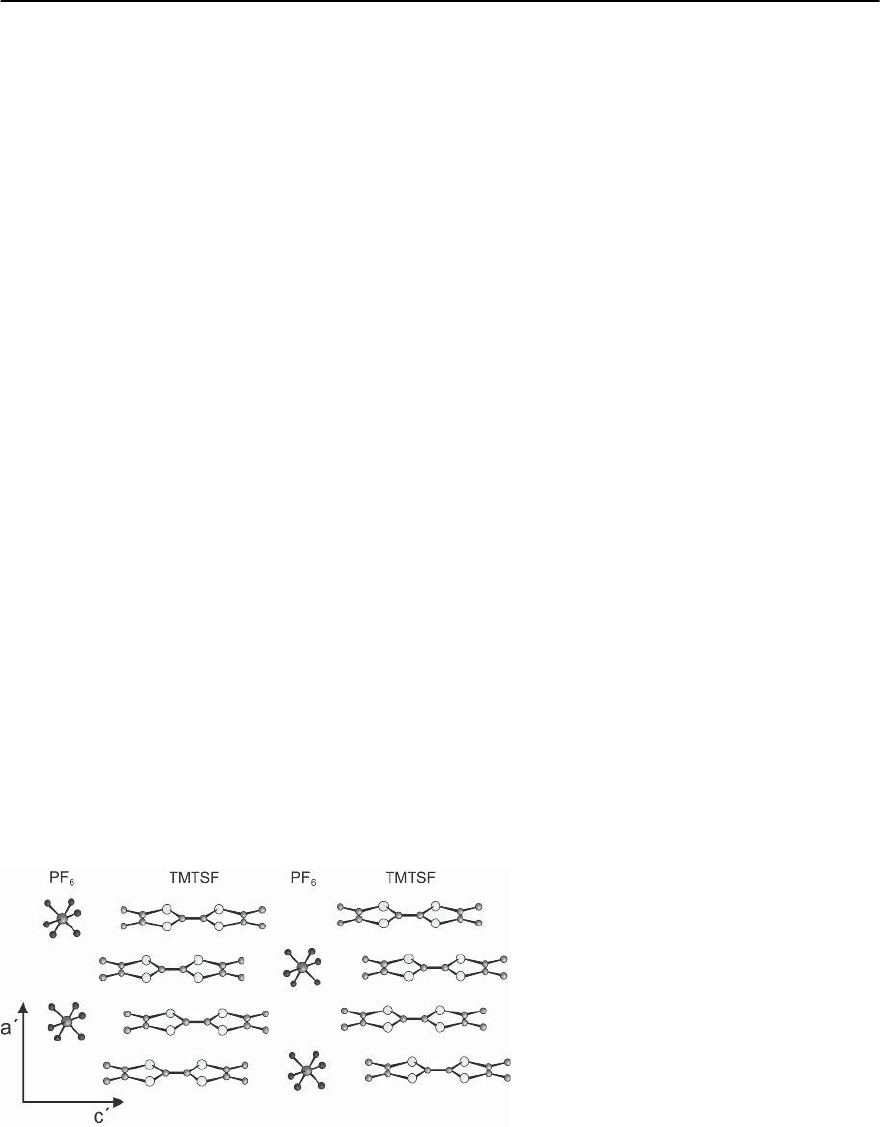
crystal structure which is depicted in Fig. 13.63

for (TMTSF)

[404]. Some crystal parameters

are given in Table 13.6. TMTSF is a planar brick-

like molecule that tends to stack in columns. These

columns are formed along the a-axis, which is the

axis with the highest conductivity since in this di-

rection the Se–Se distances are short, resulting in a

strong -orbital overlap. Along the b-axis the Se–Se

Fig. 13.63. Crystal structure of (TMTSF)

viewed somewhat tilted relative to the b di-

rection. a



and c



are projections of a,the

most-conducting direction, and c,theleast-

conducting direction

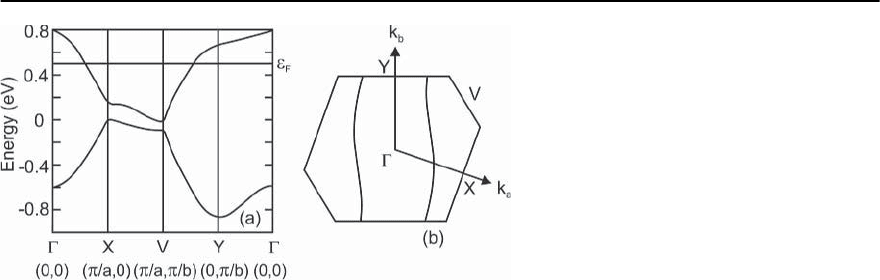
698 M.B. Maple et al.

Fig. 13.64. (a) Calculated dispersion relation

and (b) Fermi surface of (TMTSF)

.These

results are representativeof all (TMTSF)

X salts

[406]

distances are similar; however, the overlap integrals

are much smaller due to the side-by-side orientation

of the Se atoms.Along the c-axis, the PF

−

anions (see

Fig. 13.63) separate the columns.This results in only

a weak electronic coupling along c with correspond-

ingly small overlap integrals. The approximate ratio

of the overlap integrals along the crystallographic

directions is t

: t

= 300 : 30 : 1. These numbers

reveal that the Bechgaard salts are by no means ideal

one-dimensional metals. It is, however, exactly this

tendency towards two dimensionality which shifts

the insulating SDW state to low temperatures and

allows superconductivity under moderate pressure

(see below).

Thecalculationoftheelectronicbandstructure

of the organic charge-transfer salts from first prin-

ciples is a highly sophisticated task.Although steady

progress has been made in dealing with the large

unit cells in high-level first-principle studies [405],

the usual method for obtaining the band structure is

based on the extended H¨uckel tight-binding approx-

imation. These calculations result in surprisingly re-

liable band structures for most 1D and 2D organic

metals, at least for the dispersion relations in the

neighborhood of the Fermi energy. The calculated

band structure and the 2D Fermi surface (FS) for

(TMTSF)

is shown inFig.13.64.Fordifferent an-

ions, the dispersion relations and the FS are almost

indistinguishable [406].

For the Bechgaard salts, there is a strong mutual

interaction between the 1D electronic system and

the three-dimensional lattice. In general, metals with

a pronounced 1D character have a tendency to de-

velopaninsulatinggroundstate ina combinedlattice

and electronic phase transition. They are unstable

against a Peierls transition, which is a perturbation

with a wave (or nesting) vector of 2k

,wherek

the Fermi wave vector. This perturbation can either

be a charge redistribution, i.e., a charge density wave

(CDW), or a spin redistribution (SDW). This leads

to a reconstruction of the crystallographic or mag-

netic unit cell resulting in a modified band structure

and Fermi surface. Whether a Peierls transition oc-

curs and at which temperature crucially depends on

the dimensionality of the electronic system. For an

ideal one-dimensional metal, a Peierls transition is

inevitable.

Most of the 1D Bechgaard salts show a Peierls

transition at ambient pressure. However, this metal-

insulator transition occurs at rather low temper-

atures, T

∼ 10 K (Fig. 13.65) since, as stated

above, an appreciable overlap along the b direc-

tion leads to an increased dimensionality and re-

duces the electronic energy gained at the Peierls

phase transition. The transition is clearly seen in

the electrical-resistivity and magnetic-susceptibility

data (Fig. 13.65) [407, 408]. These data prove the

SDW nature of the distortion [409]; in contrast to

an isotropic decrease of the magnetic susceptibil-

ity expected for a CDW, the susceptibility vanishes

exponentially only along the b



direction (inset of

Fig. 13.65(b)) 13.65(b)) [408]. Such behavior is char-

acteristic of an antiferromagnetically ordered state

with alternating spin orientation along the b



direc-

tion. This type of spin ordering has been confirmed

by a number of additional experimental techniques,

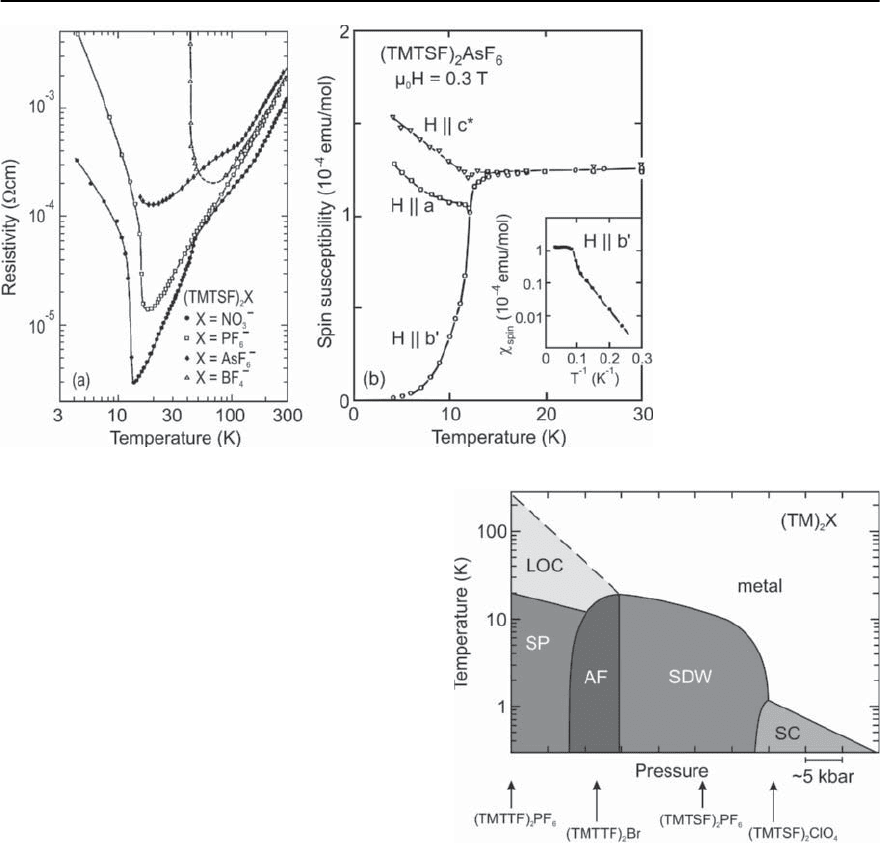
13 Unconventional Superconductivity in Novel Materials 699

Fig. 13.65. (a) Temperature

dependence of the resistiv-

ity for various (TMTSF)

salts [407].BelowthePeierls

transition, the resistivity

shows insulating behav-

ior. (b) Temperature depen-

dence of the static spin

susceptibilityof (TMTSF)

AsF

.Theinset shows the

exponential decrease of the

susceptibility in the b



di-

rection (perpendicular to a

and c

∗

) below the metal-

insulator transition [408]

and by the fact that the measured SDW nesting vec-

tor is compatible with the calculated band structure

shown in Fig. 13.64 [389,410].

As mentioned previously, moderate pressure of a

few kbar suppresses the SDW state and supercon-

ductivity evolves. The generic pressure-temperature

(P − T) phase diagram for the (TM)

Xsaltsisshown

in Fig. 13.66. TM stands for TMTSF or TMTTF (=

tetramethyltetrathiofulvalene), the sulfur analogue

of TMTSF. For the organic metals and superconduc-

tors, the crucial parameter is pressure, not charge-

carrier concentration, as is the case for the cuprate

superconductors (see Sect. 13.5). An increasing pres-

sure is equivalent to a better overlap of the molec-

ular wave functions which results in a reduced

anisotropy and,consequently,in an increased dimen-

sionality.The only Bechgaard salt which becomes su-

perconducting at ambient pressure (T

=1.2K) is

(TMTSF)

ClO

[411]. In order for this to occur, the

material has to be cooled slowly to allow the ran-

domly oriented low-symmetry ClO

anions to order

at about 24 K, where a superlattice is formed with a

doubledlattice parameteralongthe b direction [389].

If, on the other hand, the sample is quenched rapidly

with a cooling rate greater than 50 K/min, the high-

temperature anion disorder persists down to low

temperature and superconductivity does not appear.

Fig. 13.66. Schematic pressure-temperature phase diagram

of the (TM)

X salts based on TMTSF and TMTTF. The

arrows indicate the ambient pressure of various molecu-

lar crystals. LOC = charge-localized insulator, SP = spin-

Peierls, SDW = spin density wave, AF = antiferromagnetic

insulator, and SC = superconductor

TMTTF-based salts are electronically more one

dimensional than the TMTSF analogues. For

(TMTTF)

, which is located at the far left side of

the phase diagram in Fig. 13.66 at ambient pressure,

an insulating state (LOC) occurs at high tempera-

700 M.B. Maple et al.

tures of about 230 K. The localization of the interact-

ing electrons is believed to be due to a Mott–Hubbard

transition.At lower temperatures, a spin-Peierls (SP)

transition takes place resulting in a dimerization of

the antiferromagnetically ordered spin chains. In re-

cent sophisticated high-pressure studies [412–414],

two groups were able to tune (TMTTF)

through

all of the phases shown in Fig. 13.66. Indeed, at

pressures of about 50 kbar superconductivity was in-

duced. These data validate the P − T phase diagram

that was originally proposed by J´erome on the basis

of experimental data obtained for different (TM)

salts [415].

More information on the directional dependence

of the strongly anisotropic molecular interactions

can be gained by uniaxial-pressure studies. How-

ever, these are hampered by the high fragility

of the organic superconductors. One of the few

uniaxial-pressure investigations was reported for

(TMTSF)

[416]. Surprisingly, the strongest sup-

pression of the SDW transition temperature was

found for pressure applied along the most-con-

ducting a direction. The larger overlap along a re-

sults in an increased bandwidth and, concomitantly,

in a decreased density of states and, therefore, a re-

duced energy gain in the electronic system at the

SDW transition. Apparently, this effect is more im-

portant than the simultaneous increase in Fermi-

surface nesting [417]. A more detailed overview on

uniaxial-pressure studies is given in [418].

Magnetic fields can have a strong inﬂuence on the

electronic properties of the organic superconductors.

One fascinating phenomenon is the occurrence of

field-induced spin density waves (FISDW) which be-

come evident in Shubnikov–de Haas (SdH)-like os-

cillations in the longitudinal resistivity and in quan-

tized steps of the Hall resistivity.Indeed,this was the

first observation of a quantum Hall effect in a bulk

crystal [419,420]. Stimulated by these intriguing re-

sults, a theory now commonly known as the “stan-

dard model” for FISDW was developed [421]. The

principal effect of the applied magnetic field is an

effective reduction of the electronic dimensionality.

Therefore, the magnetic field can counterbalance the

pressure-increased dimensionality leading to a tran-

sition intoa SDW stateat highenoughfieldstrengths.

For a more thorough discussion ofthis phenomenon,

see [389].

In the 1D organic metals, a number of electrical-

transport properties exist that cannot be explained

by the conventional Fermi-liquid theory. In fact, for

1D metalsa non-Fermi-liquidstate,i.e.,aspin-charge

separatedLuttinger liquid,is predicted.Theobserved

field and temperature dependences of the resistivity

[422,423]as well as recent thermal-conductivity data

[424] have been interpreted along these lines.At zero

field,(TMTSF)

is believed to be a marginal three-

dimensional Fermi liquid which can be destabilized

by theapplication of small fields in certain directions

[425, 426]. Above a threshold field (∼ 0.2T along

the b direction), electronic transport shows incoher-

ent non-Fermi-liquid properties. Consequently, the

“normal state” of the Bechgaard salts behaves non-

metallically in the presence of small magnetic fields

(see [427, 428] for recent reviews). This picture got

support from thermal-transport experiments that

for special field orientations showed Nernst signals

several orders of magnitude larger than estimated

from usual Fermi-liquid models [429].

Quasi-Two-Dimensional Organic Metals

In contrast to the isostructural 1D Bechgaard salts,

a number of different crystal structures exist for the

ET-based charge-transfer salts. They mainly differ in

the packing motifs of the nonplanar ET molecules

and are labeled by different Greek letters (see Table

13.6). [389,390].

Figure 13.67 shows the schematic crystal struc-

ture of ˇ-(ET)

, a typical example of most (ET)

salts. The common structural feature is the pack-

ing of the ET molecules into layers which are sep-

arated by poorly conducting anion planes (I

−

planes

in Fig. 13.67). The principal difference between the

crystal structures of the ET families (˛, ˇ, ˇ



, ,etc.)

is thearrangement of theET donors within thelayers.

There are, however, always sufficiently close contacts

between the  orbitals of the sulfur atoms of neigh-

boring intralayer ET molecules. This results in the

formation of molecular bands with only relatively

small in-plane anisotropies (typically within a fac-

tor of two). The anions which are responsible for