Bennemann K.H., Ketterson J.B. Superconductivity: Volume 1: Conventional and Unconventional Superconductors; Volume 2: Novel Superconductors
Подождите немного. Документ загружается.


5 Theory of Superconducting Alloys 217
information regarding quasi-particles and the den-
sity of states is contained in G(p; !
n
)or
¯
G(!
n
)ifan-
alytically continued from the upper Matsubara axis
i!
n
into the whole complex plane i!
n
→ z [3]. We
will not elaborate on the thermodynamics of a super-
conducting paramagnetic alloy but focus only on a
new fundamental feature of its energy spectrum: the
appearance of the so-called“gapless”regime.For this
purpose let us consider the spectral representation of
the Green function G(p, z) (a similar representation
exists for the anomalous functions, F and F
†
)
G(p, z )=
∞
−∞
(p, x)dx
x − i!
n
. (5.65)
Being analytically continued from the upper (lower)
complex plane z = i!
n
, the resulting function
coincides with the retarded (advanced) functions
G
R(A)
(p; !) on the real axis z = ! ± iı.AtT =0
the imaginary part of the latter is of the form
ImG
R(A)
(p; !)=±
l
l
(p)ı(! − !
l0
) ,
with !
l0
= E
l
−E
0
being the excitation energies of the
system from its ground state,E
0
,into an excited state,
E
l
(for the details of the derivation see [3]). The fre-
quency !
∗
at which an imaginary part first ocurs in
G(p; !
n
)or
¯
G(!
n
) after being analytically continued
to the physical frequency axis (z = i!
n
→ ! ±iı),is
the threshold, or the gap in the excitation spectrum.
With this in mind, we return to Eqs. (5.62)–(5.64)
and formally replace !
n
by −i!,andu
n
by −iu (with
˜
n
→
˜
(!)). Equation (5.64) is then written
!
= u
1−
1
s
1
√
1−u
2
.
For low impurity concentrations, i.e. beginning with
small (1/
s
), it is clear, that in order to have real
values for the square root on the imaginary axis,
u → iu
n
as it is given by the equations, one must
choose thebranch of the real positiveradical
√
1−u
2
for −1 < u < 1; this analytical continuation pro-
cedure fully defines the function u(z)inthewhole
z-plane [3].
Let us now return to the retarded (advanced)
Green functions on the physical axis. With ! > 0
on the real axis one sees that a maximum occurs at
u
0
=
1−(1/
s
)
2/3
1/2
. (5.66)
This defines the position of the branching point for
u(!) and, hence, the appearance of an imaginary
component in G
R(A)
(p; !)orF
†R(A)
(p; !). The en-
ergy gap !
∗
according to Eq. (5.66), is:
!
∗
=
1−(1/
s
)
2/3
3/2
. (5.67)
This is equal to zero for
s
=1.Westillhavetode-
termine the corresponding concentration value for
which this happens.
We again use the self-consistency condition
Eq.(5.15)andintegratefirstoverd in the expression
for the averaged F
†
(p; !
n
) .However, the new defini-
tions for ˜!
n
and
˜
n
are taken into account.At T =0
the sum over frequencies, T
n
, becomes the inte-
gral (1/2)
d! along the Matsubara axis, z = i!.
Thus,
0
= |g|
mp
F
4
3
˜!
0
d!
u
2
(!)+1
. (5.68)
To handle the cutoff, ˜!, we add and subtract un-
der the integral on the right hand side the proper
compensating term
0
(!
2
+
2
0
)
−1/2
and make use
of the corresponding definition for the gap,
00
,of
the pure superconductor (we also distinguish
0
,the
gap of an impure superconductor at T =0,from
00
=
/
T
c0
, the gap value for the parent mate-
rial). After some simple transformations one arrives
at the equation
0
ln
0
00
=
∞
0
d!
1
1
√
u
2
+1
−
0
!
2
+
2
0
2
. (5.69)
To c alculate
0
as a function of 1/
s
,itisconvenient
to convert the integration over ! into an integration
over u, making use of Eq. (5.64). We do not discuss
the details of the calculations. (A helpful comment,
however, is that depending on 1/
s
0
the lower limit,
! = 0, in the integral Eq. (5.69) changes from 0
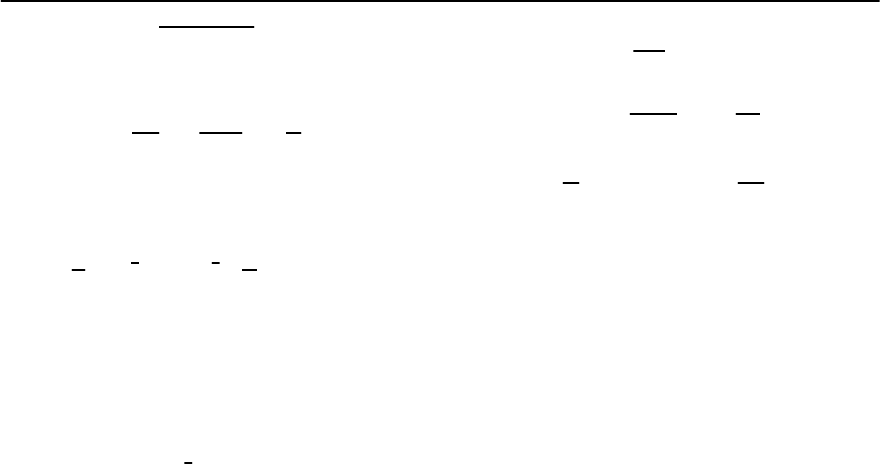
218 L.P. Gor’kov
at 1/
s
< 1to
(
s
0
)
−2
−1at 1/
s
0
> 1). For
1/
s
0
= 1, where the energy gap disappears,we find
ln
0
00
=−
4
s
0
≡ −
4
, (5.70)
or in terms of the concentration (i.e., 1/
s
)
1
s
=e
−
4
00
=e
−
4
T
c0
. (5.71)
Since the critical concentration n
cr
at which su-
perconductivity is destroyed is given by (1/
s
)
cr
=
(/2 )T
c0
, one sees from Eq. (5.71) that the energy
gap disappears at the smaller concentration
n
=2e
−
4
n
cr
0.91n
cr
. (5.72)
Therefore, in superconductors with paramagnetic
impurities there exists a gapless regime in which the
specific heat C
p
(T)atlowT has a linear slope (A.A.
Abrikosov and L.P. Gor’kov, 1960 [7])
C
s
(T)=
1−(
s
0
)
2
1/2
T . (5.73)
The non-zero superconducting order parameter in
the absence of an energy gap in the excitation spec-
tra for the s-wave superconductivity is due to broken
time reversal symmetry in the electronic sub-system
(intheHamiltonian)Eq.(5.26).A gaplessregimemay
also appear in the presence of a magnetic field which
also breaks t ⇒ −t invariance (K. Maki, 1964 [12];
P.G. de Gennes and M. Tinkham, 1964) [22]. For a d-
type or other non-trivial order parameter a gapless
spectrum is intrinsicdue to the presence of nodes in
the gap. In addition, the nodes can be easily smeared
by ordinary defects.
To complete the discussion of the superconduct-
ing gapless regime Eq. (5.72) caused by the presence
of paramagnetic defects, we give without derivation
a generalized GL equation for (r) and the current
j(r). Near the critical concentration, n
cr
,(T
c
T
c0
)
one has
2
(T
2
− T
2
c
)+
||
2
2
∗
(5.74)
+
2v
2
F
tr
s
∇ +
2ie
c
A
2
∗
=0,
j = N
s
tr
ie
m
(∇
∗
−
∗
∇)−
4e
2
mc
A||
2
.
(5.75)
(A.A. Abrikosov and L. P. Gor’kov, 1960 [7]).
5.5 Eilenberger Equations
5.5.1 Quasi-Classical Approximation
In the preceding Sections we obtained some basic
results for the theory of superconducting alloys. So
far, for each of these problems an application of the
cross-technique provided a natural and straightfor-
ward approach which led directly to a final answer.
However, when considering more complicated prob-
lems, such as nonlinear behavior of a superconduc-
tor in a strong magnetic field and at temperatures
far from the critical temperature T
c
,asolutionof
the Gor’kov equations, even in the absence of im-
purities, becomes a difficult task that requires nu-
merical methods.When considering alloys the cross-
technique becomes also rapidly more tedious. Thus,
while for a homogeneous superconductor one needs
to average only the Green functions themselves,spa-
tial variations of the order parameter (r)and
†
(r)
introduce other correlators of the Green functions,
for example,suchas an averageof thesumof the“lad-
der” diagrams associated with
†
(p, k; !
n
)which
we considered for the linearized gap equation in the
presence of an external magnetic field.
Fortunately, at least for ordinary(i.e.the BCS-like)
superconductors,further simplifications turn out to
be possible for the Gor’kov equations. Using the
method of quasi-classical Green functions one can
derive the less-complicated Eilenberger equations.
The new formalism significantly simplifies the cal-
culations for most problems,especially for supercon-
ducting alloys (G. Eilenberger, 1968 [23]; A.I. Larkin
and Yu.N. Ovchinnikov, 1968 [24]; G.M. Eliashberg,
1971 [25]). The applicability of the quasi-classical

5 Theory of Superconducting Alloys 219
approach, as follows from its very name, is based
on the fact that in metals the typical wavelength of
an electron, p
−1
F
,isontheatomicscaleand,hence,is
much shorter then any other length scale under con-
sideration: the mean free path, l = v
F
,themagnetic
field penetration depth, ı, or the coherence length
(the“size”of the Cooper pair),
0
= v
F
/2T
c
(P.G. de
Gennes,1964 [22]; E.A.Shapoval, 1964, 1965 [26]; for
the review see J.W. Serene and D. Rainer, 1983 [27]).
The possibility of neglecting all crossing diagrams,
such as shown in Fig. 5.2(c), is also due to the in-
equality, p
F
l 1. This very important observation
allows one to write down the Gor’kov equations for
alloys, and as a consequence the Eilenberger equa-
tions, in closed form.
5.5.2 Derivation of the Eilenberger Equations
To begin with let us return again to equations (5.14)
and rewrite them in a more compact form for a
general case where, in addition to the impurity po-
tential, a superconductor experiences an inhomoge-
neousmagnetic field B(r)=[∇×A(r)] (whereA(r)is
the vector potential). Indeed, the Gor’kov equations
can be presented in matrix form [3]):
ˆ
G
−1
0
−
ˆ
V
·
ˆ
G(x, x
)=
ˆ
ı(x − x
), (5.76)
where
ˆ
G(x, x
)=
G(x, x
)−F(x , x
)
F
†
(x, x
) G(x
, x)
%
, (5.77)
and
ˆ
V is given by
ˆ
V ≡
ˆ
V(r) ≡
a
U(r − r
a
)
%
·ˆe , (5.78)
(
ˆ
V is a sum over all impurity potentials multiplied
by the two-by-two unit matrix ˆe.) The operator for
the inverse (“bare”) Green’s function is
ˆ
G
−1
0
=
−@/@ −(
ˆ
H
0
− ) (r)
−
†
(r) @/@ −(
ˆ
H
0
− )
%
. (5.79)
As in Sect. 5.2,
ˆ
H
0
and
ˆ
H
0
are single particle Hamilto-
nians including the vector potential associated with
an external magnetic field. For the isotropic model
one has
ˆ
H
0
≡
1
2m
−i∇ −
e
c
A(r)
2
,
ˆ
H
0
≡
1
2m
−i∇ +
e
c
A(r)
2
. (5.80)
It was suggested that (r)and
†
(r)areself-
averaging variables. Local distortions produced in
the vicinity of an impurity decay rapidly as does the
magnetic field. Therefore, both the order parameters
(r),
†
(r) and the vector potential A(r)areslowly
varying functions of the coordinates with character-
istic scales (l)orı(l),respectively.These depend on
impurity concentration through the mean-free path
l. These scales are much longer than the effective dis-
tances over which the averaging actually takes place.
In what follows we restrict ourselves to station-
ary problems only. Therefore, the dependence on the
“imaginary time” coordinates ,
again reduces to
the“time”difference( −
). Correspondingly, we
can rewrite Eq. (5.76) in terms of the Fourier fre-
quencies,
ˆ
G(r, r
; !
n
). This can be easily achieved by
the substitution: @/@ ⇒ −i!
n
in expression (5.79)
for the operator
ˆ
G
−1
0
.Wefurthertransformthema-
trix equation (5.76) to the momentum representa-
tion:
ˆ
G(r, r
; !
n
) ⇒
ˆ
G(p, p
; !
n
). Without going into
details, let us recall that in all Green functions the
characteristic scale for the values of two momenta
p and p
is given by the Fermi momentum p
F
.As
usual within the quasi-classical approximation we
canmakeinEq.(5.80)theapproximation
ˆ
H
0
p
2
F
/2m −
e
c
v
F
· A(r) ,
ˆ
H
0
p
2
F
/2m +
e
c
v
F
·A(r) .
The fact that both A(r)and(r),
†
(r) depend on r
needs to be taken into account for all equations writ-
ten in the momentum representation. This is, how-
ever,atrivial matter that does not affect the averaging
over impurities and will be discussed later.
After these preliminary remarks it becomes clear
that one can apply the same averaging procedure (see
the diagrammatic cross-technique of Sect. 5.2.1) to
the matrix equation (5.76). Indeed, let us formally

220 L.P. Gor’kov
expand Eq.(5.76) in
ˆ
V, the impurity potential, to ob-
tain the perturbation series. This is shown in Fig.5.1.
The transform matrix expression (5.78) is given by
1
(2)
3
a
U(p − p
) ·exp i(p − p
) ·r
a
%
·ˆe .
Analysis of the relative significance of the differ-
ent diagrams in Fig. 5.2 can be applied without any
changes. The new Dyson equation for the averaged
matrix function
ˆ
G(p, p
; !
n
)showninFig.5.9bythe
line (without arrows) looks like that in Fig.5.4,except
that both
ˆ
G
0
(p, p
; !
n
), the Green function for the
pure superconductor, and the average
ˆ
G(p, p
; !
n
),
depend on two momenta. This is due to the spatial
dependence of the gap parameters and of the field.
In Fig. 5.9 the three vectors, k, l and s which come
about due to such a dependence are small compared
to p and p
. The equations describing Fig. 5.9 are:
ˆ
G(p, p − k; !
n
)=
ˆ
G
0
(p, p − k; !
n
) (5.81)
+
ˆ
G
0
(p, p − l; !
n
)
ˆ
G(p
, p
− s; !
n
)
×
ˆ
G(p − l − s, p − k; !
n
)
×
U(p
− p + l)
2
d
3
l d
3
s d
3
p
(2)
3
.
Let us again present this equation in a symbolic form:
(
ˆ
G
−1
0
−
ˆ
£)
ˆ
G
= ˆe. (5.82)
The notations in Eq.(5.82) have the following simple
meaning. According to the previous equations,
ˆ
G
−1
0
in addition to its trivial part is given by
i!
n
− v
F
·(p − p
F
)0
0−i!
n
− v
F
· (p − p
F
)
%
.
A(m), (m)and
†
(m) contribute to Eq. (5.82) as
d
3
m
(2)
3
e
c
v
F
· A(m) (m)
−
†
(m)−
e
c
v
F
· A(m)
%
×
ˆ
G(p − m, p − k; !
n
).
Here we use the conventional definition of the
Fourier transform
y(r)=
y(m)e
im·r
d
3
m
(2)
3
. (5.83)
For the “self-energy”
ˆ
£ one has
ˆ
£(p, m; !
n
)=n
|U(p − p
)|
2
(5.84)
×
ˆ
G(p
, p
− m; !
n
)
d
3
p
(2)
3
.
The dependence on m corresponds to the spatial
variation (in the absence of an external magnetic
field, £’s may depend only on the direction of the
momentum p for an anisotropic scattering).It is con-
venient to change slightly the notations so that
ˆ
G(r
1
, r
2
; !
n
)=
ˆ
G(p, p − k; !
n
) (5.85)
× e
ip·(r
1
−r
2
)+ik·r
2
d
3
p
(2)
3
d
3
k
(2)
3
.
Note, the Fourier transform is symmetric with re-
spect to each of the two variables, r
1
and r
2
.Theuse
Fig. 5.9. Schematic representation of the
Dysonequationfortheaveragedmatrix
Green function

5 Theory of Superconducting Alloys 221
of
ˆ
G instead of
ˆ
G in Eq. (5.84) allows us to write the
spatial dependence of the£’s on an equal footing with
that of A(r),(r)and
†
(r).In practice the transition
from
ˆ
G to
ˆ
G involves nothing but an additional factor
(2)
3
in the old definitions of the Green functions.
For a homogeneous superconductor one has
ˆ
G ≡ (2)
3
ˆ
G(p; !
n
)ı(k) . (5.86)
From the previous calculations it is seen that the
dependence on = v
F
(p − p
F
) can be integrated out
in the self-energy parts . All integrals rapidly con-
verge at small . This mathematical trick comprises
the essence of the Eilenberger formulation in terms
of “quasi-classical Green functions”. Since the latter
is defined only in the vicinity of the Fermi surface.
There is no need for a solution of the more general
equations for the Gor’kov Green functions. Before
writing the equations,let us make a few other helpful
comments.
First we note that many physical properties are
defined by the Green functions at r
1
= r
2
= r.Thus,
the density of electrons n(r) is given by [3]
n(r)=2T
!
n
e
i!
n
ı
G(r, r; !
n
) . (5.87)
The current density j(r)is
j(r)=2T
!
n
e
i!
n
ı
ie
2m
(∇
r
− ∇
r
)
r
→r
(5.88)
× G(r, r; !
n
)−
e
2
m
A(r) G(r, r; !
n
)
.
Here the factor two arises from the two spin direc-
tions. For the model with a “contact” interaction one
would have
(r)=gT
!
n
F(r, r
; !
n
)(r → r
) . (5.89)
At r
1
= r
2
we can write
ˆ
G(r, r; !
n
)=
ˆ
G(p, r; !
n
)
d
3
p
(2)
3
. (5.90)
One may write down the self-energy Eq.(5.84) in the
coordinate representation to show that these can also
be expressed in terms of
ˆ
G(p, r; !
n
):
ˆ
£(p, r; !
n
)=n
|U(p − p
)|
2
(5.91)
×
ˆ
G(p
, r; !
n
)
d
3
p
(2)
3
.
We can write the integration over p
as
d
3
p
(2)
3
=
mp
F
2
2
d§
p
4
d
p
. (5.92)
Convergence of all the integrals over
p
has already
been discussed in Sect. 5.2. The contribution from
the momenta lying far from the Fermi surface is the
same as in the normal phase and can be included
in the definition of the chemical potential. The re-
maining integrations over
p
in Eq. (5.91) converge
rapidly. It is helpful for the following to introduce the
definition
W(p − p
)=
mp
F
(2)
2
|U(p − p
)|
2
. (5.93)
One can immediately verify that Eq.(5.93) is nothing
but the differential probability for the elastic scat-
tering between the two states with momenta p and
p
lying on the Fermi surface and calculated in the
Born approximation. With this in mind we define
the“quasi-classical Green functions”(G.Eilenberger,
1968;A. I. Larkin and Yu. N. Ovchinnikov, 1968) as:
ˆg
p
(r; !
n
)=
d
p
i
ˆ
G(p, r; !
n
) . (5.94)
The self-energy parts Eq. (5.91), after making use of
Eqs.(5.92) and (5.93), acquire the form
ˆ
£(p, r; !
n
)=
i
2
nW(p − p
)ˆg
p
(r; !
n
)d§
p
. (5.95)
Let us now return to the Gor’kov equation (5.82).
We will compare the “left-side” of equation (5.82)
with its “right-side”. We assume that all operators,
@/@,
ˆ
H
0
,
ˆ
¯
H
0
,etc.,apply to the variable x
of the Green
functions
ˆ
G(x, x
) in Eq. (5.77). Correspondingly, in-
stead of Eqs.(5.76) and (5.79) one uses
ˆ
G(x, x
) ·
ˆ
G
0
∗ −1
−
ˆ
V
= ı(x − x
) , (5.96)
where
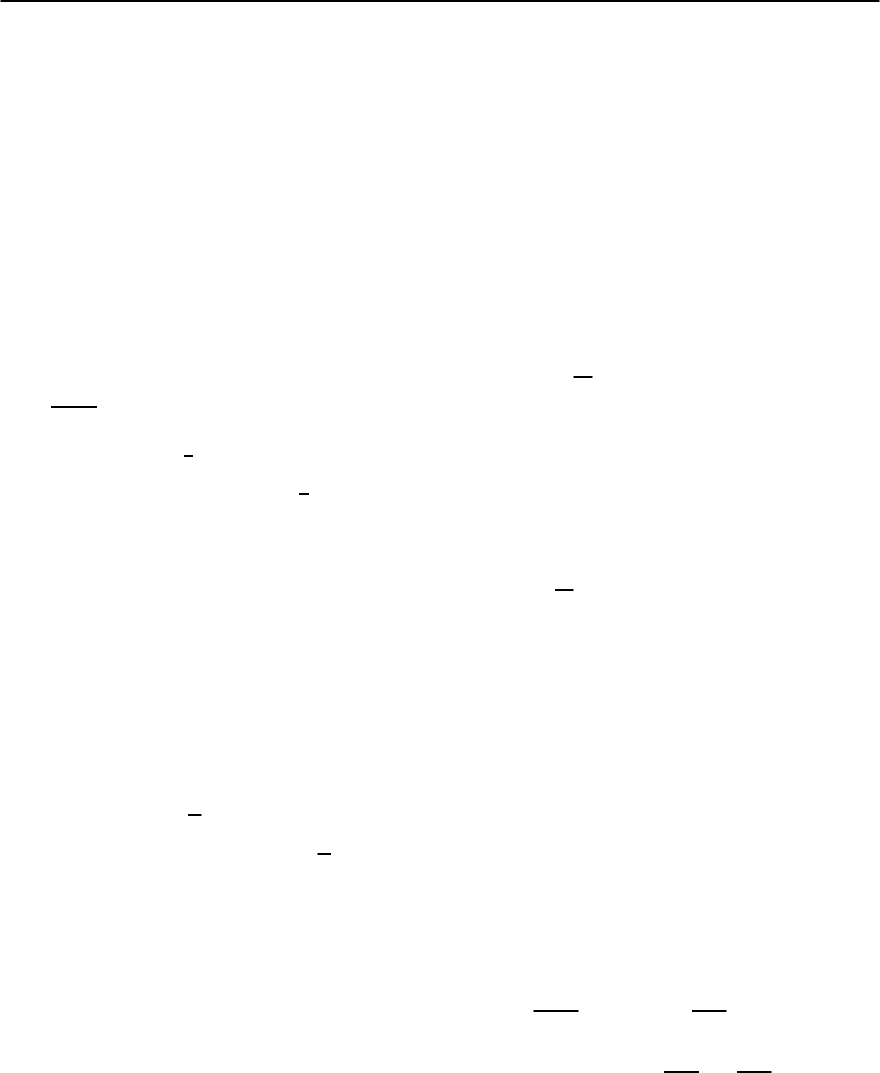
222 L.P. Gor’kov
ˆ
G
0
∗ −1
=
@/@
−(
ˆ
¯
H
0
− ) (r
)
−
†
(r
)−@/@
−(
ˆ
H
0
− )
%
.
(5.97)
There is no needto repeat all the steps that are similar
to those that led us to equations (5.82). One obtains
ˆ
G
ˆ
G
0
∗ −1
−
ˆ
£
= ˆe. (5.98)
Here, the matrices
i!
n
−
p
+ v
F
· k 0
0−i!
n
−
p
+ v
F
· k
%
and
d
3
m
(2)
3
ˆ
G(p, p + m − k; !
n
)
×
⎛
⎝
e
c
v
F
· A(m) (m)
−
†
(m)−
e
c
v
F
· A(m)
⎞
⎠
appear. Upon subtracting equation (5.98) from
Eq. (5.82) one sees that
p
drops out. We write down
the resulting equations for ˆg
p
(r; !
n
)directlyinthe
coordinate representation using as always for k the
correspondence k ⇒ −i∇
r
.Thus,
v
F
·∇
r
ˆg
p
(r; !
n
)+
ˆ
M
p
(r; !
n
) , ˆg
p
(r; !
n
)
=0, (5.99)
where [
ˆ
A,
ˆ
B] denotes the matrix commutation, and
for
ˆ
M
p
(r; !
n
)onehas
ˆ
M
p
(r; !
n
)=
⎛
⎜
⎝
−!
n
+
ie
c
v
F
· A(r) i(r)
−i
†
(r) !
n
−
ie
c
v
F
· A(r)
⎞
⎟
⎠
−
ˆ
£(p, r; !
n
) . (5.100)
Taking the trace of the matrix equation (5.99) one
obtains
(
v
F
·∇
r
)
Tr
ˆg
p
(r; !
n
)
=0. (5.101)
Similarly, for the trace of
ˆg
p
(r; !
n
)
2
one finds the
equation
(
v
F
·∇
r
)
Tr
ˆg
p
(r; !
n
)
2
=0. (5.102)
From Eq. (5.101) it follows
g
p
(r; !
n
)+g
p
(r;−!
n
)=0,
and from Eq. (5.102) we get
g
2
p
(r; !
n
)−f
p
(r; !
n
) f
†
p
(r; !
n
)=1.
So, as one can see, we used the results for a homoge-
neous superconductor (and the boundary conditions
for Eqs (5.101) and (5.102)). One also concludes that
there are actually only two independent functions.
It is instructiveto write the equations forf
p
(r; !
n
)
and f
†
p
(r; !
n
):
v
F
·
−i∇ −
2e
c
A(r)
f
p
(r; !
n
)
−2i!
n
f
p
(r; !
n
)−2(r)g
p
(r;−!
n
)
+
(
i/2
)
n
W(p − p
)
g
p
(r; !
n
)f
p
(r; !
n
)
− f
p
(r; !
n
)g
p
(r; !
n
)
d§
p
=0
and
v
F
·
i∇ −
2e
c
A(r)
f
†
p
(r; !
n
)
−2i!
n
f
†
p
(r; !
n
)−2
†
(r)g
p
(r;−!
n
)
+
(
i/2
)
n
W(p − p
)
g
p
(r; !
n
)f
†
p
(r; !
n
)
− f
†
p
(r; !
n
)g
p
(r; !
n
)
d§
p
=0.
In this form the equations bear some resemblance to
the kinetic equation for the elastic scattering from
impurities and can be treated similarly (see the next
section). One also sees explicitly the gauge invari-
ance of the quasi-classical theory. For the reader’s
convenience we will write down the self-consistency
equation and the expression for the electromagnetic
currents in terms of the quasi-classical Green func-
tions:
(r)=
gmp
F
2
2
iT
n
d§
p
4
f
p
(r; !
n
) , (5.103)
j(r)=−i(2e)2T
!
n
>0
mp
F
2
2
d§
p
4
v
F
g
p
(r; !
n
).
(5.104)
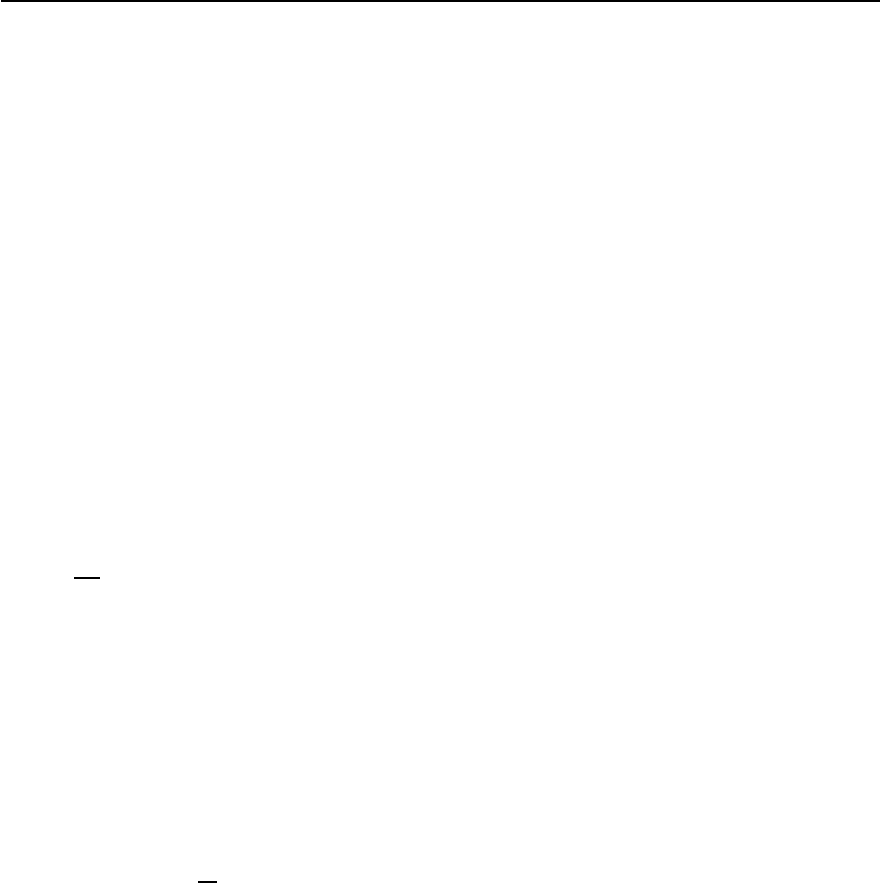
5 Theory of Superconducting Alloys 223
5.5.3 ``Dirty´´ Alloys: Usadel Equations
The limiting case of a short mean-free path, l
0
,
is of special interest from a practical point of view.
As we have seen the critical field is then consider-
ably larger. One also expects some simplifications in
the mathematical apparatus as well. From the formal
standpoint, the “collision terms” are of the order of
1/ and are therefore large. This allows one to write
an expansion for the matrix ˆg
p
(r; !
n
)intheform
ˆg
p
(r; !
n
) ˆg
0
(r; !
n
)+v
F
·ˆg(r; !
n
) , (5.105)
with ˆg ∼ ˆg
0
. Indeed, the isotropic part, ˆg
0
,cancels
the “collision” term. With this in mind, it is easy to
write down the Eilenberger equations in the dirty
limit (K. D. Usadel, 1970 [29]). From the normaliza-
tion condition ˆg
2
=1oneobtains
ˆg
0
ˆg + ˆg ˆg
0
=0. (5.106)
Averaging Eq. (5.99) over momentum directions
gives the following equation
v
2
F
3
ˆ
@ ·ˆg + i (
ˆ
h ˆg
0
− ˆg
0
ˆ
h)=0, (5.107)
whereweintroducethematrices
ˆ
@g =
∇·g −(∇ −2ieA(r)) ·f
−(∇ +2ieA(r)) · f
†
−∇·g
,
(5.108)
and
ˆ
h =
−i!
n
−(r)
†
(r) i!
n
. (5.109)
Multiplying Eq. (5.99) by v
F
and integrating over the
p-direction one arrives at
v
F
ˆ
@ˆg
0
+ i
ˆ
hˆg − ˆg
ˆ
h
+
1
tr
ˆg
0
ˆg − ˆgˆg
0
=0. (5.110)
FinallymultiplyingEq.(5.110) by ˆg
0
one obtains with
the help of Eq. (5.106)
ˆg =−l
tr
ˆg
0
ˆ
@ ˆg
0
(5.111)
(Here, small terms are omitted).After simple algebra
the equation becomes
iD
(
∇ −2ieA
)
·
g
0
(∇ −2ieA)f
0
− f
0
∇g
0
=
2g
0
+2i!
n
f
0
,
and
iD
(
∇ +2ieA
)
·
g
0
(∇ +2ieA)f
†
0
− f
†
0
∇g
0
=
2
∗
g
0
+2i!
n
f
†
0
. (5.112)
Here, D is the diffusion coefficient, D = v
F
l
tr
/3. In
the limit ,
∗
→ 0, the equations for the tempera-
ture dependence of theupper critical field,H
c2
(T)are
found.These Equations were first derived by K.D.Us-
adel (K.D.Usadel,1970 [29]).Anextended discussion
of the Eilenberger formalism and the Usadel equa-
tions and their applications can be found in [30,31].
5.6 Final Remark
As was emphasized from the very beginning, this
chapter is not intended to provide a summary of
the known results for superconducting alloys or the
influence of defects of various kinds on supercon-
ducting properties. The literature on the subject is
enormous. Many applications are concerned with
vortices and their pinning, different phases of the
vortex lattice, etc. With the advent of the era of non-
conventional superconductors, for which the role of
defectsisofutmostimportance,thenumberofpub-
lications on the subject has increased dramatically.
In some of the new superconductors, such as many
cuprates,superconductivity itself arises due to a dop-
ing process (which inevitably produces a disorder).
Nevertheless, the methods described above remain a
major tool to study impurity effects theoretically.

224 L.P. Gor’kov
References
1. A.A.Abrikosov and L.P. Gor’kov,Zh.Exp.Theor. Fiz. 35, 1558, (1958); Sov.Phys.JETP 8,1090 (1959); ibid. 9, 220 (1959)
2. S.F. Edwards, Phylosoph. Magazine 3,1020 (1958)
3. A.A. Abrikosov, L.P. Gor’kov and I.E. Dzyaloshinski, In: Methods of Quantum Field Theory in Statistical Physics
(Prentice-Hall, Englewood Cliffs, NJ,1963)
4. E.M. Lifshitz and L.P. Pitaevskii, In: Statistical Physics, Part 2 (Pergamon Press,Oxford, 1986)
5. L.P. Gor’kov, Sov. Phys. JETP 7, 505 (1958)
6. P.W. Anderson, Jour. Phys. Chem. Solids 11, 26 (1959)
7. A.A.Abrikosov and L.P. Gor’kov, Sov. Phys. JETP 12, 1243 (1960)
8. A.A.Abrikosov and L.P. Gor’kov, Sov. Phys. JETP 15, 752 (1962)
9. A.A. Abrikosov, In: Fundumentals of the Theory of Metals (Elsevier, North-Holland,Amsterdam, 1988)
10. L.C. Hebel and C.P. Slichter, Phys. Rev. 107, 901 (1957); ibid., 113, 1504, (1959)
11. L.P. Gor’kov, Sov. Phys. JETP 10, 998 (1960)
12. K. Maki, Physics 1, 21 (1964)
13. L.P. Gor’kov, Sov. Phys. JETP 9, 1364 (1959)
14. L.P. Gor’kov, Sov. Phys. JETP 10, 593 (1960)
15. V.L.GinzburgandL.D.Landau,Zh.Exp.Teor.Fiz.20, 1064 (1950)
16. A.A.Abrikosov, Sov. Phys.JETP 5, 1174 (1957)
17. L.P. Gor’kov, Sov. Phys. JETP 17, 518 (1963)
18. A.M. Clogston,Phys.Rev. Lett. 9, 266 (1962)
19. B.S. Chandrasekhar, Appl. Phys. Lett. 1, 7 (1962)
20. K.MakiandT.Tsuneto,Progr.Theor.Phys.31, 945 (1964)
21. L.N. Bulaevskii, Sov. Phys. JETP 38, 634 (1974)
22. P. G. de Gennes and M. Tinkham, Physics 1,107 (1964)
23. G. Eilenberger, Z. Physik 217, 195 (1968)
24. A.I. Larkin and Yu.V. Ovchinnikov, Sov. Phys. JETP 28,1200 (1969)
25. G.M. Eliashberg, Sov. Phys. JETP 34, 668 (1972)
26. E.A. Shapoval, Sov. Phys. JETP 20, 675 (1965); ibid. 22, 647 (1966)
27. J.W.Serene and D. Rainer, Phys. Rep. 101, 222 (1983)
28. L.D. Landau and E. M. Lifshitz, In: Quantum Mechanics: Part 1 (Pergamon Press,London,1985)
29. K.D. Usadel, Phys.Rev. Lett.25, 507 (1970)
30. G.L¨uders and K.D. Usadel, In:
The methods of the Correlation Function in Superconductivity Theory (S
pringer, Berlin,
1971)
31. N. Kopnin, In: Theory of Nonequilibrium Superconductivity (Clarendon Press, Oxford, 2001)

6 Impurity Nanostructures and Quantum Interference in Superconductors
D. K. Morr University of Illinois at Chicago, Illinois, USA
6.1 Introduction ..............................................................................225
6.2 Review: Single Impurity Effects in Conventional and Unconventional Superconductors ...........228
6.2.1 Impurities in s-waveSuperconductors...................................................228
6.2.2 Resonant Impurity States in d
x
2
−y
2
-waveSuperconductors.................................229
6.2.3ImpuritiesandFirstOrderQuantumPhaseTransitions....................................230
6.3 Formalism ................................................................................231
6.3.1
ˆ
T-matrixFormalism...................................................................231
6.3.2Bogoliubov–deGennesFormalism......................................................232
6.3.3Real-SpaceDysonEquationforMolecules................................................233
6.4 Quantum Interference and Quantum Imaging in s-wave Superconductors .......................234
6.4.1 Quantum Interference Induced Quantum Phase Transitions in s-waveSuperconductors.......234
6.4.2 Quantum Corrals and Quantum Imaging in s-waveSuperconductors........................240
6.5 Quantum Interference Phenomena in d
x
2
− y
2
-wave Superconductors ............................244
6.6 Molecules on the Surface of d
x
2
− y
2
-wave Superconductors .....................................250
6.7 Conclusion ................................................................................253
References................................................................................256
6.1 Introduction
The study of impurities in superconductors has
attracted significant experimental and theoretical
interest since the mid 1950s. Impurities were first
studied in the context of disorder effects that are
induced by a finite concentration of impurities in
the physical properties of s-wave superconductors.
Motivated by experiments on “dirty” s-wave super-
conductors, Anderson [1], as well as Abrikosov and
Gorkov [2] proposed that since non-magnetic im-
purities do not perturb the pairing of time-reversed
states in an s-wave superconductor, the bulk T
c
as
well as the average bulk superconducting gap are
unaffected by weak non-magnetic impurity scatter-
ing, a result now known as the Ander son theorem.
Subsequently, Abrikosov and Gorkov (AG) [3] in-
vestigated the effects of magnetic impurities on the
bulk properties of s-wave superconductors. They
showed that magnetic impurities are pairbreaking,
since they lift the degeneracy of the spin-↑ and
spin-↓ states. This pairbreaking effect leads to a re-
duction of the bulk superconducting gap, the bulk
critical temperature, T
c
,andtomodificationsinthe
disorder-averaged density of states. The predictions
of the AG theory were subsequently confirmed in
tunneling experiments by Woolf and Reif [4] who
measured the dependence of the density of states on
the impurity concentration. Abrikosov and Gorkov
also predicted the possibility for gapless supercon-
ductivity in s-wave superconductors,an effect which
arises since with increasing impurity concentration
the superconducting gap is suppressed faster than
the superconducting order parameter.

226 D.K.Morr
Incontrast to disorder-averagedproperties,theef-
fect of a single magnetic impurity on the local elec-
tronic structure of an s-wave superconductor were
first studied in the mid 1960s by Lu [5], Shiba [6],
and Rusinov [7]. They demonstrated that the pair-
breaking nature of a magnetic impurity leads to the
formation of a fermionic bound state,whose spectro-
scopic signature in the local density of states (LDOS)
are peaks at energies below the superconducting gap.
Experimental advances in scanning tunneling mi-
croscopy (STM) since the early 1990s, made it pos-
sible to directly test these predictions by measuring
the LDOS in the vicinity of single magnetic and non-
magnetic impurities. In high-resolution STM exper-
iments, Yazdani et al. [8] showed that when a mag-
neticGdatomisplacedonthesurfaceofthes-wave
superconductor Nb, a fermionic bound states is in-
duced in its vicinity whose energy lies inside the su-
perconducting gap. In contrast, the LDOS around a
non-magneticAuatom doesnotexhibitthesignature
of a fermionic bound state. These results confirmed
the predictions by Anderson [1] and Abrikosov and
Gorkov [2,3], as well as those by Lu [5], Shiba [6] and
Rusinov [7] on an atomic length scale.
Thediscoveryofthehigh-temperaturesupercon-
ductors has spurred a significant theoretical [9–24]
and experimental [25–28] interest in the study of sin-
gle impurity effects in these materials. The reasons
for this profound interest are twofold. First, the un-
conventional d
x
2
−y
2
-wave symmetry of the cuprate
superconductors implies that magnetic as well as
non-magnetic impurities are pair-breaking, thus in-
duce fermionic resonance states and hence possess
a dramatic effect on the properties of the super-
conducting state. In addition, due to the d
x
2
−y
2
-wave
symmetry of the superconducting order parameter,
the spatial form of the resonance states is highly
anisotropic. This spatial anisotropy in turn is an im-
portant experimental tool for identifying the sym-
metry of unconventional superconductors in general.
Second, by perturbing the local electronic structure
of a superconductor, impurities can provide insight
into the still elusive microscopicmechanism of high-
temperature superconductivity, which is a topic of
ongoing scientific debate. For example,it was argued
that impurities can provide insight into the nature
of collective excitations in the cuprates [29–31], and
might even be able to reveal the nature of the bosonic
pairing mode giving rise to high-temperature super-
conductivity [32]. Moreover, it was recently shown
that quantum interference effects due to scattering of
multiple impurities yield information on the spatial
and frequency dependence of the superconducting
correlations [33–37] (for a more comprehensive dis-
cussion of impurity effects in superconductors, we
refer the reader to the excellent review by Balatsky,
Vekhter and Zhu [24]).
In contrast to the type of impurities studied in
superconductors, molecules possess internal vibra-
tional or rotational modes whose effects on metallic
surfaces have been extensively investigated in the
context of inelastic electron tunneling spectroscopy
(for a recent review, see [38]) Starting with the study
of trapped (i.e., localized) phonon modes at inter-
faces [39, 40], it was shown that molecular modes
lead to a step-like feature in the LDOS, and a peak
in its first derivative, at the mode frequency [41–43].
Recently,Grossetal.[44]demonstratedthatthe
spatial pattern of the LDOS around a large Lander
molecule is determined by quantum interference be-
tween electrons that are scattered by different parts
of the molecule [45]. Such a molecule therefore rep-
resents a molecular nanostructure in which spatially
separated localized bosonic modes interact with
conduction electrons giving rise to novel quantum
interference effects.
Over the last few years, a new research direction
has emerged in which ordered nanoscale impurity
structures, so-called quantum corrals, are used to
reveal the wavelike nature of electrons in condensed
matter systems [46–51]. Nanostructures are suitable
for this task since their length scale is of the same or-
der as the typical wavelength of electrons, ∼ 1/k
F
(k
F
is the Fermi wave-vector). In a groundbreaking
experiment,Manoharan et al.[48] demonstrated that
electronic waves, similar to light waves, can be used
to form quantum images. In this experiment, an el-
liptical quantum corral consisting of magnetic Co
atoms, was placed on a metallic Cu(111) surface. An
additional Co atom was placed in one of the foci of
the ellipse, while the other focus was left empty. At
low temperature, Manoharan et al. observed Kondo-
