Beer F.P., Johnston E.R., DeWolf J.T., Mazurek D.F. Mechanics of Materials

Подождите немного. Документ загружается.

Apago PDF Enhancer

477

Problems

7.93 The state of plane stress shown will occur at a critical point in an

aluminum casting that is made of an alloy for which s

5 10 ksi

and s

5 25 ksi. Using Mohr’s criterion, determine the shearing

stress t

for which failure should be expected.

8 ksi

␶

Fig. P7.93

80 MP

␶

Fig. P7.94

7.94 The state of plane stress shown will occur at a critical point in

a pipe made of an aluminum alloy for which s

5 75 MPa and

5 150 MPa. Using Mohr’s criterion, determine the shearing

stress t

for which failure should be expected.

7.95 The cast-aluminum rod shown is made of an alloy for which s

60 MPa and s

5 120 MPa. Using Mohr’s criterion, determine the

magnitude of the torque T for which failure should be expected.

26 kN

32 mm

Fig. P7.95

7.96 The cast-aluminum rod shown is made of an alloy for which s

70 MPa and s

5 175 MPa. Knowing that the magnitude T of the

applied torques is slowly increased and using Mohr’s criterion, deter-

mine the shearing stress t

that should be expected at rupture.

7.97 A machine component is made of a grade of cast iron for which

5 8 ksi and s

5 20 ksi. For each of the states of stress

shown, and using Mohr’s criterion, determine the normal stress s

at which rupture of the component should be expected.

␶

Fig. P7.96

␴

(a)(b)(c)

Fig. P7.97

bee80288_ch07_436-511.indd Page 477 11/19/10 2:55:30 PM user-f499bee80288_ch07_436-511.indd Page 477 11/19/10 2:55:30 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07

Apago PDF Enhancer

478

Transformations of Stress and Strain

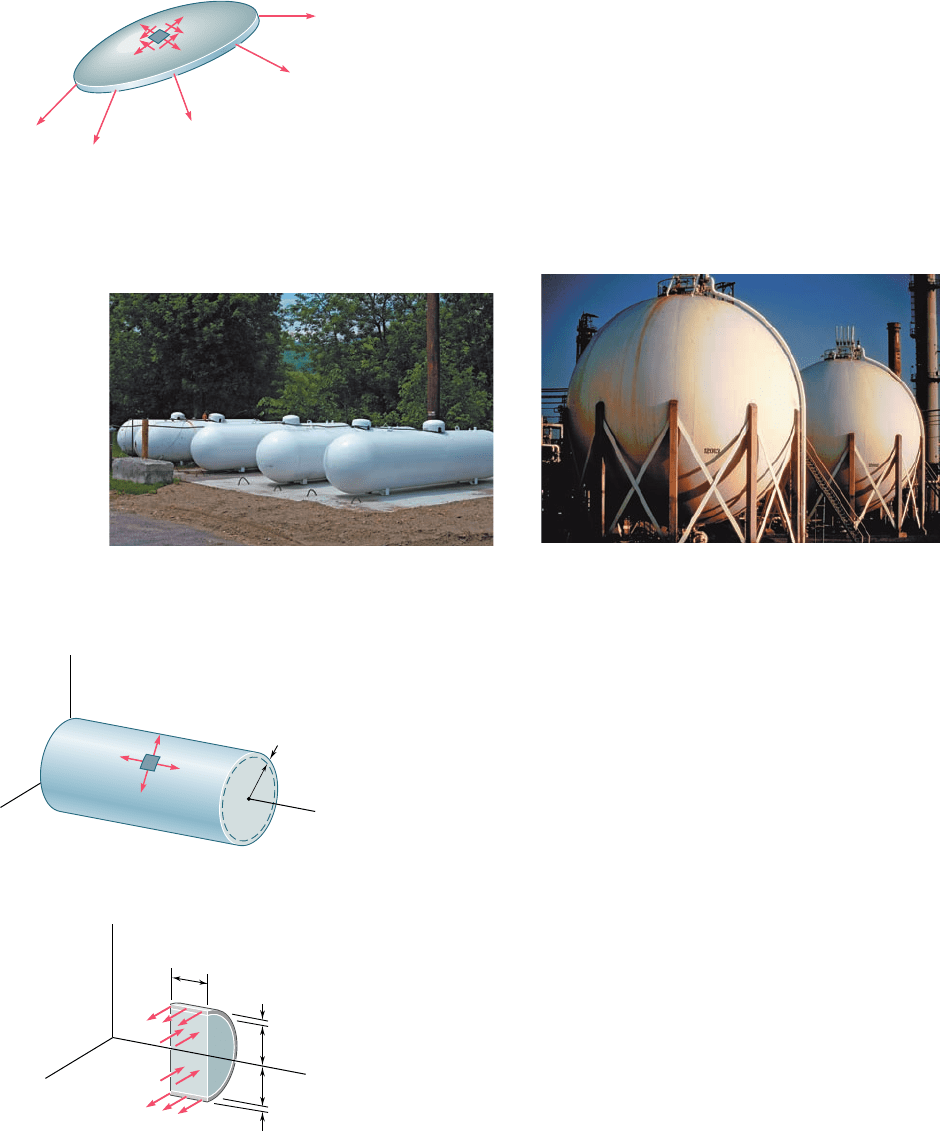
7.9 STRESSES IN THIN-WALLED PRESSURE VESSELS

Thin-walled pressure vessels provide an important application of the

analysis of plane stress. Since their walls offer little resistance to

bending, it can be assumed that the internal forces exerted on a given

portion of wall are tangent to the surface of the vessel (Fig. 7.46).

The resulting stresses on an element of wall will thus be contained

in a plane tangent to the surface of the vessel.

Our analysis of stresses in thin-walled pressure vessels will be lim-

ited to the two types of vessels most frequently encountered: cylindrical

pressure vessels and spherical pressure vessels (Photos 7.3 and 7.4).

Fig. 7.46 Assumed stress distribution

in thin-walled pressure vessels.

Photo 7.3 Cylindrical pressure vessels.

Photo 7.4 Spherical pressure vessels.

Consider a cylindrical vessel of inner radius r and wall thickness

t containing a fluid under pressure (Fig. 7.47). We propose to deter-

mine the stresses exerted on a small element of wall with sides

respectively parallel and perpendicular to the axis of the cylinder.

Because of the axisymmetry of the vessel and its contents, it is clear

that no shearing stress is exerted on the element. The normal stresses

and s

shown in Fig. 7.47 are therefore principal stresses. The

stress s

is known as the hoop stress, because it is the type of stress

found in hoops used to hold together the various slats of a wooden

barrel, and the stress s

is called the longitudinal stress.

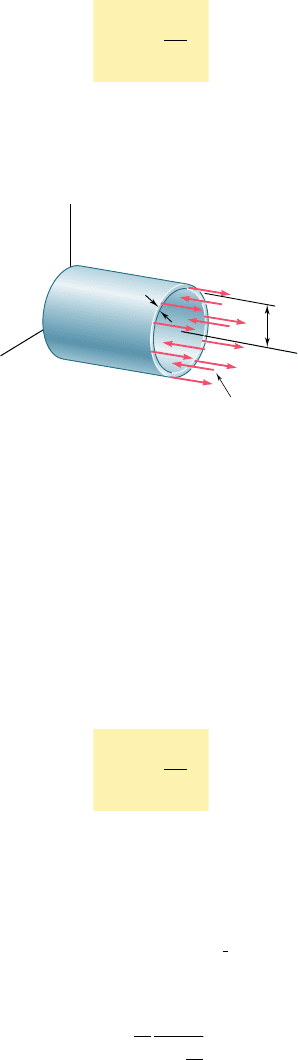
In order to determine the hoop stress s

, we detach a portion of

the vessel and its contents bounded by the xy plane and by two planes

parallel to the yz plane at a distance Dx from each other (Fig. 7.48).

The forces parallel to the z axis acting on the free body defined in this

fashion consist of the elementary internal forces s

dA on the wall

sections, and of the elementary pressure forces p dA exerted on the

portion of fluid included in the free body. Note that p denotes the gage

pressure of the fluid, i.e., the excess of the inside pressure over the

outside atmospheric pressure. The resultant of the internal forces s

is equal to the product of s

and of the cross-sectional area 2t Dx of

the wall, while the resultant of the pressure forces p dA is equal to the

product of p and of the area 2r Dx. Writing the equilibrium equation

5 0, we have

␴

Fig. 7.47 Pressurized cylindrical vessel.

Fig. 7.48 Free body to determine

hoop stress.

␴

p dA

␴

⌬

bee80288_ch07_436-511.indd Page 478 11/19/10 2:55:52 PM user-f499bee80288_ch07_436-511.indd Page 478 11/19/10 2:55:52 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07

Apago PDF Enhancer

479

©F

5 0: s

2t ¢x

2 p

2r ¢x

5 0

and, solving for the hoop stress s

(7.30)

To determine the longitudinal stress s

, we now pass a section

perpendicular to the x axis and consider the free body consisting of the

portion of the vessel and its contents located to the left of the section

7.9 Stresses in Thin-Walled Pressure Vessels

†Using the mean radius of the wall section, r

5 r 1

t, in computing the resultant of

the forces on that section, we would obtain a more accurate value of the longitudinal

stress, namely,

1 1

(7.319)

However, for a thin-walled pressure vessel, the term ty2r is sufficiently small to allow the

use of Eq. (7.31) for engineering design and analysis. If a pressure vessel is not thin-walled

(i.e., if ty2r is not small), the stresses s

and s

vary across the wall and must be deter-

mined by the methods of the theory of elasticity.



p dA

Fig. 7.49 Free body to determine

longitudinal stress.

(Fig. 7.49). The forces acting on this free body are the elementary inter-

nal forces s

dA on the wall section and the elementary pressure forces

p dA exerted on the portion of fluid included in the free body. Noting

that the area of the fluid section is pr

and that the area of the wall

section can be obtained by multiplying the circumference 2pr of the

cylinder by its wall thickness t, we write the equilibrium equation:†

5 0: s

12prt22 p1pr

25 0

and, solving for the longitudinal stress s

(7.31)

We note from Eqs. (7.30) and (7.31) that the hoop stress s

is twice

as large as the longitudinal stress s

(7.32)

bee80288_ch07_436-511.indd Page 479 10/30/10 1:42:01 AM user-f499bee80288_ch07_436-511.indd Page 479 10/30/10 1:42:01 AM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07

Apago PDF Enhancer

480

Transformations of Stress and Strain

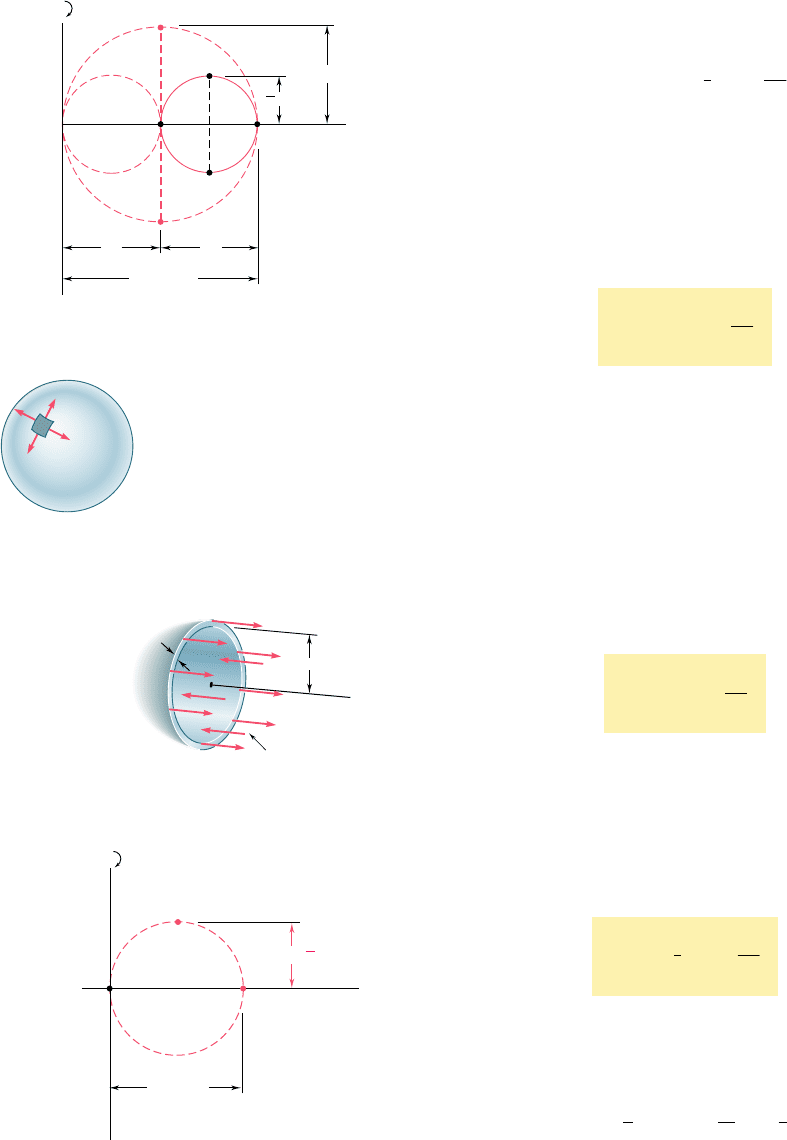
Drawing Mohr’s circle through the points A and B that corre-

spond respectively to the principal stresses s

and s

(Fig. 7.50), and

recalling that the maximum in-plane shearing stress is equal to the

radius of this circle, we have

max 1in plane2

(7.33)

This stress corresponds to points D and E and is exerted on an ele-

ment obtained by rotating the original element of Fig. 7.47 through

458 within the plane tangent to the surface of the vessel. The maxi-

mum shearing stress in the wall of the vessel, however, is larger. It

is equal to the radius of the circle of diameter OA and corresponds

to a rotation of 458 about a longitudinal axis and out of the plane of

stress.† We have

max

5 s

(7.34)

We now consider a spherical vessel of inner radius r and wall

thickness t, containing a fluid under a gage pressure p. For reasons

of symmetry, the stresses exerted on the four faces of a small element

of wall must be equal (Fig. 7.51). We have

(7.35)

To determine the value of the stress, we pass a section through the

center C of the vessel and consider the free body consisting of the

portion of the vessel and its contents located to the left of the section

(Fig. 7.52). The equation of equilibrium for this free body is the

same as for the free body of Fig. 7.49. We thus conclude that, for a

spherical vessel,

5 s

(7.36)

Since the principal stresses s

and s

are equal, Mohr’s circle for

transformations of stress within the plane tangent to the surface of the

vessel reduces to a point (Fig. 7.53); we conclude that the in-plane

normal stress is constant and that the in-plane maximum shearing stress

is zero. The maximum shearing stress in the wall of the vessel, however,

is not zero; it is equal to the radius of the circle of diameter OA and

corresponds to a rotation of 458 out of the plane of stress. We have

max

(7.37)









max





Fig. 7.50 Mohr’s circle for element of

cylindrical pressure vessel.





Fig. 7.51 Pressurized

spherical vessel.



p dA

Fig. 7.52 Free body to

determine wall stress.

max











Fig. 7.53 Mohr’s circle for element

of spherical pressure vessel.

†It should be observed that, while the third principal stress is zero on the outer surface of

the vessel, it is equal to 2p on the inner surface, and is represented by a point C(2p, 0)

on a Mohr-circle diagram. Thus, close to the inside surface of the vessel, the maximum

shearing stress is equal to the radius of a circle of diameter CA, and we have

max

1 p25

1 1

For a thin-walled vessel, however, the term t/r is small, and we can neglect the variation

of t

max

across the wall section. This remark also applies to spherical pressure vessels.

bee80288_ch07_436-511.indd Page 480 10/30/10 1:42:04 AM user-f499bee80288_ch07_436-511.indd Page 480 10/30/10 1:42:04 AM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07

Apago PDF Enhancer

481

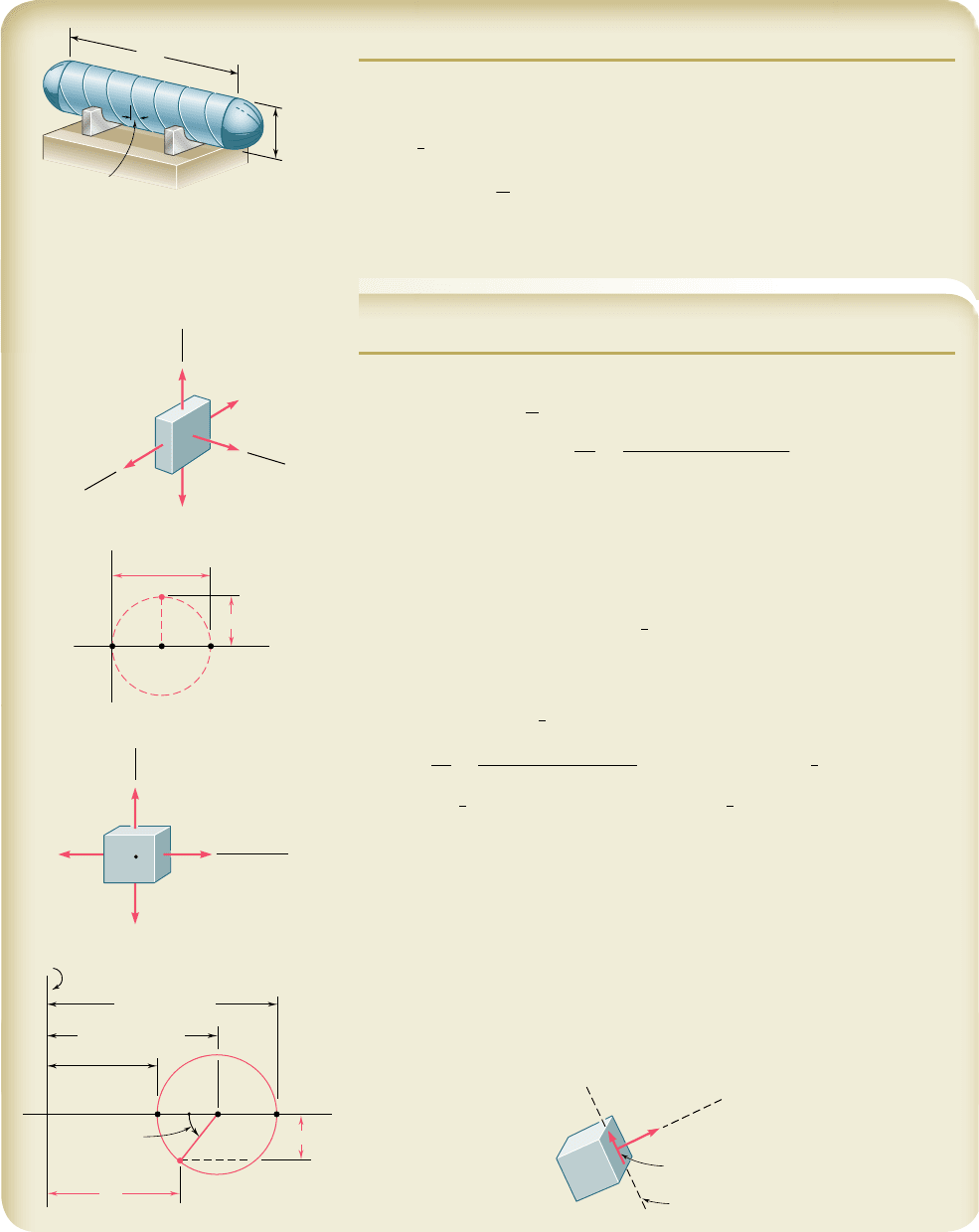
SAMPLE PROBLEM 7.5

A compressed-air tank is supported by two cradles as shown; one of the cra-

dles is designed so that it does not exert any longitudinal force on the tank.

The cylindrical body of the tank has a 30-in. outer diameter and is fabricated

from a

-in. steel plate by butt welding along a helix that forms an angle of

258 with a transverse plane. The end caps are spherical and have a uniform

wall thickness of

in. For an internal gage pressure of 180 psi, determine

(a) the normal stress and the maximum shearing stress in the spherical caps.

(b) the stresses in directions perpendicular and parallel to the helical weld.

SOLUTION

a. Spherical Cap. Using Eq. (7.36), we write

5 180

si, t 5

in. 5 0.3125 in., r 5 15 2 0.3125 5 14.688 in.

5 s

1180 psi2114.688 in.

210.3125 in.2

s 5 4230 psib

We note that for stresses in a plane tangent to the cap, Mohr’s circle reduces

to a point (A, B) on the horizontal axis and that all in-plane shearing stresses

are zero. On the surface of the cap the third principal stress is zero and cor-

responds to point O. On a Mohr’s circle of diameter AO, point D9 represents

the maximum shearing stress; it occurs on planes at 458 to the plane tangent

to the cap.

max

14230 psi2 t

max

5 2115 psi

b. Cylindrical Body of the Tank. We first determine the hoop stress

and the longitudinal stress s

. Using Eqs. (7.30) and (7.32), we write

5 180

si, t 5

in. 5 0.375 in., r 5 15 2 0.375 5 14.625 in.

180 psi

14.625 in.

375 in

5 7020 psis

5 3510 psi

ave

1 s

25 5265 psiR 5

2 s

25 1755 psi

Stresses at the Weld. Noting that both the hoop stress and the longi-

tudinal stress are principal stresses, we draw Mohr’s circle as shown.

An element having a face parallel to the weld is obtained by rotating the

face perpendicular to the axis Ob counterclockwise through 258. Therefore, on

Mohr’s circle we locate the point X9 corresponding to the stress components

on the weld by rotating radius CB counterclockwise through 2u 5 508.

5 s

ave

2 R cos 50° 5 5265 2 1755 cos 50° s

514140 psib

5 R sin 50° 5 1755 sin 50° t

5 



1344 psib

Since X9 is below the horizontal axis, t

tends to rotate the element

counterclockwise.

8 ft

30 in.

25°



 0



max





 4230 psi

A, B



 3510 psi

 7020 psi



 7020 psi

ave



 5265 psi



 3510 psi

 1755 psi





2  50°







 4140 psi



 1344 psi

Weld

bee80288_ch07_436-511.indd Page 481 11/17/10 11:11:11 PM user-f499bee80288_ch07_436-511.indd Page 481 11/17/10 11:11:11 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07

Apago PDF Enhancer

PROBLEMS

482

7.98 A spherical gas container made of steel has a 5-m outer diameter

and a wall thickness of 6 mm. Knowing that the internal pressure

is 350 kPa, determine the maximum normal stress and the maxi-

mum shearing stress in the container.

7.99 The maximum gage pressure is known to be 8 MPa in a spherical

steel pressure vessel having a 250-mm outer diameter and a 6-mm

wall thickness. Knowing that the ultimate stress in the steel used

is s

5 400 MPa, determine the factor of safety with respect to

tensile failure.

7.100 A basketball has a 9.5-in. outer diameter and a 0.125-in. wall thick-

ness. Determine the normal stress in the wall when the basketball

is inflated to a 9-psi gage pressure.

7.101 A spherical pressure vessel of 900-mm outer diameter is to be

fabricated from a steel having an ultimate stress s

5 400 MPa.

Knowing that a factor of safety of 4.0 is desired and that the gage

pressure can reach 3.5 MPa, determine the smallest wall thickness

that should be used.

7.102 A spherical pressure vessel has an outer diameter of 10 ft and a

wall thickness of 0.5 in. Knowing that for the steel used s

all

12 ksi, E 5 29 3 10

psi, and n 5 0.29, determine (a) the allowable

gage pressure, (b) the corresponding increase in the diameter of

the vessel.

7.103 A spherical gas container having an outer diameter of 5 m and a

wall thickness of 22 mm is made of steel for which E 5 200 GPa

and n 5 0.29. Knowing that the gage pressure in the container is

increased from zero to 1.7 MPa, determine (a) the maximum nor-

mal stress in the container, (b) the corresponding increase in the

diameter of the container.

7.104 A steel penstock has a 750-mm outer diameter, a 12-mm wall thick-

ness, and connects a reservoir at A with a generating station at B.

Knowing that the density of water is 1000 kg/m

, determine the

maximum normal stress and the maximum shearing stress in the

penstock under static conditions.

7.105 A steel penstock has a 750-mm outer diameter and connects a

reservoir at A with a generating station at B. Knowing that the

density of water is 1000 kg/m

and that the allowable normal stress

in the steel is 85 MPa, determine the smallest thickness that can

be used for the penstock.

7.106 The bulk storage tank shown in Photo 7.3 has an outer diameter

of 3.3 m and a wall thickness of 18 mm. At a time when the internal

pressure of the tank is 1.5 MPa, determine the maximum normal

stress and the maximum shearing stress in the tank.

750 mm

300 m

Fig. P7.104 and P7.105

bee80288_ch07_436-511.indd Page 482 10/30/10 1:42:29 AM user-f499bee80288_ch07_436-511.indd Page 482 10/30/10 1:42:29 AM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07

Apago PDF Enhancer

483

Problems

7.107 Determine the largest internal pressure that can be applied to a

cylindrical tank of 5.5-ft outer diameter and

-in. wall thickness if

the ultimate normal stress of the steel used is 65 ksi and a factor

of safety of 5.0 is desired.

7.108 A cylindrical storage tank contains liquefied propane under a pres-

sure of 1.5 MPa at a temperature of 388C. Knowing that the tank

has an outer diameter of 320 mm and a wall thickness of 3 mm,

determine the maximum normal stress and the maximum shearing

stress in the tank.

7.109 The unpressurized cylindrical storage tank shown has a

-in. wall

thickness and is made of steel having a 60-ksi ultimate strength in

tension. Determine the maximum height h to which it can be filled

with water if a factor of safety of 4.0 is desired. (Specific weight

of water 5 62.4 lb/ft

7.110 For the storage tank of Prob. 7.109, determine the maximum nor-

mal stress and the maximum shearing stress in the cylindrical wall

when the tank is filled to capacity (h 5 48 ft).

7.111 A standard-weight steel pipe of 12-in. nominal diameter carries

water under a pressure of 400 psi. (a) Knowing that the outside

diameter is 12.75 in. and the wall thickness is 0.375 in., determine

the maximum tensile stress in the pipe. (b) Solve part a, assuming

an extra-strong pipe is used, of 12.75-in. outside diameter and

0.5-in. wall thickness.

7.112 The pressure tank shown has a 8-mm wall thickness and butt-welded

seams forming an angle b 5 208 with a transverse plane. For a gage

pressure of 600 kPa, determine, (a) the normal stress perpendicular

to the weld, (b) the shearing stress parallel to the weld.

7.113 For the tank of Prob. 7.112, determine the largest allowable gage

pressure, knowing that the allowable normal stress perpendicular

to the weld is 120 MPa and the allowable shearing stress parallel

to the weld is 80 MPa.

7.114 For the tank of Prob. 7.112, determine the range of values of b

that can be used if the shearing stress parallel to the weld is not

to exceed 12 MPa when the gage pressure is 600 kPa.

7.115 The steel pressure tank shown has a 750-mm inner diameter and

a 9-mm wall thickness. Knowing that the butt-welded seams form

an angle b 5 508 with the longitudinal axis of the tank and that

the gage pressure in the tank is 1.5 MPa, determine, (a) the normal

stress perpendicular to the weld, (b) the shearing stress parallel to

the weld.

7.116 The pressurized tank shown was fabricated by welding strips of

plate along a helix forming an angle b with a transverse plane.

Determine the largest value of b that can be used if the normal

stress perpendicular to the weld is not to be larger than 85 percent

of the maximum stress in the tank.

25 ft

48 ft

Fig. P7.109

3 m

1.6 m



Fig. P7.112



Fig. P7.115 and P7.116

bee80288_ch07_436-511.indd Page 483 10/30/10 1:42:33 AM user-f499bee80288_ch07_436-511.indd Page 483 10/30/10 1:42:33 AM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07

Apago PDF Enhancer

484

Transformations of Stress and Strain

7.117 The cylindrical portion of the compressed-air tank shown is fabri-

cated of 0.25-in.-thick plate welded along a helix forming an angle

b 5 308 with the horizontal. Knowing that the allowable stress

normal to the weld is 10.5 ksi, determine the largest gage pressure

that can be used in the tank.

7.118 For the compressed-air tank of Prob. 7.117, determine the gage

pressure that will cause a shearing stress parallel to the weld of

4 ksi.

7.119 Square plates, each of 0.5-in. thickness, can be bent and welded

together in either of the two ways shown to form the cylindrical

portion of a compressed-air tank. Knowing that the allowable nor-

mal stress perpendicular to the weld is 12 ksi, determine the largest

allowable gage pressure in each case.

20 in.



60 in.

Fig. P7.117

20 ft

12 ft 12 ft

45

(a)(b)

Fig. P7.119

7.120 The compressed-air tank AB has an inner diameter of 450 mm and

a uniform wall thickness of 6 mm. Knowing that the gage pressure

inside the tank is 1.2 MPa, determine the maximum normal stress

and the maximum in-plane shearing stress at point a on the top of

the tank.

7.121 For the compressed-air tank and loading of Prob. 7.120, determine

the maximum normal stress and the maximum in-plane shearing

stress at point b on the top of the tank.

750 mm

500 mm

750 mm

5 kN

Fig. P7.120

bee80288_ch07_436-511.indd Page 484 10/30/10 1:42:40 AM user-f499bee80288_ch07_436-511.indd Page 484 10/30/10 1:42:40 AM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07

Apago PDF Enhancer

485

Problems

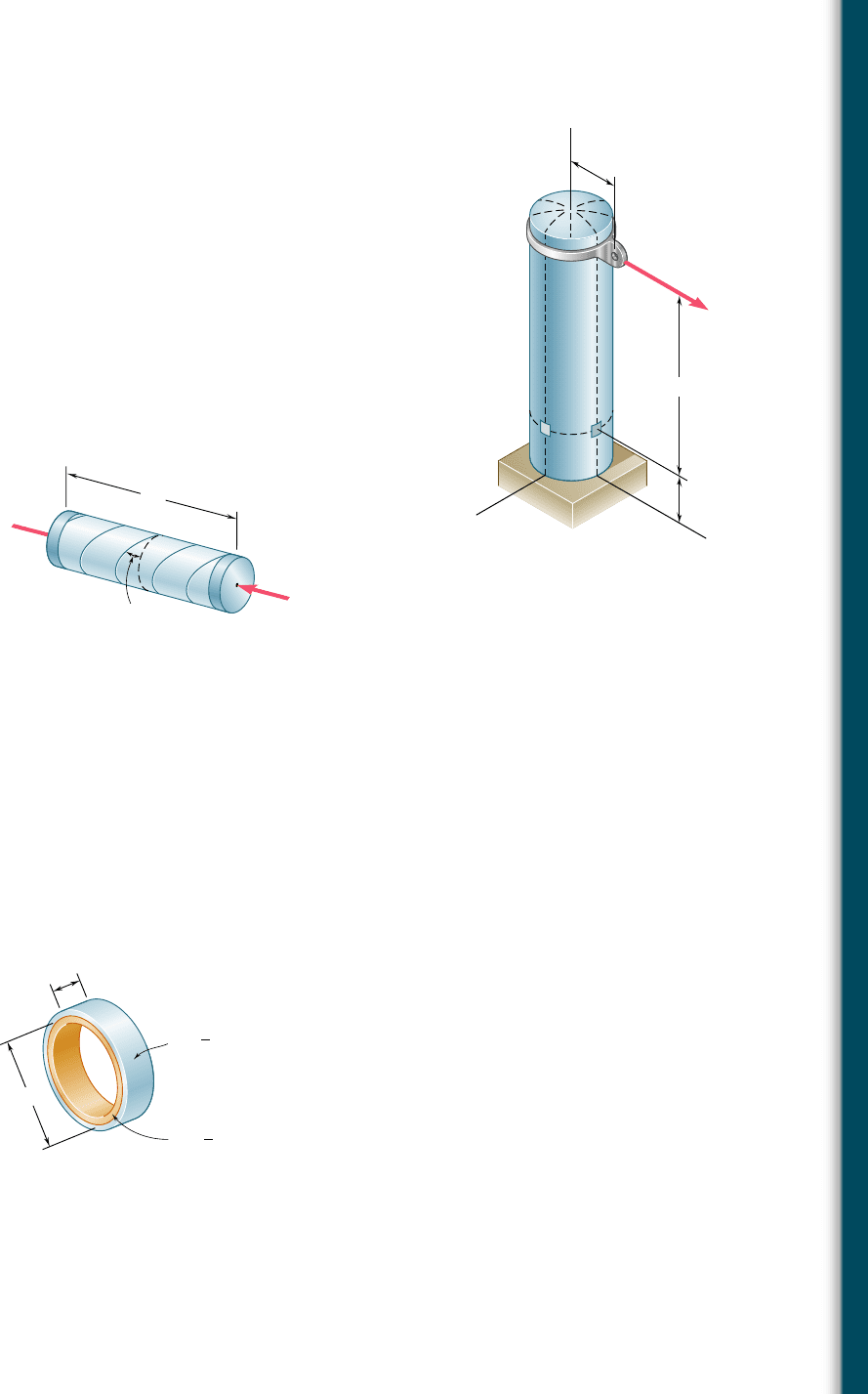
7.122 The compressed-air tank AB has a 250-mm outside diameter and

an 8-mm wall thickness. It is fitted with a collar by which a 40-kN

force P is applied at B in the horizontal direction. Knowing that

the gage pressure inside the tank is 5 MPa, determine the maxi-

mum normal stress and the maximum shearing stress at point K.

7.123 In Prob. 7.122, determine the maximum normal stress and the

maximum shearing stress at point L.

7.124 A pressure vessel of 10-in. inner diameter and 0.25-in. wall thick-

ness is fabricated from a 4-ft section of spirally-welded pipe AB

and is equipped with two rigid end plates. The gage pressure inside

the vessel is 300 psi and 10-kip centric axial forces P and P9 are

applied to the end plates. Determine (a) the normal stress perpen-

dicular to the weld, (b) the shearing stress parallel to the weld.

7.125 Solve Prob. 7.124, assuming that the magnitude P of the two forces

is increased to 30 kips.

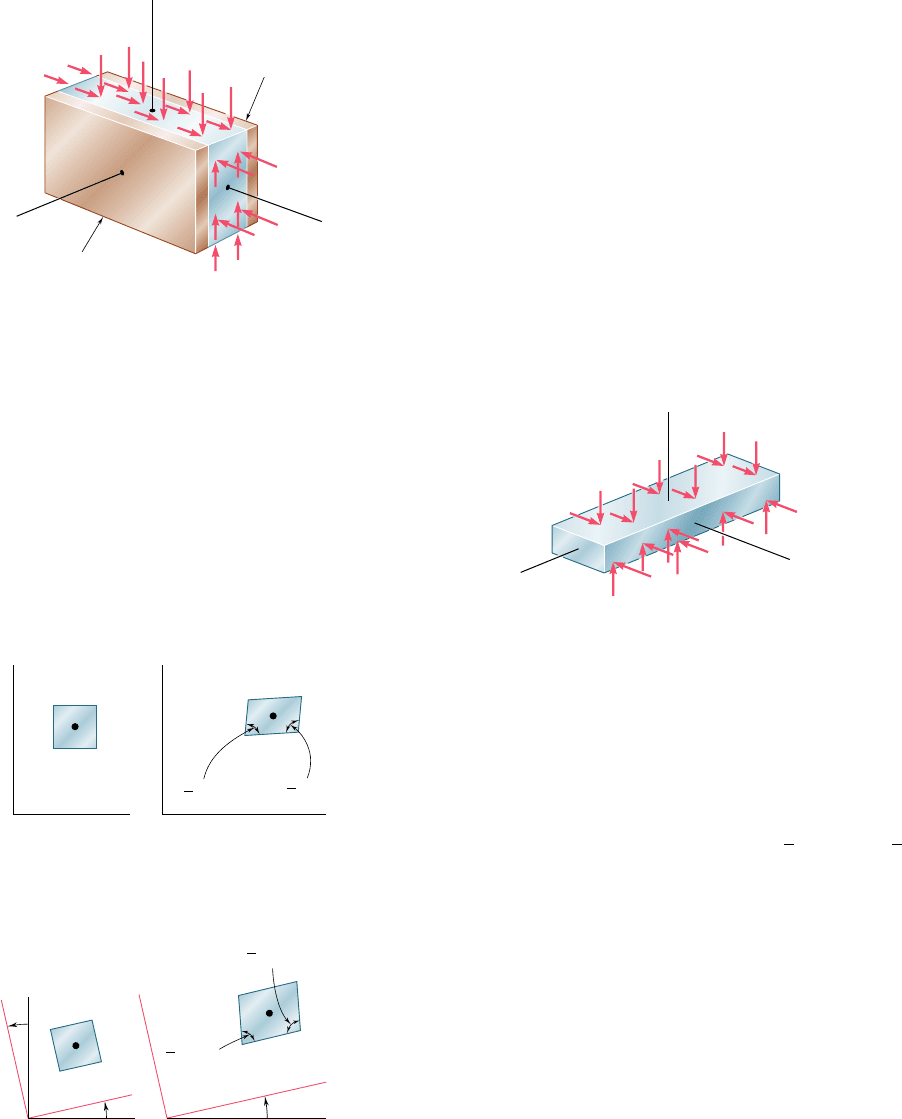
7.126 A brass ring of 5-in. outer diameter and 0.25-in. thickness fits

exactly inside a steel ring of 5-in. inner diameter and 0.125-in.

thickness when the temperature of both rings is 508F. Knowing

that the temperature of both rings is then raised to 1258F, deter-

mine (a) the tensile stress in the steel ring, (b) the corresponding

pressure exerted by the brass ring on the steel ring.

600 mm

150 mm

Fig. P7.122

4 ft

35

Fig. P7.124

7.127 Solve Prob. 7.126, assuming that the brass ring is 0.125 in. thick

and the steel ring is 0.25 in. thick.

STEEL



 29  10

psi



 6.5  10

–6

/F

in.



BRASS



 15  10

psi



 11.6  10

–

/F

in.



1.5 in.

5 in.

Fig. P7.126

bee80288_ch07_436-511.indd Page 485 10/30/10 1:42:47 AM user-f499bee80288_ch07_436-511.indd Page 485 10/30/10 1:42:47 AM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07

Apago PDF Enhancer

486

Transformations of Stress and Strain

*7.10 TRANSFORMATION OF PLANE STRAIN

Transformations of strain under a rotation of the coordinate axes will

now be considered. Our analysis will first be limited to states of plane

strain, i.e., to situations where the deformations of the material take

place within parallel planes, and are the same in each of these planes.

If the z axis is chosen perpendicular to the planes in which the defor-

mations take place, we have P

5 g

5 0, and the only remaining

strain components are P

, P

, and g

. Such a situation occurs in a plate

subjected along its edges to uniformly distributed loads and restrained

from expanding or contracting laterally by smooth, rigid, and fixed sup-

ports (Fig. 7.54). It would also be found in a bar of infinite length

subjected on its sides to uniformly distributed loads since, by reason of

symmetry, the elements located in a given transverse plane cannot

move out of that plane. This idealized model shows that, in the actual

case of a long bar subjected to uniformly distributed transverse loads

(Fig. 7.55), a state of plane strain exists in any given transverse section

that is not located too close to either end of the bar.†

†It should be observed that a state of plane strain and a state of plane stress (cf. Sec. 7.1)

do not occur simultaneously, except for ideal materials with a Poisson ratio equal to zero.

The constraints placed on the elements of the plate of Fig. 7.54 and of the bar of Fig. 7.55

result in a stress s

different from zero. On the other hand, in the case of the plate of Fig.

7.3, the absence of any lateral restraint results in s

5 0 and P

Z 0.

Fixed support

Fig. 7.54 Plane strain example: laterally

restrained plate.

Fig. 7.55 Plane strain example: bar

of infinite length.

s

s (1  )



)





s (1 











Fig. 7.56 Plane strain element deformation.

s



s

s (1  )



s (1  )





x'y'







x'y'







Fig. 7.57 Transformation of plane strain

element.

Let us assume that a state of plane strain exists at point Q (with

5 g

5 0), and that it is defined by the strain components

, P

, and g

associated with the x and y axes. As we know from

Secs. 2.12 and 2.14, this means that a square element of center Q,

with sides of length Ds respectively parallel to the x and y axes, is

deformed into a parallelogram with sides of length respectively equal

to Ds (1 1 P

) and Ds (1 1 P

), forming angles of

2 g

and

1 g

with each other (Fig. 7.56). We recall that, as a result of the defor-

mations of the other elements located in the xy plane, the element

considered may also undergo a rigid-body motion, but such a motion

is irrelevant to the determination of the strains at point Q and will

be ignored in this analysis. Our purpose is to determine in terms of

, P

, g

, and u the strain components P

, P

, and g

x9y9

associated

with the frame of reference x9y9 obtained by rotating the x and y

axes through the angle u. As shown in Fig. 7.57, these new strain

bee80288_ch07_436-511.indd Page 486 10/30/10 3:32:34 PM user-f499bee80288_ch07_436-511.indd Page 486 10/30/10 3:32:34 PM user-f499/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch07/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch