Beer F.P., Johnston E.R., DeWolf J.T., Mazurek D.F. Mechanics of Materials

Подождите немного. Документ загружается.

Apago PDF Enhancer

307

Review and Summary

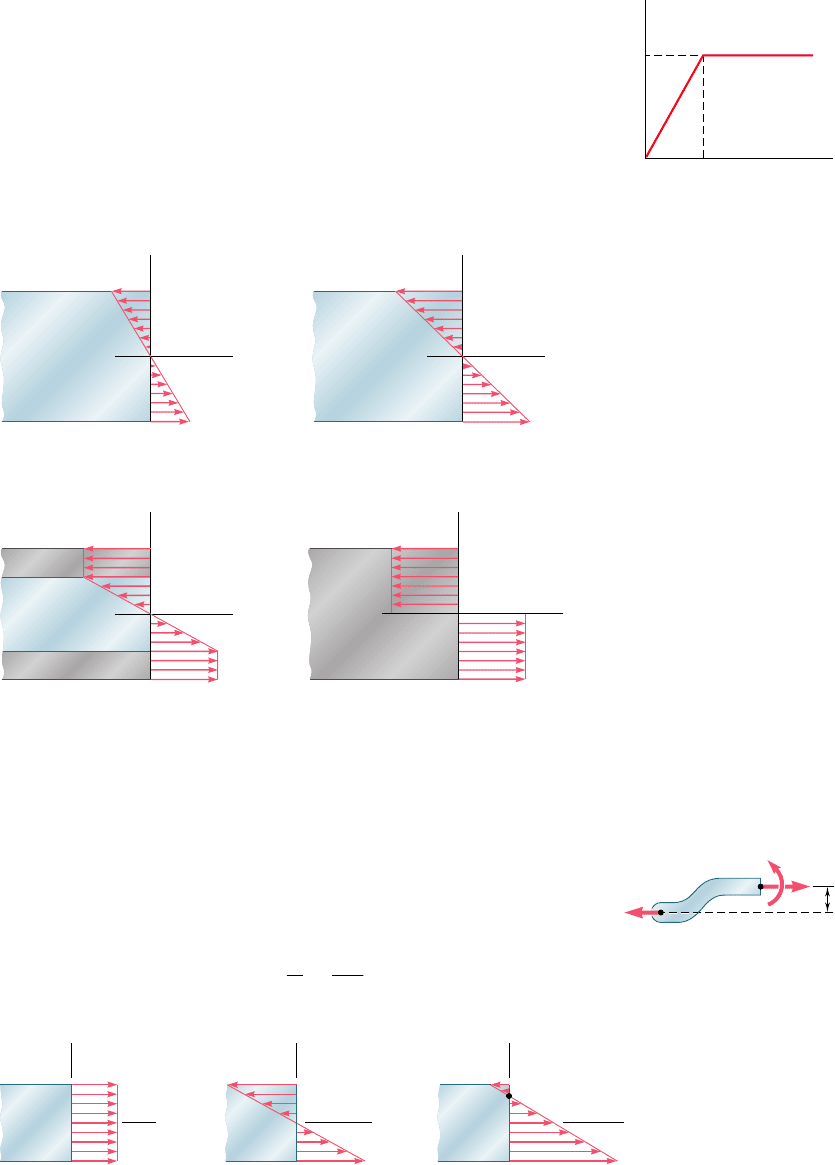
We next investigated members made of materials that do not

follow Hooke’s law [Sec. 4.8]. A rectangular beam made of an elas-

toplastic material (Fig. 4.82) was analyzed as the magnitude of the

bending moment was increased. The maximum elastic moment M

occurred when yielding was initiated in the beam (Fig. 4.83). As the

bending moment was further increased, plastic zones developed and

the size of the elastic core of the member decreased [Sec. 4.9].

Finally the beam became fully plastic and we obtained the maximum

or plastic moment M

. In Sec. 4.11, we found that permanent defor-

mations and residual stresses remain in a member after the loads

that caused yielding have been removed.





Fig. 4.82



N.A.



Fig. 4.85

ELASTIC



c



max









(a) MM

ELASTIC



c



max









(b) MM



ELASTIC

PLASTIC

c



max















PLASTIC

c







(d) MM



Fig. 4.83

Plastic deformations

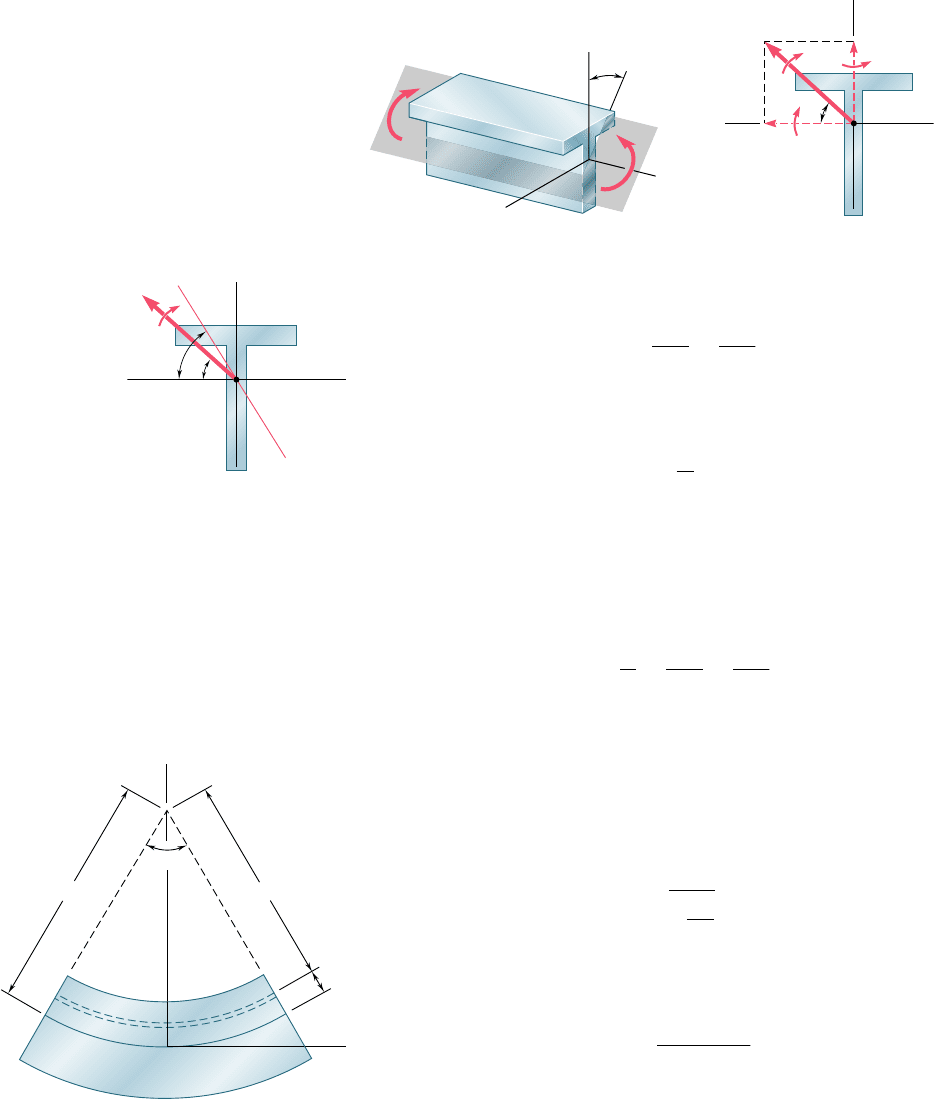
In Sec. 4.12, we studied the stresses in members loaded eccentrically

in a plane of symmetry. Our analysis made use of methods developed

earlier. We replaced the eccentric load by a force-couple system

located at the centroid of the cross section (Fig. 4.84) and then

superposed stresses due to the centric load and the bending couple

(Fig. 4.85):

(4.50)

Fig. 4.84

Eccentric axial loading

bee80288_ch04_220-313.indd Page 307 10/26/10 4:35:47 PM user-f499bee80288_ch04_220-313.indd Page 307 10/26/10 4:35:47 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles

Apago PDF Enhancer

308

Pure Bending

The bending of members of unsymmetric cross section was consid-

ered next [Sec. 4.13]. We found that the flexure formula may be

used, provided that the couple vector M is directed along one of the

principal centroidal axes of the cross section. When necessary we

resolved M into components along the principal axes and superposed

the stresses due to the component couples (Figs. 4.86 and 4.87).

(4.55)

For the couple M shown in Fig. 4.88, we determined the ori-

entation of the neutral axis by writing

tan f 5

tan u

(4.57)

The general case of eccentric axial loading was considered in

Sec. 4.14, where we again replaced the load by a force-couple system

located at the centroid. We then superposed the stresses due to the

centric load and two component couples directed along the principal

axes:

(4.58)

The chapter concluded with the analysis of stresses in curved mem-

bers (Fig. 4.89). While transverse sections remain plane when the

member is subjected to bending, we found that the stresses do not

vary linearly and the neutral surface does not pass through the cen-

troid of the section. The distance R from the center of curvature of

the member to the neutral surface was found to be

R 5

(4.66)

where A is the area of the cross section. The normal stress at a dis-

tance y from the neutral surface was expressed as

Ae1R 2 y2

(4.70)

where M is the bending moment and e the distance from the cen-

troid of the section to the neutral surface.

Unsymmetric bending



Fig. 4.86



Fig. 4.87

N. A.





Fig. 4.88



Fig. 4.89

General eccentric axial loading

Curved members

bee80288_ch04_220-313.indd Page 308 11/11/10 3:03:22 PM user-f499bee80288_ch04_220-313.indd Page 308 11/11/10 3:03:22 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04

Apago PDF Enhancer

309

REVIEW PROBLEMS

4.192 Two vertical forces are applied to a beam of the cross section

shown. Determine the maximum tensile and compressive stresses

in portion BC of the beam.

4.193 Straight rods of 6-mm diameter and 30-m length are stored by

coiling the rods inside a drum of 1.25-m inside diameter. Assum-

ing that the yield strength is not exceeded, determine (a) the maxi-

mum stress in a coiled rod, (b) the corresponding bending moment

in the rod. Use E 5 200 GPa.

DCBA

6 in.

2 in.

3 in.3 in.

15 kips 15 kips

3 in.

40 in. 40 in.

60 in.

Fig. P4.192

Fig. P4.193

4.194 Knowing that for the beam shown the allowable stress is 12 ksi in

tension and 16 ksi in compression, determine the largest couple M

that can be applied.

4.195 In order to increase corrosion resistance, a 2-mm-thick cladding of

aluminum has been added to a steel bar as shown. The modulus

of elasticity is 200 GPa for steel and 70 GPa for aluminum. For a

bending moment of 300 N ? m, determine (a) the maximum stress

in the steel, (b) the maximum stress in the aluminum, (c) the radius

of curvature of the bar.

1.2 in.

0.75 in.

2.4 in.

Fig. P4.194

46 mm

50 mm

M  300 N · m

30 mm

26 mm

Fig. P4.195

4.196 A single vertical force P is applied to a short steel post as shown.

Gages located at A, B, and C indicate the following strains:

5 2500 m P

5 21000 m P

5 2200 m

Knowing that E 5 29 3 10

psi, determine (a) the magnitude of

P, (b) the line of action of P, (c) the corresponding strain at the

hidden edge of the post, where x 5 22.5 in. and z 5 21.5 in.

3 in.

5 in.

Fig. P4.196

bee80288_ch04_220-313.indd Page 309 10/26/10 4:36:50 PM user-f499bee80288_ch04_220-313.indd Page 309 10/26/10 4:36:50 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles

Apago PDF Enhancer

310

Pure Bending

4.197 For the split ring shown, determine the stress at (a) point A,

(b) point B.

90 mm

40 mm

14 mm

2500 N

Fig. P4.197



8 kN · m

200



19.3

5

Fig. P4.198

4.198 A couple M of moment 8 kN ? m acting in a vertical plane is

applied to a W200 3 19.3 rolled-steel beam as shown. Determine

(a) the angle that the neutral axis forms with the horizontal plane,

(b) the maximum stress in the beam.

4.199 Determine the maximum stress in each of the two machine ele-

ments shown.

400 lb

2.5

1.5

0.5

2.5

1.5

0.5

r ⫽ 0.3

(a)

(b)

Fig. P4.199 All dimensions given in inches.

90⬚

Fig. P4.200

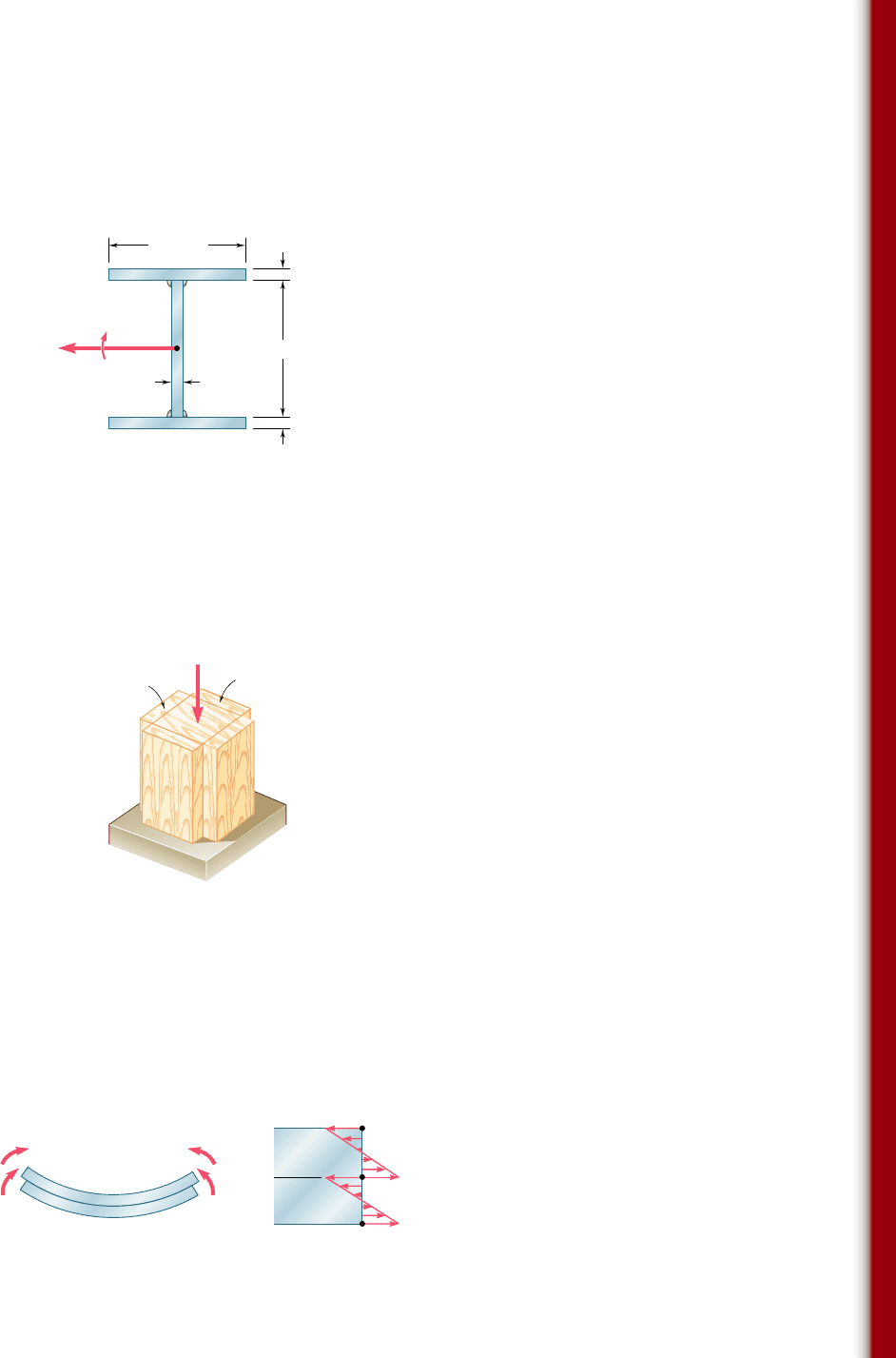
4.200 The shape shown was formed by bending a thin steel plate. Assum-

ing that the thickness t is small compared to the length a of a side

of the shape, determine the stress (a) at A, (b) at B, (c) at C.

bee80288_ch04_220-313.indd Page 310 11/11/10 8:01:54 PM user-f499bee80288_ch04_220-313.indd Page 310 11/11/10 8:01:54 PM user-f499 /Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04/Users/user-f499/Desktop/Temp Work/Don't Delete Job/MHDQ251:Beer:201/ch04

Apago PDF Enhancer

311

Review Problems

10 mm

120 mm

10 mm

120 mm

10 mm

Fig. P4.201

4.201 Three 120 3 10-mm steel plates have been welded together to

form the beam shown. Assuming that the steel is elastoplastic with

E 5 200 GPa and s

5 300 MPa, determine (a) the bending

moment for which the plastic zones at the top and bottom of the

beam are 40 mm thick, (b) the corresponding radius of curvature

of the beam.

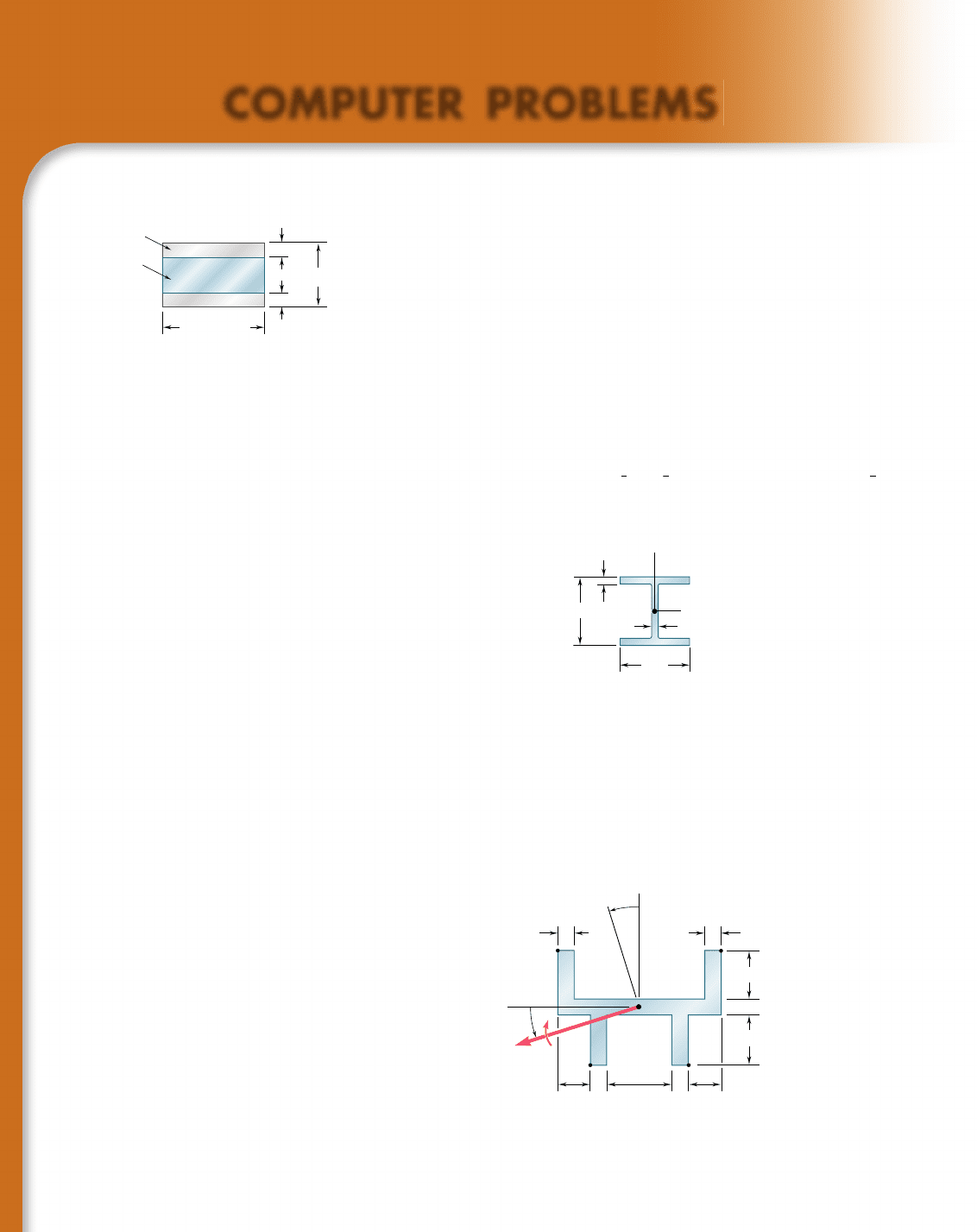
4.202 A short column is made by nailing four 1 3 4-in. planks to a

4 3 4-in. timber. Determine the largest compressive stress created

in the column by a 16-kip load applied as shown in the center

of the top section of the timber if (a) the column is as described,

(b) plank 1 is removed, (c) planks 1 and 2 are removed, (d) planks

1, 2, and 3 are removed, (e) all planks are removed.

16 kips

Fig. P4.202

4.203 Two thin strips of the same material and same cross section are

bent by couples of the same magnitude and glued together. After

the two surfaces of contact have been securely bonded, the couples

are removed. Denoting by s

the maximum stress and by r

the

radius of curvature of each strip while the couples were applied,

determine (a) the final stresses at points A, B, C, and D, (b) the

final radius of curvature.



Fig. P4.203

bee80288_ch04_220-313.indd Page 311 10/26/10 4:37:11 PM user-f499bee80288_ch04_220-313.indd Page 311 10/26/10 4:37:11 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles

Apago PDF Enhancer

312

COMPUTER PROBLEMS

The following problems are designed to be solved with a computer.

4.C1 Two aluminum strips and a steel strip are to be bonded together

to form a composite member of width b 5 60 mm and depth h 5 40 mm.

The modulus of elasticity is 200 GPa for the steel and 75 GPa for the alu-

minum. Knowing that M 5 1500 N ? m, write a computer program to cal-

culate the maximum stress in the aluminum and in the steel for values of a

from 0 to 20 mm using 2-mm increments. Using appropriate smaller incre-

ments, determine (a) the largest stress that can occur in the steel, (b) the

corresponding value of a.

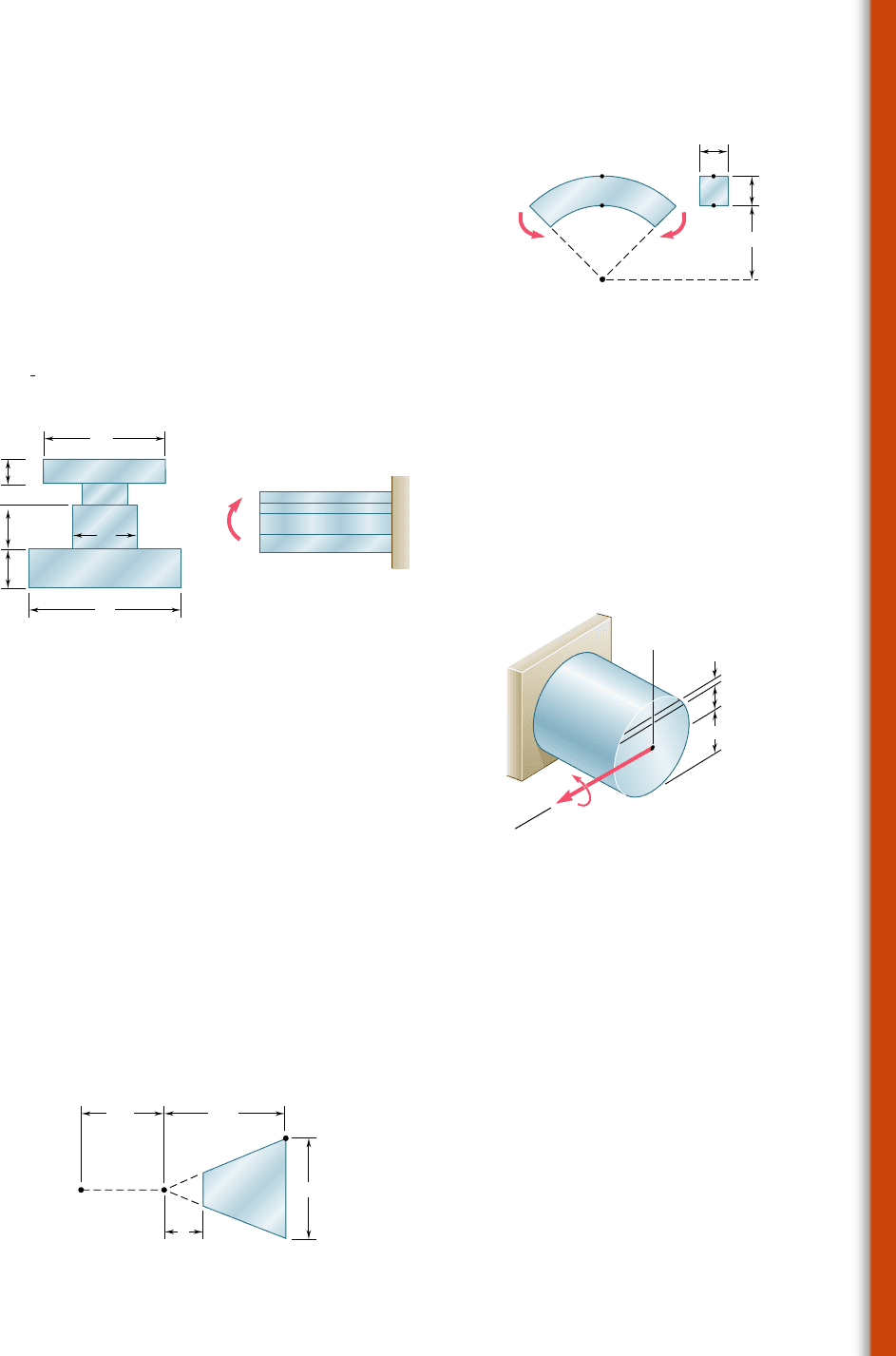
4.C2 A beam of the cross section shown, made of a steel that is assumed

to be elastoplastic with a yield strength s

and a modulus of elasticity E, is

bent about the x axis. (a) Denoting by y

the half thickness of the elastic core,

write a computer program to calculate the bending moment M and the radius

of curvature r for values of y

from

d to

d using decrements equal to

Neglect the effect of fillets. (b) Use this program to solve Prob. 4.201.



60 mm



40 mm

Steel

Aluminum

Fig. P4.C1

Fig. P4.C2

4.C3 An 8-kip ? in. couple M is applied to a beam of the cross section

shown in a plane forming an angle b with the vertical. Noting that the cen-

troid of the cross section is located at C and that the y and z axes are principal

axes, write a computer program to calculate the stress at A, B, C, and D for

values of b from 0 to 1808 using 108 increments. (Given: I

5 6.23 in

and

5 1.481 in

0.40.4

1.6

1.2

0.4

1.2

0.4 0.8

Dimensions in inches

0.40.8



Fig. P4.C3

bee80288_ch04_220-313.indd Page 312 10/26/10 4:37:17 PM user-f499bee80288_ch04_220-313.indd Page 312 10/26/10 4:37:17 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles

Apago PDF Enhancer

313

Computer Problems

4.C4 Couples of moment M 5 2 kN ? m are applied as shown to a

curved bar having a rectangular cross section with h 5 100 mm and b 5

25 mm. Write a computer program and use it to calculate the stresses at

points A and B for values of the ratio r

/h from 10 to 1 using decrements

of 1, and from 1 to 0.1 using decrements of 0.1. Using appropriate smaller

increments, determine the ratio r

/h for which the maximum stress in the

curved bar is 50% larger than the maximum stress in a straight bar of the

same cross section.

4.C5 The couple M is applied to a beam of the cross section shown.

(a) Write a computer program that, for loads expressed in either SI or

U.S. customary units, can be used to calculate the maximum tensile and

compressive stresses in the beam. (b) Use this program to solve Probs. 4.10,

4.11, and 4.192.

Fig. P4.C4

Fig. P4.C5

4.C6 A solid rod of radius c 5 1.2 in. is made of a steel that is assumed

to be elastoplastic with E 5 29,000 ksi and s

5 42 ksi. The rod is subjected

to a couple of moment M that increases from zero to the maximum elastic

moment M

and then to the plastic moment M

. Denoting by y

the half

thickness of the elastic core, write a computer program and use it to calcu-

late the bending moment M and the radius of curvature r for values of y

from 1.2 in. to 0 using 0.2-in. decrements. (Hint: Divide the cross section

into 80 horizontal elements of 0.03-in. height.)

4.C7 The machine element of Prob. 4.182 is to be redesigned by remov-

ing part of the triangular cross section. It is believed that the removal of a

small triangular area of width a will lower the maximum stress in the ele-

ment. In order to verify this design concept, write a computer program to

calculate the maximum stress in the element for values of a from 0 to 1 in.

using 0.1-in. increments. Using appropriate smaller increments, determine

the distance a for which the maximum stress is as small as possible and the

corresponding value of the maximum stress.

3 in.2 in.

2.5 in.C

Fig. P4.C7

y

Fig. P4.C6

bee80288_ch04_220-313.indd Page 313 10/26/10 4:37:28 PM user-f499bee80288_ch04_220-313.indd Page 313 10/26/10 4:37:28 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles

Apago PDF Enhancer

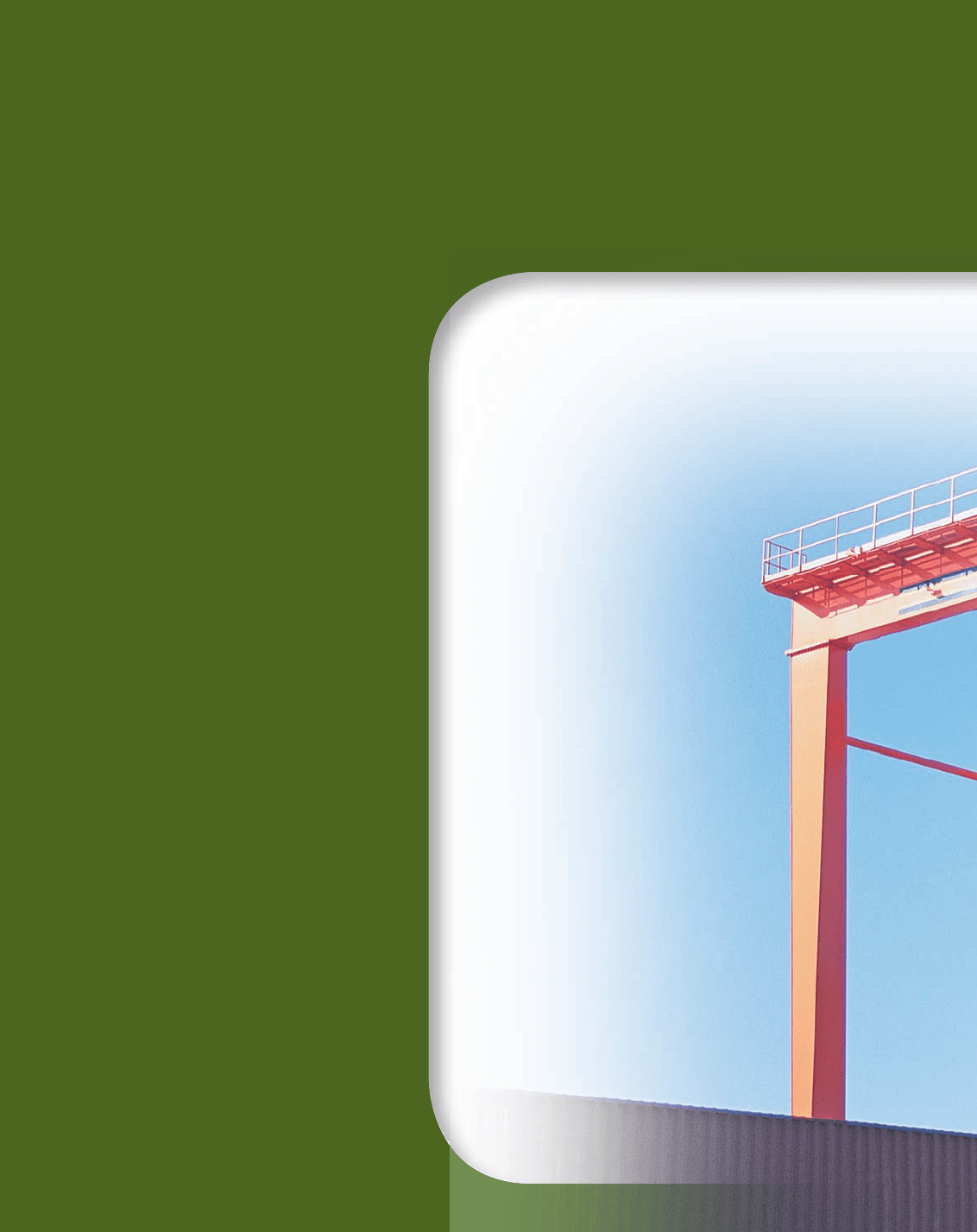
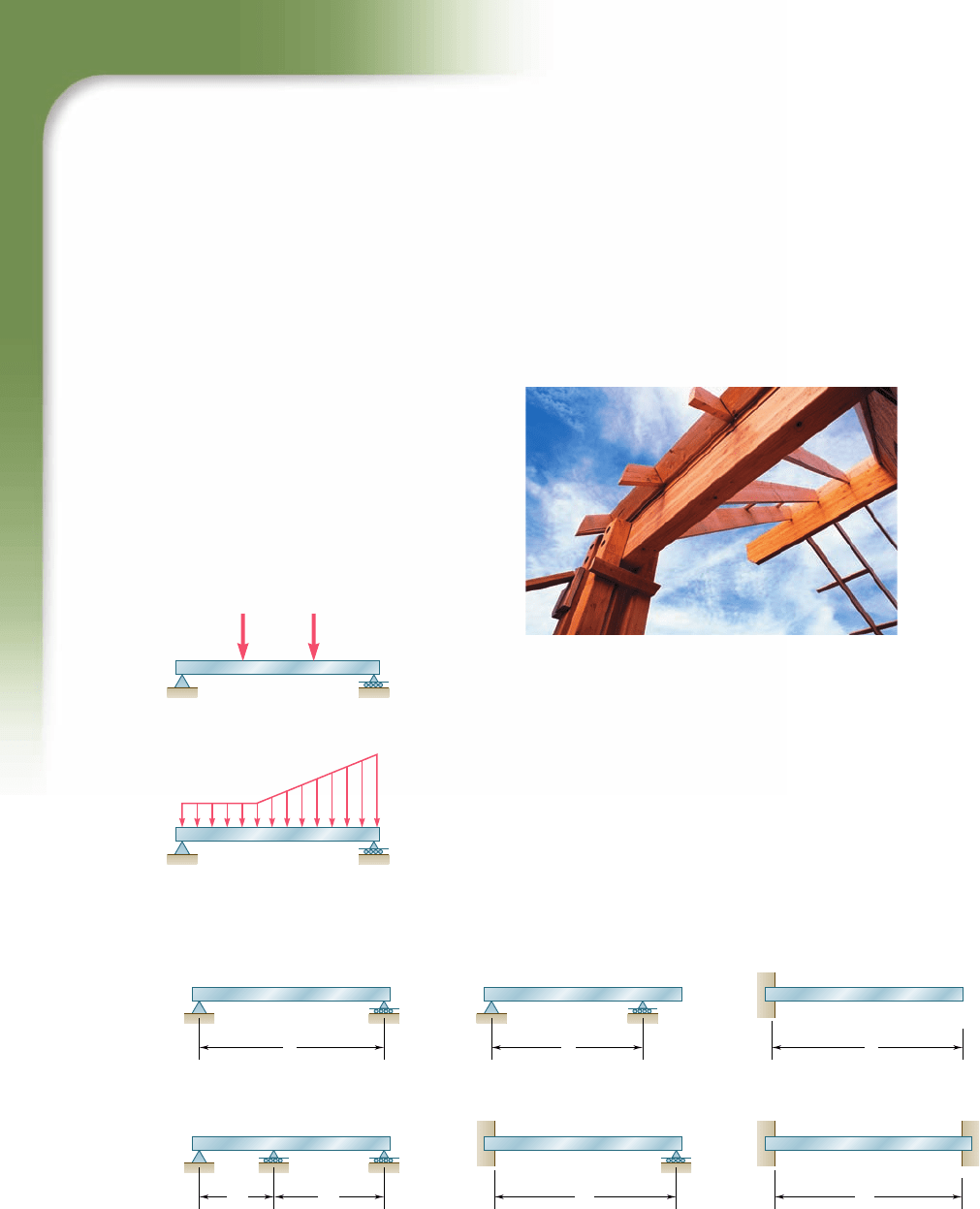
The beams supporting the multiple

overhead cranes system shown in this

picture are subjected to transverse

loads causing the beams to bend. The

normal stresses resulting from such

loadings will be determined in this

chapter.

314

bee80288_ch05_314-379.indd Page 314 10/27/10 9:48:37 PM user-f499bee80288_ch05_314-379.indd Page 314 10/27/10 9:48:37 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles

Apago PDF Enhancer

CHAPTER

315

Analysis and Design

of Beams for Bending

bee80288_ch05_314-379.indd Page 315 10/27/10 9:50:57 PM user-f499bee80288_ch05_314-379.indd Page 315 10/27/10 9:50:57 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles

Apago PDF Enhancer

316

Chapter 5 Analysis and Design

of Beams for Bending

5.1 Introduction

5.2 Shear and Bending-Moment

Diagrams

5.3 Relations Among Load, Shear,

and Bending Moment

5.4 Design of Prismatic Beams for

Bending

*5.5 Using Singularity Functions to

Determine Shear and Bending

Moment in a Beam

*5.6 Nonprismatic Beams

5.1 INTRODUCTION

This chapter and most of the next one will be devoted to the analysis

and the design of beams, i.e., structural members supporting loads

applied at various points along the member. Beams are usually long,

straight prismatic members, as shown in the photo on the previous

page. Steel and aluminum beams play an important part in both struc-

tural and mechanical engineering. Timber beams are widely used in

home construction (Photo 5.1). In most cases, the loads are perpen-

dicular to the axis of the beam. Such a transverse loading causes only

bending and shear in the beam. When the loads are not at a right

angle to the beam, they also produce axial forces in the beam.

Photo 5.1 Timber beams used in residential

dwelling.

(a) Concentrated loads

(b) Distributed load

Fig. 5.1 Transversely loaded

beams.

The transverse loading of a beam may consist of concentrated

loads P

, P

, . . . , expressed in newtons, pounds, or their multiples,

kilonewtons and kips (Fig. 5.1a), of a distributed load w, expressed

in N/m, kN/m, lb/ft, or kips/ft (Fig. 5.1b), or of a combination of

both. When the load w per unit length has a constant value over part

of the beam (as between A and B in Fig. 5.1b), the load is said to

be uniformly distributed over that part of the beam.

Beams are classified according to the way in which they are

supported. Several types of beams frequently used are shown in

Fig. 5.2. The distance L shown in the various parts of the figure is

Fig. 5.2 Common beam support configurations.

(a) Simply supported beam

Statically

Determinate

Beams

Statically

Indeterminate

Beams

(d) Continuous beam

(b) Overhanging beam

Beam fixed at one end

and simply supported

at the other end

(e)

( f ) Fixed beam

bee80288_ch05_314-379.indd Page 316 10/29/10 7:07:38 PM user-f499bee80288_ch05_314-379.indd Page 316 10/29/10 7:07:38 PM user-f499 /Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles/Volumes/201/MHDQ251/bee80288_disk1of1/0073380288/bee80288_pagefiles